Which polynomial function has a leading coefficient of 2, root –4 with multiplicity 3, and root 10 with multiplicity 1?
f(x) = 2(x – 4)(x – 4)(x – 4)(x + 10)
f(x) = 2(x + 4)(x + 4)(x + 4)(x – 10)
f(x) = 3(x – 4)(x – 4)(x + 10)
f(x) = 3(x + 4)(x + 4)(x – 10)

El número "Novecientos mil cuatrocientos ochenta y nueve" se representa como 900,489 en notación numérica.

Por otro lado, el número "cuarenta mil dos" se representa como 40,002.

Si estamos buscando determinar cuál de estos dos números es mayor, podemos comparar las cifras en cada posición.

Comenzando desde la izquierda, el primer dígito de 900,489 es 9, mientras que el primer dígito de 40,002 es 4.

Dado que 9 es mayor que 4, podemos concluir que 900,489 es mayor que 40,002.

En general, al comparar números, se debe observar cada posición en orden de mayor a menor importancia.

Esto significa que el primer dígito a la izquierda es el más significativo y tiene más peso en el valor total del número.

Si los dígitos en la posición más significativa son iguales, se debe pasar a la siguiente posición hasta que se encuentre una diferencia.

En este caso, dado que el primer dígito de 900,489 es mayor que el primer dígito de 40,002, no es necesario comparar los dígitos en posiciones posteriores.

Por lo tanto, podemos concluir que el número "Novecientos mil cuatrocientos ochenta y nueve" (900,489) es mayor que "cuarenta mil dos" (40,002).

For similar questions on Novecientos

https://brainly.com/question/1753221

#SPJ8

Answer:

z = \(\frac{1}{2}\) xy

Step-by-step explanation:

given z is directly proportional to xy then the equation relating them is

z = kxy ← k is the constant of proportion

to find k use the condition z = 21 when x = 6 and y = 7

21 = k × 6 × 7 = 42k ( divide both sides by 42 )

\(\frac{21}{42}\) = k , that is

k = \(\frac{1}{2}\)

equation relating them is

z = \(\frac{1}{2}\) xy

The measure of the angle s 50. 2 degrees

To determine the angle, we need to know the different trigonometric identities.

These trigonometric identities are;

From the information given, we have that;

The ratios of the identities are;

cos θ = adjacent/hypotenuse

tan θ = opposite/adjacent

sin θ = opposite/hypotenuse

Then, we have that;

cos A = 16/25

divide the values

cos A = 0. 64

Find the inverse

A = 50. 2 degrees

Learn about trigonometric identities at: https://brainly.com/question/22591162

#SPJ1

Answer:

x > 8

Step-by-step explanation:

When you divide or multiply both sides of an inequality by a negative number, the equality is switched.

-2x + 3 < -13

(-2x + 3) - 3 < -13 - 3

-2x < -16

-2x/-2 < -16/-2

x > 8

Answer:

x > 8

Step-by-step explanation:

In order to solve the inequality for x, we must get x by itself on one side of the equation.

-2x + 3 < -13

3 is being added to -2x. The inverse of addition is subtraction. Subtract 3 from both sides of the equation.

-2x + (3-3) < (-13-3)

-2x < -13 -3

-2x < -16

x is being multiplied by -2. The inverse of multiplication is division. Divide both sides of the equation by -2.

-2x/-2 < -16/-2

When dividing or multiplying by a negative number, remember to flip the inequality sign.

-2x/-2 > -16/-2

x > -16/-2

x > 8

The solution to the inequality is x>8

Answer: Step-by-step explanation:

To find the average rate of change of a function over an interval, we can use the following formula:

average rate of change = (y2 - y1)/(x2 - x1)

Where x1 and x2 are the values of x at the beginning and end of the interval, and y1 and y2 are the corresponding values of the function at those points.

In this case, we are asked to compare the average rate of change of the functions g(x) and f(x) over the interval where -1≤x≤3.

For the function g(x) = x²+6x, we can plug in the given values for x1, x2, y1, and y2 to find the average rate of change:

average rate of change = (g(3) - g(-1))/(3 - (-1))

= (9 + 18 - (1 - 6))/(4)

= 27/4

= 6.75

For the function f(x) = 2, we can plug in the given values for x1, x2, y1, and y2 to find the average rate of change:

average rate of change = (f(3) - f(-1))/(3 - (-1))

= (2 - 2)/(4)

= 0

Since the average rate of change of the function g(x) is greater than the average rate of change of the function f(x), the function g(x) has a greater average rate of change over the interval where -1≤x≤3.

I hope this helps clarify the comparison of the average rate of change for these two functions. Do you have any other questions?

Step-by-step explanation:

The definite integral of sin^5(x)dx from 0 to pi/2 can be evaluated using the method of substitution.

Let u = sin(x), then du = cos(x)dx

The integral becomes:

∫sin^5(x)dx = ∫u^5du from 0 to sin(π/2)

= (u^6)/6 evaluated at sin(π/2) and 0

= (sin^6(π/2))/6 - 0

= (1^6)/6

= 1/6

So, the definite integral of sin^5(x)dx from 0 to pi/2 is equal to 1/6.

Answer:

Step-by-step explanation:

198 is not a perfect square

All the given numbers are perfect squares except 198

The given numbers:

To find:

A perfect square is obtained by multiplying two equal integers

The perfect squares obtained by multiplying two equal integers are shown below.

The non-perfect square is shown below

Thus, all the given numbers are perfect squares except 198

Learn more here: https://brainly.in/question/4881002?tbs_match=3

Answer:

They are both 42 cm

Step-by-step explanation:

Answer:

  30 ft

Step-by-step explanation:

The Pythagorean theorem can be used to find the length of the unknown side. It tells you the sum of the squares of the legs is equal to the square of the hypotenuse.

  12² +a² = 13²

  a² = 169 -144 = 25

  a = √25 = 5

The sum of the side lengths is ...

  (12 +5 +13) ft = 30 ft

The perimeter is 30 feet.

Answer: 32 ounces

Step-by-step explanation:

1 pint = 16 ounces, 16 x 2 = 32 ounces.

Answer:

First term = 64

Step-by-step explanation:

We are given the formula for the nth term of a geometric series to be;

S_n = (a1(1 - rⁿ))/(1 - r)

Where:

S_n is the sum of n terms

A1 is first term

r is common ratio

We are given;

S_112 = 256

r = ¾

Thus;

256 = a1(1 - ¾^(112))/(1 - ¾)

256 = a1(4)

a1 = 256/4

a1 = 64

Answer:

8:05 or 8:10

Step-by-step explanation:

6:35 plus 60 minutes 6:95 then change it to 7:60 to 8:05 pm or 8:10 pm

the answer is 8:05 to 8:10

Answer:

8:10

Step-by-step explanation:

The subsets of the set {e, n, t} are given as follows:

{{}, {e}, {n}, {t}, {e,n}, {e,t}, {n,t}, {e,n,t}}.

Considering a set with n elements, the number of subsets in the set is the nth power of 2, that is:

\(2^n\)

The set for this problem is given as follows:

{e, n, t}

The cardinality is given as follows:

n = 3.

Hence the number of subsets is given as follows:

2³ = 8.

The subsets are given as follows:

{{}, {e}, {n}, {t}, {e,n}, {e,t}, {n,t}, {e,n,t}}.

In which {} is the empty set.

More can be learned about the subsets of a set at brainly.com/question/13458417

#SPJ1

Answer:

recycled: 10 pounds

cardstock: 14 pounds

Explanation:

Let's call x the weight of one ream of recycled paper and y the weight of one ream of cardstock.

If 3 reams of recycled paper and 5 reams of cardstock weigh 100 pounds, we can write the following equation

3x + 5y = 100

Additionally, if 7 reams of recycled paper weigh as much as 5 cardstock, we get

7x = 5y

So, taking into account the last equation, we can replace 5y by 7x in the first equation

3x + 5y = 100

3x + 7x = 100

Then, we can solve for x

10x = 100

10x/10 = 100/10

x = 10

Finally, we can replace x = 10 on 7x = 5y and solve for y as follows

7x = 5y

7(10) = 5y

70 = 5y

70/5 = 5y/5

14 = y

Therefore, the weight of one ream of each type of paper is

recycled: 10 pounds

cardstock: 14 pounds

Answer:

75 degrees

Step-by-step explanation:

Isosceles triangle with 3rd angle being 30 degrees:

2x + 30 = 180

2x = 150

X = 75

The slope of the given line ( 2 / x ) + ( 3 / y ) = 0 which is calculated by solving the equation is equal to ( - 3 / 2).

A line's slope is defined as the ratio of the change in y coordinate to the change in x coordinate.

The net change in y-coordinate is represented by Δy and the net change in x-coordinate is represented by Δx.

Hence, the expression for the change in y-coordinate with respect to the change in x-coordinate is,

m = y/x = y/x = change in y/change in x

where "m" represents a line's slope.

Furthermore, the slope of the line can be shown as

tan θ = Δy/Δx

So, tan θ to be the slope of a line.

As given in the question,

Given equation of the line is equal to ( 2 / x ) + ( 3 / y ) = 0

Simplify the given equation to get the slope intercept form :

( 2 / x ) + ( 3 / y ) = 0

⇒ ( 2 / x ) = - ( 3 / y )

⇒ 2y = -3x

⇒ y = ( - 3 / 2 ) x + 0  __(1)

Standard slope intercept form of the line is given by :

y = mx + c  __(2)

Where m is the slope of the line

Compare (1) and (2) to get the slope of the line we get,

Slope of the line 'm' = ( - 3 / 2 )

Therefore, the slope of the given line by simplify the equation

( 2 / x ) + ( 3 / y ) = 0 in slope intercept form is equal to ( - 3/2).

Learn more about slope here:

brainly.com/question/3605446

#SPJ4

Yes, because for each input there is exactly one output. Therefore, the relation represented by the mapping diagram is a function.

To determine if the relation represented by the given mapping diagram is a function, we need to check if each input (x value) has exactly one output (y value) associated with it.

From the given mapping diagram, we can see that:

The input value -3 is associated with the output value 0.

The input value -1 is associated with the output value 2.

The input value 1 is associated with the output value 0.

The input value 3 is associated with the output value 2.

The input value 5 is associated with the output value 5.

As we can see, each input value has exactly one output value associated with it. Therefore, the relation represented by the mapping diagram is a function.

for such more question on mapping diagram

https://brainly.com/question/27991506

#SPJ11

Answer:

Step-by-step explanation:

Let d represent the number of dimes. Then d-9 is the number of nickels. The total value (in cents) is ...

  10d +5(d-9) = 180

  15d -45 = 180 . . . . . simplify

  d -3 = 12 . . . . . . . . . .divide by 15

  d = 15

  15 -9 = 6 = number of nickels

Sue has 15 dimes and 6 nickels.

Answer:

15 dimes, 6 nickels

Step-by-step explanation:

D = # of dimes, and N = # of nickels

10D + 5N = 180

D = N + 9

Substitute:

10 (N + 9) + 5N = 180

10N + 90 + 5N = 180

15N = 90

N = 6

D = 15

Answer:

The linear equation satisfying the given condition is,

\(x=t+15\)

where x is Ravi's age and t is the number of years

(after 10 years (t=10) his age becomes 25)

Step-by-step explanation:

Let x be Ravi's age

Now, his  current age is unknown, but if you add 10 to it, then it becomes 25, so

x + 10 =25

so, his current age is x = 25 =10

x = 15

Now, we find the linear equation satisfying the given condition,

Let t be the number of years that have passed since the present time

so, if 0 years have passed, his age will be 15 years

after 1 year, his age will be 16 years

after 10 years, his age will be 25 years and so on

so

\(x = t + 15\)

this satisfies the given condition, since if we add ten years i.e t = 10,

then we get 25

The equation is:

Work/explanation:

Here's the linear equation for Ravi's age.

Ravi's age is x.

If you add 10 to x you get x + 10.

That gives 25.

So the linear equation is x + 10 = 25.

The dot product of vectors a and b is option D. 30.

To find the dot product of two vectors, you need to multiply their corresponding components and then sum the results.

Let's calculate the dot product of vectors a = <5, 2> and b = <4, 5>:

a • b = (5 x 4) + (2 x 5)

= 20 + 10

= 30

Therefore, the dot product of vectors a and b is 30.

learn more about product of vectors: https://brainly.com/question/30394406

#SPJ1

The required equations can be used to model the situation is x = 1/2y - 3 and y = 10(x-1)

What is equation ?

An equation is a mathematical statement that proves two mathematical expressions are equal in algebra, and this is how it is most commonly used. In the equation 3x + 5 = 14, for instance, the two expressions 3x + 5 and 14 are separated by the symbol "equal."

Equations can be classified as either conditional equations or identities. Any value of the variables results in an identity being true. Only certain values of the variables in a conditional equation result in truth. When two expressions are joined together by the equals sign ("="), the result is an equation.

If the smaller number is 3 less than half a larger number, this is expressed as:

x = 1/2y - 3 ⇒(1)

If the larger number is 10 times 1 less than the smaller number, this is expressed as;

y = 10(x-1) ⇒ (2)

Substitute equation 2 into 1 as shown;

x = 1/2y - 3

x = 1/2(10x-10)- 3

x = 5x - 5 - 3

-4x = -8

x = 2

The required equations can be used to model the situation is x = 1/2y - 3 and y = 10(x-1)

To learn more about equation from the given link  

https://brainly.com/question/2972832

#SPJ1

Answer:

a d

Step-by-step explanation:

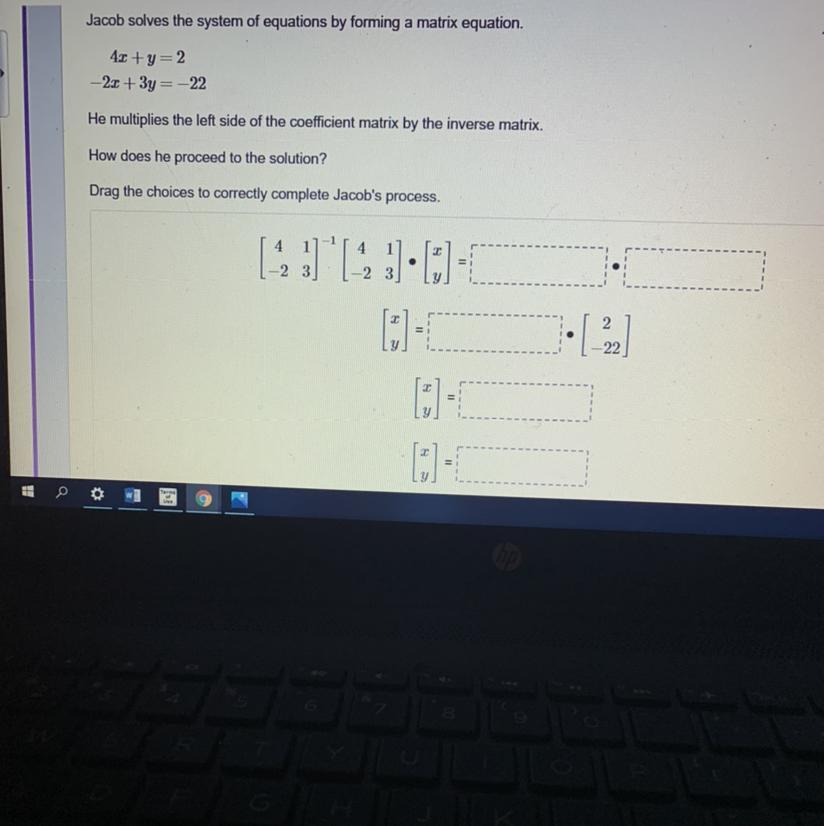
The inverse of a matrix can be calculated as:

Then, let's start by calculating the inverse of the given matrix:

The problem says he multiplies the left side of the coefficient matrix by the inverse matrix, thus:

*These matrices will be the options to put on the first and second boxes.

Then:

Answer:

54 blocks in 18 min

Step-by-step explanation:

3 blocks per min

3x18=54 ur welcome hope this helps

The correct option regarding the normal distribution is given as follows:

2) A value in this interval is not unusual.

The z-score of a measure X of a variable that has mean symbolized by \(\mu\) and standard deviation symbolized by \(\sigma\) is obtained by the rule presented as follows:

\(Z = \frac{X - \mu}{\sigma}\)

The parameters for this problem are given as follows:

\(\mu = 12.601, \sigma = 1.5707\)

The probability is the p-value of Z when X = 13.86 subtracted by the p-value of Z when X = 10.2, hence:

\(Z = \frac{X - \mu}{\sigma}\)

Z = (13.86 - 12.601)/1.5707

Z = 0.8

Z = 0.8 has a p-value of 0.7881.

\(Z = \frac{X - \mu}{\sigma}\)

Z = (10.2 - 12.601)/1.5707

Z = -1.53

Z = -1.53 has a p-value of 0.0630.

Hence:

0.7881 - 0.0630 = 0.7251 > 0.5 -> not unusual.

More can be learned about the normal distribution at https://brainly.com/question/25800303

#SPJ1

Answer:

Side of cube = 4 cm

Step-by-step explanation:

Given:

Volume of cube = 64 cm³

Find:

Side of cube

Computation:

Volume of cube = side³

64 = side³

Side of cube = 4 cm

Answer:

8

Step-by-step explanation:

k - 2 = 6

+2      +2

k = 8

Whenever solving for these your first goal is to get rid of any constants isolating the variable by itself. To get rid of -2 we add 2 to both sides, whatever we do to the left we do to the right and vice versa. When we add 2 to 6 we get 8 and that is how we got our answer.

Answer:

$\(45\)

Step-by-step explanation:

\(30\)% \(\mathrm{of}\) $\(150\)

\(=\frac{30}{100}\times 150\\\)

\(=\) $\(45\)