Find the x intercepts of the graph. ( enter your answer as a comma separated list. Use n as an integer constant.) y=sin pix over 2 plus 1
X=

Answers
The x-intercept of the graph are - 1 and 3.
What is x-intercept?The intersection of a function's graph and the x-axis is known as the x-intercept in mathematics. The function has a root or a solution for the equation f(x) = 0 at this time since the y-coordinate of the graph is zero.
The zero or function's root are other names for the x-intercept. The x-value for which the function f(x) equals zero is known as the zero x value.
When a function has an x-intercept, we set f(x) = 0 and then solve for x. The value(s) of x that are obtained provide the x-coordinate(s) of the point(s) where the function's graph crosses the x-axis.
The x-intercept of the graph is obtained when the value of the y-coordinate is 0.
Thus, from the graph we see that the x-intercept is at the points -1 and 3.
Hence, the x-intercept of the graph are - 1 and 3.
Learn more about x-intercept here:
https://brainly.com/question/14180189
#SPJ1
Related Questions
I would appreciate an answer!
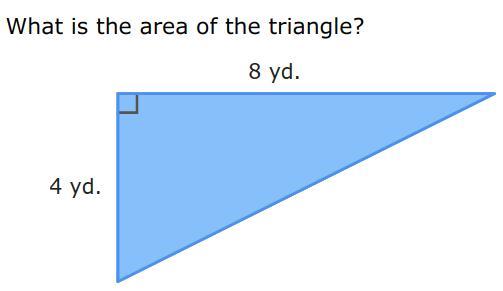
Answers
Answer:
Step-by-step explanation:
so for the area of a triangle, it is base x hight and all divided by 2. Therefore it is 8 x 4 = 32 32/2 = 16
the area of the triangle is 16
Answer:
16 yd²
Step-by-step explanation:
In this question, we need to find the area of the triangle.
To find the area, we would use the equation A = 1/2bh or A = b·h/2
Plug in and solve:
A = 4·8/2
A = 32/2
A = 16
The area of the triangle is 16 yd²
Don't forget that the unit is squared when its related to the area of a figure.
?? look at the picture attached

Answers
Answer:
C
Step-by-step explanation:
H( -4 , -7) &G (2,9)
m:n = 5 :3
\(\left( \dfrac{mx_{2}+nx_{1}}{m+n} , \dfrac{my_{2}+ny_{1}}{m+n} \right)\\\\\\=\left(\dfrac{10-12}{8},\dfrac{45-21}{8} \right)\\\\\\=\left(\dfrac{-2}{8},\dfrac{24}{8}\right)\\\\\\\)
= (-0.25, 3)
A runner gets a 30.0 meter head start then runs 5.0km/hr. Write an equation which will represent the runners distance from the start line at any second
Answers
Answer:
d = 1.4 t + 30
Step-by-step explanation:
Head start means any advantage achieved or advantage gained in any race or competition.
In the context, the runner is gained a head start of 30 meter from the starting point.
It is also given that the speed with which the runner is running is 5.0 km per hour.
Therefore, the equation that gives the distance covered by the runner from his start line at any time in seconds is given by d = 1.4 t + 30.
A spiral staircase turns as it rises 10 feet. The radius of the staircase is 3 feet. What is the number of feet in the length of the handrail
Answers
The number of feet in the length of the handrail is 17.3 feet.
What is a Pythagorean Theorem?Pythagorean theorem, is a geometric theorem that the total of a squares on the sides of a right triangle equals the square upon that hypotenuse (a side opposite its right angle)—or, using familiar algebraic notation, the sum of squared on the hypotenuse equals the square also on hypotenuse.
H² = B² + P²
A spiral would be a shape that wraps around and around with each curve being higher or lower than the one before it.
Now, according to the question;
The simplest way to understand this is to assume that we may "unfold" this spiral to form a rectangle.
Its bottom part of rectangle is simply an arc length of a 3 ft radius circle spun around 270°= (3/4) of a full revolution.
Thus,
= (3/4)× (2\(\pi\)) ×(3)
= (9/2)×\(\pi\) ft
= 4.5×\(\pi\) ft
The height of the staircase will be the side of rectangle = 10ft.
And the rectangle's diagonal will correspond to the length of a railing.
To solve this, we can apply the Pythagorean Theorem.
Handrail length = √ [ (4.5 ×\(\pi\))² + 10² ]
Handrail length ≈ 17.3 ft
Therefore, the the number of feet in the length of the handrail is 17.3 feet.
To know more about Pythagorean Theorem, here
https://brainly.com/question/343682
#SPJ4
The complete question is-
A spiral staircase turns 270° as it rises 10 feet. The radius of the staircase is 3 feet. What is the number of feet in the length of the handrail?
Calculate the missing angle and give a reason for your answer

Answers
Answer:
c = 106°
Step-by-step explanation:
The unknown angle and c° form a straight line which is 180°
So, c° + unknown angle = 180°
Remember, the sum of interior angles of a trapezoid is 360°
So, 126° + 108° + 52° + unknown angle = 360°
unknown angle = 360° - 126° + 108° + 52°
unknown angle = 74°
Now, c° + 74° = 180°
c = 180° - 74°
c = 106°
carbon-14 decays continuously at a rate of 0.0121% a year. if a 5-gram sample currently weighs 3.2-grams, how old is the sample?
Show your work
Answers
Answer:
Step-by-step explanation:
if 1 = 2 than 3 is 4

Find the volume of the solid. PLEASE HELPPPPPPPP

Answers
Answer:
V≈743.25
Step-by-step explanation:
V=5
12tan(54°)ha2=5
12·tan(54°)·4·182≈743.24624
A normal distribution has μ = 30 and Ï = 5.
(a) Find the z score corresponding to x = 25.
(b) Find the z score corresponding to x = 42.
(c) Find the raw score corresponding to z = â3.
(d) Find the raw score corresponding to z = 1.5.
Answers
(a) The z-score corresponding to x = 25 is -1. (b)The z-score corresponding to x = 42 is 2.4.(c) The raw score corresponding to z = -3 is 15. (d) The raw score corresponding to z = 1.5 is 37.5.
For a normal distribution with mean μ = 30 and standard deviation σ = 5:
(a) To find the z-score corresponding to x = 25, we use the formula:
z = (x - μ) / σ
Substituting the values, we get:
z = (25 - 30) / 5 = -1
Therefore, the z-score corresponding to x = 25 is -1.
(b) To find the z-score corresponding to x = 42, we again use the formula:
z = (x - μ) / σ
Substituting the values, we get:
z = (42 - 30) / 5 = 2.4
Therefore, the z-score corresponding to x = 42 is 2.4.
(c) To find the raw score (x) corresponding to z = -3, we use the formula:
z = (x - μ) / σ
Rearranging the formula, we get:
x = μ + zσ
Substituting the values, we get:
x = 30 + (-3) x 5 = 15
Therefore, the raw score corresponding to z = -3 is 15.
(d) To find the raw score (x) corresponding to z = 1.5, we use the same formula:
x = μ + zσ
Substituting the values, we get:
x = 30 + 1.5 x 5 = 37.5
Therefore, the raw score corresponding to z = 1.5 is 37.5.
To know more about z-score click here
brainly.com/question/31613365
#SPJ11
Adam, Chris, and jason have 2 1/2, 3 1/3, and 1/6 pounds of fish, respectively, to bring to the fish fry. how many pounds of fish do they have combined. 
Answers
Answer:
7lbs
Step-by-step explanation:
State whether the variable is discrete or continuous: The cost of a Statistics book.* discrete continuous both neither 3 statistics professors and 7 chemistry professors are available to be advisors to a student organization. The student organization needs two of the professors to be advisors, what is the probability that both professors are chemistry professors? * 0.111 0.233 O 0.1 0.467
Answers
The probability that both professors are chemistry professors is 0.467.
The cost of a Statistics book is a continuous variable because it can take any value within a given range, including decimals, and is not limited to specific, separate values.
For the probability question, there are 10 professors in total (3 statistics + 7 chemistry), and the organization needs 2 advisors. The probability of choosing two chemistry professors can be calculated using the formula: P(Chemistry) = (Number of Chemistry professors) / (Total Number of professors).
First, find the probability of selecting a chemistry professor for the first advisor:
P(First Chemistry) = 7/10.
Next, find the probability of selecting a chemistry professor for the second advisor, considering one chemistry professor has already been selected:
P(Second Chemistry) = 6/9.
Now, multiply both probabilities together to get the probability of selecting two chemistry professors:
P(Both Chemistry) = P(First Chemistry) × P(Second Chemistry) = (7/10) × (6/9) = 0.467.
So, the probability that both professors are chemistry professors is 0.467.
Visit here to learn more about probability : https://brainly.com/question/30034780
#SPJ11
A car can cover a distance of 522 km on 36 liters of petrol. How far can it travel on 14 liters of petrol?
Answers
522km / 36= 14.5km PER litre
14.5 x 14= 203
Angles 2 and 8 are what type of angles?
A. Same side interior angles
B. Alternate exterior angles
C. Alternate interior angles
D. Corresponding angles
Help!!!

Answers
Answer:
B. Alternate exterior angles
Step-by-step explanation:
Alternate exterior angles are angles that are outside two lines intersected by the transversal and are located on opposite sides of the transversal. Also, they have the same degree! So, the answer is B.
What is the inverse of the function f(x) = 2x + 1?
h(x) = one-halfx – one-half
h(x) = one-halfx + one-half
h(x) = one-halfx – 2
h(x) = one-halfx + 2
f(–2) = g(–2)
f(0) = g(–2)
f(–2) = g(0)
Answers
The inverse of the function f(x) = 2x+1 is (x-1)/2.
What is inverse of function?The output of a function is returned by the inverse function, which also returns the initial value. Consider the inverse relationship between the functions f and g: f(g(x)) = g(f(x)) = x. The initial value is fetched via a function that is its inverse.
Given;
Function, f(x) = 2x + 1.
To find the inverse of the function:
First, let y = f(x)
That is,
y = 2x +1
Then, make x the subject of the equation
y = 2x + 1
To make x the subject of this equation:
First, subtract 1 from both sides
We get
y -1 = 2x
Now, divide both sides by 2.
x = (y-1)/2
Now, rewrite as f⁻¹(x) by replacing y by x.
That is,
f⁻¹(x) = (x-1)/2
Therefore, the inverse of the function f(x) is (x-1)/2.
To learn more about the inverse of function;
https://brainly.com/question/17872426
#SPJ1
find center and radius with all steps x^2-6x+y^2+4y=12
Answers
The center of the circle is (3, -2) and the radius is 5.
To find the center and radius of the circle given by the equation x^2 - 6x + y^2 + 4y = 12, we need to complete the square for both the x and y terms.
First, let's complete the square for the x terms:
x^2 - 6x = x^2 - 6x + 9 - 9 = (x - 3)^2 - 9
Next, let's complete the square for the y terms:
y^2 + 4y = y^2 + 4y + 4 - 4 = (y + 2)^2 - 4
Now we can substitute these expressions back into the original equation:
(x - 3)^2 - 9 + (y + 2)^2 - 4 = 12
Simplifying:
(x - 3)^2 + (y + 2)^2 = 25
So the equation is now in standard form, (x - h)^2 + (y - k)^2 = r^2, where the center is (h, k) and the radius is r. Therefore, we can see that the center of the circle is (3, -2) and the radius is sqrt(25) = 5.
Therefore, the center of the circle is (3, -2) and the radius is 5.
Learn more about circle here
https://brainly.com/question/28162977
#SPJ11
with nothing but nickles and pennies in her pocket Rupert can afford 77 cents worth of candy if he has 21 coins in her pocket how many coins is in his pocket how many coins dose he have of each ???
Answers
Answer:
no one has give the answer cause it is only 7 point English question you have point 50 e at that questions people are trying to solve that but they can't coz 2 people has already answered your question Ola hunger off points
Step-by-step explanation:
I don't know this answer if I know then I'll tell you please mark as a brainlist
21 people travelled to a meeting.
12 used a train.
6 used a car.
7 did not use a train or a car.
Some used a train and a car.
Two people are chosen at random from those who used a train.
Find the probability that both these people also used a car.
Answers
Answer:
answer = 1/11
Step-by-step explanation:

The addition rule of probability.
P (T + C) = P(T) + P(C) - P(T ∩ C)
The probability that both these people also used a car given that both of them use a train is 1/11.
What is probability?It is the chance of an event to occur from a total number of outcomes.
The formula for probability is given as:
Probability = Number of required events / Total number of outcomes.
We have,
The number of people who used the train and the car.
P (T U C) = 21 - 7 = 14
P(T) = Probability of the number of people who used the train = 12
P(C) = Probability of the number of people who used the car = 6
From the addition rule of probability.
P (T + C) = P(T) + P(C) - P(T ∩ C)
14 = 12 + 6 - P(T ∩ C)
P(T ∩ C) = 12 + 6 - 14 = 18 - 14 = 4
Now,
Two people are chosen.
P(C/T):
= P(T ∩ C) / P(T)
= \(^4C_2\) / \(^{12}C_2\)
= (4 x 3 / 2) / (12 x 11 / 2)
= 6 / 6 x 11
= 1/11
Thus,
The probability that both these people also used a car given that both of them use a train is 1/11.
Learn more about probability here:
https://brainly.com/question/14099682
#SPJ2
Write an equivalent expression. (x+5)+4
Answers
Answer:
x+9
Step-by-step explanation:
if you expand...you could get x+9, which is the same as the given expression
Peanuts are sold at $6 per kg. Cashew nuts are sold at $12 per kg. How many kilograms of cashew nuts are needed to mix with 12 kg of peanuts to obtain a mixture of $8.4 per kg?
Answers
Answer:
c = 8 kg of cashews
Step-by-step explanation:
hope this helps :)
i thought it was a different type of problem at first, but i realized you're looking for cost per kg. i worked it out keeping the units because i thought that would help make sure that we end up getting our answer in the correct units. feel free to let me know if something's unclear :)

-34 -2p = 8(8 - 2p)
Answers
Answer:
p = 7
Step-by-step explanation:
Look at the picture hope this helps!

Your bill at a restaurant is $68 and you want to leave a %20 tip what is the total amount you will leave
Answers
Answer:
$81.6
Step-by-step explanation:
You multiply 0.2 by 68, in which you will get 13.6, and then you add that to 68.
Find the sum of the interior angles for a hexagon. 540° 720° 900° 1,080°
Answers
The sum of interior Angles of Hexagon is always 720°
Any hexagon's internal angles add up to 720° in all cases. By dividing 720° by 6, we may get the size of each interior angle of a regular hexagon. As a result, we have:
720°÷6 = 120°
In a regular hexagon, each inside angle is 120°.
An example of a regular hexagon with equal-length sides and angles is shown in the diagram below. By multiplying the six 120° angles together, we can demonstrate that the result is 720°.
Therefore, the interior angles of a hexagon are always added up to 720°.
Learn more about hexagon :
brainly.com/question/3295271
#SPJ4

Please Help me with this (ASAP) 20 POINTS

Answers
The value of y when x = -2, 0,2 is 1/49, 7, 49.
Simplification is an algebraic expression is when we utilize a range of approaches to make algebraic expressions more efficient and compact - in their simplest form - without changing the value of the original expression.
Given function y =\(7^{x}\)
We have to determine the y values when x = -2, 0, 2
Now substitute the x values in the given function
If x = -2 then
Y = 7(-2)
Y = 1/49
If x = 0 then
Y = 7(0)
Y = 1
If x = 2 then
Y = 7(2)
Y = 49
Therefore the value of y when x = -2, 0 ,2 is 1/49, 7 , 49
To learn more about simplifications visit
https://brainly.com/question/4450673
#SPJ1
Historically, the members of the chess club have had an average height of 5′ 6′′ with a standard deviation of 2". What is the probability of a player being between 5′ 2′′ and 5' 6"? (Submit your answer as a whole number. For example if you calculate 0.653 (or 65.3% ), enter 65. )
Answers
The probability of approximately 48% that a player will have a height between 5'2" and 5'6".
To calculate the probability of a player being between 5'2" and 5'6" given that historically, the members of the chess club have had an average height of 5'6" with a standard deviation of 2", we can use the standard normal distribution.
First, we need to convert the heights to z-scores using the formula:
z = (x - μ) / σ
where x is the height we want to find the probability for, μ is the mean height, and σ is the standard deviation.
For 5'2":
z = (62 - 66) / 2 = -2
For 5'6":
z = (66 - 66) / 2 = 0
Next, we can use a standard normal distribution table or calculator to find the area under the curve between these two z-scores.
Using a standard normal distribution table, we can find that the area to the left of z = -2 is 0.0228 and the area to the left of z = 0 is 0.5. Therefore, the area between z = -2 and z = 0 is:
0.5 - 0.0228 = 0.4772
Multiplying this by 100 gives us a probability of approximately 48% that a player will have a height between 5'2" and 5'6".
To know more about probability refer here:
https://brainly.com/question/11034287#
#SPJ11

Which survey has the best example of a sample error

Answers
A survey of car owners to determine the best bus route. Option C is correct.
Explanations:What are sampling errors?These are errors that are derived from a population estimate using statistics from a given sample. Most sampling errors are caused by lack of precision and bias.
From the given option, we can see that Option C is the best example of sampling error because there are possibilities that car owners are not familiar with bus routes.
if p(x) is divided by (x 1) three times and has remainder of 1 at the end, then -1 is a double root.
Answers
If the polynomial p(x) is divided by (x-1) three times and has a remainder of 1 at the end, then -1 is a double root of p(x). This means that (x+1) is a factor of p(x) raised to the power of 2.
When a polynomial is divided by (x-1), the remainder represents the value of the polynomial at x=1. Since the remainder is 1, it implies that p(1) = 1. Dividing p(x) by (x-1) three times indicates that the polynomial has been factored by (x-1) three times. Consequently, the polynomial can be written as p(x) = (x-1)^3 * q(x) + 1, where q(x) is the quotient obtained after dividing p(x) by (x-1) three times. Since the remainder is 1, it means that when x=1, p(x) leaves a remainder of 1.
Thus, (1-1)^3 * q(1) + 1 = 1, which simplifies to q(1) = 0. This implies that (x-1) is a factor of q(x), meaning that q(x) can be written as q(x) = (x-1) * r(x), where r(x) is another polynomial.
Substituting this into the earlier expression for p(x), we get p(x) = (x-1)^3 * (x-1) * r(x) + 1. Simplifying further, p(x) = (x-1)^4 * r(x) + 1. Now, we can see that p(x) is divisible by (x+1) since (x+1) is a factor of (x-1)^4, and the remainder is 1. Therefore, -1 is a double root of p(x) because (x+1) appears twice in the factored form of p(x).
Learn more about polynomial click here:brainly.com/question/27287760
#SPJ11
The length of a rectangular garden is 555 meters long. The area of the garden is 101010 square meters.
P.S NEED HELP
Answers
Answer:
Step-by-step explanation:
no
PLEASE HELP ANSWER! ! : (
The dot plots show the distribution of heights, in inches, for third grade girls in two classrooms. Which statement is true?
A.
The center of the graph of class 1 is best measured by the median, and the center of the graph of class 2 is best measured by the mean.
B.
The center of the graph of class 1 is best measured by the mean, and the center of the graph of class 2 is best measured by the median.
C.
The centers of the graphs of class 1 and class 2 are best measured by the median.
D.
The centers of the graphs of class 1 and class 2 are best measured by the mean.

Answers
The correct statement is: the center of the graph of class 1 is best measured by the mean, and the center of the graph of class 2 is best measured by the median.
Given is a dot plots show the distribution of heights, in inches, for third grade girls in two classrooms.
The dot plot of class 1 is uneven and that of class 2 is even.
So, the center of the graph will be calculated by mean and that of class 2 by median.
Hence. the correct statement is: the center of the graph of class 1 is best measured by the mean, and the center of the graph of class 2 is best measured by the median.
Learn more about mean and median, click;
https://brainly.com/question/30891252
#SPJ1
if 1,000 points are selected randomly inside the square with all points equally likely to be selected, how many of those points are expected to lie in
Answers
If 1,000 points are selected randomly inside the square with all points equally likely to be selected, points to lie inside the circle is 785.4 points.
We can find the answer by finding the ratio of the areas of the regions and then multiplying by the total number of points.
The square has an area of 1 (since it has sides of length 1).
The circle has a radius of 1/2 and an area of pi/4.
So the ratio of the areas is:
(π/4) / 1 = π/4
Therefore, we would expect:
(π/4) * 1000 = ( 3.14 / 4 ) 1000
(π/4) * 1000 = 1000 × ( 0.785 ) = 785 points
points to lie inside the circle. This is approximately 785 points.
Your question is incomplete but mos probably your full question was
If 1,000 points are selected randomly inside the square with all points equally likely to be selected, how many of those points are expected to lie inside the circle?
Learn more about circle at https://brainly.com/question/24810873
#SPJ11
Ill love u forever if u answer correct Point F is reflected over the y-axis to create F’. Use an ordered pair to name the location of F’, and determine the distance between F and F’.

Answers
Answer:
F' = (-6,3)
Distance = 12 units
Step-by-step explanation:
F is currently at (6,3).
Reflected over the y-axis, it would be at (-6,3).
That puts them 12 units apart.
i really need help i’m going to fail so answer if you can ! <3
The commission of a sales representative can be modeled by C(x) = 4x -
10, where x is the number of items sold. If the sales representative sales 30
items, how much commission will they earn? *
options:
$20
$5
$120
$110
Answers
Answer:
110
Step-by-step explanation:
you place 30 in the place of X, and it becomes 4×30-10. in PEMDAS, multiplication goes first and 4×30=120. 120-10=110