Find the difference 32 1/6 - 19 5/9
Answers
Answer:
Decimal-12.6111111111
Rounded decimal- 12.6
Fraction 1- 126/10
Fraction 2- 63/5
Mixed number- 12 3/5
Related Questions
2p x 3q x 5r in its simplest form
Answers
Answer:
30pqr
Step-by-step explanation:
\( \rm \: 2p \times 3q \times 5r = 2 \times 3 \times 5 \times p \times q \times r\)
Multiplying 2, 3, and 5 gives 30, so we have:
\( \rm \: 2p \times 3q \times 5r = 30 \times p \times q \times r\)
Therefore, the simplest form of 2p x 3q x 5r is 30pqr
i clicked an answer accidentally but i have a lot of HW and i need help!!





Answers
Given
225 is 25 % of a number
Answer
let number be x
\(undefined\)Robin paid a $120 a month on a credit card. She was late on a payment so her monthly bill was raised to $150 a month. which of these shows how Robin can calculate the percent increase she was charged
Answers
Answer: One way to calculate the percent increase in Robin's monthly bill is to use the following formula:
% increase = (new amount - original amount) / original amount x 100
In this case, the original amount is $120 and the new amount is $150. So, the calculation would be:
% increase = (150 - 120) / 120 x 100 = 30 / 120 x 100 = 0.25 x 100 = 25
Therefore, the percent increase in Robin's monthly bill is 25%.
Another way to calculate the percent increase is to use the formula:
(New amount - original amount) / original amount
In this case: (150-120)/120 = 0.25, and multiply by 100 to get 25%
So, both formula gives the same answer 25%
Step-by-step explanation:
Suppose triangle ABC is reflected over the x-axis. If the distance between point A and A’ is 14, what is the distance between the x-axis and A’.
1. 7
2. -7
3. 3.5
4. There is not enough information given.
Answers
Given:
The triangle ABC is reflected over the x-axis.
The distance between point A and A’ is 14.
To find:
The distance between the x-axis and A’.
Solution:
If a figure is reflected across the x-axis then the corresponding parts are mirror image of each other about the x-axis.
It means the distance between A and x-axis is same as the distance between x-axis and A'.
The distance between point A and A’ is 14.
Let d be the distance between the x-axis and A’. Then,
\(d+d=14\)
\(2d=14\)
\(d=\dfrac{14}{2}\)
\(d=7\)
Therefore, the correct option is 1.
Solve the equation. Please help

Answers
\(\cfrac{2y}{3}-\cfrac{3}{4}=\cfrac{1}{12}\implies \stackrel{\textit{multiplying both sides by }\stackrel{LCD}{12}}{12\left( \cfrac{2y}{3}-\cfrac{3}{4} \right)=12\left( \cfrac{1}{12} \right)}\implies 8y-9=1 \\\\\\ 8y=10\implies y=\cfrac{10}{8}\implies y=\cfrac{5}{4}\)
Step 1: Add 3/4 on both sides
We get
2y/3 = 1/12+3/4
We add fractions by calculating a common denominator, adjusting the numerators accordingly and adding them. See this in action below:
12 and 4 have a common multiple in 12 which will be our common denominator. Now, 1/12 does not need to be adjusted, but 3/4 becomes 3*3 / 3*4 = 9/12.
In total 1/12 + 9/12 = 10/12
So, we have 2y/3 = 10/12
Now we multiply both sides by 3, so that the 3 will cancel out the 3 in the denominator on the left side:
2y =30/12
Now we just need to divide by 2 and get the solution:
y = 15/12 = 3*5 / 3*4 = 5/4
Therefore the final answer is A:
The solution set is {5/4}
Which value is a solution to the inequality x 4

Answers
Answer:
0
Step-by-step explanation:
x < 4 means all numbers less than 4, excluding 4 itself.
4.5 > 4.
5 > 4.
so 0 is the solution
Answer:
4
Step-by-step explanation:
interval notation ( negative infinity, 4)
hi I really need help with this

Answers
Answer:
t - 4 ≤ 4
Step-by-step explanation:
Hi I really need help with this
The number line represents t ≤ 8
Since the line is on 8 and moving towards the negative part of the number like (less than)
The equivalent equation will be;
t - 4 ≤ 4
Ads 4 to both sides
t-4+4≤4+4
t ≤ 8
Since the solution is similar to the expression above hence t - 4 ≤ 4 is correct
through: (0, 3) and (-4, -1)
Answers
Answer:
\(m = 1\) -- Slope
\(y = x + 3\) --- Equation
Step-by-step explanation:
Given
\((x_1,y_1) = (0,3)\)
\((x_2,y_2) = (-4,-1)\)
Required
The slope and the equation
The slope (m) is calculated using:
\(m=\frac{y_2 - y_1}{x_2 - x_1}\)
\(m=\frac{-1-3}{-4- 0}\)
\(m=\frac{-4}{-4}\)
\(m = 1\)
The equation is calculated using:
\(y = m(x - x_1) + y_1\)
This gives:
\(y = 1(x - 0) + 3\)
\(y = 1(x) + 3\)
\(y = x + 3\)
help 50 points need help math 7

Answers
Answer:
-5v + 6.2w - 3
Step-by-step explanation:
- (5v - 6.2w) -3
= -5v + 6.2w - 3

What is the volume of a square pyramid with base edges of 18 cm and a slant height of 15 cm?
Answers
Answer:
the volume of the square pyramid is 2430 cubic cm
Answer:
1296 cm³
Step-by-step explanation:
V = a² x [√s²- (a/2)²] / 3
a = 18 cm
s = 15 cm
V = 18² x [√15²-(18/2)²] / 3 = 18² x [√225-81] / 3
V = 324 x (√144/3) = 1296 cm³
The image of B translated using (x + 2, y + 3) would have what coordinates?

Answers
Answer:
(5, 4)
Step-by-step explanation:
(x + 2, y + 3)
Substitute x and y for that of B's position.(3 + 2, 1 + 3)
(5, 4)
Answer:
B' (5, 4 )
Step-by-step explanation:
the translation rule (x, y ) → (x + 2, y + 3 )
means add 2 to the original x- coordinate and add 3 to the original y- coordinate , then
B (3, 1 ) → B' (3 + 2, 1 + 3 ) → B' (5, 4 )
Listed below is a table showing the number of employees. 20 years or older by gender in the United states
Answers
The total number of workers that were studied can be found to be 139,340,000.
The percent of workers unemployed would be 5. 4 %.
Percentage of unemployed men is 5. 6 % and unemployed women is 5. 1%.
How to find the employment figures ?Number of employed workers :
= 74,624,000 + 64, 716, 000
= 139,340,000
Percentage unemployed :
= ( 4, 209,000 + 3,314,000 ) / 139,340,000
= 5. 4 %
Percentage of unemployed men :
= 4,209,000 / 74,624,000
= 5.6 %
Percentage of unemployed women:
= 3,314,000 / 64, 716, 000
= 5. 1 %
Find out more on unemployment at https://brainly.com/question/13280244
#SPJ1
The full question is:
a. How many workers were studied?
b. What percent of the workers were unemployed?
c. Compare the percent unemployed for the men and the women.

What is true about the image ΔQ'R'S'? Select three options.
Which statements are true?
DO,2 (x,y) = (2x, 2y)
Side Q'S' lies on a line with a slope of -1.
QR is longer than Q'R'.
The vertices of the image are closer to the origin than those of the pre-image.
The distance from Q' to the origin is twice the distance from Q to the origin.
Answers
Answer:
\(D_{O,2} (x,y) = (2x, 2y)\)
Side Q'S' lies on a line with a slope of -1.
The distance from Q' to the origin is twice the distance from Q to the origin.
Step-by-step explanation:
The explanations are as follows
As we can see from the attached figure that the ΔQ'R'S' is dilated i.e transformation is made or change in size
Now
Based on
(x,y) = (2x,2y)
The intercepts are as follows
For S
(-1,1) = (-2,2)
For Q
(-3,3) = (-6,6)
For S
(2,4) = (4,8)
Based on this we can say that the three above options are correct

Answer:
A, B, E on edge
Step-by-step explanation:
took the test :)
does a debit card use a pin or a credit card, or do both?
Answers
True or false? The graph represents a function
5
-5
A True
B. False

Answers
Answer:
True
Step-by-step explanation:
It passes the vertical line test, meaning you can draw a vertical line anywhere on the graph and it will only intersect at one point
this means that for every input (x value) there is only one output (y value)
Yes, The graph represents a function.
What is mean by Function?A relation between a set of inputs having one output each is called a function. and an expression, rule, or law that defines a relationship between one variable (the independent variable) and another variable (the dependent variable).
We have to show a graph.
Now, We know that;
The vertical line test, meaning you can draw a vertical line anywhere on the graph and it will only intersect at one point, then it represent a function.
Here, The graph satisfy vertical line test, this means that for every input (x value) there is only one output (y value).
Thus, The graph represents a function.
Learn more about the function visit:
https://brainly.com/question/11624077
#SPJ7
classify the following differential equation s given in both standard and differential form. Q5 only
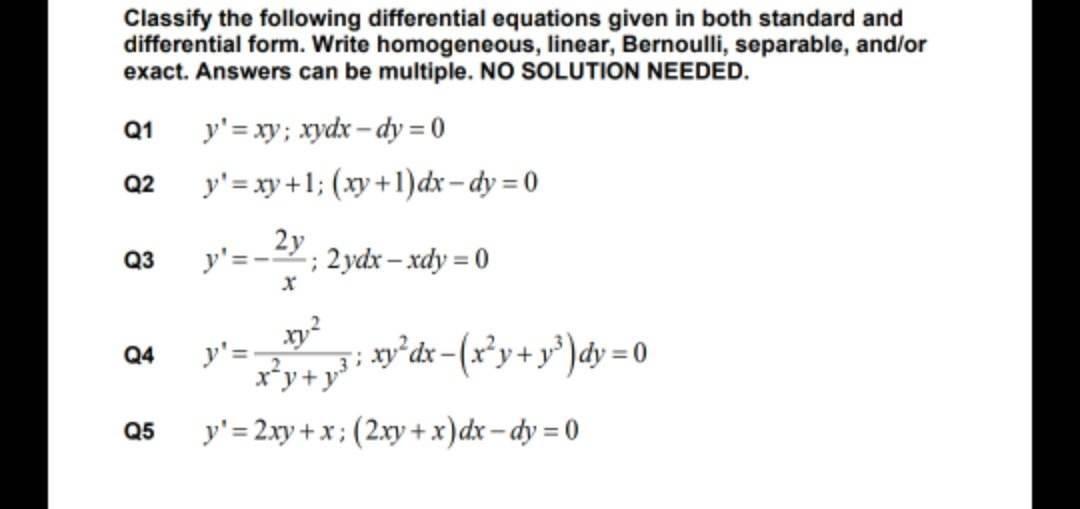
Answers
y' = 2xy + x
Rewriting it in differential form, we get:
(2xy + x)dx - dy = 0
This is a first-order linear differential equation, which can be written in the form:
y' + P(x)y = Q(x)
where P(x) = -2x and Q(x) = x.
Therefore, the differential equation is a first-order linear differential equation.
1. Tim wants air conditioning in his room. The air conditioner costs $40. Each day the
electricity costs $1. Write an expression that can be used to find the cost to run the air
conditioner for d days.
Answers
Answer:
the answer/expression you require is 40 - 1 = 1 day x - 1 = day 2 (repeat day 2 forever
Step-by-step explanation:
i say this because - 40 for the air conditioner and if it’s - 1 for electricity a day then unless your buying an air conditioner every day i don’t think you,l be repeating day 1 every day
:D
The park charges a $5 rental fee for a paddle boat in addition to
charging $3 an hour for usage. Find the total cost of renting the boat for
the number of hours.

Answers
Answer:
y=3x+5
0 hours is $5
1 hour is $8
2 hours is $11
3 hours is $14
Step-by-step explanation:
y=mx+b
m = slope and b = y intercept
in this case 3 dollars an hour (x) would be m
and 5 rental fee would be B
Lola bought x pencils that cost $0.25 each and y erasers that cost $0.50. She spent less than $3. Which graph represents Lola’s purchase?
Answers
Answer:
Graph C is the answer.
Step-by-step explanation:
Lola bought X pencils that cost $0.25 and Y erasers that cost $0.50.
Total expenditure is less than $3.
If we represent expense in the equation form then it will be
Expense on pencils + expense on erasers = Total expense which is < 3
0.25X + 0.50Y < 3
Now we divide the inequality by 0.25
X + 2Y < 12
This inequality when graphed, line will be plotted in dots and area below the line will be in the shaded form.
Slope of this line is = (-1/2) {from the standard equation of line y = mx + c)
Now we come to the graphs. Here dotted line graphs are A or C.
We will calculate the slopes of the lines from the graphs A and C to get tha answer.
For Graph A
The end points are (12, 0) and (0, 3)
So slope = (y-y')/(x-x') = (3-0)/(0-12) = -3/12 = -1/4
For Graph C.
End points the line are (0, 6) and (12, 0)
Slope of the line = (0-6)/(12-0) = -6/12 = -1/2
Therefore Graph C is the answer.
Answer:
Graph C is the answer.
Step-by-step explanation:
Lola bought X pencils that cost $0.25 and Y erasers that cost $0.50.
Total expenditure is less than $3.
If we represent expense in the equation form then it will be
Expense on pencils + expense on erasers = Total expense which is < 3
0.25X + 0.50Y < 3
Now we divide the inequality by 0.25
X + 2Y < 12
This inequality when graphed, line will be plotted in dots and area below the line will be in the shaded form.
Slope of this line is = (-1/2) {from the standard equation of line y = mx + c)
Now we come to the graphs. Here dotted line graphs are A or C.
We will calculate the slopes of the lines from the graphs A and C to get tha answer.
For Graph A
The end points are (12, 0) and (0, 3)
So slope = (y-y')/(x-x') = (3-0)/(0-12) = -3/12 = -1/4
For Graph C.
End points the line are (0, 6) and (12, 0)
Slope of the line = (0-6)/(12-0) = -6/12 = -1/2
Therefore Graph C is the answer.
Step-by-step explanation:
make brainiest please
Every month Tanesha pays a fixed fee of $10 to use the parking lot at her workplace. She must also pays $2 each day that she parks her car in the lot. If she parks there x days in one month, which of the following represents the amount in dollars that she must pay for using the parking lot that month?
Answers
The equation that represents the amount in dollars that she must pay for using the parking lot that month is y = 2x +10, the correct option is B.
What is an Equation?An equation is a mathematical statement formed when two algebraic expressions are equated using an equal sign.
The equations are useful in determination of unknown parameters.
The equations are of various types, such as Trigonometric equations, quadratic equations.
The amount Tanesha pays to use the parking lot is $10
The daily amount she pays to use the parking is $2
Let x represents the days in one month.
Let y represents the amount in dollars that she must pay for using the parking lot that month.
The unknown parameters are x and y
The equation that can be formed is
y = 2x +10
It seems that your question is incomplete, the complete question is as follows:
A . y = 2x
B. y = 2x +10
C. y = 10x +2
D. y = 10x
To know more about Equation
https://brainly.com/question/10413253
#SPJ5
which of the following are solutions to the quadratic equation check all that apply x ^ 2 + 10x + 25 = 2
Answers
Answer:
to solve the equation you first need to bring it to factors and by doing that you first need to let the equation equal 0 hence you need to minus 2 on both sides of the equation therefore
x^2 + 10x + 25 - 2 =2 - 2
therefore
x^2 + 10x +23 = 0
now since the equation cannot be factored, we use the formula.
x= \(\frac{-b +- \sqrt{b^{2}-4ac } }{2a}\)
where
a=1
b=10
c=23
note we use the coefficients only.
therefore x = \(\frac{-10 -+ \sqrt{10^{2}-4(1)(23) } }{2(1)}\)
=\(\frac{-10-+\sqrt{100-92} }{2}\)
=\(\frac{-10-+\sqrt{8} }{2}\)
then we form two equations according to negative and positive symbols
x=\(\frac{-10+\sqrt{8} }{2} or x =\frac{-10-\sqrt{8} }{2}\)
therefore x = \(-5+\sqrt{2}\) or x=\(-5-\sqrt{2}\)
Mr. Hooper has a tree in his front yard that grows every year. If the tree was 3 feet tall when he planted it 6 years ago , what is the current height of the tree in terms of f?
A. 3f + 6 feet
B. 6f + 3 feet
C. 3f + 18 feet
D. 6f + 18 feet
Answers
Therefore, the current height of the tree in terms of "f" would be 3f + 6 feet. So the correct answer is A. 3f + 6 feet.
What's the vertex for 6x^2-48x-54=0?
Answers
Hello!
\(\large\boxed{(4, -150)}\)
We can solve for the vertex by completing the square. Begin by factoring out 6 from the equation to simplify the process:
6(x² - 8x) - 54 = 0
To complete the square, we must look at the first two terms (x² - 8x).
Remember that squaring a binomial uses the format a² + 2ab + b². We are already given a² and 2ab², so solve for b:
-8 / 2 = -4. This is the value of b.
We can rewrite this as:
(x - 4)²
However, this produces +16 which much be taken into account. Substitute (x - 4)² into the original equation:
6(x - 4)² - 54 = 0
Multiply 16 by the term in the front and subtract to cancel out this term:
6(x - 4)² - 54 - (6 · 16) = 0
Simplify:
6(x - 4)² - 150 = 0
In this form, the vertex is given as:
a(x - h)² + k, where h = x-coordinate and k = y-coordinate of the vertex.
In this instance, h = 4 and k = -150, so the coordinates of the vertex are:
(4, -150)
Answer:
y = 6x²-48x-54 has a vertex at (4,-150)
Step-by-step explanation:
There is no vertex. The solution set to 6x²-48x-54 = 0 is two points, (-1,0) and (9,0).
If you mean y = 6x²-48x-54, that is an up-opening parabola.
Put the equation into vertex form:
y = 6x²-48x-54
= 6(x²-8x)-54
= 6(x²-8x+4²)-6·4²-54
= 6(x-4)² - 150
vertex at (4,-150)
Problem
What is the measure of angle A?
54
44
34
64

Answers
Answer:
54
Step-by-step explanation:
90-36=54
What is the exponential regression equation for this Data set (2, 34) (4,45) (6,51) (8,68) (10,71) (12,79) (14,88)?
Answers
The exponential expression can be written as \(y = 25.70(1.15)^x\).
What is an exponential expression?The exponential functions are those with the formula f(x) = a. x. In this case, a ( 0) is known as the base, and x is known as the exponent. Explanation: Exponential functions are those with the formula \(a^x\).
Given that Data set (2, 34) (4,45) (6,51) (8,68) (10,71) (12,79) (14,88). The exponential expression can be written as:-
\(y = ab^x\)
At points (2, 34) and (4,45). Solve the equations and find the value of a and b.
34 = ab²
45 = ab⁴
b⁴ / b² = 45 / 34
b² = 1.32
b = √1.32
b = 1.15
The value of a will be:-
34 = ab²
34 = a x (1.15)²
a = 25.70
The exponential equation can be written as:-
\(y = ab^x\)
\(y = 25.70(1.15)^x\)
To know more about an exponential expression follow
https://brainly.com/question/2456547
#SPJ9
find the area of a rectangular carpet the measures 3 1/3 feet by 3 feet
Answers
Answer:
area = 4
Step-by-step explanation:
area = length × width
length= 3 1/3 =3/3 = 1 and width= 3
area = 1 × 3
= 4
(sec theta - tan theta)²
Answers
Answer:
(sec θ - tan θ)²
= sec² θ –2 sec θ tan θ + tan² θ
please help me !!! continuity
for the function

Answers
The function f(x) = sin⁻¹x, x ≠ π/4 and sinx, x = π/4 is continuous on[-1, π/4) ∪ (π/4, 1]. There is a removable discontinuity at x = π/4
What is the continuity of a function?A function is said to be continuous if there are no breaks or jumps in the functions
Conditions for continuity of a function
The function exists at x = aThe limit of the function as x approaches a existThe limit of the functions a s x a pproaches a equals the value of the function at x = a.Now, for the given function f(x) = sin⁻¹x, x ≠ π/4 and sinx, x = π/4
We need to determine the interval of continuity, We proceed as follows.
Since f(x) = sin⁻¹x, x ≠ π/4 , we know that the minimum value of x is - 1 and its maximum value is 1.
So, x must lie in the interval (-1, 1) for x ≠ π/4,
Now, when x = π/4 f(x) = sinx. We know that x = π/4 lies in the interval (-1, 1).
But we know that f(x) = sinx at x = π/4. So, there is a removable discontinuity at x = π/4.
So, from the left, f(x) is continuous on the interval [-1, π/4) and from the right, f(x) is continuous on the interval (π/4, 1]
So, combining both intervals, we have f(x) is continous on the interval [-1, π/4) ∪ (π/4, 1] and since f(x) = sinx at x = π/4, there is a removable discontinuity at x = π/4
So, the function f(x) is continuous on[-1, π/4) ∪ (π/4, 1]. There is a removable discontinuity at x = π/4
Learn more about continuity of a function here:
https://brainly.com/question/28830920
#SPJ1
I need help on this question?

Answers
pls help its urgent!

Answers
Since each term can be written as the previous term divided by three, this is a geometric sequence.
What is a geometric sequence?A geometric sequence is a sequence in which the result of the division of consecutive terms is always the same, called common ratio q.
The nth term of a geometric sequence is given by:
\(a_n = a_1q^{n-1}\)
In which \(a_1\) is the first term.
For this problem, we have that each term can be written as the previous term multiplied by one third or divided by three, hence the division of consecutive terms is always the same and this is a geometric sequence.
More can be learned about geometric sequences at https://brainly.com/question/11847927
#SPJ1