y = 2x – 6
4x – 2y = 14
Use the substitution method.
Answers
Explanation: 4x-2(2x-6)=14
4x-4x+12=14
X will be zero which is impossible
Related Questions
If m∠1 equals (4x + 2) and m∠3 equals (5x − 25), what is the value of x?
Answers
Step-by-step explanation: the answer is x = 1/2
The value of x is 27 units.
It is required to find the value of x.
What is angle?In Plane Geometry, a figure which is formed by two rays or lines that shares a common endpoint is called an angle and corner whether constituting a projecting part or a partially enclosed space.
Given:
If m∠1 equals (4x + 2) and m∠3 equals (5x − 25),
According to given question we have,
m∠1 =m∠3
(4x + 2)=(5x − 25)
compare the like terms and simplify we get,
2+25=5x-4x
27=x
Therefore, the value of x is 27 units.
Learn more about angle here:
https://brainly.com/question/13954458
#SPJ2
192
7) 1/2+1/2=
Your answer
Answers
Answer:
1 is the answer as 0.5 +0.5=1
haha help me be brainiest
Answer:
0.5+0.5=1
hahaha
it's so easy
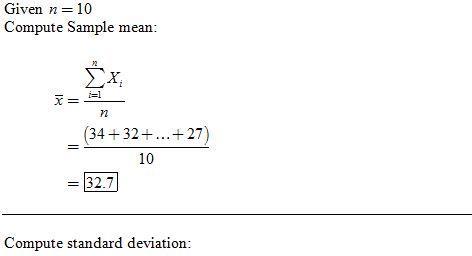
PLEASE HELP! 50 POINTS! Consider the following set of sample data: (34, 32, 34, 32, 40, 37, 31, 31, 29, 27). Which of the following will give a 95% t confidence interval for the mean of the population from which the sample was drawn?
Answers
Answer:
(15.23,41.016)
Step-by-step explanation:
WE must determine the mean of the data set: Which is the sum of the set divided by the number in the set.
\(= (21 + 24 + 25 + 32 + 35 + 31 + 29 + 28)/8 = 225/8 = 28.125\)
We must also determine the standard deviation: Which is the square root of the variance and the variance is the sum of squares of the sample number minus the mean divided by the number if the set data:
\(= ((21 - 28.125)^{2} + (24 - 28.125)^{2} +(25 - 28.125)^{2} + (32 - 28.125)^{2} + (35 - 28.125)^{2} + (31 - 28.125)^{2} + (29 - 28.125)^{2} (28 - 28.125)^{2}\)
\(= 148.877/8 = 18.6\)
The 95% confidence interval is defined as: The mean ± 1.96*standard deviation divided by the sqaure root of the number of data in the set:
\(= 28.125 + (1.96 *18.6)/(\sqrt{8} )\)
\(= 41.016\)
\(= 28.125 - (1.96 * 18.6)/(\sqrt{8}) = 15.23\)
The confidence interval for this data set is (15.23,41.016)
Answer:
32.7 ± 2.262(1.19)
Step-by-step explanation:
See attached pictures

Plot the points A(- 1, - 1); B(9, 2) * C(2,- 6) on the coordinate axes below. State the coordinates of point D such that A, B, C, and D would form a parallelogram .
Answers
Select the correct answer.
Evaluate the following expression when x = -4 and y = 4.
x
6
−
x
4
y
A.
1
,
025
4
B.
1
,
023
4
C.
16
,
385
4
D.
−
1
,
023
4

Answers
Answer:
1023/4
Step-by-step explanation:
shown in the picture

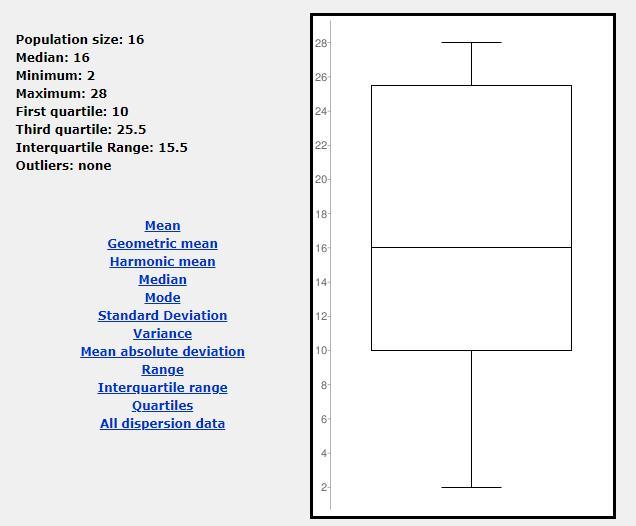
4. Which set of data could be represented by the box-and-whisker plot? (1 point)
O0, 3, 9, 9, 11, 14, 15, 16, 16, 18, 19, 25, 25, 28, 28, 30
O2, 3, 4, 7, 13, 15, 15, 15, 16, 17, 20, 22, 25, 25, 26, 28
O2, 6, 7, 8, 10, 11, 12, 14, 18, 20, 22, 24, 26, 27, 27, 28
O2, 2, 7, 10, 10, 11, 13, 15, 17, 20, 20, 24, 26, 27, 27, 28

Answers
A set of data that could be represented by the box-and-whisker plot include the following: D.
The median of the set of data is equal to 10.
What is a box-and-whisker plot?In Mathematics and Statistics, a box-and-whisker plot is a type of chart that can be used to graphically or visually represent the five-number summary of a data set with respect to locality, skewness, and spread.
Based on the information provided about the data set (2, 2, 7, 10, 10, 11, 13, 15, 17, 20, 20, 24, 26, 27, 27, 28), the five-number summary for the given data set include the following:
Minimum (Min) = 2.
First quartile (Q₁) = 16.
Median (Med) = 10.
Third quartile (Q₃) = 25.5.
Maximum (Max) = 28.
In conclusion, we can logically deduce that the median of the data set is 10 and it has no outlier.
Read more on boxplot here: brainly.com/question/29648407
#SPJ1
has a perimeter of 52 feet. Let W be the width, L be the length, and P be
the perimeter, all with units in feet.
a. Given two sets of four rectangles, find one rectangle in each set that could have a
perimeter of 52 feet.
b. Which of the symbols W, L, and P are variables?
c. Which of the symbols W, L, and P are constants?
Answers
A rectangle that could have a perimeter of 52 feet is a 12 feet by 14 feet rectangle.
The symbols W and L are variables.
The symbol P is a constant.
How to calculate the perimeter of a rectangle?In Mathematics and Geometry, the perimeter of a rectangle can be calculated by using this mathematical equation (formula);
P = 2(L + W)
Where:
P represent the perimeter of a rectangle.W represent the width of a rectangle.L represent the length of a rectangle.By substituting the given side lengths into the formula for the perimeter of a rectangle, we have the following;
P = 2(L + W)
52 = 2(12 + 14)
52 = 2(26)
52 feet = 52 feet.
Read more on perimeter of a rectangle here: brainly.com/question/28695033
#SPJ1
Derrick has 2 1/2 cups of sugar. He needs 1/8 of a cup of sugar for each recipe. How many recipes can be make
Answers
Answer:
20
Step-by-step explanation:
Waiting times (in minutes) of customers at a bank where all customers enter a single waiting line and a bank where customers wait in individual lines at three different teller windows are listed below. Find the coefficient of variation for each of the two sets of data, then compare the variation.
Bank A (single line) Bank B (individual lines)
6.5 4.0
6.6 5.4
6.7 5.9
6.7 6.2
7.1 6.8
7.4 7.7
7.5 7.7
7.7 8.5
7.7 9.4
7.7 9.8
A) The coefficient of variation for the waiting times at Bank A is
(Round to one decimal place as needed.)
B) The coefficient of variation for the waiting times at Bank B is
(Round to one decimal place as needed.)
C)Is there a difference in variation between the two data sets?
Answers
The coefficient of variation for Bank A is approximately 8.04%, while the coefficient of variation for Bank B is approximately 25.55%.
To find the coefficient of variation for each set of data, we need to calculate the mean and standard deviation for each set. The coefficient of variation is then calculated by dividing the standard deviation by the mean and multiplying by 100.
Let's calculate the coefficient of variation for each set of data:
Bank A (single line):
Mean: Calculate the mean of the data set.
Mean = (6.5 + 6.6 + 6.7 + 6.7 + 7.1 + 7.4 + 7.5 + 7.7 + 7.7 + 7.7) / 10 = 7.03 minutes
Standard deviation: Calculate the standard deviation of the data set.
Standard deviation = √[(6.5 - 7.03)² + (6.6 - 7.03)² + ... + (7.7 - 7.03)²] / 10 ≈ 0.565 minutes
Coefficient of variation:
Coefficient of variation = (0.565 / 7.03) * 100 ≈ 8.04%
Bank B (individual lines):
Mean: Calculate the mean of the data set.
Mean = (4.0 + 5.4 + 5.9 + 6.2 + 6.8 + 7.7 + 7.7 + 8.5 + 9.4 + 9.8) / 10 = 7.5 minutes
Standard deviation: Calculate the standard deviation of the data set.
Standard deviation = √[(4.0 - 7.5)² + (5.4 - 7.5)² + ... + (9.8 - 7.5)²] / 10 ≈ 1.916 minutes
Coefficient of variation:
Coefficient of variation = (1.916 / 7.5) * 100 ≈ 25.55%
Comparing the variation:
The coefficient of variation for Bank A is approximately 8.04%, while the coefficient of variation for Bank B is approximately 25.55%. Since the coefficient of variation measures the relative variability of the data, we can conclude that the waiting times at Bank B (individual lines) have a higher variation compared to Bank A (single line).
for such more question on coefficient
https://brainly.com/question/1038771
#SPJ8
how many hours is 4,480 minutes.
Answers
Answer:74.67
Step-by-step explanation:There ya go :>
For each value of u determine whether it is a solution to -2u-6<-20
Answers
Answer:
u > 7
Step-by-step explanation:
-2u -6 < -20 Add 6 to both sides
-2u - 6 + 6 < -20 + 6
-2u < -14 Divide both sides by -2. When you multiply or divide both sides by a negative number, you must flig the sign.
\(\frac{-2u}{-2}\) > \(\frac{-14}{-2}\)
u > 7
Helping in the name of Jesus.
The answer is:
u > 7
In-depth explanation:
You haven't told me what the values of u are, but I'll solve the equation for u.
Add 6 on each side:
\(\bf{-2u-6 < -20}\)
\(\bf{-2u < -14}\)
Divide each side by -1 and reverse the sign:
\(\bf{2u > 14}\)
Now divide each side by 2
\(\bf{u > 7}\)
Given that p(x)=2(5−x)2+1 , what is the value of p(-4)? Responses
Answers
Answer:
37
Step-by-step explanation:
x=-4
=2(5-(-4)2+1
=2(5+4)2+1
=2(9)2+1
=18(2)+1
=36+1
=37
PLEASE HELPP ASAPPP pleaseeeee

Answers
answer: 28561^2in the area involves multiplying by the 4 sides of the shaded square and the shaded is the colored square.
in the number 234.567 in the whole numbers place is _____________?
Answers
- Suppose y varies directly as x. If y = -7 when x = -14, find y when x=3
Answers
Answer:1.5
Step-by-step explanation:
Y=1/2 of x
It takes 16 cups of chicken broth to make a chicken soup recipe. How much is this in quarts?
Use the table below. Include the correct unit in your answer.
Volume
Unit
Symbol
Fact
fluid Ounce
fl oz
cup
с
1c = 8 fl oz
pint
pt
1 pt = 2c
quart
qt
1 qt = 2 pt
gallon
gal
1 gal = 4 qt
Answers
Answer:
Hi
Step-by-step explanation:
it's 4
Answer:
4 quarts
Step-by-step explanation:
Write y = 5(x - 8)^2 + 4 in standard form
Answers
Answer:
\(y=5x^2-80x+324\)
Step-by-step explanation:
Find the Z score to the nearest hundred thousand satisfies the given condition 0.166 square units of the standard normal distribution is to the left of the Z
Answers
The sum of the total area of a distribution is equal to 1. In our problem, we have 0.166 square units to the left of our value, therefore, we have the following area on the right
\(1-0.166=0.834\)Using a z-table, we can find the corresponding z-value for this area to the right
The corresponding z-score to this area is 0.97, however, this value is below the mean, therefore it is negative.
The coresponding z-value is
\(z=-0.97\)
A radioactive isotope is decaying at a rate of 15% every hour. Currently there are 150 grams of the substance. Write an equation that will represent the number of grams present after n hours. How much will be left one day from now?A radioactive isotope is decaying at a rate of 15% every hour. Currently there are 150 grams of the substance. Write an equation that will represent the number of grams present after n hours. How much will be left one day from now?
Answers
Answer:
The equation that will represent the number of grams present after n hours is \(A(n) = 150(0.85)^n\)
3.035 grams will be left one day from now.
Step-by-step explanation:
Exponential equation for the amount of a substance:
The exponential equation for the amount of a substance is given by:
\(A(t) = A(0)(1-r)^t\)
In which A(0) is the initial amount and r is the decay rate, as a decimal, and t is the time period.
A radioactive isotope is decaying at a rate of 15% every hour.
This means that \(r = 0.15\)
Currently there are 150 grams of the substance.
This means that \(A(0) = 150\)
Write an equation that will represent the number of grams present after n hours.
\(A(n) = A(0)(1-r)^n\)
\(A(n) = 150(1-0.15)^n\)
\(A(n) = 150(0.85)^n\)
How much will be left one day from now?
One day is 24 hours, so this is A(24).
\(A(24) = 150(0.85)^{24} = 3.035\)
3.035 grams will be left one day from now.
Which ordered pair will be solution for the function y=8+ X?
Answers
Answer:
x - (-8,0)
y - (0,8)
Step-by-step explanation:
To find the x-intercept, substitute in 0 for y and solve for x . To find the y-intercept, substitute in 0 for x and solve for y .
can somone help with this

Answers
If the cylindrical-barrel has volume of 785.4 ft³, then the radius of the barrel is 5 ft, option(c) is correct.
We know that volume of cylindrical barrel is given by formula : V = πr²h
where V denotes volume, r denotes radius, and h denotes height of the cylinder.
In this case, we are given that the volume of the barrel is 785.4 ft³ and the height is 10 ft.
Substituting these values and taking π ≈ 3.14, into the Volume-formula,
We get,
785.4 = 3.14 × r² × (10);
Simplifying this equation,
We get,
785.4/31.4 = r²,
r = √(785.4/31.4),
r ≈ 5 ft
Therefore, the radius of the barrel is approximately 5 feet, correct option is (c).
Learn more about Volume here
https://brainly.com/question/27033747
#SPJ1
General Hospital has noted that they admit an average of 9 patients per hour.
Answers
Answer:
(a) The probability that during the next hour less than 3 patients will be admitted is 0.00623.
(b) The probability that during the next two hours exactly 8 patients will be admitted is 0.00416.
Step-by-step explanation:
The complete question is: General Hospital has noted that they admit an average of 8 patients per hour.
(a) What is the probability that during the next hour less than 3 patients will be admitted?
(b) What is the probability that during the next two hours exactly 8 patients will be admitted?
The above situation can be represented through Poisson distribution as it includes the arrival rate of the pattern. So, the probability distribution of the Poisson distribution is given by;
\(P(X = x) = \frac{e^{-\lambda} \times \lambda^{x} }{x!} ; x = 0,1,2,......\)
Here X = Number of patients admitted in the hospital
\(\lambda\) = arrival rate of patients per hour = 9 patients
So, X ~ Poisson(\(\lambda\) = 9)
(a) The probability that during the next hour less than 3 patients will be admitted is given by = P(X < 3)
P(X < 3) = P(X = 0) + P(X = 1) + P(X = 2)
= \(\frac{e^{-9} \times 9^{0} }{0!} + \frac{e^{-9} \times 9^{1} }{1!} + \frac{e^{-9} \times 9^{2} }{2!}\)
= \(e^{-9} +(e^{-9} \times 9)+ \frac{e^{-9} \times 81}{2}\)
= 0.00623
(b) Here, \(\lambda = 9 \times 2\) = 18 because we have to find the probability for the next two hours and we are given in the question of per hour.
So, X ~ Poisson(\(\lambda\) = 18)
Now, the probability that during the next two hours exactly 8 patients will be admitted is given by = P(X = 8)
P(X = 8) = \(\frac{e^{-18} \times 18^{8} }{8!}\)
= 0.00416
In circle P, BC=24ft. What is the length of arc AC?
Answers
List all the factors of
36
Answers
We can see that 36 is a composite number.
We also know that \(36=1\times36\), \(3\times15\), or \(4\times9\). All of these numbers are prime numbers so they are all factors of 36.
Thus we see that the factors of 36 are 1, 2, 3, 4, 6, 9, 12, 18, and 36.
9. If 15 men working 10 days earn $500. How much will 12 men earn working 14,
days? [Ans. $560]
7
Answers
Answer:
Firstly need to understand the questions.
If 15 men working 10 days, They earn 500.
If 12 men working 14 days, how much they will earn?
Step-by-step explanation:
560
Solution:
15 men working for 10 days =500
1 man working for 1 day = 500/(10*15) =10/3
Now 12 men working for 14 days = 12*14*10/3 =560.
I am pretty sure that answer is right.
Thank you :)
Maria took a history test with 25 questions on it. She correctly answered 22 questions. Write her test score as a percent.
Your answer:
Answers
Answer:
(100/25)*22 = 88%
Step-by-step explanation:
100% = 25, simply divide 100 by 25 and multiply by 22 = 88%
A survey of 400 students yielded the following information: 262 were seniors, 215 were commuters, and 150 of the seniors were commuters. How many of the 400 surveyed students were seniors or were commuters?
Answers
Out of the 400 surveyed students, 327 were either seniors or commuters.
To find the number of students who were either seniors or commuters out of the 400 surveyed students, we need to add the number of seniors and the number of commuters while avoiding double-counting those who fall into both categories.
According to the information given:
There were 262 seniors.
There were 215 commuters.
150 of the seniors were also commuters.
To avoid double-counting, we need to subtract the number of seniors who were also commuters from the total count of seniors and commuters.
Seniors or commuters = Total seniors + Total commuters - Seniors who are also commuters
= 262 + 215 - 150
= 327
Therefore, out of the 400 surveyed students, 327 were either seniors or commuters.
It's important to note that in this calculation, we accounted for the overlap between seniors and commuters (150 students who were both seniors and commuters) to avoid counting them twice.
This ensures an accurate count of the students who fall into either category.
For similar question on surveyed.
https://brainly.com/question/14610641
#SPJ8
Identifying the values a, b, and c is the first step in using the Quadratic Formula to find solution(s) to a quadratic equation. What are the values a, b, and c in the following quadratic equation? -3x^2 -5x +9 =0
Answers
Answer:
The quadratic equation is in the standard form: ax^2 + bx + c = 0. To use the quadratic formula to find the solutions, we need to identify the values of a, b, and c in the equation:
a is the coefficient of the x^2 term, which is -3 in this case.
b is the coefficient of the x term, which is -5 in this case.
c is the constant term, which is 9 in this case.
Therefore, the values of a, b, and c in the quadratic equation -3x^2 - 5x + 9 = 0 are:
a = -3
b = -5
c = 9
addition and subtraction of polynomials 1. 3ab +(- 10ab) 2. (4a - 4b) + ( 2a + 6b ) 3. (-11m + 7) - (20m - 11) 4. (2m- 2n ) -( -15 m + 10n) 5. -6xy- (-16xy) plz pa answer ASAP show
solution step-by-step
Answers
Step-by-step explanation:
1. 3ab +(-10ab)
-7ab
2.4a -4b
4 (a-b)
3.(-11m+7) -(20m-11)
-11m+7-20m+11
-31m+18
4.(2m-2n)-(15m+10n)
-13m-12n
5.-6xy- (-16xy)
-6xy+16xy
10xy
What’s it the correct answer? I will mark as brainlest

Answers
Answer:
Step-by-step explanation:
(216/4)-20+3(5)
(54)-20+15
34+15 = 49
option 1
Answer:
49
Step-by-step explanation:
(6³ / 4) - 4 * 5 + 3(7 - 2)
= (216 / 4) - 20 + 3 * 5
= (216 / 4) - 20 + 15
= 54 - 20 + 15
= 34 + 15
= 49
Hope it helps