write three hundred and ninety six million two hundred and forty two thousand and two as a numeral
Answers
Answer:
Below.
Step-by-step explanation:
396,242,002
Related Questions
which equation has a solution x= -3
A) 2x - 7 = -1
B) 3x + 8 = 1
C) 1/2x + 8 = 10
D) 1/2(2x - 6) = -6
Answers
Answer:
D
Step-by-step explanation:
1/2 ( 2x - 6) = -6
1/2 = 0.5
0.5(2x-6) = -6
1x-3=-6
1x=-6+3
x=-3/1
x=-3
Step by step:
1/2(2x - 6) = -6
-6 -6
1/2 (-12) = -6
-6 = -6
While making desserts for Fun Day, Methuki used 5/6 of a scoop of brown sugar as well as 1/2 of a scoop of white sugar. How much more brown sugar did she use?
Answers
Hey there! I'm happy to help!
We want to find how much more 5/6 is than 1/2. To do this, we simply subtract 1/2 from 5/6. This will show us how much more 5/6 is.
First, we multiply 1/2 by 3/3 so that the denominator is 6.
1/2×3/3=3/6
Now, we subtract this from 5/6.
5/6-3/6=2/6
We can simplify this to 1/3.
Therefore, Methuki used 1/3 scoop more of brown sugar than white sugar while making desserts for Fun Day.
Have a wonderful day! :D
In this system of equations, which variable would be easier to solve for?

Answers
Answer:
the easiest to solve for is x in the first equation
Answer:
"The easiets to solve for is x in the first equation"
Step-by-step explanation:
All you have to do to solve for x in the first equation is one step, and that's subtracting by 3y. After you subtract by 3y you get x = -3y + 13. All the other ways of solving invole two or more steps.
Simplify \(\frac{sec(a)-csc(a)}{sec(a)+csc(a)}\)
Answers
The simplified version of (sec a - cosec a) / (sec a + cosec a) is cosec 2a(cosec 2a - 2) / (sec²a - cosec²a).
What is trigonometry?The study of correlations between triangles' side lengths and angles is known as trigonometry. The field was created in the Hellenistic era in the third century BC as a result of the use of geometry in astronomical research.
Given:
(sec a - cosec a) / (sec a + cosec a)
Multiply the numerator and denominator by (sec a - cosec a)
(sec a - cosec a) / (sec a + cosec a) × (sec a - cosec a)
(sec²a + cosec²a -2sec a cosec a) / (sec²a - cosec²a)
As we know,
\(sec^2a + cosec^2a = sec^2a \ cosec^2a\)
sec² a cosec² a - 2sec a cosec a / (sec²a - cosec²a)
sec a cosec a (sec a cosec a - 2) / (sec²a - cosec²a)
cosec 2a(cosec 2a - 2) / (sec²a - cosec²a)
To know more about trigonometry:
https://brainly.com/question/14272510
#SPJ1
Please help me with this homework

Answers
Answer:
its sideways
Step-by-step explanation:
i cant understand it sorry
can someone help me with this question?

Answers
Answer: the answer is b,
Step-by-step explanation:
the girls are longer cause their shoes combined is 12 and 3/8
Answer:
Both daughters shoes combines
Step-by-step explanation:
8 5/8 plus 3 6/8 = 12 3 /8
"The combines length of the daughters shoes are longer since they are 12 and 3/8"
5/8 plus 6/8 = 11/8
11/8= 1 3/8
11+ 1+ 3/8 = 12 3/8
5. Using complete sentences, describe how the variable h and the variable k of the general formula for a cube root function effects the graph. The general formula is
y=a³√√x-h+k
Answers
Answer: The variables h and k in the general formula for a cube root function affect the graph by causing a horizontal and vertical shift, respectively. The value of h determines the horizontal position of the graph by shifting it to the right or left along the x-axis. If h is positive, the graph will shift to the right, and if h is negative, the graph will shift to the left. On the other hand, the value of k determines the vertical position of the graph by shifting it up or down along the y-axis. If k is positive, the graph will shift upward, and if k is negative, the graph will shift downward. It is important to note that these shifts do not affect the shape of the graph, only its position on the coordinate plane. Therefore, changing the values of h and k in the general formula for a cube root function will cause the graph to shift, but the overall shape of the graph will remain the same.
Step-by-step explanation:
Find the Taylor series for f(x) centered at the given value of a. [Assume that f has a power series expansion. Do not show that Rn(x) → 0.]
f(x) = x⁴ - 6x² + 1, a = 2
Answers
This is the Taylor series expansion for the function f(x) = x⁴ - 6x² + 1 centered at a = 2.
What is Taylor Series?
In mathematics, the Taylor series or Taylor expansion of a function is an infinite sum of terms that are expressed in terms of the derivative of the function at a single point. For most common functions, the function and the sum of its Taylor series near this point are the same.
To find the Taylor series for the function f(x) = x⁴ - 6x² + 1 centered at a = 2, we can use the formula for the Taylor series expansion:
f(x) = f(a) + f'(a)(x - a)/1! + f''(a)(x - a)²/2! + f'''(a)(x - a)³/3! + ...
First, let's find the values of f(a) and its derivatives at x = a = 2:
f(2) = (2)⁴ - 6(2)² + 1 = 16 - 24 + 1 = -7
f'(x) = 4x³ - 12x
f'(2) = 4(2)³ - 12(2) = 32 - 24 = 8
f''(x) = 12x² - 12
f''(2) = 12(2)² - 12 = 48 - 12 = 36
f'''(x) = 24x
f'''(2) = 24(2) = 48
Now, we can substitute these values into the Taylor series formula:
f(x) = f(a) + f'(a)(x - a)/1! + f''(a)(x - a)²/2! + f'''(a)(x - a)³/3! + ...
f(x) = -7 + 8(x - 2)/1! + 36(x - 2)²/2! + 48(x - 2)³/3! + ...
Simplifying the terms:
f(x) = -7 + 8(x - 2) + 18(x - 2)² + 8(x - 2)³ + ...
This is the Taylor series expansion for the function f(x) = x⁴ - 6x² + 1 centered at a = 2.
To learn more about Taylor Series from the given link
https://brainly.com/question/12800011
#SPJ4
simplify the following numerical expression as much as possible. write your answer in exponential form. let a and b be positive integers. 23a x 23b
Answers
The simplified expression in exponential form would be 23^(a + b).
To simplify the numerical expression 23a x 23b, we can combine the like terms.
Step 1: Simplify 23a and 23b individually.
The expression 23a means multiplying 23 by a, and 23b means multiplying 23 by b. We cannot simplify them further because we do not have specific values for a and b.
Step 2: Combine the simplified terms.
When we multiply 23a by 23b, we multiply the coefficients (23 x 23) and the variables (a x b). Therefore, the simplified expression is:
(23 x 23) x (a x b)
Step 3: Calculate the coefficient and write the answer in exponential form.
The coefficient (23 x 23) is equal to 529. In exponential form, we can write it as 23^2. So the final simplified expression is:
23^2 x (a x b)
In summary, the simplified numerical expression 23a x 23b can be written as 23^2 x (a x b) in exponential form.
To know more about exponential form refer here:
https://brainly.com/question/29166310
#SPJ11
why is 3 root square 4 equal to 4 1/3?

Answers
Answer: A
Step-by-step explanation:
let t:r3→r2t:r3→r2 be the linear transformation that first projects points onto the yzyz-plane and then reflects around the line y=−zy=−z. find the standard matrix aa for tt.
Answers
The standard matrix A for the linear transformation T is:
A = [[0, 0, 0], [0, 1, 1]]
To find the standard matrix A for the given linear transformation T: R^3 -> R^2, we can consider the effect of the transformation on the standard basis vectors in R^3.
The standard basis vectors in R^3 are:
e1 = [1, 0, 0]
e2 = [0, 1, 0]
e3 = [0, 0, 1]
We can apply the transformation T to these basis vectors and observe their images in R^2.
1. Projection onto the yz-plane:
The projection onto the yz-plane sets the x-coordinate of a point to zero while keeping the y and z coordinates unchanged. Therefore, the images of the standard basis vectors under this projection are:
T(e1) = [0, 0]
T(e2) = [0, 1]
T(e3) = [0, 1]
2. Reflection around the line y = -z:
This reflection replaces the y-coordinate of a point with its negative z-coordinate and replaces the z-coordinate with its negative y-coordinate. Therefore, the images of the vectors after the reflection are:
T'(T(e1)) = [0, 0]
T'(T(e2)) = [-1, 1]
T'(T(e3)) = [-1, 1]
Now, we can assemble the column vectors of the images into a matrix to obtain the standard matrix A for T:
A = [T(e1) | T(e2) | T(e3)]
= [0 0 0]
[0 1 1]
So, the standard matrix A for the linear transformation T is:
A = [[0, 0, 0], [0, 1, 1]]
To know more about linear transformation refer here:
https://brainly.com/question/13595405?#
#SPJ11
For the graphed function f(x) = -(3)x1+3, calculate the average rate of change from x = 1 to x = 3.
-4
-2
2
4

Answers
Answer:
The average rate of change is -4
Step-by-step explanation:
The given function is f(x) = -(3)x1+3
We want to find the average rate of exchange of this function from x=1 to x=3.
This is the slope of the secant line joining the points (1,f(1)) and (3,f(3)).
From the graph, f(1)=2 and f(3)= -6
The average rate of exchange
=f(3)-f(1)/3-1
=-6-2/3-1
=-8/2
=-4
Which equation is equivalent to the given equation? If anyone can help it means a lot

Answers
Answer:
x^2 + 16x + 60 = 0
Step-by-step explanation:
Add 16x to both sides of the given equation. We get x^2 + 16x + 60 = 0.
f(x) = 4x-9. Write a function h that is a reflection in the x-axis of f(x)
Answers
A function h that is a reflection in the x-axis of g(x) is -4 x+9.
What is function ?
A function is a type of rule that produces one output for a single input. Source of the image: Alex Federspiel. This is illustrated by the equation y=x2. Any input for x results in a single output for y. Considering that x is the input value, we would state that y is a function of x.
So we know that:
f(x)=4 x+9
To reflect across the y-axis, instead of x and -x .
Therefore:
g (x)=f(-x)=4(-x)+9
Simplify:
g(x)=-4 x+9
To learn more about function visit:https://brainly.com/question/21145944
#SPJ1
A function h that is a reflection in the x-axis of g(x) is -4 x+9.
What is function?Function is a mathematical expression, rule, or law that establishes the relationship between an independent variable and a dependent variable. Functions are used frequently in mathematics and are crucial for constructing physical relationships in the sciences. German mathematician Peter Dirichlet first offered the contemporary definition of function in 1837.
So we know that:
f(x)=4 x+9
To reflect across the y-axis, instead of x and -x .
Therefore:
g (x)=f(-x)=4(-x)+9
Simplify:
g(x)=-4 x+9
To learn more about function visit:
https://brainly.com/question/5975436
#SPJ1
chegg suppose we take a single observqation froma bernouilli population, where the mean is known to be restruction p [1/3, 2/3] what is the maximum likellhiood estimator
Answers
The maximum likelihood estimator (MLE) for a single observation from a Bernoulli population, where the mean is known to be restricted to p [1/3, 2/3], is p = 0.
The maximum likelihood estimator (MLE) for a single observation from a Bernoulli population with a known mean restriction p [1/3, 2/3] can be found by maximizing the likelihood function.
In this case, the likelihood function can be defined as the probability of obtaining the observed value given the parameter p. Since the population follows a Bernoulli distribution, the likelihood function can be expressed as:
\(L(p) = p^x * (1-p)^(1-x)\)
where x is the observed value (0 or 1).
To find the MLE, we need to find the value of p that maximizes the likelihood function. Taking the derivative of the log-likelihood function with respect to p and setting it equal to zero, we can solve for the MLE.
The log-likelihood function for a single observation from a Bernoulli distribution is:
\(log L(p) = x * log(p) + (1-x) * log(1-p)\)
Taking the derivative with respect to p:
\(d/dp (log L(p)) = (x/p) - ((1-x)/(1-p))\)
Setting it equal to zero and solving for p:
\((x/p) - ((1-x)/(1-p)) = 0\)
Simplifying the equation, we get:
\(x(1-p) - (1-x)p = 0\)
Expanding the equation further, we get:
x - px - p + xp = 0
2xp - 2p = x
Factoring out p, we get:
\(p(2x-2) = x\)
Dividing both sides by (2x-2), we get:
p = x / (2x-2)
In this case, since the mean is restricted to the range [1/3, 2/3], we need to consider the possible values of x (0 or 1) and substitute them into the equation to find the MLE.
For x = 0:
p = 0 / (2*0-2)
= 0
For x = 1:
p = 1 / (2*1-2)
= 1 / 0
= undefined
Therefore, the maximum likelihood estimator (MLE) for a single observation from a Bernoulli population, where the mean is known to be restricted to p [1/3, 2/3], is p = 0.
To know more about population visit-
https://brainly.com/question/15889243
#SPJ11
How can ΔWXY be mapped to ΔMNQ?
Triangles W X Y and M N Q are shown. The lengths of sides M N and W X are 18 centimeters. The lengths of sides Y W and M Q are 30 centimeters. Angles Y W X and N M Q are congruent. Triangle W X Y is slightly up and to the right of triangle M N Q. Angle Y W X is the bottom angle and angle N M Q is the left angle.
Translate vertex W to vertex M, then reflect across the line containing _______________.
Answers
Answer:
A. WX
Step-by-step explanation:
May I have brainliest please? :)
Triangle WXY can be mapped to triangle MNQ by:
Translating vertex W to vertex M.Then, reflect ΔWXY across the line containing line segment WX.What is transformation?Transformation involves changing the size and the position of a shape
From the figure (see attachment), we have the following highlights
Both triangles are not on the same height (i.e. translation is required)Both triangles are not on the same orientation (i.e. one of the triangles need to be reflected)Hence, Triangle WXY can be mapped to triangle MNQ by translating vertex W to vertex M, and then, reflecting ΔWXY across the line containing line segment WX.
Read more about transformation at:
https://brainly.com/question/4057530

A prescription drug company produces a capsule shaped like a cylinder with a hemisphere attached to each base. The cylinder and hemispheres all have a diameter of 8 millimeters, and the cylinder has a height of 15 millimeters. The outside of the capsule is coated with a film to improve the flavor and protect the medicine.What is the approximate area of the capsule that is coated with the film?
Answers
The two photos are both for the same question please help I dont know how to solve the revered graph


Answers
Answer:
point b is plotted incorrectly
Step-by-step explanation:
the number of contaminating particles on a silicon wafer prior to a certain rinsing process was determined for each wafer in a sample of size 100, resulting in the following frequencies: number of particles 0 1 2 3 4 5 6 7 frequency 1 2 3 12 11 15 18 10 number of particles 8 9 10 11 12 13 14 frequency 12 4 5 3 1 2 1 a. what proportion of the sampled wafers had at least one particle? at least five particles? b. what proportion of the sampled wafers had between five and ten particles, inclusive? strictly between five and ten particles? c. draw a histogram using relative frequency on the vertical axis. how would you describe the shape of the histogram?
Answers
The solution is,
1. a, 99% b71%
2. 64%, 44%
3. Number of particles 0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14
frequencies: 1, 2, 3, 12, 11, 15, 18, 10, 12, 4, 5, 3, 1, 2, 1
Relative frequencies 0.01, 0.02,0.03,0.12,0.11,0.15, 0.18,0.1, 0.12,0.04, 0.05,0.03,0.01,0.02,0.01
What is histogram?A histogram is an approximate representation of the distribution of numerical data.
here, we have,
Number of particles: 0, 1, 2, 3, 4, , 5, 6, 7
Frequency: 1, 2, 3, 12, 11, 15, 18, 10
Number of particles: 8, 9, 10, 11, 12, 13, 14
Frequency: 12, 4, 5, 3, 1, 2, 1
we solve by saying that . one of th smaples wafer is with any particles. we get the proportion by subtracting 1 from the total divided by 100
100-1/100
.99
at least five particles , then we can subtract the number of those with particles less than five from 100 , divided by 100
100-(11+12+3+2+1)=71/100
0.71 or 71%
b. those particles within 5 and 10 inclusive
15+18+10+12+4+5=64/100
0.64
0r 64%
five and 10 exclusively will be
18+10+12+4=44/100
0.44
44%
c. Draw an histogram. first we have frequency table first
Number of particles 0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14
frequencies: 1, 2, 3, 12, 11, 15, 18, 10, 12, 4, 5, 3, 1, 2, 1
Relative frequencies 0.01, 0.02,0.03,0.12,0.11,0.15, 0.18,0.1, 0.12,0.04, 0.05,0.03,0.01,0.02,0.01
Relative frequencies is derived by dividing each frequencies over the total frequencies
we can say that it is unimodal or say that it is slightly positively skewed
To learn more on histogram click:
brainly.com/question/17145899
#SPJ1

Pls show all steps and I will make youbrainlist
Use Tan Ratio
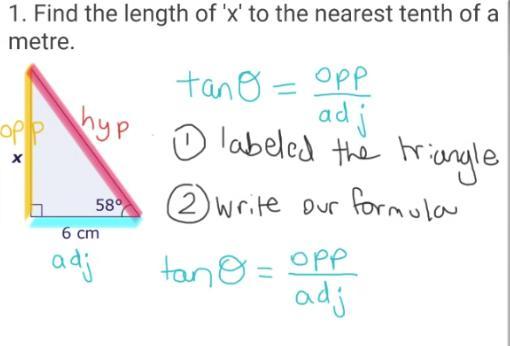
Answers
Answer:
x ≈ 9.6 cm
Step-by-step explanation:
tan58° = \(\frac{opposite}{adjacent}\) = \(\frac{x}{6}\) ( multiply both sides by 6 )
6 × tan58° = x , then
x ≈ 9.6 cm ( to the nearest tenth )
what are the roots of: y=x^2+3x-98
Answers
Answer:
Step-by-step explanation:

The endpoint of AB are A(9,-7) and B(8,2) find the coordinates of the midpoint M
Answers
Answer: (8.5, -2.5)
Step-by-step explanation:
The midpoint formula tells us the midpoint of two points...
\(M =( \frac{x1 + x2}{2} ,\frac{y1 + y2}{2})\)
And hopefully this makes sense; we are averaging the x and y values to find their "middle"!
plugging in, you'd get the midpoint to be (8.5, -2.5)
(2x^2 - 4x+1)+(5x+x^2 - 1) sum PLEASE QUICKKKKKK
Answers
In the coordinate plane, the point X (2, 2) is translated to the point X (0, 4). Under the same translation, the points Y(-2, 0) and Z (0, 6) are
translated to Y and Z', respectively. What are the coordinates of Y' and Z?
Answers
The points Y and Z after transition are (-4,-2) and (-2,4)
In this case, we are required to find x' and y' to find the direction of transition
x' =x coordinate of point 2 - x coordinate of point 1
= 0-2 = -2
y'= y coordinate of point 2 - y coordinate of point 1
=4-2 = 2
Now we are required to subtract these transition from the points given,
Transition of point Y = (-2-2 ,0-2) =(-4,-2)
Transition of point Z =(0-2,6-2) = (-2,4)
Hence the points after transition are Y as(-4,-2) and Z as (-2,4)
For further reference, use the given link:
https://brainly.com/question/18773856?referrer=searchResults
#SPJ9
Why is the Area for my shape not correct and can you explain why?

Answers
\(\bold{\huge{\underline{ Solution }}}\)
Here, We have given
2 squares , In which 1 square is enclosed within the another square and it arranged in a form that it forms 4 right angled triangle The height and base of the given right angled triangles are 6 and 3 each.We know that,
Area of triangle
\({\sf{=}}{\sf{\dfrac{1}{2}}}{\sf{ {\times} base {\times} height }}\)
Subsitute the required values,
\({\sf{=}}{\sf{\dfrac{1}{2}}}{\sf{ {\times} 3 {\times} 6}}\)
\(\sf{ = 3 {\times} 3 }\)
\(\bold{ = 9 }\)
Therefore,
Area covered by 4 right angled triangles
\(\sf{ = 4 {\times} 9 }\)
\(\bold{ = 36}\)
Now,
We have to find the area of the big square
The length of the side of the big square\(\sf{ = 6 + 3 = 9 }\)We know that,
Area of square
\(\sf{ = Side {\times} Side }\)
Subsitute the required values,
\(\sf{ = 9 {\times} 9 }\)
\(\bold{ = 81 }\)
Therefore,
The total area of shaded region
= Area of big square - Area covered by 4 right angled triangle
\(\sf{ = 81 - 36 }\)
\(\bold{ = 45 }\)
Hence, The total area of shaded region is 45 .
Part 2 :-Here,
We have to find the area of non shaded region
According to the question
Hypotenuse = The length of squareLet the hypotenuse of the given right angled triangle be x
Therefore,
By using Pythagoras theorem,
This theorem states that the sum of the squares of the base and perpendicular height is equal to the square of hypotenuse.That is,
\(\sf{ (Perpendicular)^{2} + (Base)^{2} = (Hypotenuse)^{2} }\)
Subsitute the required values
\(\sf{ (6)^{2} + (3)^{2} = (x)^{2} }\)
\(\sf{ 36 + 9 = (x)^{2} }\)
\(\sf{ x = \sqrt{45}}\)
\(\bold{ x = 6.7 }\)
That means,
The length of the small square = 6.7We know that ,
Area of square
\(\sf{ = Side {\times} Side }\)
Subsitute the required values,
\(\sf{ = 6.7 {\times} 6.7 }\)
\(\bold{ = 44.89 \:\: or \:\: 44.9 }\)
Therefore ,
Area of non shaded region
= Area of big square - Area of small square
\(\sf{ = 81 - 44.9 }\)
\(\bold{ = 36.1 }\)
Hence, The total area of non shaded region is 36.1 or 36 (approx) .
Part 3 :-Here, we have to
find the total area of the figure Therefore,The total area of the figure
= Non shaded region + Shaded region
\(\sf{= 36 + 45 }\)
\(\bold{= 81}\)
Hence, The total area of the given figure is 81 .
Graph 4/3 on the number line.

Answers
Answer:
The line on the right, closest to 1.
Step-by-step explanation:
We know since it is four thirds, that it is more than 1. That shows us it'll be at least on or over the 1 point.
Then we have one third, there's three points. Put it on the first point leading to 2.
Because you're working a late shift you will get increasing your wage you'll get additional 10% per hour from 6 pm to 12 am edit additional 50% from 12 am to what is it i am working from 2 pm to 12 am between 2 pm and 6 pm i will make $12 per hour between 6 pm and 12 am i will make per hour
Answers
Between 6:00 PM and 12:00 AM, I will make $13.20 per hour the answer is $13.20.
What is the percentage?It's the ratio of two integers stated as a fraction of a hundred parts. It is a metric for comparing two sets of data, and it is expressed as a percentage using the percent symbol.
The question is incomplete.
The complete question is:
Supervisor: "Because you are working a late shift, you will get an increase in your wage. You will get an additional 10% per hour from 6:00 PM to 12:00 AM and an additional 15% from 12:00 AM to 1:00 AM." Employee: "I am working from 2:00 PM to 12:00 AM. Between 2:00 PM and 6:00 PM, I will make $12.00 per hour. Between 6:00 PM and 12:00 AM, I will make __________ per hour."
We have:
Per hour wage = $12 per hour
The employee gets money for working from 6.00 PM to 12.00 AM :
= (10/100)×12
= $1.2 per hour
The employee gets money for working from 12.00 AM to 01.00 AM:
= (15/100)×12
= $1.8 per hour
An employee works from 2.00 PM to 12.00 AM:
The employee gets money for Working from 2.00 PM to 6.00 PM + 10%
= 12 + 1.20
= $13.20
Thus, between 6:00 PM and 12:00 AM, I will make $13.20 per hour the answer is $13.20.
Learn more about the percentage here:
brainly.com/question/8011401
#SPJ1
On a number line point is located at 2, point c is located at 5 and points b lies between points a and c what is location of b such that the ratio of ab :bc is 1:3
Answers
The location of point b n a number line with a point is located at 2 is 2.8.
What is ratio and proportions?According to the idea of ratio proportionality, two ratios are proportional if they are equal. To put it another way, if a/b = c/d, then an is to b what c is to d. Several branches of mathematics and science, including geometry, physics, and statistics, all make use of this idea. Also, it is useful in daily life when comparing product costs or figuring out cooking ratios.
Let the location of point b = x.
Using the ratio and proportion we have:
ab/bc = 1/3
Substituting ab and bc in terms of x we have:
(x-2)/(5-x) = 1/3
3x - 6 = 5 - x
x = 2.8
Hence, the location of point b n a number line with a point is located at 2 is 2.8.
Learn more about ratio here:
https://brainly.com/question/29774220
#SPJ1
Help anyone can help me do the question,I will mark brainlest.

Answers
Part (a)
The radius is r = 42 because OA = 42.
The circumference, aka distance around the circle, is
C = 2*pi*r
C = 2*(22/7)*42
C = 264
We're told that arc AB is 110 mm which is 110/264 = 5/12 of the full distance around the circle.
So we'll apply 5/12 to the full rotation 360 to get (5/12)*360 = 150
Answer: 150 degrees==============================================================
Part (b)
Compute the area of the full circle
A = pi*r^2
A = (22/7)*(42)^2
A = 5544
Then take 5/12 of this because we only want 5/12 of the full circle area (to get the area of the shaded pizza slice)
(5/12)*(5544) = 2310
Answer: 2310 square mm==============================================================
Side note: Both answers are approximate because pi = 22/7 is approximate.
In triangle ABC, AC = 21, BC = 28, and ∠ACB = 90◦
. The bisector of ∠ACB meets AB at D.
Find the length BD and CD
Sorry don't have image
Answers
The length of BD and CD are 15 units and 20 units respectively
How to find the length BD and CD?By the Angle Bisector Theorem, we have:
BD/DC = AC/BC = 21/28
We can use the Pythagorean Theorem to find AC:
AC² + BC² = AB²
21² + 28² = AB²
AB = 35 units
Now we can use the Angle Bisector Theorem to find BD:
BD/DC = 21/28
BD + DC = AB
BD = (21/49) × AB
BD = (21/49) × 35
BD = 15 units
To find CD, we can use the fact that BD + DC = AB:
CD = AB - BD
CD = 35 - 15
CD = 20 units
Therefore, the length are BD = 15 and CD = 20 units.
Learn more about angle bisector theorem on:
brainly.com/question/24334771
#SPJ1