What polynomial must be added to 3x^2 +4x + 7 to obtain a sum of 0?
Answers
Answer:
\( - 3x^2 - 4x - 7\)
Step-by-step explanation:
Let A should be added in the given polynomial to obtain a sum of zero.
Therefore,
\(A + 3 {x}^{2} + 4x + 7= 0 \\ \\ A = 0 - (3 {x}^{2} + 4x + 7 ) \\ \\ A = 0 - 3 {x}^{2} - 4x - 7 \\ \\ A = - 3 {x}^{2} - 4x - 7 \\ \)
Thus, \( - 3 {x}^{2} - 4x - 7\) should be added in the given polynomial to obtain a sum of zero.
The polynomial which must be added to 3x² +4x + 7 to obtain a sum of 0 is; -3x² -4x +7.
Let the polynomial which must be added to 3x² +4x + 7 to obtain a sum of 0 be represented by P.
In essence;
P + 3x² +4x + 7 = 0.By subtracting 3x² +4x + 7 from both sides of the equation to obtain the value of P; we have;
P = 0 -3x² -4x +7.Ultimately, P = -3x² -4x +7.
Read more on polynomials;
https://brainly.com/question/2833285
Related Questions
I will give brainliest and ratings if you get this correct

Answers
Using Cramer's rule for first-order condition:
x₁ = -149/444x₂ = -69/222x₃ = 139/444Using Hessian for the second-order condition, critical point (x₁, x₂, x₃) = (-149/444, -69/222, 139/444) is the unique minimum of y.
How to determine 1st and 2nd order condition?(a) Using Cramer's rule for the first-order condition:
To optimize the function y, find the critical points where the gradient is equal to zero. The gradient of y is given by:
∇y = [6x₁ - x₂ - 3x₃ - 5, -x₁ + 12x₂ + 2x₃ - 4, 2x₂ + 8x₃ + 2 - 3x₁]
Setting the gradient equal to zero:
6x₁ - x₂ - 3x₃ - 5 = 0 (1)
-x₁ + 12x₂ + 2x₃ - 4 = 0 (2)
2x₂ + 8x₃ + 2 - 3x₁ = 0 (3)
Using Cramer's rule to solve this system of linear equations, the determinant of the coefficient matrix is:
|A| =
| 6 -1 -3 |
|-1 12 2 |
|-3 2 -3|
|A| = 444
The determinant of the matrix obtained by replacing the first column of A with the constants on the right-hand side of the equations is:
|A₁| =
| 5 -1 -3 |
| 0 12 2 |
| 0 2 -3|
|A₁| = -149
The determinant of the matrix obtained by replacing the second column of A with the constants is:
|A₂| =
| 6 5 -3 |
|-1 0 2 |
|-3 0 -3|
|A₂| = -138
The determinant of the matrix obtained by replacing the third column of A with the constants is:
|A₃| =
| 6 -1 5 |
|-1 12 0 |
|-3 2 2|
|A₃| = -278
Therefore, using Cramer's rule:
x₁ = |A₁|/|A| = -149/444
x₂ = |A₂|/|A| = -69/222
x₃ = |A₃|/|A| = 139/444
(b) Using the Hessian for the second-order condition:
To check whether the critical point found in part (a) is a maximum, minimum or saddle point, we need to use the Hessian matrix evaluated at the critical point. The Hessian of y is given by:
(y) =
| 6 0 -3 |
| 0 12 2 |
|-3 2 8 |
Evaluating H(y) at the critical point (x₁, x₂, x₃) = (-149/444, -69/222, 139/444):
H(y) =
| 6 0 -3 |
| 0 12 2 |
|-3 2 8 |
The eigenvalues of H(y) are 2, 6, and 18, which are all positive. Therefore, H(y) is positive definite, and the critical point is a minimum.
Therefore, the critical point (x₁, x₂, x₃) = (-149/444, -69/222, 139/444) is the unique minimum of y.
Find out more on first-order condition here: https://brainly.com/question/31451579
#SPJ1
A blueprint of a house shows a kitchen that is 4 inches long and 3 inches wide. The actual kitchen will be 16 feet long. How wide will
the actual kitchen be?
Answers
Answer:
12 feet wide
Step-by-step explanation:
We are solving for a ratio between the long side of the actual kitchen, and the long side of the house on the blueprint. To find this ratio, we divide 16 by 4 to get 4:
16/4=4
Therefore, our ratio is 4. We can multiply the width of the blueprint length (3) by the the ratio of the real width:
3*4=12
Therefore, the actual kitchen will be 12 feet wide.
Solve for x: 3(x+1) = -6
A.-2
B.-3
C. 1
D.7/3
Answers
Answer: x = -3
Step-by-step explanation:
3(x + 1) = -6
Divide both sides by 3: 3(x + 1) / 3 = -6 / 3
x + 1 = -2
Subtract 1 from each side: x + 1 - 1 = -2 - 1
x = -3
Mariah is a manager of a fitness training center that serves a certain region. She is interested in the amount of water adult residents in her region consume on average per day. She conducts a survey, where she selects 645 adult residents from the region at random and asks each individual to track how much water, in fluid ounces he or she consumes on average per day Among the 645 in the sample, the average is approximately 62.7 fluid Ounces Explain whether the results of Mariah's survey could be extended to all the residents of the region (1 point)
A) The results of the survey could be extended to all residents of the region because Mariah's fitness training center serves the region that contains the population of interest.
B) The results of the survey cannot be extended to all residents of the region because Maria only selected adult residents of the region at random.
C) The results of the survey could be extended to all residents of the region because all of the individuals in Mariah's sample are residents of the region
D)The results of the survey could be extended because the adult residents in Mariah's sample are representative of al the residents of the region.
Answers
HELP ASAP Subtract the expressions.
(9.12n − 4.3) − (8 + 13.6n)
−44.80n − 4.5
−4.48n − 12.3
13.42n − 21.6
22.72n + (−12.3)
Answers
The result of subtracting the expressions is -4.48n - 12.3. The correct option is the second option −4.48n − 12.3
Subtracting expressionsFrom the question, we are to evaluate the expression by subtracting
From the given information,
The given expression is
(9.12n − 4.3) − (8 + 13.6n)
Now, we will simplify the expression
(9.12n − 4.3) − (8 + 13.6n)
Clear the parentheses by applying the distributive property
9.12n - 4.3 - 8 - 13.6n
Collect like terms
9.12n - 13.6n - 4.3 - 8
Simplify further
-4.48n - 12.3
Hence, the expression is -4.48n - 12.3
Learn more on Subtracting expressions here: https://brainly.com/question/21374487
#SPJ1
What is the value of n 8n-5=139
Answers
The value of 'n' is 18 in the given equation 8n - 5 = 139.
We have to find the value of 'n'.
To do so, we need to solve the given equation.
Let us see how we can solve the given equation.8n - 5 = 139
We need to find the value of 'n'.
Let us bring the constant term to the right side by adding 5 to both the sides of the equation.8n - 5 + 5 = 139 + 5On simplifying, we get8n = 144Now, we need to isolate 'n' to find its value.
To do so, we divide both the sides by 8.8n/8 = 144/8On simplifying, we get n = 18
Therefore, the value of 'n' is 18.
Hence, the value of 'n' is 18 in the given equation 8n - 5 = 139.
For more questions on equation
https://brainly.com/question/17145398
#SPJ8
If you flip two coins 68 times, what is the best prediction possible for the number of times both coins will land on heads?the number of times it will land on tails?
Answers
If you flip two coins 68 times, the best prediction for the number of times both coins will land on heads is 17.
If you flip two coins 68 times, the best prediction for the number of times both coins will land on tails is 17.
What is the probability of getting two heads?The possible outcomes when two coins are flipped are;
head - head = HH
tail - tail = TT
Head and tail = HT
Tail and head = TH
The probability of getting two heads = 1/4
If you flip two coins 68 times, the best prediction for the number of times both coins will land on heads is calculated as;
Expected value = (68 flips) x (1/4 probability of getting HH) = 17
The probability of getting two tails = 1/4
Expected value = 68 x 1/4 = 17
Learn more about probability here: https://brainly.com/question/24756209
#SPJ1
4 x 2/7 Pls solve it for me
Answers
Answer:
8/7 or 1 1/7
Step-by-step explanation:
4/1 x 2/7 = 8/7
(And as a mixed fraction it’s 1 1/7)
After watching baking shows on T.V., Angie signs up for a cake-decorating class. To practice her new skills, she decorates a batch of cupcakes with sugar flowers. Angie puts 4 sugar flowers on each cupcake. In all, Angie puts 32 sugar flowers on the cupcakes.
Which equation can you use to find the number of cupcakes c Angie decorates?
Solve this equation for c to find the number of cupcakes Angie decorates.
Answers
Angie decorates 8 cupcakes after putting 4 sugar flowers on each cupcake.
To find the number of cupcakes c Angie decorates, we can use the equation:4c = 32where 'c' is the number of cupcakes Angie decorates.
4 represents the number of sugar flowers Angie puts on each cupcake, and 32 is the total number of sugar flowers Angie puts on the cupcakes.
To solve this equation for c, we need to isolate c on one side of the equation. We can do this by dividing both sides of the equation by 4. This gives us:c = 8
Therefore, Angie decorates 8 cupcakes.Here's how we get to this answer:4c = 32Divide both sides by 4 to isolate c:c/4 = 32/4c = 8
For more questions on isolate
https://brainly.com/question/11389214
#SPJ8
An archaeologist found a fossil whose length is489.44 in.
Consult the conversion table to calculate the length of the fossil infeet.
Round your answer to the nearest tenth.
Answers
If the archaeologist found a fossil whose length is 489.44 inches, using the conversion table, the length in feet, to the nearest tenth, is 40.8 feet.
What is the conversion table?The conversion table refers to the tabulated standard units of measurement, showing temperature, length, area, volume, weight, and metric conversions
For instance, the conversion table shows that 12 inches equal 1 foot.
The length of the fossil = 489.44 inches
12 inches = 1 foot
489.44 inches = 40.8 feet (489.44 ÷ 12)
Thus, using the conversion table, which relies on multiplication or division operations using the conversion factors, the length in feet of the fossil is 40.8 feet.
Learn more about conversion tables at https://brainly.com/question/29255897.
#SPJ1
Evaluate the double integral ∬R(3x−y)dA, where R is the region in the first quadrant enclosed by the circle x2+y2=16 and the lines x=0 and y=x, by changing to polar coordinates.
Answers
Answer:
\(\displaystyle 64-32\sqrt{2}+\frac{32\sqrt{2}}{3}\approx3.66\)
Step-by-step explanation:
\(\displaystyle \iint_R(3x-y)\,dA\\\\=\int^\frac{\pi}{2}_\frac{\pi}{4}\int^4_0(3r\cos\theta-r\sin\theta)\,r\,dr\,d\theta\\\\=\int^\frac{\pi}{2}_\frac{\pi}{4}\int^4_0(3r^2\cos\theta-r^2\sin\theta)\,dr\,d\theta\\\\=\int^\frac{\pi}{2}_\frac{\pi}{4}\int^4_0r^2(3\cos\theta-\sin\theta)\,dr\,d\theta\\\\=\int^\frac{\pi}{2}_\frac{\pi}{4}\frac{64}{3}(3\cos\theta-\sin\theta)\,d\theta\\\\=\int^\frac{\pi}{2}_\frac{\pi}{4}\biggr(64\cos\theta-\frac{64}{3}\sin\theta\biggr)\,d\theta\)
\(\displaystyle =\biggr(64\sin\theta+\frac{64}{3}\cos\theta\biggr)\biggr|^\frac{\pi}{2}_\frac{\pi}{4}\\\\=\biggr(64\sin\frac{\pi}{2}+\frac{64}{3}\cos\frac{\pi}{2}\biggr)-\biggr(64\sin\frac{\pi}{4}+\frac{64}{3}\cos\frac{\pi}{4}\biggr)\\\\=64-\biggr(64\cdot{\frac{\sqrt{2}}{2}}+\frac{64}{3}\cdot{\frac{\sqrt{2}}{2}}\biggr)\\\\=64-32\sqrt{2}+\frac{32\sqrt{2}}{3}\biggr\\\\\approx3.66\)
Help please!! Find the equation of the linear function represented by the table below in slope-
intercept form.
Answer:
X
1
2
3
4
y
-1
-3
-5
-7
Answers
The slope intercept form is y= 2x+1
What is slope ?
A line's steepness is determined by its slope. In mathematics, slope is determined by "rise over run" (change in y divided by change in x).
An equation is a mathematical statement that proves two mathematical expressions are equal in algebra, and this is how it is most commonly used. In the equation 3x + 5 = 14, for instance, the two expressions 3x + 5 and 14 are separated.
Let's take 2 points :
(x1,y1) = ( 1, -1)
(x2,y2) = ( 2, -3)
slope , m = (y2-y1) /(x2-x1)
= (-3-(-1))/(2-1)
= (-3+1)/1
= -2
Slope intercept form:
y-y1=m(x-x1)
y-(-1) = -2 (x-1)
y+1=-2x+2
=> y=2x+2-1
=> y= 2x+1
Thus, the slope intercept form is y= 2x+1
To learn more about the slope from the given link
https://brainly.com/question/16949303
#SPJ1
R varies directly as S and inversely as T. if R = 8 when S = 4 and T = 3, then R is equal to
Answers
Answer:
R = 6S/T
Step-by-step explanation:
R ∝ S/ T
》R = kS/ T
》k = RT/ S
If R = 8 when S = 4 and T = 3
then, k = (8 × 3)/ 4
k = 6
∴ R = (6 × S)/ T
Rewrite in radical form

Answers
Answer:
6 1/3
Step-by-step explanation:
URGENT DUE IN FEW MINS6th grade math Calculate to TOTAL Surface Area.
Do NOT count the surface under the pyramid.

Answers
The surface area of the solid is 281 ft².
Given that, a solid figure made of a rectangular prism and a square pyramid,
We need to find its surface area,
To find the same, we will find the lateral surface area of square pyramid, and total surface area of rectangular prism,
So,
Surface area = 2×side×slant height + 2(length·width+width·height+height·length)-base area of the square pyramid,
= 2×5×5 + 2(4·12+12·5+4·5)-5²
= 50+256-25
= 281 ft²
Hence the surface area of the solid is 281 ft².
Learn more about surface area click;
https://brainly.com/question/29298005
#SPJ1
change each mixed number into an improper fraction 1 2/3
Answers
Answer:
5/3
Step-by-step explanation:
Multiply the denominator by the whole number
3 x 1= 3
Add the answer from above to the numerator
3 + 2= 5
Write the answer from the step above over to the denominator
5/3
6+r=2r+3 how to solve this
Answers
Answer:
soln
6+r =2r+3
6-3=2r-r
3=r
ans r=3Patrick managed to sell the 20th most bicycles last year of all the salespeople at the company.He also managed to sell the 20th least bicycles last year of all the salespeople at that company.How many salespeople were there at that company?Assume that no one sold the same number of bicycles than another
Answers
definitely missing a number so it's prolly something like
a/20=20
sorry if this doesn't help you didn't give us much to work on
Help plz aidjkeksnszhosksoaoaoaozizbzbzjsijssj
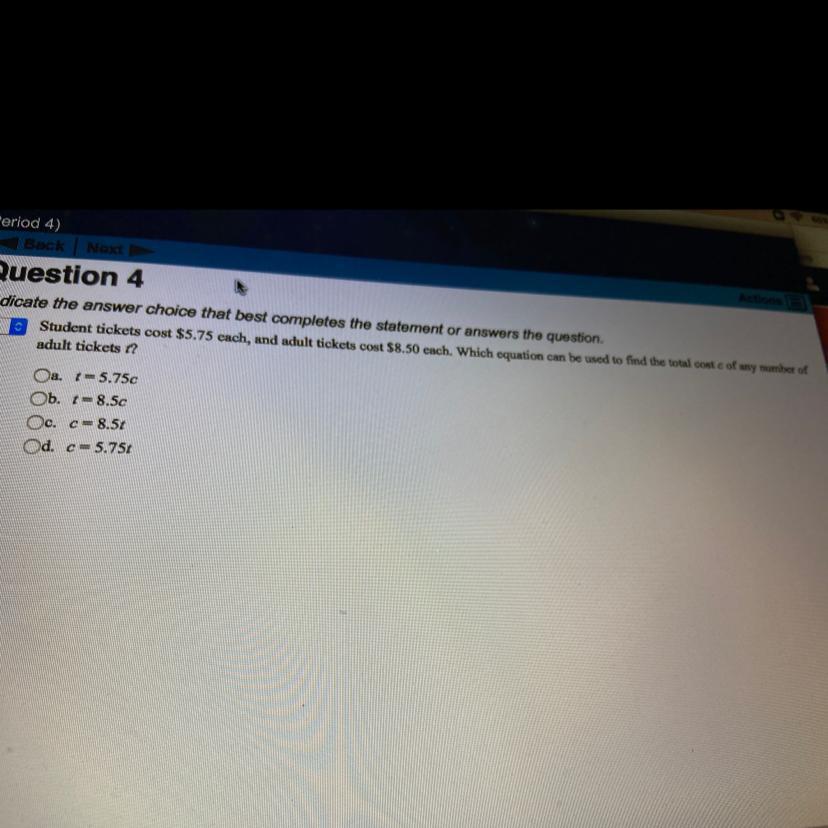
Answers
Mrs alvares rents skis and poles for 3 days what is the total cost of rental

Answers
The total cost of rents is $180.
In the given table,
The cost of skis per day = $48
The cost of pole per day = $12
Now since given that,
Alveres rents for 3 days
Therefore,
The cost of skis for 3 days = $48 x 3
= $144
The cost of pole for 3 days = $12 x 3
= $36
To find the total cost of rental,
Adding the cost of 3 days of skis and cost of 3 days of poles,
Hence,
Total cost of rents = $144 + $36
= $180
Learn more about the addition visit:
https://brainly.com/question/25421984
#SPJ1
Let XY be a random draw from the following box of tickets0 0 1 1 21 2 0 1 0Find E(X+Y)^2
Answers
We can start by finding the expected value of X and Y separately: (X) = (0+0+1+1+2+1+0+1+0)/9 = 6/9 = 2/3, E(Y) = (0+0+1+1+2+0+1+0+0)/9 = 5/9
Next, we can expand (X+Y)^2: (X+Y)^2 = X^2 + 2XY + Y^2
Taking the expected value of both sides, we get: E((X+Y)^2) = E(X^2) + 2E(XY) + E(Y^2)
To find E(XY), we can create a table of the possible values of X and Y, and their corresponding probabilities: [table in the attatchment]
So, we have:
E(XY) = 0*(3/9) + 0*(2/9) + 0*(1/9) + 0*(2/9) + 1*(1/9) + 2*(1/9) + 0*(1/9) + 2*(1/9) + 4*(1/9) = 1
Next, we need to find E(X^2) and E(Y^2): E(X^2) = (0^2+0^2+1^2+1^2+2^2+1^2+0^2+1^2+0^2)/9 = 8/9
E(Y^2) = (0^2+0^2+1^2+1^2+2^2+0^2+1^2+0^2+0^2)/9 = 5/9
Putting it all together, we get: E((X+Y)^2) = E(X^2) + 2E(XY) + E(Y^2) = 8/9 + 2*1 + 5/9 = 23/9. Therefore, the answer is 23/9.
You can read more about random draw at https://brainly.com/question/30560729
#SPJ4

Which of the following is equivalent to the complex number i^32
Answers
Answer:
What?
Step-by-step explanation:
a textbook store sold a combined total of 366 psychology and history textbooks in a week. The number of history textbooks sold was 40 less than the number of psychology textbooks sold. how many textbooks of each type were sold?
Answers
Answer:
Step-by-step explanation:
We have two types of textbooks. Let's use "x" to represent the number of math textbooks and "y" to represent the number of history textbooks.
Altogether, the bookseller sold 240 math and history textbooks. So:
x + y = 240
Now, the number of history textbooks sold was 72 less than the number of math books sold. So:
y = x - 72
Now we can substitute for y in the equation and solve:
x + y = 240
x + (x - 72) = 240
2x - 72 = 240
2x = 312
x = 156
The bookseller sold 156 math textbooks.
Since y = x - 72, he must have sold 84 history textbooks.
Let's check our answers:
x + y = 240
156 + 84 = 240
That is true, so we did get the correct answers
$2.95+$3.09+$5.85+$4.10
Answers
Step-by-step explanation:
the value of P(B|A)P(B∣A), rounding to the nearest thousandth
$15.99
Explanation:
All you have to do is add them together
What is the area of
the segment? Express
the answer in terms
of pi.

Answers
The area of the segment is 9( π-2) units²
What is area of segment?The area of a figure is the number of unit squares that cover the surface of a closed figure.
A segment is the area occupied by a chord and an arc. A segment can be a major segment or minor segment.
Area of segment = area of sector - area of triangle
area of sector = 90/360 × πr²
= 1/4 × π × 36
= 9π
area of triangle = 1/2bh
= 1/2 × 6²
= 18
area of segment = 9π -18
= 9( π -2) units²
therefore the area of the segment is 9(π-2) units²
learn more about area of segment from
https://brainly.com/question/22599425
#SPJ1
based on the graph how many tiles are im figure 0

Answers
For figure {0}, the number of tiles will be equal to 2.
What is a mathematical function, equation and expression?Function : In mathematics, a function from a set X to a set Y assigns to each element of X exactly one element of Y. The set X is called the domain of the function and the set Y is called the codomain of the function
Expression : A mathematical expression is made up of terms (constants and variables) separated by mathematical operators
Equation : A mathematical equation is used to equate two expressions.
Given is a graph as shown in the image.
The line passes through point -
(3, 8) and (4, 10)
So, the slope of the line will be -
m = (10 - 8)/(4 - 3)
m = 2
y = 2x + c
For the point (3, 8), we can write -
8 = 6 + c
c = 2
For figure {0}, the number of tiles will be equal to 2.
Therefore, for figure {0}, the number of tiles will be equal to 2.
To solve more questions on functions, expressions and polynomials, visit the link below -
brainly.com/question/17421223
#SPJ1
Fill in the blanks to complete the rule

Answers
uh i could never, sorry
A $25,000 deposit for 4 years at a 3.56% compounded
continuously.
Principal:
Interest:
Balance:
Answers
Answer:
B. principal
Step-by-step explanation:
A ball is thrown upward with an initial velocity of 60 mph. it is thrown from a height of 5 feet. what is the maximum height it reaches?
Answers
Answer:
h = 61.25 m
Step-by-step explanation:
It is given that,
The initial velocity of the ball, v = 60 m/s
It is thrown from a height of 5 feet, \(h_o=5\ ft\)
We need to find the maximum height it reaches. The height reached by the projectile as a function of time t is given by :
\(h=-16t^2+vt+h_0\)
Putting all the values,
\(h=-16t^2+60t+5\) .....(1)
For maximum height, put
\(\dfrac{dh}{dt}=0\\\\\dfrac{-16t^2+60t+5}{dt}=0\\\\-32t+60=0\\\\t=\dfrac{-60}{-32}\\\\t=1.875\ s\)
Put t = 1.875 in equation (1)
\(h=-16(1.875)^2+60(1.875)+5\\\\h=61.25\ m\)
So, the maximum height reached by the ball is 61.25 m.
What translation would map C onto A?
A. (x – 7, y + 3)
B. (x + 3, y – 7)
C. (x – 3, y + 7)
D. (x + 7, y – 3)

Answers
Answer:
A
Step-by-step explanation:
consider the coordinates of points C and A
C (4, - 1 ) and A (- 3, 2 )
4 → - 3 in the x- direction is - 7
- 1 → 2 in the y- direction is + 3
then the translation rule is
(x, y ) → (x - 7, y + 3 )