what is the range of this exponiental function y=6×
Answers
Range of the Exponential Function
The range of a function y = f(x) is the set of all the functions' values when x moves through its domain.
It's required to find the range of the function:
\(y=6^x^{}\)The domain of the function is the set of all the real numbers because x can be given any possible value and y would still exist.
For example, let's give x the values x={-3,-1,0,2,4}
\(f(-3)=6^{-3}=0.005\)\(f(-1)=6^{-1}=0.167\)\(f(0)=6^0=1\)\(\begin{gathered} f(2)=6^2=36 \\ f(4)=6^4=1296 \end{gathered}\)It's clear that for negative values of x, the function tends to zero and for positive values, the function tends to infinity.
Thus, the range of the function is (0,
Related Questions
Mike borrowed some money for 2 years at 8% simple interest. If he had to pay back a total of $4060 how much did he borrow, how much did he originally borrow?
Answers
Answer:P = $ 25,375.00
Step-by-step explanation:
P = 4060 / ( 0.08 × 2 ) = 25375
P = $ 25,375.00
Solve the following inequality.
8x-1<15
Answers
Answer:
the answer is X<2
Step-by-step explanation:
ok so first, 8x-1<15
add one to both sides, 8x<16
divide both sides by 8, x<2
your final answer is x<2
Hope this helps! :)
g Let X and Y be two independent standard normal random variables, i.e., each follows the distribution N (0, 1). Now, define two new random variables as Find cov(Z, W)
Answers
Answer: hello your question is incomplete below is the complete question
Let X and Y be two independent standard normal random variables, i.e., each follows the distribution N (0, 1). Now, define two new random variables as Z = 11 - X + X²Y, W = 3-Y. find cov ( Z, W )
answer : Cov ( Z, W ) = -1
Step-by-step explanation:
Z = 11 - X + X²Y
w = 3 - Y
Cov ( Z, W ) = Cov ( 11 - x + x^2 Y , 3 - Y )
= Cov ( 11, 3 ) - Cov ( 11, Y ) - Cov(x,3) + cov (x,y) + cov(x^2y, 3) -cov (x^2y, y )
attached below is the remaining part of the detailed solution
hence
Cov ( Z, W ) = -1

Let V be the space spanned by the two functions cos(t) and sin(t). Find the matrix A of the linear transformation T(f(t))=f′′(t)+3f′(t)+7f(t) from V into itself with respect to the basis {cos(t),sin(t)}.
Answers
Answer: The matrix A of the linear transformation T(f(t)) = f''(t) + 3f'(t) + 7f(t) from the space spanned by the functions cos(t) and sin(t) into itself with respect to the basis {cos(t), sin(t)} can be found by computing the images of the basis vectors under T and expressing those images as linear combinations of the basis vectors.
We have:
T(cos(t)) = -cos(t)'' - 3cos(t)' - 7cos(t) = -cos(t) - 3(-sin(t)) - 7cos(t) = -8cos(t) - 3sin(t)
T(sin(t)) = -sin(t)'' - 3sin(t)' - 7sin(t) = -sin(t) - 3cos(t) - 7sin(t) = -8sin(t) + 3cos(t)
So, with respect to the basis {cos(t), sin(t)}, the matrix A is:
A = [ -8, -3; 3, -8 ]
This is the matrix representation of the linear transformation T with respect to the basis {cos(t), sin(t)}.
Step-by-step explanation:
Please help giving brainliest please help

Answers
Answer:
G
Step-by-step explanation:
In the equation, 8.5 will represent how much money Oscar earns per hour, and the x represents how many hours he works. The inequality sign shows that the sum of 8.5x and 100 has to be at least 397.50 (397.50 and up), so H and J are automatically wrong. If Oscar had given his brother 100 dollars, there would be a subtraction sign, but there is an addition sign instead, meaning he gained 100 dollars that did not come from his job. It would be the most logical to say that his brother had given it to him, so G is the correct answer.
Please look at the picture and answer it.

Answers
Answer:
y=24
Step-by-step explanation:
4y+7+3y+5=180
7y=168
y=24
Distributive property to simplify 6(8) + 6(-2)
Answers
Answer:
36
Step-by-step explanation:
You would multiply 6x8+6x(-2), and that would equal 48+(-12) which is basically 48-12 = 36.
PLEASE ANSWER AND EXPLAIN I’LL MARK BRAINLIEST

Answers
let A(-1,2)andB(3,7)be any two points.
slope of lineAB=put here distance formula
=4-7/5-3=3/2=1
6. The fixed costs of producing a Wild Widget are $34,000. The variable costs are $5.00 per widget. What is the average cost per widget of producing 7,000 Wild Widgets? Round to the nearest cent. :))))
Answers
Answer: To calculate the average cost per widget, we need to consider both the fixed costs and the variable costs.
Fixed costs: $34,000
Variable costs per widget: $5.00
Total costs = Fixed costs + (Variable costs per widget × Number of widgets)
Total costs = $34,000 + ($5.00 × 7,000)
Total costs = $34,000 + $35,000
Total costs = $69,000
Average cost per widget = Total costs / Number of widgets
Average cost per widget = $69,000 / 7,000
Average cost per widget ≈ $9.86
Therefore, the average cost per widget of producing 7,000 Wild Widgets is approximately $9.86.
Step-by-step explanation: :)
I WILL GIVE BRAINLIEST PLEASE HELP Picasso's Phone Shop charges $33.16 for its activation fee plus $5.63 for every gigabyte, g, of data you use over your limit. The equation that
models the total cost (T) is T (9) = 5.639 + 33.16 Which value in the equation represents the rate of change?
A
the number of gigabytes used over
B
$5.63
$33.16
D
$38.79
E
I don't know yet
Answers
Answer:
i think it is $5.63. I might be wrong....
Step-by-step explanation:
because depending on how much gb of data you use the price can change.
5.63 for gigabyte.
increases by 5.63 every time you use more Gb
increase rate of change is 5.63.
Sharlene wraps a cylindrical package that has a height of 15 inches and
a radius of 3 inches. What is the package’s surface area? Use 3.14 for
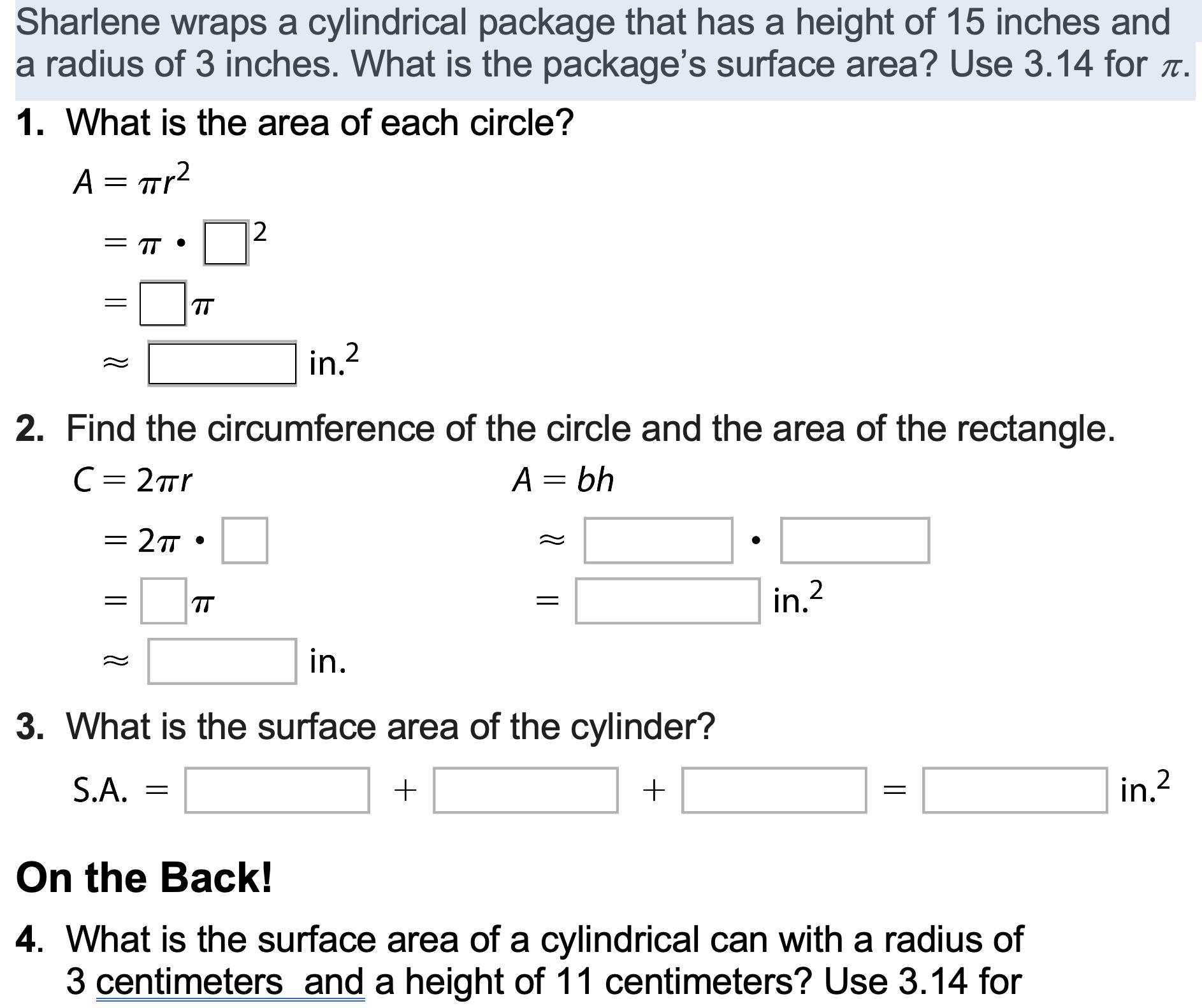
Answers
Answer:
Surface Area = (2 • π • r²) + (2 • π • r • height)
Surface Area = 2 * 3.14 * 3*3 + 2 * 3.14 * 3 * 15
Surface Area = 56.52 + 282.60
Surface Area = 339.12
Formulas: http://www.1728.org/diamform.htm
Step-by-step explanation:
A rock is thrown upward with a velocity of 22
meters per second from the top of a 45
meter high cliff, and it misses the cliff on the way back down. When will the rock be 9
meters from ground level? Round your answer to two decimal places.
Gravity Formula
Answers
The rock will be 9 meters from ground level at a time of:
x = 5.76 seconds.
How to model the situation?The quadratic function that models a projectile's height is given as follows:
y = -4.9t² + v(0)t + h(0)
In which:
v(0) is the initial velocity.h(0) is the initial height.The parameters for this problem are given as follows:
v(0) = 22, h(0) = 45.
Hence the height function is given as follows:
y = -4.9t² + 22t + 45.
The rock is 9 meters from ground level when:
9 = -4.9t² + 22t + 45.
-4.9t² + 22t + 36 = 0
4.9t² - 22t - 36 = 0.
Hence the discriminant is given as follows:
D = 22² - 4(4.9)(-36)
D = 1189.6.
The positive root, representing the time for the height of 9 meters, is given as follows:
x = (22 + sqrt(1189.6))/9.8
x = 5.76 seconds.
More can be learned about quadratic functions at https://brainly.com/question/1214333
#SPJ1
PLS HELP will give brainliest answer both

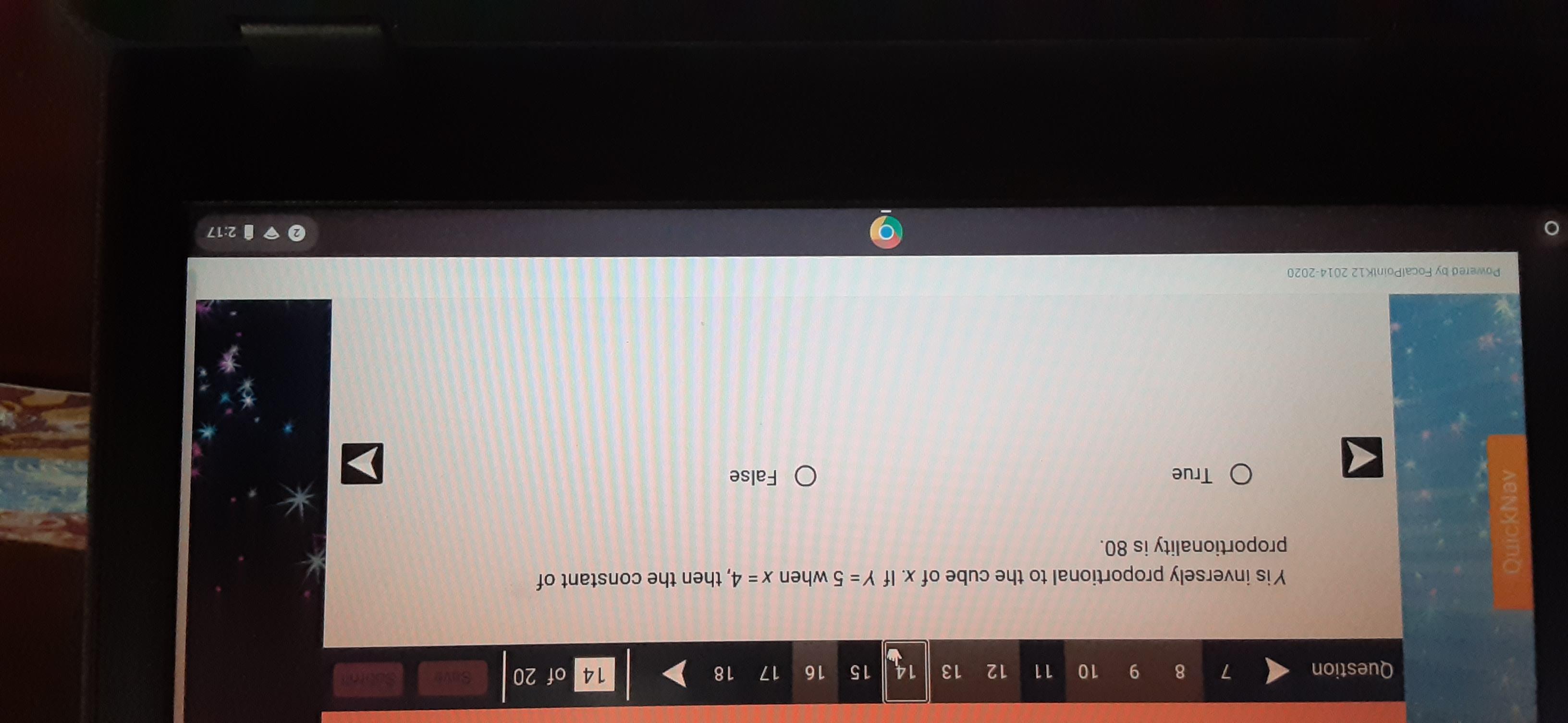
Answers
Answer:
8 3/5
false
Step-by-step explanation:
The parking garage at the airport has 72 empty parking spaces and 28 full parking spaces.
What percentage of the spaces in the garage are empty?
Answers
Answer:
72% of the parking spaces are empty.
Step-by-step explanation:
There are 72 open spaces out of 100 total. 72/100=.72, .72*100= 72%.
Answer:
72% because 72+28 is 100 and 72/100 is 72%
Step-by-step explanation:
I also need help on this, it would mean millions to me as this is for a grade. :)

Answers
Answer:
The answer is the 3rd one, not equivalent. 8x+4y=4(2x+y)
who can do it? Try to solve it if u can

Answers
Answer:
The other girls answer was correct
for the top boxes from left to right...
3.5 plus 4.5
bottom boxes
9.5 minus 3.5
Step-by-step explanation:
Use the image below to solve
Please HELP

Answers
The value of x is given as follows:
x = 18.
What are similar triangles?Similar triangles are triangles that share these following two features:
Congruent angle measures.Proportional side lengths.The similar triangles for this problem are listed as follows:
ABD and EDC.
The proportional side lengths are given as follows:
x and 6.12 and 4.Hence the value of x is obtained as follows:
x/6 = 12/4
x/6 = 3.
x = 18.
(using the proportional relationship for the equivalent side lengths of the similar triangles).
More can be learned about similar triangles at brainly.com/question/14285697
#SPJ1
What is the probability that exactly 7 of the ten will get Huntington’s disease?
Answers
As a result, the likelihood that 7 out of every 10 people will develop Huntington's illness is 0.9375, or almost 93.75%.
The probability formula is what?The probability is typically stated to be the proportion of favorable events to every other result in the sample. The likelihood of an occurrence P(E) = (Number of favorable outcomes) is how it is stated (Sample space).
The binomial likelihood formula must be used to determine the likelihood that precisely seven out of ten people will develop Huntington's disease:
\(P(X = k) = (n choose k)\) × \(p^k (1 - p)^(n - k)\)
where the likelihood of obtaining k successes is P(X = k).
The number of trials is n.
The binomial coefficient, n pick k, denotes the number of possible methods to select k successes from n trials, where p is the success rate in each trial.
In this instance, n = 10 (since there are 10 people)
As we are interested in the likelihood that exactly 7 persons will have Huntington's illness, we set k = 7.
p = 0.5 (because there is a 50% chance that each person will inherit the gene)
(n choose k) = 10 select 7 = 120 (since there are 120 ways to choose 7 people from 10)
Given these numbers, we can get the probability using the formula below:
\(P(X = 7) = (10 choose 7)\)× \(0.5^7\) × \((1 - 0.5)^(10 - 7)\)
= 120 × \(0.5^7\) × \(0.5^3\)
= 120 × 0.0078125
= 0.9375
To know more about Probability visit:
https://brainly.com/question/11234923
#SPJ1
an art gallery is selling replicas of some famous art work. a copy of the art work is dilated by a scale factor of 1/3 to create a replica of the original piece. if the area of some original artwork was 12 square feet what will be the area of the replica?
Answers
Answer:
4/3 square feet.
Step-by-step explanation:
To find the area of the replica, you need to use a formula that relates the area of the original figure and the area of the dilated figure by the scale factor1. The formula is:
Area of dilated figure = Area of original figure x (Scale factor)^2
In this case, the area of the original artwork is 12 square feet and the scale factor is 1/3. So, we plug these values into the formula and get:
Area of replica = 12 x (1/3)^2
Area of replica = 12 x 1/9
Area of replica = 4/3
So, the area of the replica is 4/3 square feet.
Hope this helps :)
Are the experimental probabilities after 300 trials closer to the theoretical probabilities?
Answers
After 300 trials, the experimental probabilities may not align perfectly with the theoretical probabilities. However, with more trials, the experimental probabilities tend to converge towards the theoretical probabilities for closer alignment.
To examine whether experimental probabilities after 300 trials align closely with theoretical probabilities, let's consider an example of flipping a fair coin.
Theoretical probability: When flipping a fair coin, the theoretical probability of obtaining heads or tails is 0.5 each. This assumes that the coin is unbiased and has an equal chance of landing on either side.
Experimental probability: After conducting 300 trials of flipping the coin, we record the outcomes and calculate the experimental probabilities. Let's assume that heads occurred 160 times and tails occurred 140 times.
Experimental probability of heads: 160/300 = 0.5333
Experimental probability of tails: 140/300 = 0.4667
Comparing the experimental probabilities to the theoretical probabilities, we can observe that the experimental probability of heads is slightly higher than the theoretical probability, while the experimental probability of tails is slightly lower.
In this particular example, the experimental probabilities after 300 trials do not align perfectly with the theoretical probabilities. However, it is important to note that these differences can be attributed to sampling variability, as the experimental outcomes are subject to random fluctuations.
To draw a more definitive conclusion about the alignment between experimental and theoretical probabilities, a larger number of trials would need to be conducted. As the number of trials increases, the experimental probabilities tend to converge towards the theoretical probabilities, providing a closer alignment between the two.
For more such information on: probabilities
https://brainly.com/question/30390037
#SPJ8
The question probable may be:
Do experimental probabilities after 300 trials tend to align closely with theoretical probabilities? Consider an example scenario and calculate both the theoretical and experimental probabilities to determine if they are close.
a committee of 5 people randomly selects someone to take the notes of each meeting what is the probablility that a person takes notes less than twice in 10 meetigs
Answers
The probability that a person takes notes less than twice in 10 meetings is 37.58%.
We have to solve the probability when X<2 to determine the likelihood that someone takes notes fewer than twice in 10 meetings. The likelihood of the given distribution in P(X<2)=P(0)+P(1)
Here, random variable X is selecting for taking notes or not.
What is binomial distribution?A common probability distribution known as the binomial distribution simulates the likelihood of getting one of two outcomes given a set of parameters.
There are only two possibilities, so we use binomial distribution. The pmf is as follows.
\(P(X)= ^{n}C_{x} p^{x} q^{n-x}\)
n=10, p=1/5, q=1-1/5=4/5.
When X=0,
\(P(0)= ^{10}C_{0} \frac{1}{5}^{0} \frac{4}{5}^{10-0}\)
\(P(0)= \ \frac{4}{5}^{10}\)
P(0)=0.1074
P(0)=10.74%
When X=1,
\(P(1)= ^{10}C_{1} \frac{1}{5}^{1} \frac{4}{5}^{9}\)
\(P(1)= 10\times \frac{1}{5}^{1} \times \frac{4}{5}^{9}\)
P(1)=0.2684
P(1)=26.84%
P(X<2)= P(0)+P(1)
=0.1074+0.2684
=0.3758
=37.58%
Therefore, the probability that a person takes notes less than twice in 10 meetings is 37.58%.
To learn more about probability refer here :
https://brainly.com/question/29381779#
#SPJ4
Find AB
А.
B
N
M
-3. finele ooie 7
35.
D
22 uz to
с
29

Answers
Answer:
AB would equal 15
Step-by-step explanation:
since 22 is 7 away from 29, you just have to subtract 7 from 22. since MD and AM are equal, so is the distance in-between
Let S be the part of the plane 2x + 1y + z = 2 which lies in the first octant, oriented upward. Find the flux of the vector field F = 1i + 1j + 3k across the surface S.
Answers
The vector field F = 1i + 1j + 3k has a flux of 2 across the surface S. We use Green's Theorem to determine the vector-flux field.
Explain the Green's Theorem?The simple formula for Green's theorem is the link between the total amount of microscopic circulation inside of curve C and the macroscopic circulation revolving around it.Green's Theorem in flux form for the specified vector-field:φ = ∫ F.n ds
φ = ∫∫ F. divG.dA
In which, G is equivalent to a part of given plane = 2x + 1y + z = 2.
And, F = 1i + 1j + 2k
Thus,
div G = div(2x + 1y + z = 2) = 2i + 4j + k
Flux = ∫(1i + 1j + 3k) (2x + 1y + z).dA
φ = ∫ (2 + 4 + 2).dA
φ = 8∫dA
A = 1/2 XY (on this given x-y plane)
2x+4y =2
For, x = 0, y = 1/2
y = 0, x = 1
1/2 (1*1/2) = 1/4
Thus, flux = 8*1/4 = 2
φ = 2.
There are several uses for Green's theorem. Solving two-dimensional flow integrals, whose assert that its sum of fluid outflows from just a volume equals the sum of outflows around an enclosed area, is one way to accomplish this.
To know more about the Green's Theorem, here
https://brainly.com/question/23265902
#SPJ4
The complete question is-
Let S be the part of the plane 2x + 1y + z = 2 which lies in the first octant, oriented upward. Find the flux of the vector field F = 1i + 1j + 3k across the surface S. F = 1i + 1j + 3k across the surface s2x + 1y + z = 2 which lies in the first octant, oriented upward. Find the flux of the vector field.
Quadrilateral YFGT can be mapped onto quadrilateral MKNR by a translation. If TY = 2, find RM.
Answers
Based on the information given, we can conclude that RM = 2, but we cannot determine the lengths of the other sides of the quadrilaterals without further information.
Given that quadrilateral YFGT can be mapped onto quadrilateral MKNR by a translation, we can use the information to determine the length of RM.
A translation is a transformation that moves every point of a figure by the same distance and in the same direction. In this case, the translation is such that the corresponding sides of the quadrilaterals are parallel.
Since TY = 2, and the translation moves every point by the same distance, we can conclude that the distance between the corresponding points R and M is also 2 units.
Therefore, RM = 2.
By the properties of a translation, corresponding sides of the two quadrilaterals are congruent. Hence, side YG of quadrilateral YFGT is congruent to side MK of quadrilateral MKNR, and side GT is congruent to NR. However, the given information does not provide any additional details or measurements to determine the lengths of these sides.
For more such questions on quadrilaterals
https://brainly.com/question/27991573
#SPJ8
Pls help
Find the surface area of the cylinder.

Answers
In the picture there is a cylinder with height 5 inches and diameter 4 inches. The surface area of cylinder is 87.62.
Given that,
In the picture there is a cylinder with height 5 inches and diameter 4 inches.
The surface area of a cylinder is the area that is enclosed by the flat bases and the curving surface of the cylinder. The cylinder's total surface area includes the area of the curving surface as well as the areas of the two circle-shaped bases of the cylinder.
The surface area of cylinder is 2πr²+2πrh
r is radius which is diameter/2
r=4/2=2
h is 5
Surface area of cylinder= 2πr²+2πrh
=2π×2²+2π×2×5
=8π+20π
=28π
=28×3.14
=87.92
Therefore, the surface area of cylinder is 87.62.
To learn more about cylinder visit: https://brainly.com/question/16134180
#SPJ1
A gambler plays roulette 100 times betting $1 on two numbers, 7 and 11, each time. If the ball lands on either 7 or 11 then gambler wins $17, if the ball lands on any of the other 36 numbers the gambler loses $1. The roulette wheel has 38 slots numbered 1-36, 0, and 00 a) Which is the appropriate box model? 1. The box has 38 tickets: 1 marked "7" and 1 marked "11" and the rest marked 2. The box has 38 tickets: one each of 1,2,3,...36,0, and 00 3. The box has 38 tickets: 2 marked "17" and 36 marked 4. The box has 38 tickets:2 marked "17" and 36 marked "-1"
Answers
Question 16 of 25
Use the zeros and the labeled point to write the quadratic function shown in
the graph
30
(1,8)
O A. y=2x2+2x– 12
O B. y=-2 - X+6
O C. y=-272 - 2x + 12
O D. y=x+x-6
SUBMIT

Answers
Answer:
x+x-6
Step-by-step explanation:
The correct statements describing the given functions are -
The domain of h⁻¹(x) is the range of h(x).The x-coordinate of the x-intercept of h⁻¹(x) is the y- coordinate of the y-intercept of h(x).The maximum of h(x) is the largest x-value of h⁻¹(x).What is function?A function is a relation between a dependent and independent variable. We can write the examples of functions as -
y = f(x) = ax + b
y = f(x, y, z) = ax + by + cz
Given are the functions. It is asked to identify the statements that describe these functions.
The given functions are h(x) and its inverse h⁻¹(x). For the given functions, as we can see in the table, the following statements are true -
The domain of h⁻¹(x) is the range of h(x).The x-coordinate of the x-intercept of h⁻¹(x) is the y- coordinate of the y-intercept of h(x).The maximum of h(x) is the largest x-value of h⁻¹(x).Therefore, the correct statements describing the given functions are -
The domain of h⁻¹(x) is the range of h(x).The x-coordinate of the x-intercept of h⁻¹(x) is the y- coordinate of the y-intercept of h(x).The maximum of h(x) is the largest x-value of h⁻¹(x).To solve more questions on functions, visit the link below-
https://brainly.com/question/30194223
#SPJ7
Help i don't understand this


Answers
Answer:
yes it is possible to get a common denominator grater than 12 and still get the right answer.
Step-by-step explanation:
This is because the numerator is always directly corelated to de denominator therefore the fraction wouldn't change because when simplified it is the same fraction as it would be with 12 as the denominator
Need help please find all three answers

Answers
Answer:
What are we supposed to do
Step-by-step explanation:
10. Use synthetic division to completely factor y = x3 - 4x2 - 9x + 36 by x + 3.
O.
y = (x + 3)(x - 4)(x - 3)
y = (x + 3)(x - 4)(x + 3)
y = (x + 3)(x + 4)(x - 3)
y = (x + 3)(x + 4)(x + 3)
Answers
Answer: A
Step-by-step explanation:
\(P(x)= x^3 - 4x^2 - 9x + 36 \\D(x)=x + 3\\\\\begin{array}{|c|c|c|c|c|}&x^3&x^2&x&1\\---&---&---&---&---\\&1&-4&-9&36\\x=-3&&-3&21&-36\\---&---&---&---&---\\&1&-7&12&0\\\end{array}\\\\\\P(x)= x^3 - 4x^2 - 9x + 36 \\=(x+3)(x^2-7x+12)\\=(x+3)(x^2-3x-4x+12)\\=(x+3)(x(x-3)-4(x-3))\\=(x+3)(x-3)(x-4)\\\\Answer \ A\)
Start with,
\((x^3-4x^2-9x+36)\div(x+3)\)
Look at first terms, \(x^3\) and \(x\) in divisor, with what do you have to multiply \(x\) so that you end up with \(x^3\)? The answer is \(x^2\).
So you write,
\((x^3-4x^2-9x+36)\div(x+3)=x^2\)
\(-(x^3+3x^2)\) (because you are subtracting \(x^2(x+3)\).
Which becomes when u subtract columns,
\((-7x^2-9x+36)\div(x+3)=x^2\)
Now repeat the procedure,
\((-7x^2-9x+36)\div(x+3)=x^2-7x\)
\(-(-7x^2-21x)\)
which becomes
\((12x+36)\div(x+3)=x^2-7x\)
And again,
\((12x+36)\div(x+3)=x^2-7x+12\)
\(-(12x+36)\)
becomes,
\(0=x^2-7x+12\)
Since the RHS is 0 that means \((x+3)\) is a factor.
Now you can factor \(x^2-7x+12=(x-4)(x-3)\) because \(-4\cdot-3=12\) and \(-4+(-3)=-7\).
Combine the factors and get \((x+3)(x-4)(x-3)\).
Hope this helps :)