The coordinates of the vertices of ABC are A(3, 3), B(7,7), and C(7, -1) What type of triangle is triangle ABC? Use coordinate geometry to answer the question.

The next crow observed will be black is not supported by the premises in any meaningful way. The premises do not guarantee the outcome of the next observation.  Argument B is the stronger argument.

Argument A is an example of an inductive argument, as it generalizes from a limited sample to make a broader claim about the entire population. This argument form is called induction and can be evaluated on its strength.

Argument B is a deductive argument, as it uses a conditional premise to reach a definite conclusion. In this form of argument, the conclusion necessarily follows from the premises. The strength of a deductive argument depends on the logical structure of the premises and conclusion.

The better argument of the two is argument B. Because in deductive arguments, the conclusion necessarily follows from the premises if the premises are true.

And this argument, the premises state that if 90% of observed crows are black, then the next crow observed will be black.

Additionally, it is also claimed that 90% of observed crows are black. Therefore, it can be logically deduced that the next crow observed will be black

The argument in A, however, is an inductive argument, where the conclusion is not necessarily true even if the premises are true. Even if 90% of observed crows are black, it does not necessarily follow that 90% of all crows are black.

argument B is the stronger argument.

Learn more deductive arguments:

https://brainly.com/question/28571732

#SPJ11

Answer:

option C 5×10-6 is right answer

Answer:

\(c \: ) \: 5 \times 10 - 6\)

c is the answer

Answer:

I'm from California I'm new to this

Answer:

explanshun

Step-by-step explanation:

Step 1:

you use the rules of exponents to isolate a logarithmic expression (with the same base) on both sides in your equation.

Step 2:

Set the arguments equal out each other.

Step 3:

Solve you resulting equation.

Step 4:

Check your answer. 

Step 5:

Solve.

A + B are correct

via a p e x

The correct statements are A and B

'A triangle in which one of the interior angles is 90° is called a right-angled triangle. The longest side of the right triangle, which is also the side opposite the right angle, is the hypotenuse and the two arms of the right angle are the height and the base.'

According to the given problem,

cos(A−B) = cosAcosB + sinAsinB .

Let A=90∘, B = a

Therefore.

cos(90∘−a) = cos90∘cosa + sin90∘sina.

Here, we have,

cos90∘ = 0, sin90∘ = 1.

cos(90∘−a) = sina

Now, we know,

sin∅ = \(\frac{Perpendicular}{Hypotenuse}\)

cos∅ = \(\frac{Base}{Hypotenuse}\)

For ∠BAC,

cosA = \(\frac{AB}{AC}\)

For ∠ACB,

sinC =  \(\frac{AB}{AC}\)

Hence, we can conclude, A and B are the true statements for the given right-angled triangle.

Learn more about right-angled triangle here:

https://brainly.com/question/13263113

#SPJ2

Answer: See the explanation Below

Step-by-step explanation:

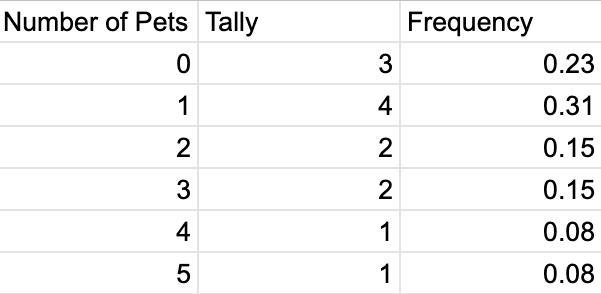
The problem with the frequency table in the problem occurs within the "number of pets" column. Instead of recording the numbers 0-5 one time, Katie gave a row for every occurrence of each number. However, this defeats the purpose of having a tally column, which is much more efficient.

A better data table would look like the one I have attached to the problem.

For some additional help:

The tally column is filling in the number of times we see each number in the responses. For instance, 0 is given as an answer 3 times, so in the 0 tally box, you would write 3 tally marks.

The frequency table is found by dividing the number of that specific response by the total number of responses. For 0, we have 3 responses and 13 responses total. 3/13 = 0.23, which becomes the frequency. In the end, the totals for the frequency column should equal 1.

I hope this helps!  

Answer:

y = 5x + 7

Step-by-step explanation:

I am guessing, but you are leaving something out.

y = the total cost.

x = the number of shirts.

Answer:

The total cost for the 7 shirts including tax is 7n + 6

Step-by-step explanation:

We want to find the Cost of 7 t-shirts and $6 tax.

Now, the let the cost of the 7 shirts be n. Thus, we can now say that cost of 7 shirts is 7n.

Now, since there is an additional cost of $6 for tax, we can now say that the total cost for the 7 shirts including tax is expressed as;

7n + 6

Thus, we conclude that the total cost for the 7 shirts including tax is 7n + 6

Answer:

2 rays: Two examples of rays are AB and AC, both emanating from a common endpoint A and extending infinitely in opposite directions.

2 line segments: Two examples of line segments are AB and CD, both of which have two endpoints and a finite length.

2 lines (not including the parallel lines): Two examples of lines are AB and CD, which intersect at a point E.

2 sets of parallel lines: Two examples of sets of parallel lines are AB and CD, and EF and GH, where AB and CD are parallel to each other, and EF and GH are parallel to each other.

2 acute angles (not incl. the ones in the As): Two examples of acute angles are ∠BAC and ∠EFG, both of which measure less than 90 degrees.

2 obtuse angles (not incl. the ones in the As): Two examples of obtuse angles are ∠PQR and ∠XYZ, both of which measure greater than 90 degrees.

2 right angles (not incl. the ones in the As): Two examples of right angles are ∠ABC and ∠EFG, both of which measure 90 degrees.

2 clear examples of supplementary angles: Two examples of supplementary angles are ∠ABC and ∠DEF, and ∠PQR and ∠RST, where the sum of the angles in each pair is 180 degrees.

2 clear examples of complementary angles: Two examples of complementary angles are ∠ABC and ∠PQR, and ∠DEF and ∠RST, where the sum of the angles in each pair is 90 degrees.

2 clear examples of more than two angles on a line that add up to 180°: Two examples of sets of angles on a line that add up to 180 degrees are ∠ABC, ∠BCD, and ∠CDE, and ∠PQR, ∠QRS, and ∠RST.

2 right triangles: Two examples of right triangles are ΔABC and ΔPQR, where ∠CAB and ∠QRP are right angles.

2 acute triangles: Two examples of acute triangles are ΔDEF and ΔGHI, where all angles are acute.

The sum of the measures of angles within each triangle:

In ΔABC, the sum of the measures of the angles is 180 degrees, where ∠A measures 90 degrees, and ∠B and ∠C measure 45 degrees each.

In ΔPQR, the sum of the measures of the angles is 180 degrees, where ∠P and ∠R measure 90 degrees each, and ∠Q measures 0 degrees.

In ΔDEF, the sum of the measures of the angles is 180 degrees, where all angles are acute, and ∠D, ∠E, and ∠F measure 60 degrees each.

In ΔGHI, the sum of the measures of the angles is 180 degrees, where all angles are acute, and ∠G, ∠H, and ∠I measure 40 degrees each.

In ΔJKL, the sum of the measures of the angles is 180 degrees, where ∠K measures 90 degrees, and ∠J and ∠L measure 45 degrees each.

In ΔMNO, the sum of the measures of the angles is 180 degrees, where ∠O measures 90 degrees, and ∠M and ∠N measure 45 degrees each.

The probability that randomly selected mechanical component weighs: more than 11.5 lbf is 0.0099, less than 8.7 lbf is 0.3208,  less than 5.0 lbf is 0.0228, between 6.2 lbf and 7.0 lbf is 0.1314, between 10.3 lbf and 14.0 lbf is 0.0436, between 6.8 lbf and 8.9 lbf is 0.3464.

We can use the standard normal distribution and z-scores to answer these questions:

P(X > 11.5) = P(Z > (11.5 - 8) / 1.5) = P(Z > 2.33) = 0.0099

Therefore, the probability that a randomly selected mechanical component weighs more than 11.5 lbf is 0.0099, or about 1%.

P(X < 8.7) = P(Z < (8.7 - 8) / 1.5) = P(Z < 0.47) = 0.3208

Therefore, the probability that a randomly selected mechanical component weighs less than 8.7 lbf is 0.3208, or about 32%.

P(X < 5.0) = P(Z < (5 - 8) / 1.5) = P(Z < -2) = 0.0228

Therefore, the probability that a randomly selected mechanical component weighs less than 5.0 lbf is 0.0228, or about 2%.

P(6.2 < X < 7.0) = P((6.2 - 8) / 1.5 < Z < (7 - 8) / 1.5) = P(-1.2 < Z < -0.67) = 0.1314

Therefore, the probability that a randomly selected mechanical component weighs between 6.2 lbf and 7.0 lbf is 0.1314, or about 13%.

P(10.3 < X < 14.0) = P((10.3 - 8) / 1.5 < Z < (14 - 8) / 1.5) = P(1.53 < Z < 2.67) = 0.0436

Therefore, the probability that a randomly selected mechanical component weighs between 10.3 lbf and 14.0 lbf is 0.0436, or about 4%.

P(6.8 < X < 8.9) = P((6.8 - 8) / 1.5 < Z < (8.9 - 8) / 1.5) = P(-0.47 < Z < 0.60) = 0.3464

Therefore, the probability that a randomly selected mechanical component weighs between 6.8 lbf and 8.9 lbf is 0.3464, or about 35%.

To know more about probability :

https://brainly.com/question/15016913

#SPJ4

____The given question is incomplete, the complete question is given below:

Suppose that the weight of a mechanical component is normally distributed with mean p = 8.0 Ibf and standard deviation = 1.5 lbf. Answer the following questions: 1. If you randomly select a mechanical component, what is the probability that it weighs more than 11.5 lbf? 2. If you randomly select a mechanical component, what is the probability that it weighs less than 8.7 lbf? 3. If you randomly select a mechanical component, what is the probability that it weighs less than 5.0 lbf? 5. If you randomly select a mechanical component, what is the probability that it weighs between 6.2 lbf and 7.0 lbf? 6. If you randomly select a mechanical component, what is the probability that it weighs between 10.3 lbf and 14.0 lbf? 7. If you randomly select a mechanical component, what is the probability that it weighs between 6.8 lbf and 8.9 lbf?

The probability that their mean is above 215 is 0.0359.

Therefore the answer is (a) 0.0359.

To find the probability that the mean rating of 36 applicants is above 215, we can use the central limit theorem, which states that the distribution of sample means approaches a normal distribution with a mean equal to the population mean and a standard deviation equal to the population standard deviation divided by the square root of the sample size. So, the mean of the distribution of sample means is 200, and the standard deviation is 50/sqrt(36)=8.33.

We can then standardize the sample mean using the z-score formula:

z = (x* - μ) / (σ / sqrt(n))

where x* is the sample mean, μ is the population mean, σ is the population standard deviation, and n is the sample size. Plugging in the values, we get:

z = (215 - 200) / (8.33) = 1.8.

Using a standard normal distribution table or calculator, we can find that the probability of getting a z-score greater than 1.8 is 0.0359. Therefore, the answer is (a) 0.0359.

To know more on central limit theorem

https://brainly.com/question/18403552

#SPJ4

Answer:

the height is of the rectangle is 13 meters

Answer:

50 in. 48 in. 14 in.

Step-by-step explanation:

\(m\angle E=\sin \dfrac{\sqrt{10}}{2\sqrt5}=\sin \dfrac{\sqrt2}{2}=45^{\circ}\)

The solution to the given differential equation is \(y = a_0x^{[0]} + a_1x^{[1]} + a_2x^{[2]}\), where a_0, a_1, and a_2 are constants.

To fathom the given differential equation, xy'' + y' = 0, we will begin by expecting a control arrangement of the frame y = ∑(n=0 to ∞) a_nx^n, where a_n speaks to the coefficients to be decided.

Separating y with regard to x, we get:

\(y' = ∑(n=0 to ∞) a_n(nx^[(n-1))] = ∑(n=0 to ∞) na_nx^[(n-1)]\)

Separating y' with regard to x, we get:

\(y'' = ∑(n=0 to ∞) n(n-1)a_nx^[(n-2)]\)

Presently, we substitute these expressions for y and its subsidiaries into the differential condition:

\(x(∑(n=0 to ∞) n(n-1)a_nx^[(n-2))] + (∑(n=0 to ∞) na_nx^[(n-1))] =\)

After improving terms, we have:

\(∑(n=0 to ∞) n(n-1)a_nx^[(n-1)] + ∑(n=0 to ∞) na_nx^[n] =\)

Another, we compare the coefficients of like powers of x to zero, coming about in a boundless arrangement of conditions:

For n = 0: + a_0 = (condition 1)

For n = 1: + a_1 = (condition 2)

For n ≥ 2: n(n-1)a_n + na_n = (condition 3)

Disentangling condition 3, we have:

\(n^[2a]_n - n(a_n) =\)

n(n-1)a_n - na_n =

n(n-1 - 1)a_n =

(n(n-2)a_n) =

From equation 1, a_0 = 0, and from equation 2, a_1 = 0.

For n ≥ 2, we have two conceivable outcomes:

n(n-2) = 0, which gives n = or n = 2.

a_n = (minor arrangement)

So, the solution to the given differential equation is \(y = a_0x^{[0]} + a_1x^{[1]} + a_2x^{[2]}\), where a_0, a_1, and a_2 are constants.

Learn more about differential equations here:

https://brainly.com/question/28099315

#SPJ1

To organize the provided data, we can create a table comparing the samples of couples with children (sample 1) and childless couples (sample 2) as follows:

Sample Sample Size Sample Mean Sample Standard Deviation

1 78 51.1 4.7

2 94 45.2 12.1

In this table, we have listed the sample number (1 and 2), the sample size (number of couples in each group), the sample mean (average Marital Satisfaction Inventory score), and the sample standard deviation (measure of variability in the scores) for each group. This organization allows us to compare the data and proceed with hypothesis testing on the difference in population means between the two groups.

Learn more about  data from

https://brainly.com/question/30459199

#SPJ11

Answer:

40 and 67

Step-by-step explanation:

To determine the validity of the argument that "Mr. Einstein is a professor," we can use a Venn diagram. Here's how to

do it:Step 1: Draw two overlapping circles, one for "Professors" and one for "People who wear glasses."Step 2: Label the circle for professors "P" and the circle for people who wear glasses "G."Step 3: Write "Some professors wear glasses" in the area where the circles overlap.Step 4: Write "Mr. Einstein wears glasses" in the area that represents

people who wear glasses but are not professors.Step 5: We cannot conclude that Mr. Einstein is a professor based solely on these premises since there are people who wear glasses but are not professors. Therefore, the argument is invalid.Here is a visual representation of the

To know more about polygon visit:

https://brainly.com/question/24464711

#SPJ11

The unit rate per batch of cookies is 2.75 as Lennon uses 5 1/2 cups of flour for every 2 batches of cookies she bakes.

To calculate the unit rate, divide the denominator by the numerator so that the denominator equals 1. For example, if 50 kilometers are reached in 5.5 hours, the unit rate is 50 kilometers/5.5 hours = 9.09 kilometers per hour.

When expressed as a fraction, a unit rate has a denominator of one unit. To write a rate as a unit rate, divide the rate's numerator and denominator by the rate's denominator.

Based on the given conditions, formulate:

(5 1/2)/2

We have to find the common denominator and write the numerators above the common denominator.

(((5×2)/2)+(1/2))/2

Now, we have to calculate the product or quotient:

((10/2)+(1/2))/2

Now, write the numerators over common  denominator,

((10+1)/2)/2

Then, calculate the sum or difference:

(11/2)/2

=11/4

=2.75 (or) 2 3/4

Therefore, the unit rate per batch of cookies is 2.75.

To know more about unit rate, visit:

https://brainly.com/question/13795269

#SPJ1

The equation of a parabola is a quadratic equation.

The equation of the parabola is: \(\mathbf{y = 4x^2 + 32x -32}\)

The points are given as:

\(\mathbf{(x,y) = (-5,-224),(-3,-92),(1,4)}\)

Substitute these values in:

\(\mathbf{y = ax^2 + bx +c}\)

So, we have:

\(\mathbf{-224 = a(-5)^2 + b(-5) +c}\)

\(\mathbf{-224 = 25a - 5b +c}\) ------ (1)

\(\mathbf{-92 = a(-3)^2 + b(-3) +c}\)

\(\mathbf{-92 = 9a -3b +c}\) ---- (2)

\(\mathbf{4 = a(1)^2 +b(1) +c}\)

\(\mathbf{4 = a +b +c}\) ---- (3)

Subtract (3) from (2)

\(\mathbf{9a - a - 3b - b + c - c =-92 - 4}\)

\(\mathbf{8a - 4b =-96}\)

Multiply by 3

\(\mathbf{24a - 12b = -288}\)

Subtract (3) from (1)

\(\mathbf{25a -a - 5b -b +c - c = -224 - 4}\)

\(\mathbf{24a - 6b = -228}\)

Subtract \(\mathbf{24a - 6b = -228}\) from \(\mathbf{24a - 12a = -288}\)

\(\mathbf{24a- 24a - 12ba +6b = -288 + 96}\)

\(\mathbf{- 6b = -192}\)

Divide through by -6

\(\mathbf{b = 32}\)

Substitute \(\mathbf{b = 32}\) in \(\mathbf{8a - 4b =-96}\)

\(\mathbf{8a - 4 \times 32 = -96}\)

\(\mathbf{8a - 128 = -96}\)

Collect like terms

\(\mathbf{8a = 128 -96}\)

\(\mathbf{8a = 32}\)

Divide both sides by 8

\(\mathbf{a = 4}\)

Substitute \(\mathbf{a = 4}\) and \(\mathbf{b = 32}\) in \(\mathbf{4 = a +b +c}\)

\(\mathbf{4 + 32 + c = 4}\)

\(\mathbf{36 + c = 4}\)

Subtract 36 from both sides

\(\mathbf{c = -32}\)

Substitute values of a, b and c in: \(\mathbf{y = ax^2 + bx +c}\)

\(\mathbf{y = 4x^2 + 32x -32}\)

Hence, the equation of the parabola is: \(\mathbf{y = 4x^2 + 32x -32}\)

Read more about quadratics and parabolas at:

https://brainly.com/question/10738281

The errors made by Parul are She added 3.5 instead of subtracting, she should have divided by -5 as her first step, and she used a closed circle instead of an open circle on the number line. (Options: 1, 2, 5)

From the given information, Parul attempted to solve the inequality -5x - 3.5 > 6.5. Let's analyze the errors she made.

She added 3.5 to both sides when she should have subtracted.

Parul added 3.5 to both sides of the inequality, which is incorrect. To isolate the variable term (-5x) on one side, she should have subtracted 3.5 from both sides. This error affects the accuracy of the inequality.

She should have divided both sides by -5 as her first step.

Parul did not divide both sides of the inequality by -5 initially to isolate the variable x. Dividing by -5 is necessary to solve for x. Instead, she incorrectly subtracted 3.5 from both sides, as mentioned earlier.

She used a closed circle instead of an open circle on the number line.

Parul used a closed circle to represent the point -50 on the number line. However, for an inequality where x > -50, the correct representation should be an open circle at -50. This is because the point -50 itself is not included in the solution set.

Therefore, the errors made by Parul are:

She added 3.5 to both sides when she should have subtracted.

She should have divided both sides by -5 as her first step.

She used a closed circle instead of an open circle on the number line. So Option 1, 2, 5 are correct.

For more question on subtracting visit:

https://brainly.com/question/25421984

#SPJ8

The solution is, the y-coordinate of the solution is y= -5/3.

Simplify simply means to make it simple. In mathematics, simply or simplification is reducing the expression/fraction/problem in a simpler form. It makes the problem easy with calculations and solving.

here, we have,

given that, the system of equation is,

6x - 3y = -7

2x+ 3y = -9

now, we have to find the y-coordinate of the solution.

so, we get,

6x - 3y = -7 .........(1)

2x+ 3y = -9 ..........(2)

now, for solving, we add both equations, & get,

8x = -16

or, x = -2

solving we get,

y = -5/3

Hence, The solution is, the y-coordinate of the solution is y= -5/3.

To learn more on simplification click:

brainly.com/question/28996879

#SPJ1

Answer:

C

Step-by-step explanation:

324 = 4 \((3)^{2x}\) ( divide both sides by 4 )

81 = \(3^{2x}\) , that is

\(3^{4}\) = \(3^{2x}\)

Since bases on both sides are equal, both 3, then equate exponents

4 = 2x ( divide both sides by 2 )

2 = x → C

Answer:

The equation is following the mathematical rule of multiplying exponents.

Step-by-step explanation:

As an example to back up the answer, when you have half of a dollar, that is $0.50, if you took a half (1/2) of $0.50 that would be one fourth (1/4) of a dollar, but half of 50 cents ($0.50) A similar thing is happening with this problem. When you have two numbers (2 and 4) when you multiply them together, they equal to eight (8) for this problem, when you multiply two exponents together, you are raising the coefficient (a real number like 6) to the power of 2, and then taking that number and multiplying it by the power of 4. This is similar to the half of 50 cents, is equal to 1/4 of dollar  ($0.25)

Hope this helps explain multiplying exponents together, and the mathematical rule behind it.

In a contingency table, we describe the relationship between two variables measured at the ordinal or nominal level (option a).

A contingency table is a statistical table that displays the frequency distribution of two categorical variables and helps identify any associations or dependencies between them. The table organizes the data into rows and columns, with each cell representing the frequency count or proportion of observations falling into a particular combination of categories.

By examining the distribution of frequencies across the table, patterns and relationships between the variables can be discerned. This information can be useful in various fields, such as social sciences, market research, and epidemiology, for analyzing survey responses, understanding consumer preferences, or investigating the relationship between risk factors and diseases, among other applications.

To know more about contingency table, refer here:

https://brainly.com/question/30920745#

#SPJ11

change 3/4 to 6/8 and add 6/8 and 1/8 to get 7/8

he walked 7/8 miles in total :)

The value of the indefinite integral is,

\([\dfrac{1}{5} (x^2 + 44)^{5/2} - 22 (\dfrac{2}{3} )(x^2 + 44)^{3/2} ] + C\)

Where C is the constant of integration.

Now for the indefinite integral \(\int\limits x^3\sqrt {x^2 + 44}dx\), simplify the expression and then apply integration techniques.

Let us assume that;

\(u = x^2 + 44\).

\(du = 2x dx\)

Now, let's rewrite the integral using u:

\(\int\limits x^3\sqrt {x^2 + 44}dx = \int\limits (\dfrac{1}{2} )2x^2 \sqrt {(x^2 + 44} dx\)

\(= \dfrac{1}{2} \int\limits (u - 44) \sqrt {u} du\)

Expanding and simplifying the expression, we have:

\(= \dfrac{1}{2} \int\limits (u^{3/2} - 44 u^{1/2} )du\)

Now integrate each term separately:

\(\dfrac{1}{2} [\dfrac{2}{5} u^{5/2} - 44 (\dfrac{2}{3} )u^{3/2} ] + C\)

\([\dfrac{1}{5} u^{5/2} - 22 (\dfrac{2}{3} )u^{3/2} ] + C\)

Finally, we substitute back \(u = x^2 + 44\);

\([\dfrac{1}{5} (x^2 + 44)^{5/2} - 22 (\dfrac{2}{3} )(x^2 + 44)^{3/2} ] + C\)

So, the indefinite integral is,

\([\dfrac{1}{5} (x^2 + 44)^{5/2} - 22 (\dfrac{2}{3} )(x^2 + 44)^{3/2} ] + C\)

Where C is the constant of integration.

To learn more about integration visit :

brainly.com/question/18125359

#SPJ12

The complete question is,

Evaluate the indefinite integral. (Use for the constant of integration.) The given indefinite integral is \(\int\limits x^3\sqrt {x^2 + 44}dx\)

Answer:

Circle 1

28.269 ÷ 9 = 3.141

Circle 2

25.126 ÷ 8 = 3.14075

Circle

37.686 ÷ 12 = 3.1405

If this helped, please consider picking this answer as the Brainliest Answer. Thank you!

Movie Domestic Gross ($ millions) The Avengers (2012) 677 Spiderman (2002) 602 Spiderman 2 (2004) 520 Avengers: Age of Ultron (2015) 471 Iron Man 3 (2013) 434 Spiderman 3 (2007) 423 Captain America: Civil War (2016) 408 Guardians of the Galaxy Vol. 2 (2017) 389 Iron Man (2008) 384 Deadpool (2016) 363.

To learn more about variability, refer to:

https://brainly.com/question/12872866

#SPJ1

Answer:

see attachment

Step-by-step explanation:

Answer:

120

Step-by-step explanation:

280 divided by 7= 40. 40*3=120

therefore 120 is the answer