Suppose xy = 3 and dy/dt = - 4. Find dx/dt when x = 2
Answers
The value of dx/dt = 16/3 when x = 2.
How to find the value of dx/dt by using implicit differentiation?To solve this problem, we will need to use implicit differentiation. We start by differentiating both sides of the equation xy = 3 with respect to time t:
d/dt (xy) = d/dt (3)
Using the product rule for differentiation, we can rewrite the left-hand side as:
x (dy/dt) + y (dx/dt) = 0
Substituting the given value of dy/dt = -4 and the given value of xy = 3, we get:
x (-4) + y (dx/dt) = 0
Simplifying this expression, we get:
-4x + y (dx/dt) = 0
Now we can solve for dx/dt by plugging in the given value of x = 2 and solving for y:
xy = 3
2y = 3
y = 3/2
Substituting y = 3/2 and x = 2, we get:
-4(2) + (3/2) (dx/dt) = 0
Simplifying this expression, we get:
-8 + (3/2) (dx/dt) = 0
Multiplying both sides by 2/3, we get:
-16/3 + dx/dt = 0
Therefore, we have:
dx/dt = 16/3
Learn more about implicit differentiation.
brainly.com/question/30227044
#SPJ11
Related Questions
Henry's baseball practice starts at 2:00 pm. It lasts 1 hour and 45 minutes. What time does he get out of baseball practice?
Answers
Answer:
3:45 pm
Step-by-step explanation:
..............
What is the surface area of the cone? Use 3.14 for PIE. Use pencil and paper. Suppose the diameter and the slant height of a cone are cut in half. How does this affect the surface area of the cone?

Answers
Answer:
Step-by-step explanation:
1256cm^2
Consider a perfectly competitive firm that produces output from labor and capital under the following cond
2
ions: Y=100K
1/2
+40L
1/2
- P=$2 - W=$8 - R=$10 a. Suppose that the firm has decided to employ 25 units of labor and is currently employing 50 units of capital. What will its profit be at those employment levels? b. What equation describes the profit-moximizing quantity of capital for this firm? c. To raise profits, should the firm increase its capital employment (from 50 to something higher), or decrease it? Explain.
Answers
(a) The profit of the firm at the current employment levels of 25 units of labor and 50 units of capital can be calculated by subtracting the total cost from total revenue.
The total revenue is given by the output multiplied by the market price, which is $2. The total cost is the sum of the wage cost (W) and the rental cost of capital (R), multiplied by their respective quantities.
Profit = Total Revenue - Total Cost
Profit = (Output * Price) - (Wage * Labor + Rental * Capital)
Given that the output is determined by the production function Y = 100K^(1/2) + 40L^(1/2), the profit can be calculated as follows:
Profit = (100K^(1/2) + 40L^(1/2)) * $2 - ($8 * 25 + $10 * 50)
(b) The profit-maximizing quantity of capital for this firm can be determined by setting the marginal revenue product of capital (MRPK) equal to the rental cost of capital (R). MRPK represents the additional revenue generated by employing an additional unit of capital.
MRPK = R
The marginal revenue product of capital can be calculated as the partial derivative of the production function with respect to capital (K), multiplied by the market price (P):
MRPK = (∂Y/∂K) * P
Using the production function Y = 100K^(1/2) + 40L^(1/2), we can calculate the marginal revenue product of capital as follows:
MRPK = (∂Y/∂K) * P = (50K^(-1/2)) * $2
Setting this equal to the rental cost of capital (R) of $10, we have:
(50K^(-1/2)) * $2 = $10
Simplifying the equation, we find:
K^(-1/2) = 1/5
Squaring both sides of the equation, we get:
K = 25
Therefore, the profit-maximizing quantity of capital for this firm is 25 units.
(c) To raise profits, the firm should decrease its capital employment from 50 to 25 units. This is because the profit-maximizing quantity of capital is determined to be 25 units, as calculated in part (b). By employing fewer units of capital, the firm can reduce its rental cost while still maintaining the optimal level of capital for production. As a result, the firm can lower its total cost and increase its profit. Employing more capital beyond the profit-maximizing level would lead to diminishing returns, where the additional costs outweigh the additional revenue generated. Therefore, reducing capital employment to the optimal level of 25 units would be the most favorable decision for the firm to maximize its profits.
Learn more about profits here:
brainly.com/question/16168992
#SPJ11
Find the shortest distance from (1,2) to the line, x+2y=2.
Answers
The shortest distance from the point (1,2) to the line x+2y=2 is 1 / sqrt(5), which is approximately 0.447.
The shortest distance from a point to a line can be found by using the formula for the perpendicular distance between a point and a line. In this case, the given point is (1,2) and the line is x+2y=2.
To find the shortest distance, we can follow these steps:
Write the equation of the given line in slope-intercept form (y = mx + b):
x + 2y = 2
2y = -x + 2
y = (-1/2)x + 1
Identify the slope of the line, which is -1/2. The perpendicular line will have a slope that is the negative reciprocal of -1/2, which is 2.
Use the formula for the perpendicular distance between a point (x1, y1) and a line y = mx + b:
Distance = |2x1 - y1 + b| / sqrt(1² + m²)
Substitute the coordinates of the point (1,2) and the slope of the perpendicular line (m = 2) into the formula:
Distance = |2(1) - 2 + 1| / √(1² + 2²)
= |2 - 2 + 1| / √(1 + 4)
= |1| / √(5)
= 1 / sqrt(5)
Therefore, the shortest distance from the point (1,2) to the line x+2y=2 is 1 / sqrt(5), which is approximately 0.447.
Learn more about slope-intercept here:
brainly.com/question/30216543
#SPJ11
Because age cannot be an independent variable, research on aging uses a(n) ______________ type of design.
Answers
Research on aging uses a **longitudinal** design.
A longitudinal design is a research design in which the same participants are studied over time. This is in contrast to a **cross-sectional** design, in which different participants are studied at different times.
Because age cannot be manipulated as an independent variable, research on aging must use a longitudinal design. This allows researchers to track changes in participants' behavior, cognition, and other factors as they age.
Longitudinal studies can be expensive and time-consuming to conduct, but they can provide valuable insights into the aging process. For example, longitudinal studies have shown that cognitive decline is not inevitable with age, and that certain lifestyle factors, such as exercise and social engagement, can help to protect against cognitive decline.
Here are some examples of longitudinal studies on aging:
* The Baltimore Longitudinal Study of Aging, which has been following a group of adults for over 70 years.
* The Framingham Heart Study, which has been following a group of adults for over 70 years.
* The Study of Adult Development and Aging, which has been following a group of adults for over 80 years.
These studies have provided valuable insights into the aging process, and they continue to be an important source of information for researchers and policymakers.
to learn more about independent variable click here:
brainly.com/question/28351996
#SPJ11
The following box plot represents the average heights of the students in Mr. Taylor's fourth grade math class.

Answers
1) In this question, we need to remember that in any boxplot the line in the middle of the box indicates the median.
Based on that, we can tell the Median is 140
2) In the Interquartile Range, we need to find the range between the lower quartile and the upper one, based on that boxplot. We can tell the IQR is:
\(IQR=Q_3-Q_1\Rightarrow141-138=3\)Note that the boundaries of the box show us the lower and the upper quartile:

What is the slope of this line?
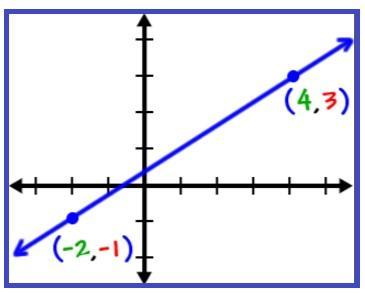
Answers
Answer:
\(m=\frac{2}{3}\)
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDASAlgebra I
Slope Formula: \(m=\frac{y_2-y_1}{x_2-x_1}\)Step-by-step explanation:
Step 1: Define
Point (-2, -1)
Point (4, 3)
Step 2: Find slope m
Substitute: \(m=\frac{3+1}{4+2}\)Add: \(m=\frac{4}{6}\)Simplify: \(m=\frac{2}{3}\)How will the solution of the system Y 2x two thirds and Y 2x one third change if the inequality sign on both inequalities is reversed to?.
Answers
The system of inequalities did not have any solution, but after reversing the sign we will get a solution of the system of inequalities.
The inequalities are : y > 2x + 2/3 and y < 2x + 1/3
There is no intersection and no solution to the system since the region above the line y = 2x + 2/3 and the region below the line y = 2x + 1/3, respectively, are the solutions to the inequality y > 2x + 2/3 and y <2x + 1/3, respectively.
Now when the signs are changed we get:
y < 2x + 2/3 and y > 2x + 1/3
The region below the line y = 2x + 2/3 is the solution of the inequality
y= 2x + 2/3, and the region above the line y = 2x + 1/3 is the solution of the inequality y > 2x + 1/3. This implies that the area between the two lines is where the system's solution lies.
Disclaimer: The complete question is :
How will the solution of the system y>2x+2/3 and y<2x+1/3 change if the inequality sign on both inequalities is reversed to y<2x+2/3 and y>2x+1/3?
To learn more about inequality visit:
https://brainly.com/question/20383699
#SPJ4
Answer:
There is no solution to the system in its original form. There are no points in common. If the signs are reversed, the system has an intersection with an infinite number of solutions.
Step-by-step explanation:
Sample answer
If Sheila drove 152 km at an average speed of 95 km/h, how much time did her trip take?
(NEED TO SHOW YOUR WORK)
Answers
1.6 hours
152 km total divided by her speed, 95 km/h =1.6
152 divided by 95 equals 1.6 hrs
Hope this helps learned in math class and that is the answer
Pls give me BRAINLIEST
Consider the expression
-4b + 8c + 12 - 8b - 2c + 6.
Part B
What is the value of the expression when b = 2 and c = -3?
Answers
Answer:
8
Step-by-step explanation:
-4(2)+8(-3)+12-8(2)-2(-3)+6=-8-24+12-16+6+6=8
Answer:
-24
Step-by-step explanation:
-4b + 8c + 12 - 8b - 2c + 6
Combine like terms
6c+18-12b
Let b=2 c=-3
6(-3) +18-12(2)
-18 +18 -24
-24
Select all that would apply as possible side lengths for a TRIANGLE with two sides that are 15 and 9.

Answers
Answer:
22
13
6
9
8
Step-by-step explanation:
Remember, the triangle-sides inequatily, which states that the sum of smaller two sides must be greater the larger side.
Hence there are two situtations, in this case "x" represents the unknown side. For an answer to be valid, at has to satisfy one of these inequalities.
x + 9 > 15; x<15
15 + 9 > x
Answers;
22
13
6
9
8
I need help. please
business weekly conducted a survey of graduates from 30 top mba programs. on the basis of the survey, assume the mean annual salary for graduates 10 years after graduation is $187,000. assume the standard deviation is $40,000. suppose you take a simple random sample of 14 graduates. round all answers to four decimal places if necessary.
Answers
The probability that the mean annual salary of a simple random sample of 14 graduates is more than $200,000 is approximately 0.1134.
Based on the given information, the mean annual salary for graduates 10 years after graduation is $187,000, with a standard deviation of $40,000.
Suppose you take a simple random sample of 14 graduates.
To find the probability that the mean annual salary of this sample is more than $200,000, we can use the Central Limit Theorem.
First, we need to calculate the standard error of the sample mean, which is equal to the standard deviation divided by the square root of the sample size.
The standard error (SE) = $40,000 / √(14)
= $10,697.0577 (rounded to four decimal places).
Next, we can calculate the z-score using the formula:
z = (sample mean - population mean) / standard error.
In this case, the population mean is $187,000 and the sample mean is $200,000.
z = ($200,000 - $187,000) / $10,697.0577
= 1.2147 (rounded to four decimal places).
Finally, we can use a standard normal distribution table or a calculator to find the probability associated with the z-score of 1.2147.
The probability is approximately 0.1134 (rounded to four decimal places).
Therefore, the probability that the mean annual salary of a simple random sample of 14 graduates is more than $200,000 is approximately 0.1134.
To know more about probability visit:
https://brainly.com/question/31828911
#SPJ11
About how many times greater was change in price per gallon in 2007 than 2000? Show your work or explain how u determind your answer.
Answers
The required, in 2007 the price per gallon was 7b more than the price of a gallon of fuel in the year 2000. Where b is the inflation factor.
What is simplification?The process in mathematics to operate and interpret the function to make the function or expression simple or more understandable is called simplifying and the process is called simplification.
Here,
Let 'a' be the cost per gallon of fuel in the year 2000, and 'b' be the inflation rate per year. If the rate of inflation is constant then
After 7 year inflation = 7b
Cost of fuel in 2007 = a + 7b
Now,
according to the question
Change in cost of fuel
= cost in 2007 - cost in 2000
= a + 7b - a
= 7b
Thus, the required, in 2007 the price per gallon was 7b more than the price of a gallon of fuel in the year 2000. Where b is the inflation factor.
Learn more about simplification here:
https://brainly.com/question/12501526
#SPJ1
Complete this item.
For the following figure, can you conclude that / | | m? Select true or false.

Answers
Answer:
true
Step-by-step explanation:
:)
The length of each of two sides of a triangle is The third side's length is 15sqrt(2) . Classify the triangle

Answers
Answer:
45-45-90 isosceles triangle
Step-by-step explanation:
based on size, which of the following groups would be the most
stable?
a. 15 people
b. 5 people
c. 10 people
d. 2 people
Answers
The group that would be the most stable based on size would be 10 people. So, the correct answer is c. 10 people.
What is a group?A group is a set of people or objects that are deemed to be equivalent or share a common feature. Group members may collaborate and share knowledge to achieve a common goal. The group's performance is frequently greater than the sum of its members' individual efforts, and this is known as the group effect.
What is group stability?Group stability is defined as the ability of a group to remain together and keep working toward its objective over time. It is essential for a group to achieve its objectives and is typically linked to the group's size and objective. A stable group can withstand external pressures and is less susceptible to breaking up or being disbanded.
How does size affect group stability?A group's size has a significant influence on its stability. Small groups are often more cohesive and productive than large groups. On the other hand, as a group's size grows, it becomes more challenging to sustain cohesion and productivity. A group of 10 people would be the most stable since it's not too large or too small. Thus, the correct option is c. 10 people.
To know more about group refer here:
https://brainly.com/question/14885504#
#SPJ11
What value of will make the triangles similar by the similarity theorem?
Answers
As similarity theorem, the value of x that will make the triangles similar by SSS similarity theorem is 77.
Similarity theorem:
In math, similarity theorem refers the line segment splits two sides of a triangle into proportional segments if and only if the segment is parallel to the triangle's third side.
Given,
Here we need to find the t value of will make the triangles similar by the similarity theorem.
For example, we are told that the 2 triangles are similar by SSS theorem.
Here we know that, SSS means Side - Side -Side and it is a congruence theorem which states that the 3 corresponding sides of two triangles have same ratio, then we can say that the two triangles are congruent by SSS theorem
Therefore, in the triangles ,applying the SSS postulate gives;
=> x/35 = 44/20
Then by applying the multiplication property of equality, let us multiply both sides by 35 to get;
=> x = (44 * 35)/20
When we simplify this one then we get,
=> x = 77
Therefore, the value of x is 77.
To know more about Similarity theorem here.
https://brainly.com/question/24167205
#SPJ4
A pile of pebbles has a weight of 85 kg.
Some of the pebbles were put into a small sack.
The rest of the pebbles is put into a large sack.
The pebbles in the large sack weigh 35 kg more than the pebbles in the small sack.
What is the weight of the pebbles in the large sack?
Answers
Variables:
x is the small sack’s weight.
Equation:
x = small sack’s weight - 25
x + 35 = bigs sack‘s weight - 60
2x + 35 = 85
x = 25
PLEASE HELP ME
Carlos needs to buy some new pencils from the school supply store. Carlos asks his classmates if they know the price for pencils. Angela says she bought 2 pencils for $0.50. Paige bought 3 pencils for $0.75, and Spencer bought 4 pencils for $1.00.
Answers
Answer:
0.25
Step-by-step explanation:
The answer is 0.25 i think
Find the night of the parallelogram wh hen it's :area is 2.5mm squared base is 5mm
Answers
Answer:
Height is 0.5
Step-by-step explanation:
height of parallelogram = area ÷ base
= 2.5 ÷ 5 = 0.5
what's the value of given expression
=》
\( \sqrt{12 + 4} \)
Answers
Answer: 4
Answer:
√{12+3}=√16=√4²=±4 is your answer
Solve the equation 15x + 22 = 7x +62
Answers
Answer:
hope it helps you........


Answer:
x = 5
Step-by-step explanation:
1. Subtract 22 from both sides.
15x = 7x + 62 - 22
2. Simplify 7x + 62 - 22 to 7x + 40.
15x = 7x + 40
3. Subtract 7x from both sides.
15x - 7x = 40
4. Simplify 15x - 7x to 8x.
8x = 40
5. Divide both sides by 8.
x = \(\frac{40}{8}\)
6. Simplify \(\frac{40}{8}\) to 5.
x = 5
how many possibilities are there for the win, place, and show (first, second, and third) positions in a horse race with 14 horses if all orders of finish are possible?
Answers
2,184 possibilities are there for the win, place, and show (first, second, and third) positions in a horse race with 14 horses if all orders of finish are possible.
What is permutation?
A permutation is the number of ways a set can be arranged or the number of ways things can be arranged.
P(n, r) = n!/(n-r)!
where n is number of elements and r is number of permutation
According to the given data:
We used the permutation formula
P(n, r) = n!/(n-r)!
Here n = number of elements = 14
and r = number of permutation = 3 ( 1st,2nd,3rd)
By putting the values in the formula
P(14,3) = 14!/(14–3)!
= 14.13.12.11!/11!
= 14.13.12
= 2,184
Hence 2184 possibilities are there for the win, place and show positions.
To know more about permutation visit
https://brainly.com/question/1216161
#SPJ4
Which of the x-values are solutions to the following inequality?
X < 365

Answers
The cost of 7 scarves is $85.75. What is the unit price
Answers
Answer:
The cost of 1 scarf is $12.25 each.
Step-by-step explanation:
can you help me with this

Answers
Answer:
she took 6 courses
Step-by-step explanation:
1. 200-50= 150
2. 150÷25 = 6
B. 6 courses
sign-up cost = $50
cost per course = $25
Piper paid = $200
First, subtract the sign-up cost from the amount paid:
$200 - $50 = $150
Now, divide the cost per course to the amount that is left to get the number of courses she took:
$150 ÷ $25 = 6 courses
triangle angle - sum theorem
what is the value of y?

Answers
Answer:
y = 5
Step-by-step explanation:
All angle measures in a triangle add up to 180°.
This means that 63 + 82 + 7y = 180Solving for Y:
Step 1: Combine like terms.
\((7y) + (63+82)=180\) \(7y + 145 = 180\)Step 2: Subtract 145 from both sides.
\(7y + 145 - 145 = 180 - 145\) \(7y = 35\)Step 3: Divide both sides by 7.
\(\frac{7y}{7} = \frac{35}{7}\) \(y = 5\)Step 4: Check if solution is correct.
\(7(5) + 63 + 82 = 180\) \(35 + 63 + 82 = 180\) \(98 + 82 = 180\) \(180 = 180\)Therefore, y = 5.
Have a lovely rest of your day/night, and good luck with your assignments! ♡
Property agent charges a commission of 5% on the first $10,000 and 2 1/4% on the remainder of the selling price. Find the amount of commission he will receive if he sells a piece of property for $46,000
Answers
The property agent will receive a commission of $1,310 if he sells the property for $46,000.
The property agent charges a commission of 5% on the first $10,000 of the selling price and 2 1/4% on the remainder of the selling price.
To find the amount of commission he will receive if he sells a piece of property for $46,000, we can break it down into two parts:
1. First, let's calculate the commission on the first $10,000. Since the commission rate is 5%, we can multiply $10,000 by 0.05 to find the commission on this portion:
Commission on first $10,000 = $10,000 * 0.05 = $500
2. Next, let's calculate the commission on the remainder of the selling price. To find the remainder, we subtract $10,000 from the selling price:
Remainder = Selling price - $10,000
Remainder = $46,000 - $10,000 = $36,000
The commission rate on the remainder is 2 1/4%, which can be expressed as a decimal as 0.0225. We can then multiply the remainder by this commission rate to find the commission on this portion:
Commission on remainder = $36,000 * 0.0225 = $810
Now, we can add the commission on the first $10,000 to the commission on the remainder to find the total commission:
Total commission = Commission on first $10,000 + Commission on remainder
Total commission = $500 + $810 = $1,310
Therefore, the property agent will receive a commission of $1,310 if he sells the property for $46,000.
To know more about the word remainder, visit:
https://brainly.com/question/29019179
#SPJ11
Suppose a random variable has a Poisson distribution. Find: a) P(y ≤ 2) when λ=3 b) P(y = 3) when λ=5
Answers
The probability values of the Poisson distribution are P(y ≤ 2) = 0.423 and P(y = 3) = 0.140
How to determine the probability of the Poisson distributionProbability 1
The given parameters here are:
P(y ≤ 2) when λ=3
The probability of a Poisson distribution is represented as
P(x) = λˣe⁻λ/x!
In this case,
x = y ≤ 2
This means that
y = 0, 1 and 2
So, we have
P(y ≤ 2) = P(0) + P(1) + P(2)
This gives
\(P(y \le 2) = \frac{3^0 \times e^{-3}}{0!} + \frac{3^1 \times e^{-3}}{1!} +\frac{3^2 \times e^{-3}}{2!}\)
Evaluate
\(P(y \le 2) = \frac{1 \times e^{-3}}{1} + \frac{3 \times e^{-3}}{1} +\frac{9 \times e^{-3}}{2}\)
Solving further, we have
P(y ≤ 2) = 0.050 + 0.149 +0.224
P(y ≤ 2) = 0.423
Probability 2
The given parameters here are:
P(y = 3) when λ = 5
The probability of a Poisson distribution is represented as
P(x) = λˣe⁻λ/x!
In this case,
x = y = 3
This gives
P(y = 3) = 5³ * e⁻⁵/3!
Evaluate
P(y = 3) = 125 * e⁻⁵/6
Solving further, we have
P(y = 3) = 0.140
Hence, the probability is 0.140
Read more about probability at
https://brainly.com/question/24756209
#SPJ1
Please guys, I need help with this. Find tan A. If necessary, write your answer as a fraction.

Answers
Answer:
tanA = \(\frac{55}{48}\)
Step-by-step explanation:
tanA = \(\frac{opposite}{adjacent}\) = \(\frac{BC}{AC}\) = \(\frac{55}{48}\)