Answers
Answer:
A. complementary
Step-by-step explanation:
Complementary is when 2 angles add up to 90°. Therefore, the angle in the image shown is a 90° angle. Hope this helps :)
Related Questions
What is the slope-intercept form of −16x−4y=−56?
Answers
Answer:
y=4x-4
move all terms containing y to the right side of the equation
−4y=-16x+16
divide the term and simplify
y=4x-4
Answer:
Step-by-step explanation:
m = -4
b = 14
Explanation in the pic below:-

I need help really bad plsss

Answers
Answer:
0
Step-by-step explanation:
(f-g)(x) = 12-sqrt(x)
plugging in 144, we get 12 - 12 = 0
one could also do f(x)-g(x) which would yield 24-24=0
PLEASE HELP ME!!!! WILL MARK BRAINLIEST

Answers
Answer:
h = 6
Step-by-step explanation:
Answer:
yikes! i hope someone helps you!!!!
Write the sum as a product. Simplify the product.
(–6) + (–6) + (–6) + (–6) + (–6) + (–6)
Answers
Answer:
-36
Step-by-step explanation:
what is the elasticity of demand when the quantity demanded is 8 tons and the price is $211? (round your answer to one decimal place.)
Answers
The elasticity of demand when the quantity demanded is 8 tons and the price is $211 = -3.8.
The elasticity of demand is the degree of how responsive the quantity demanded a good or provider is to trade in its price. The method for calculating the elasticity of a call is the share change in the amount demanded divided with the aid of the percentage alternate in price.
Using this method, we are able to calculate the elasticity of demand on your query as follows:
First, we want to find the share exchange in the amount demanded and the share change in charge.
To do that, we want to understand the initial and final values of quantity and price. Let’s expect that the initial amount demanded became 10 heaps and the initial fee turned into $200. Then, the very last quantity demanded is eight lots and the very last price is $211.
Next, we plug these values into the components for percent alternate: % trade = (final - preliminary) / (final + initial) * 100
The percentage exchange in quantity demanded is: (8 - 10) / (eight + 10) * 100 = -10%
The percentage exchange in rate is: (211 - 200) / (211 + 200) * 100 = 2.6%
Finally, we divide the proportion change in the amount demanded by using the share exchange in charge to get the pliancy of demand: -10% / 2.6% = -3.8
Therefore, the pliancy of demand while the amount demanded is 8 lots and the price is $211 is -3.8.
This way that the call for this top or service is inelastic, which means that a trade-in fee does now not have an effect on the quantity demanded.
To know more about the elasticity of demand,
https://brainly.com/question/14997249
Snow Instructions
Question 1 (1 point)
1) Tr +1= 7(1 +r)
a
One Solution
b
No Solution
C
Infinite Solutions
Answers
Answer:
C
Step-by-step explanation:
tr+1=7(1+r) Step 1: Add -7r to both sides. rt+1+−7r=7r+7+−7r rt−7r+1=7 Step 2: Add -1 to both sides. rt−7r+1+−1=7+−1 rt−7r=6 Step 3: Factor out variable r. r(t−7)=6 Step 4: Divide both sides by t-7. r(t−7) t−7 = 6 t−7 r= 6 t−7 Answer: r= 6 t−7
Hoped I helped
The formula below gives the area of a semi-circle, A, with a radius of r.
Solve the formula for the radius
Answers
The area of a semicircle with respect to radius is √2A/π sq. units.
What is a semicircle?A semicircle is half of a circle. It is a 2D shape formed when a circle is cut into two equal parts. We can create two semi-circles from any circle.
The area of a semicircle = π × radius² / 2
So, the area with respect to radius will be =
A = π × radius² / 2
2A = π × radius²
2A / π = radius²
Radius = √2A/π
Hence, the area of a semicircle with respect to radius is √2A/π sq. units.
Learn more about semicircles, click;
https://brainly.com/question/30023569
#SPJ7
charlyn walks completely around the boundary of a square whose sides are exactly 5 km long. from any point on her path she can see exactly 1 km horizontally in all directions. what is the area of the region consisting of a
Answers
The area of the region consisting of all the points Charlyn can see while walking around the boundary of a square whose sides are exactly 5 km long is 113 km².
Charlyn walks completely around the boundary of a square whose sides are exactly 5 km long. From any point on her path, she can see exactly 1 km horizontally in all directions.
The area Charlyn can see from any point of her path can be calculated by calculating the area of a square of side 2km (since 1 km horizontally in all directions is the same as a 2 km side square).
Hence, the area Charlyn can see is 2²=4 km².
The total distance Charlyn walks is 20 km.
So, the area she can see is 4 × 20 = 80 km².
However, we cannot take into account the corners of the square since Charlyn can only see halfway around the corner squares. She has 4 corners and from each corner, she sees half of the square. Hence the area seen from the corners is 4 × 0.5 × 2² = 8 km² (she can see half of the square).
Therefore, the total area of the region is 80 + 8 = 88 km².
Finally, we need to add the area of the square to get the final answer:
Area of the square= (5 km)² ⇒ 25 km²
Hence, the total area of the region consisting of all the points Charlyn can see while walking around the boundary of a square whose sides are exactly 5 km long is
88 km² + 25 km² ⇒ 113 km²
To know more about the "area of the region": https://brainly.com/question/1297097
#SPJ11
Alice brought 6 container of lemonade each container had 1 1/2 of a cup lemonade how many cups of lemonade did she have in all
Answers
*REMEMBER: 1 1/2 = 3/2*
can you help me tell me if its a blurry pic

Answers
Answer:
73
Step-by-step explanation:
Use the PEMDAS method. Start off with exponents, which is 3x3, which equals 9. Then do multiplication, because it comes before addition. So you multiply 9 by 8 to get 72. Then it says +1 so 72+1 equals 73. (Final Answer)
a canister of cheese ball measures 12 inches high and its base has a diameter of 6 inches. what is the volume of a canister (rounded to the nearest 10
Answers
The volume of the canister is 339.1 cubic inches.
The volume of a cylinder is calculated by using the formula V=πr²h, where r is the radius of the cylinder and h is the height of the cylinder.
The radius of the cylinder is half of the diameter, so the radius of the canister is 3 inches.
Using the formula, we can calculate the volume of the canister as follows:
V = π×3²×12
V = 108×3.14
V = 339.1 cubic inches
Therefore, the volume of the canister is 339.1 cubic inches.
To learn more about the volume visit:
https://brainly.com/question/13338592.
#SPJ1
2nd brainliest for the day
answer
⬇⬇
Answers
Answer:
..............
...............
Step-by-step explanation:
Answer:
?
Step-by-step explanation:
wow free points thanks
Fill in the missing numbers to complete the linear equation that gives the rules for this tablex: 4, 5, 6, 7y: 56, 70, 84, 98y = ?x + ?
Answers
the two points are
A(4,56) and B(5,70)
so, the linear equation is
\(y-70=\frac{70-56}{5-4}(x-5)\)\(\begin{gathered} y-70=\frac{14}{1}(x-5)_{} \\ y-70=14x-70 \end{gathered}\)\(y=14x\)thus, the equation is
y = 14x + 0
so we can put 14 in first place and 0 in second
Use z scores to compare the given values.
In a recent awards ceremony, the age of the winner for best actor was
32
and the age of the winner for best actress was
53.
For all best actors, the mean age is
43.7
years and the standard deviation is
8.8
years. For all best actresses, the mean age is
34.5
years and the standard deviation is
11.2
years. (All ages are determined at the time of the awards ceremony.) Relative to their genders, who had the more extreme age when winning the award, the actor or the actress? Explain.
Question content area bottom
Part 1
Since the z score for the actor is
z=enter your response here
and the z score for the actress is
z=enter your response here,
the
▼
actor
actress
had the more extreme age.
(Round to two decimal places.)
Answers
The z score for the actor is -2.04, and the z score for the actress is 1.69. The actor had the more extreme age as their z score was further away from the mean compared to the actress.
z score for actor
z = (32 - 43.7) / 8.8 = -2.04
z score for actress
z = (53 - 34.5) / 11.2 = 1.69
Since the z score for the actor is more negative than the z score for the actress, the actor had the more extreme age.
The z score is a measure of how far a specific value is away from the mean, in terms of standard deviations. A negative z score indicates that the value is below the mean, while a positive z score indicates that the value is above the mean.
In this case, both the actor and the actress had ages that were below the mean for their respective genders. However, the actor's age was further below the mean than the actress's age. Therefore, the actor had the more extreme age.
To know more about z score refer here :
https://brainly.com/question/33566793#
#SPJ11
(-5)x (-5) x (-5) x (-5) =
What would the answer be
20 points
Answers
Answer:
\(625x^{3}\)
Step-by-step explanation:
what is x:6-3(3x-5)= -24
Answers
To solve x:
1- Use distributive property to remove the parenthesis:
\(\begin{gathered} a(b\pm c)=ab\pm ac \\ \\ 6-9x+15=-24 \\ 21-9x=-24 \end{gathered}\)2- Subtract 21 in both sides of the equation:
\(\begin{gathered} 21-21-9x=-24-21 \\ -9x=-45 \end{gathered}\)3- Divide both sides of the equation into -9:
\(\begin{gathered} \frac{-9}{-9}x=\frac{-45}{-9} \\ \\ x=5 \end{gathered}\)Then, x is 5only six and seven needed
6- G and P are collinear
7- Points D,H and P are collinear

Answers
Answer: 67 87 34
Step-by-step explanation:
A robotic vacuum cleaner vacuums one hotel room in 3 hour. At this rate, how many rooms of the same size will it vacuum in 3 hours
Answers
Pedro is a salesman for a department store. He earns a commission of 8.5% on his sales. If he sold $1,200 in merchandise, what would his total commission be?
Answers
Answer: $102
Step-by-step explanation:
From the question, we are informed that Pedro earns a commission of 8.5% on his sales and that he sold $1,200 in merchandise.
Therefore, his total commission will be:
= 8.5% × $1200
= 8.5/100 × $1200
= 0.085 × $1200
= $102
A cell phone company charges $39.99 flat fee each month, plus $0.05 per minute. If m represents the number of minutes used in a month, which expression can be used to calculate a monthly payment?
39.99 + m
39.99 + m
0.05m + 39.99
0.05 m + 39.99
39.99m + 0.05
39.99 m + 0.05
0.05m
0.05 m
Answers
Answer:
Step-by-step explanation: A cell phone company charges $39.99 flat fee each month, plus $0.05 per minute. If m represents the number of minutes used in a month, which expression can be used to calculate a monthly payment?
39.99 + m
39.99 + m
0.05m + 39.99
0.05 m + 39.99
39.99m + 0.05
39.99 m + 0.05
0.05m
0.05 m
PLZ help it is due today

Answers
Answer:
45 gallons
Step-by-step explanation:
60/4=15 so each quarter is 15 gallons. He already has 15 gallons so he needs 45 more.
Answer:
45 gallons
Step-by-step explanation:
60*1/4=15
15*3= 45
Gia is making frittata using 7/8 cup of vegetables for every 1/3 cup of cheese.she wants to know the amount of vegetables she uses per cup of cheese
Answers
Answer: 21/8 or 2 and 5/8
Step-by-step explanation:
What is the 10th term of the geometric sequence where a1 = 8,192 and a3 = 512? (1 point)0.031250.06250.1250.15625
Answers
Hello!
Let's write some important information that we know:
• a1 ,= 8,192
,• a3 ,= 512
,• q ,= ?
First, we have to discover the value of q, using the formula below:
\(a_n=a_1\cdot q^{n-1}\)Let's replace n as 3:
\(\begin{gathered} 512=8,192\cdot q^{3-1} \\ 512=8,192\cdot q^2 \\ 8,192q^2=512 \\ q^2=\frac{512}{8192}=\frac{1}{16} \\ \\ q^=\sqrt{\frac{1}{16}}=\frac{\sqrt{1}}{\sqrt{16}}=\frac{1}{4} \\ \end{gathered}\)So, q = 1/4.
With this information, now we are able to calculate the 10th term, using the same formula:
\(\begin{gathered} a_n=a_1\cdot q^{n-1} \\ a_{10}=8,192\cdot(\frac{1}{4})^{10-1} \\ \\ a_{10}=8,192\cdot(\frac{1}{4})^9 \\ \\ \boxed{\mathrm{a_{10}=\dfrac{1}{32}}=0.03125} \end{gathered}\)Answer:Alternative A. 0.03125
Answer:
I just did it and 0.03125 is correct
Step-by-step explanation:
Which of the following quantities are inversely proportional?
The time it takes to use a 5 lb bag of dry cat food and the number of cats, if each cat eats the same amount of food per same time interval.
Answers
Answer:
inversely proportional
Step-by-step explanation:
How much is the square root of 4761?
Answers
Answer:
square root of 4761 = 69
Step-by-step explanation:
find the distance of the point (2,6,−4)(2,6,−4) from the line r(t)=⟨1 3t,1 4t,3−2t⟩.
Answers
The distance between the point (2, 6, -4) and the line r(t) = ⟨1, 3t, 1, 4t, 3, -2t⟩ can be calculated using the formula d = ||PQ||/||v||, where PQ is the vector connecting the point P to any point Q on the line, and v is the direction vector.
To find the distance between the point P(2, 6, -4) and the line defined by the parametric equations r(t) = ⟨1, 3t, 1, 4t, 3, -2t⟩, we can use the formula for the distance between a point and a line in three-dimensional space.
The formula for the distance between a point and a line is given by:
d = ||PQ||/||v||
where PQ is the vector connecting the point P to any point Q on the line, v is the direction vector of the line, and || || represents the magnitude of a vector.
Let's first find the direction vector of the line. By examining the parametric equations, we can see that the direction vector of the line is v = ⟨1, 4, -2⟩.
Now, we need to find the vector PQ connecting the point P(2, 6, -4) to any point Q on the line. We can represent PQ as the difference between the coordinates of P and Q:
PQ = ⟨2 - 1, 6 - 3t, -4 - 1, 4t, -4 - 3, -2t⟩ = ⟨1, 6 - 3t, -5, 4t, -7, -2t⟩
Next, we calculate the magnitude of PQ:
||PQ|| = √(1^2 + (6 - 3t)^2 + (-5)^2 + (4t)^2 + (-7)^2 + (-2t)^2)
= √(1 + 36 - 36t + 9t^2 + 25 + 16t^2 + 49 + 4t^2)
= √(29t^2 - 36t + 111)
Finally, we calculate the magnitude of the direction vector v:
||v|| = √(1^2 + 4^2 + (-2)^2) = √(1 + 16 + 4) = √21
Now we can substitute these values into the formula for the distance:
d = ||PQ||/||v|| = (√(29t^2 - 36t + 111))/√21
To find the minimum distance between the point P and the line, we need to minimize the function d with respect to t. We can accomplish this by finding the critical points of the function and determining the value of t that gives the minimum distance.
Taking the derivative of d with respect to t and setting it equal to zero, we have:
d' = (29t - 18)/(√21(√(29t^2 - 36t + 111))) = 0
Solving for t, we get:
29t - 18 = 0
29t = 18
t = 18/29
By substituting this value of t into the formula for d, we can find the minimum distance between the point P and the line.
d = (√(29(18/29)^2 - 36(18/29) + 111))/√21
Simplifying this expression will give us the final value of the distance.
In summary, the distance between the point (2, 6, -4) and the line r(t) = ⟨1, 3t, 1, 4t, 3, -2t⟩ can be calculated using the formula d = ||PQ||/||v||, where PQ is the vector connecting the point P to any point Q on the line, and v is the direction vector
Learn more about distance here
https://brainly.com/question/26550516
#SPJ11
Draw a line representing the “rise” and a line representing the “run” of the line
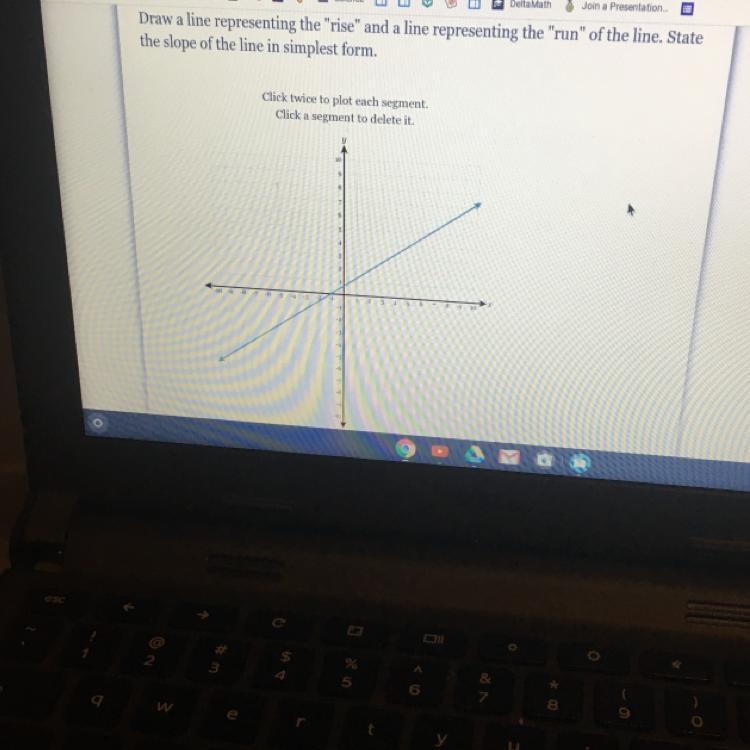
Answers
Rise is going up
run is going left or right
Slope is the straight line you get from your coordinates.
Hope that helps:)
in a supermarket each cup costs of 4$ and each bowl cost $6 if you want to spend exactly $34, write an equation in standard form modeling this situation. Let c represent the number of cups you buy and let b represent the number of bowls you buy
BEST ANSWERED GETS MARKED
Don't put a answer to get points
Answers
The GDP deflator in year 4 is 120 and the GDP deflator in year 5 is 130.
The rate of inflation between years 4 and 5 is
A) -10. B) 7.7. C) 8.33. D) 10.
11.) Refer to Table 6.5. Assume that this economy produces only two goods Good X and Good Y.
If year 1 is the base year, the value for this economy's GDP deflator in year 2 is
A) 84.5. B) 92.4. C) 105.0. D) 108.2.
12.) Refer to Table 6.5. Assume that this economy produces only two goods Good X and Good Y.
If year 2 is the base year, the value for this economy's real GDP in year 3 is
A) $270. B) $178. C) $156. D) $132.
SHOW THE MATH WORK FOR PRACTICE PROBLEMS
Answers
The rate of inflation between years 4 and 5 is is option (C) 8.33%
The Gross Domestic Product (GDP) deflator is a measure of inflation that reflects the average price level of all goods and services produced in an economy. It is calculated by dividing the nominal GDP (the total value of goods and services produced in an economy at current market prices) by the real GDP (the total value of goods and services produced in an economy at constant prices).
In this question, we are given that the GDP deflator in year 4 is 120 and the GDP deflator in year 5 is 130. This means that the average price level of goods and services in the economy has increased from year 4 to year 5.
To calculate the rate of inflation between the two years, we use the formula:
Inflation rate = ((GDP deflator in year 5 - GDP deflator in year 4) / GDP deflator in year 4) × 100%
Substituting the values given in the question, we get:
Inflation rate = ((130 - 120) / 120) × 100%
Inflation rate = (10 / 120) × 100%
Inflation rate = 8.33%
Therefore, the correct option is (C) 8.33%
Learn more about inflation rate here
brainly.com/question/30112292
#SPJ4
A locker combination has two nonzero digits, and the digits can be repeated. The first number is 3. What is the probability that the second number is 3?
A. 1/27
B. 1/9
C. 1/8
D. 8/9
WILL GIVE BRAINLIEST
Answers
Answer:
I would say A. 1/27
