Answers
Answer:
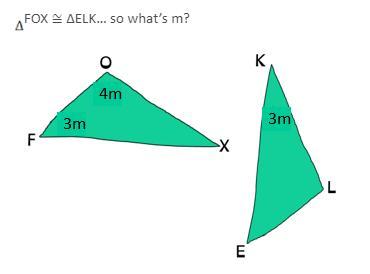
m=18 because the oxf =3m so
3m+3m+4m=10
180÷10=m
m=18
Related Questions
The Venn diagram shows the number of customers who have purchased different types of pets from a pet store, where C represents customers who have purchased cats, D represents customers who have purchased dogs, and F represents customers who have purchased fish.
Circles C, D, and F overlap. Circle C contains 15, circle D contains 21, and circle F contains 12. The overlap of C and F contains 2, the overlap of F and D contains 0, and the overlap of D and C contains 3. The overlap of all 3 circles contains 1. Number 14 is outside of the circles.
How many people are in the set C ∩ D?
4
6
36
38
Answers
The number of people in the set C ∩ D (customers who purchased both cats and dogs) is obtained by adding the overlap of D and C (3) with the overlap of all 3 circles (1), resulting in a total of 4 individuals.
The correct answer is 4.
To determine the number of people in the set C ∩ D (customers who have purchased both cats and dogs), we need to analyze the overlapping regions in the Venn diagram.
Given information:
- Circle C (cats): 15
- Circle D (dogs): 21
- Circle F (fish): 12
- Overlap of C and F: 2
- Overlap of F and D: 0
- Overlap of D and C: 3
- Overlap of all 3 circles: 1
- Number outside of circles: 14
To determine the number of people in the set C ∩ D (customers who have purchased both cats and dogs), we need to consider the overlapping region between circles C and D.
From the information given, we know that the overlap of D and C is 3. Additionally, we have the overlap of all 3 circles, which is 1. The overlap of all 3 circles includes the region where customers have purchased cats, dogs, and fish.
To calculate the number of people in the set C ∩ D, we add the overlap of D and C (3) to the overlap of all 3 circles (1). This gives us 3 + 1 = 4.
Therefore, from the options given correct one is 4.
For more such information on: set
https://brainly.com/question/24713052
#SPJ8
Answer: 4
Step-by-step explanation:
trust me bro
Translate to a system of equations and solve:Whitney has a collection of nickels and dimes, with a total value of $5.40. The number of nickels is two less than four times the number of dimes. How many nickels and how many dimes does she have?
Answers
Let n and d be the number of nickels and dimes, respectively. Since the total values is $5.40, we can write
\(0.05n+0.10d=5.40\)On the other hand, since the number of nickels is two less than four times the number of dimes, we have
\(n=4d-2\)Then, we have the following system of equations:
\(\begin{gathered} 0.05n+0.10d=5.40\ldots(a) \\ n=4d-2\ldots(b) \end{gathered}\)Solving by substitution method.
By substituting equation (b) into (a), we have
\(0.05(4d-2)+0.10d=5.40\)By distributing 0.05 into the parenthesis, we have
\(0.2d-0.1+0.10d=5.40\)and by combining similar terms, we have
\(0.3d-0.1=5.40\)then, we have
\(\begin{gathered} 0.3d=5.40-0.1 \\ 0.3d=5.30 \end{gathered}\)By dividing both sides by 0.3, we get
\(\begin{gathered} d=\frac{5.30}{0.3} \\ d=17.666 \end{gathered}\)D(5, 7), E(4, 3), and F(8, 2) form the vertices of a triangle. What is mZDEF?
Answers
Answer: \(m\angle DE F=90^{\circ}\)
Step-by-step explanation:
The slope of \(DE\) is \(\frac{7-3}{5-4}=4\).
The slope of \(EF\) is \(\frac{3-2}{4-8}=-\frac{1}{4}\).
Thus, \(DE \perp EF\), meaning \(m\angle DE F=90^{\circ}\).
Solve the equation. Enter your answer as an equation showing the value of the variable (like s = 6, or w = 1/2). Use a slash mark (/) as the fraction bar if necessary. PLEASE ANSWER ASAP AND GET BRAINLIEST FOR A GOOD ANSWER
x/2 * x = 3
Answers
Answer:
x = ±√6
Step-by-step explanation:
x/2 * x = 3
x²/2 = 3
x² = 3 * 2
x² = 6
x = ±√6
a) Basis Step
b) Inductive Step

Answers
Basis step: Let \(n=2\). Then
\(2! = 2\cdot1 = 2\)
and
\(2^2 = 4\)
so \(2! < 2^2\).
Inductive step: Assume \(k! < k^k\). We want to show that \((k+1)! < (k+1)^{k+1}\).
Now
\((k+1)! = (k+1) \cdot k! \\\\ ~~~~~~~~~~~ < (k+1) \cdot k^k \\\\ ~~~~~~~~~~~ < (k+1) \cdot k^{k+1} \\\\ ~~~~~~~~~~~ < (k+1) \cdot (k+1)^{k+1}\)
where the first inequality follows from the induction hypothesis; the second follows from multiplying the right side by some \(k>2\); and the third from adding the remaining terms to complete the binomial expansion \((k+1)^{k+1}\), all of which are positive since they are some power of \(k>2\).
QED
One side of a square is 33 inches long. The area of the square is _ square inches.

Answers
The area of the square is 1084 square inches.
What is the area of a square?The area of a square can be calculated as the square of the sides of a given figure. A square is always a rectangle, parallelogram, a rhombus and a quadrilateral but its reverse statement may or may not be true.(ie it is not always necessary that a rectangle is a square or a parallelogram is a square or a rhombus is a square).
The area of a square = side x side
Given;
One side of square= 33inches
Area of square= A^2
=33x33
=1089
Therefore, the area will be 1089m^2
More about the area of the square link is given below.
brainly.com/question/1658516
#SPJ1
How much of the circle is shaded? Write your answer as a fraction in simplest form.

Answers
Answer:
11/24
Step-by-step explanation:
1/3 =8/24
1/8= 3/24
8/24+3/24=11/24
Given (21,7) and (x,
1), find all x such that the distance between these two points is 17.
Answers
Answer:
21+/-sqrt(253)=x
So one value for x is 21+sqrt(253)
and another is 21-sqrt(253)
Problem:
Given (21,7) and (x,1), find all x such that the distance between these two points is 17.
Step-by-step explanation:
Change in x is x-21
Change in y is 7-1=6
distance^2=(change in x)^2+(change in y)^2
17^2=(x-21)^2+(6)^2
289=(x-21)^2+36
Subtract 36 on both sides:
289-36=(x-21)^2
253=(x-21)^2
Take square root of both sides:
+/-sqrt(253)=x-21
Add 21 on both sides:
21+/-sqrt(253)=x
There are two values of "x" that satisfy the given condition:
\(1. \( x = 21 + \sqrt{253} \) \ 2. \( x = 21 - \sqrt{253} \)\) These are the values of "x" such that the distance between the points (21, 7) and (x, 1) is 17.
Given two points (21, 7) and (x, 1) and the distance formula:
\(Distance = \sqrt{{(x_2 - x_1)^2 + (y_2 - y_1)^2}}\)
We want to find all the values of "x" such that the distance between these two points is 17:\(\[ 17 = \sqrt{{(x - 21)^2 + (1 - 7)^2}} \]\)
Simplifying the equation: \(\[ 289 = (x - 21)^2 + 36 \]\)
Moving the constant term to the other side:\(\[ (x - 21)^2 = 253 \]\)
Taking the square root of both sides: \(\[ x - 21 = \pm \sqrt{253} \]\)
Finally, isolating \("x": \[ x = 21 \pm \sqrt{253} \]\)
So, there are two values of "x" that satisfy the given condition:
\(1. \( x = 21 + \sqrt{253} \) \ 2. \( x = 21 - \sqrt{253} \)\)
These are the values of "x" such that the distance between the points (21, 7) and (x, 1) is 17.
To know more about distance:
https://brainly.com/question/33716087
#SPJ3
Smart Kitchen sells chocolate nonpareils in 8 oz. containers. Because the candy pieces are not uniform, the weight of each individual container varies slightly. A random sample of nine containers was weighed on a precision scale and the results are shown below:
8.09
8.20
8.03
7.86
7.87
8.06
8.43
7.99
8.08
Determine the interquartile range for this sample. Are there any outliers in this data set?
Answers
The interquartile range of the data will be 0.215.
How to calculate the valueThe lower half is:
7.86, 7.87, 7.99, 8.03
The median of the lower half is:
(Q1) = (7.87 + 7.99) / 2 = 7.93
The upper half is:
8.06, 8.08, 8.09, 8.20, 8.43
The median of the upper half is:
(Q3) = (8.09 + 8.20) / 2 = 8.145
The IQR is the difference between the third and first quartiles:
IQR = Q3 - Q1 = 8.145 - 7.93 = 0.215
Lower outlier threshold: Q1 - 1.5 x IQR = 7.93 - 1.5 x 0.215 = 7.5955
Upper outlier threshold: Q3 + 1.5 x IQR = 8.145 + 1.5 x 0.215 = 8.4795
None of the data points fall outside these thresholds, so there are no outliers in this data set.
Learn more about interquartile range on
https://brainly.com/question/28922347
#SPJ1
can someone tell me what dropped mean in skyward means if you use it pleaseeeeee???!!!!

Answers
It means the class has been removed from your schedule and replaced with another class.
which grows at the fastest rate for increasing values of x
\(f(x)=4*2^x\\h(x)=9x^2+25\\\\g(x)=15x+6\)
Answers
The function that grows at the fastest rate for increasing values of x is f(x) = 4×2ˣ.
We can see this by comparing the growth rates of the three functions for larger and larger values of x.
As x gets larger, the exponential function f(x) grows much faster than the other two functions, which are both polynomial functions.
if we plug in x = 10, we get:
f(10) = 4×2¹⁰ = 4×1024 = 4096
h(10) = 9×10² + 25 = 925
g(10) = 15×10 + 6 = 156
As we can see, f(10) is much larger than h(10) and g(10), indicating that f(x) grows at a much faster rate than the other two functions.
To learn more on Functions click:
https://brainly.com/question/30721594
#SPJ1
hay 1230 personas, entre hombres y mujeres. Si se sabe que el número de mujeres, supera en 150 al número de hombres. ¿Cuántos hombres están habitando la mini ciudad?
Answers
There are 540 men living in the mini city.
x + (x + 150) = 1230
Simplifying this equation, we get:
2x + 150 = 1230
Subtracting 150 from both sides, we get:
2x = 1080
Dividing both sides by 2, we get:
x = 540
Therefore, there are 540 men living in the mini city.
To check our answer, we can substitute x = 540 into our original equation:
540 + (540 + 150) = 1230
690 = 1230
This is false, so there must be an error in our calculation. We can double-check our work by trying a different approach.
We know that the number of women exceeds the number of men by 150, so we can represent the number of women as (x + 150). We also know that the total number of people is 1230, so we can set up an equation:
x + (x + 150) = 1230
Simplifying this equation, we get:
2x + 150 = 1230
Subtracting 150 from both sides, we get:
2x = 1080
Dividing both sides by 2, we get:
x = 540
For such more questions on mini
https://brainly.com/question/29266607
#SPJ8
Parallelogram ABCD had coordinates A(0,7) and C(2, 1) Which statement will prove ABCD is a Rectangle?
The midpoint of AC is (1, 4).
The length of BD is √40.
The slope of BD is 1/3
The slope of AB is 1/3.

Answers
Answer:
Option (2)
Step-by-step explanation:
ABCD is a parallelogram having vertices A(0,7) and C(2, 1).
Therefore, length of diagonal AC = \(\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\)
= \(\sqrt{(0-2)^2+(7-1)^2}\)
= \(\sqrt{40}\)
Since, diagonals of a rectangle measure the same and bisect each other,
Length of other diagonal BD will also measure \(\sqrt{40}\).
Therefore, Option (2) will be the correct option.
The graph above is a transformation of the function f(x) = x^2
Write an equation for the function graphed above .
Answers
Answer: There is no graph
Step-by-step explanation: you cannot solve the problem without the graph
HURRY INVERSE OF A FUCTION

Answers
First, replace f(x) with y . ...
Replace every x with a y and replace every y with an x .
Solve the equation from Step 2 for y . ...
Replace y with f−1(x) f − 1 ( x ) . ...
Verify your work by checking that (f∘f−1)(x)=x ( f ∘ f − 1 ) ( x ) = x and (f−1∘f)(x)=x ( f − 1 ∘ f ) ( x ) = x are both true.
6th grade math I mark as brainliest

Answers
Answer:
D
DDDDDDDDDDDDDDDDDDDDDDDDDDDD
Step-by-step explanation:
Answer:
D
Step-by-step explanation:
log base 4 of 9
HELPPPPPPPP
Answers
Answer:
1.58 ish
Step-by-step explanation:
For a logx y = z, x^z = y
So here, log4 9= 1.58 or so
1.5849625007
A bus holds 39 passengers. How many buses will 420 people need
Answers
Answer:
11 buses
Step-by-step explanation:
Marge wants to buy a CD player. The CD player costs $300. Marge gets 30% off. How much did Marge pay?
Answers
Answer:
300-(300*0.3) = 210
Step-by-step explanation:
Answer:
$210
Step-by-step explanation:
300*30%=90
300-90=210
(no links please and than you! :))

Answers
Answer:
Line D represents a proportional relationship.
Step-by-step explanation:
In an easy way to explain what a proportional relationship is, it is basically a line graph that passes through the origin point. When a line graph passes through the origin point (0,0), it is proportional.
Other graphs do not intersect the origin points so they are not proportional.
Answer:
Line D represents a proportional relationship.
Step-by-step explanation:
In an easy way to explain what a proportional relationship is, it is basically a line graph that passes through the origin point. When a line graph passes through the origin point (0,0), it is proportional.
Other graphs do not intersect the origin points so they are not proportional.
Please only answer if u actually know pls!

Answers
At a price of $3, the total revenue will be the greatest, and the company will sell 3 units at that price.
To find the total revenue at each price, we can multiply the price by the corresponding quantity.
Price Quantity Total Revenue
$6 0 $0
$5 1 $5
$4 2 $8
$3 3 $9
$2 4 $8
$1 5 $5
To find the price at which total revenue is the greatest, we look for the highest value in the Total Revenue column.
In this case, the highest total revenue is $9, which occurs when the price is $3.
At a price of $3, the company will sell 3 units (as indicated in the Quantity column).
To learn more on Revenue click:
https://brainly.com/question/14952769
#SPJ1
You sailed 0.055 units to the left and found treasure at 0.085 units find where the ship started
Answers
Starting position = Treasure location + distance sailed to the left
Since we know going in the left direction typically is a means of subtraction, we can use inverse operations to solve this. We can set up an addition problem to solve this:
Starting position = 0.085 + 0.055
Starting position = 0.03 units
Therefore, the ship started at 0.03 units.
Which value is an input of the function?
-14
-2
0
4

Answers
Answer:
4
Only answer on the x-axis
Answer:
It's 4.
Step-by-step explanation:
Did the test on edge2021
What is the area of the circle below in square yards?
Round your answer to the nearest tenth.
(Use 3.14 for pi.)

Answers
The area of the circle is 78.5 yards.
It is given that the radius of the circle is 5 yards.
In geometry, the area enclosed by a circle of radius r is пr².Here the Greek letter п represents the constant ratio of the circumference of any circle to its diameter, approximately equal to 3.14159.
We have to find the area of the circle.
Formula:
Area of circle = пr²
= 3.14 (5)²
= 78.5 yards²
Therefore the area of the circle is 78.5 yards².
To know more about the Area of the circle refer to the link given below:
https://brainly.com/question/10645610
#SPJ9
In each triangle ABC, angle C is a right angle. Find the value of the trig function indicated.

Answers
As given triangle ABC, angle C is a right angle if a=6, c=10 then value of trigonometric function is sin A = 3/5.
As given in the question,
In triangle ABC,
∠C = 90°
Side a is opposite to angle A
c is the hypotenuse opposite to angle C
a = 6
c = 10
sin A = ( opposite side) / Hypotenuse
⇒sin A = a/c
⇒sin A = 6/10
⇒sin A = 3/5
Therefore, as given triangle ABC, angle C is a right angle if a=6, c=10 then value of trigonometric function is sin A = 3/5.
Learn more about trigonometric function here
brainly.com/question/14746686
#SPJ1
raíz cuadrada de 28 más raíz cuadrada de 7 + 35 sobre raíz cuadrada de 7
Answers
Answer:
21.1660104885
Step-by-step explanation:
Tuve que buscarlo, no lo sabía
Find the power of 9↑1. Type your answer using digits.
Answers
Answer:
9^1 has a power of 1. it evaluates to 9
Step-by-step explanation:
Answer Explanation:
Complete the statements about the shapes on the graphs
Answers
A bag contains 4 white,5 blacks and 2 blue balls. 3 balls are drawn one after the other without replacement from the bag .what is the probability that they are of different color
Answers
Answer:
24/99
Step-by-step explanation:
From the question given above, the following data were obtained:
White (W) balls = 4
Black (B) balls = 5
Blue (Bl) balls = 2
Probability that they are of different color =?
Next, we shall determine the total number of balls in the bag. This can be obtained as follow:
White (W) balls = 4
Black (B) balls = 5
Blue (Bl) balls = 2
TOTAL = 4 + 5 + 2 = 11 balls
Next, we shall determine the possible outcome of draw. This can be obtained as follow.
The possible outcome could be:
WBLB or WBBL or BBLW or BWBL or BLBW or BLWB
Next we shall determine the probability of each of outcomes.
Since the ball is drawn without replacement, it means the total number of ball will reduce after each draw.
White (W) balls = 4
Black (B) balls = 5
Blue (Bl) balls = 2
TOTAL = 11
P(WBLB) = 4/11 × 2/10 × 5/9 = 40/990
P(WBLB) = 4/99
P(WBBL) = 4/11 × 5/10 × 2/9 = 40/990
P(WBBL) = 4/99
P(BBLW) = 5/11 × 2/10 × 4/9 = 40/990
P(BBLW) = 4/99
P(BWBL) = 5/11 × 4/10 × 2/9 = 40/990
P(BWBL) = 4/99
P(BLBW) = 2/11 × 5/10 × 4/9 = 40/990
P(BLBW) = 4/99
P(BLWB) = 2/11 × 4/10 × 5/9 = 40/990
P(BLWB) = 4/99
Finally, we shall determine the probability that they are of different color. This can be obtained as follow:
P(WBLB) = 4/99
P(WBBL) = 4/99
P(BBLW) = 4/99
P(BWBL) = 4/99
P(BLBW) = 4/99
P(BLWB) = 4/99
Probability that they are of different color =?
Probability that they are of different color = P(WBLB) + P(WBBL) + P(BBLW) + P(BWBL) + P(BLBW) + P(BLWB)
= 4/99 + 4/99 + 4/99 + 4/99 + 4/99 + 4/99
= (4 + 4 + 4 + 4 + 4 + 4)/99
= 24/99
Thus, the probability that they are of different color is 24/99
A figure is shown, where lines CE and FD intersect at point B.
.
A figure is shown, where lines CE and FD intersect at point B.
.
Angle ABC is complementary to angle DBC.
What is the measure, in degrees of ?
Answers
Answer:
Step-by-step explanation:
Its 4.51