Jason ran 7/8 of a mile in 12 minutes. How many miles can Jason run in 1 hour
Answers
Answer:
36/8 miles which is 4 4/8 or 4 1/2 miles.
Step-by-step explanation:
Hope this helps :)
The answer is a here is proof

Related Questions
Let f(x) = x4 + 2x3 + 8x² + 4x. f'(x) = ____
f'(5) = ____
f" (x) = _____
ƒ" (5) = _____
Answers
f'(x) = 4x³ + 6x² + 16x + 4
f'(5) = 4(5)³ + 6(5)² + 16(5) + 4
f"(x) = 12x² + 12x + 16
f"(5) = 12(5)² + 12(5) + 16
The derivative of a polynomial function f(x) can be found by differentiating each term of the polynomial separately. In this case, the given function is f(x) = x^4 + 2x^3 + 8x^2 + 4x. To find the derivative f'(x), we differentiate each term with respect to x. The derivative of x^n, where n is a constant, is nx^(n-1). Applying this rule, we get:
f'(x) = 4x^3 + 3(2x^2) + 2(8x) + 4 = 4x^3 + 6x^2 + 16x + 4
To find the value of f'(5), we substitute x = 5 into the derivative function:
f'(5) = 4(5)^3 + 6(5)^2 + 16(5) + 4 = 500
The second derivative, f''(x), is the derivative of the first derivative f'(x). To find f''(x), we differentiate f'(x) with respect to x:
f"(x) = 12x^2 + 6(2x) + 16 = 12x^2 + 12x + 16
To find the value of f''(5), we substitute x = 5 into the second derivative function:
f"(5) = 12(5)^2 + 12(5) + 16 = 376
In summary:
f'(x) = 4x^3 + 6x^2 + 16x + 4
f'(5) = 500
f"(x) = 12x^2 + 12x + 16
f"(5) = 376
Learn more about polynomial function f(x)
brainly.com/question/29112176
#SPJ11
If the rate at which flour is poured into a tank is given by F(t) = 36/1, in pounds per second, how much flour is poured into the tank in the first 2.5 seconds?a. 11.384 poundsb. 37.947 pounds c. 56.921 poundsd. 94.868 pounds
Answers
To find the amount of flour poured into the tank in the first 2.5 seconds, we need to calculate the definite integral of the given rate function F(t) over the interval [0, 2.5].
The rate at which flour is poured into the tank is given by F(t) = 36/1, in pounds per second. Integrating this function will give us the total amount of flour poured into the tank over the given time interval.
The integral of F(t) with respect to t can be calculated as follows:
∫ F(t) dt = ∫ (36/1) dt
Integrating the constant term 36 gives:
= 36t
To find the definite integral over the interval [0, 2.5], we substitute the upper and lower limits of integration:
= 36(2.5) - 36(0)
= 90 - 0
= 90 pounds
Therefore, the amount of flour poured into the tank in the first 2.5 seconds is 90 pounds. None of the provided answer choices (a, b, c, d) match this result.
To know more about function refer hear
https://brainly.com/question/30721594#
#SPJ11
Please help with this

Answers
Answer:
142.75
Step-by-step explanation:
This histogram shows the number of peanuts per bag of trail mix. Choose ALL statements about the data that are true? A) The most common range of peanuts per bag is 30-39. B) There are more bags with 10-19 peanuts than 40-49. C) The least common range of peanuts per bag is 10-19. D) The ranges with the same number of bags are 10-19 and 40-49. E) The ranges with the same number of bags are 20-29 and 50-59.
Answers
Answer:
A), C), E)
Step-by-step explanation:
Randy’s circular garden has a radius of 1.5 feet. He wants to enclose the garden with edging that costs $0.75 per foot. About how much will the edging cost?
Answers
Answer:
$2.25 is answer
perimeter of circular garden=2πr=2×π×1.5=3πfeet
1 foot=$0.75
3πfeet=3×$0.75=$2.25
Help me please I don’t understand

Answers
The age of Michelle is 30 years old (option A).
What is Michelle's age?The mathematical operation that would be used to determine the required value is multiplication. Multiplication is the process of determining the product of two or more numbers. The sign that represents multiplication is ×.
The first step is to determine Fiona's age.
Fiona's age = Madeline's age x 2
Fiona's age = 5 x 2 = 10
The second step is to determine Michelle's age.
Michelle's age = Fiona's age x 3
Michelle's age = 10 x 3
Michelle's age = 30
To learn more about multiplication, please check: https://brainly.com/question/13814687
#SPJ1
find the number. 1.5 of 20
Answers
Answer:
The answer is 0.3
Step-by-step explanation:
Here,
1.5 × 20/100 = 0.3
Thus, The answer is 0.3
-TheUnknownScientist 72
30
because 1.5 x 20 =30If it is 1.5% x 20 it would be = 0.3 If you add Natalie's age and Fred's age, the result is 42. If you add Fred's age to 3 times
Natalie's age, the result is 70. Write and solve a system of equations to find how old Fred and
Natalie are.
Answers
Answer:
N+F=42 & F+3N=70 System Solved: Natalie is 14 and Fred is 28
Step-by-step explanation:
Using variables N=natalies age and F=Freds age
N+F=42 ----> N=42-F (take this and plug it into the other equation)
F+3N=70 so F+3(42-F)=70 [simplify] F+126-3F=70 [further simplify]
-2F=-56 [divide -56 by -2] F=28 [then plug this number into the orig. equation]
So N+28=42 or N=14.
The study investigators conjectured that there would be an increase in weight, but that the rate of increase would level-off toward the end of the study. They also conjectured that this pattern of change may differ in the three treatment groups. Assuming an unstructured covariance matrix, construct a test of this hypothesis.
Answers
The test for this hypothesis is carried out with the help of repeated measures ANOVA.
It involves looking for an interaction effect among the treatment groups, time, and weight.
In addition, this ANOVA can determine whether the pattern of change in weight over time differs between the three treatment groups. In this case, the test can be carried out with the help of three repeated measures, and the data is grouped according to treatment. If the interaction term is significant, it indicates that the pattern of change in weight over time differs between the three treatment groups.
If the interaction term is not significant, the researchers can conclude that there is no difference in the pattern of change in weight over time between the three treatment groups.
Thus, the conclusion can be drawn from this test.
To know more about hypothesis visit:
brainly.com/question/29576929
#SPJ11
Please help I have no idea how to do this!
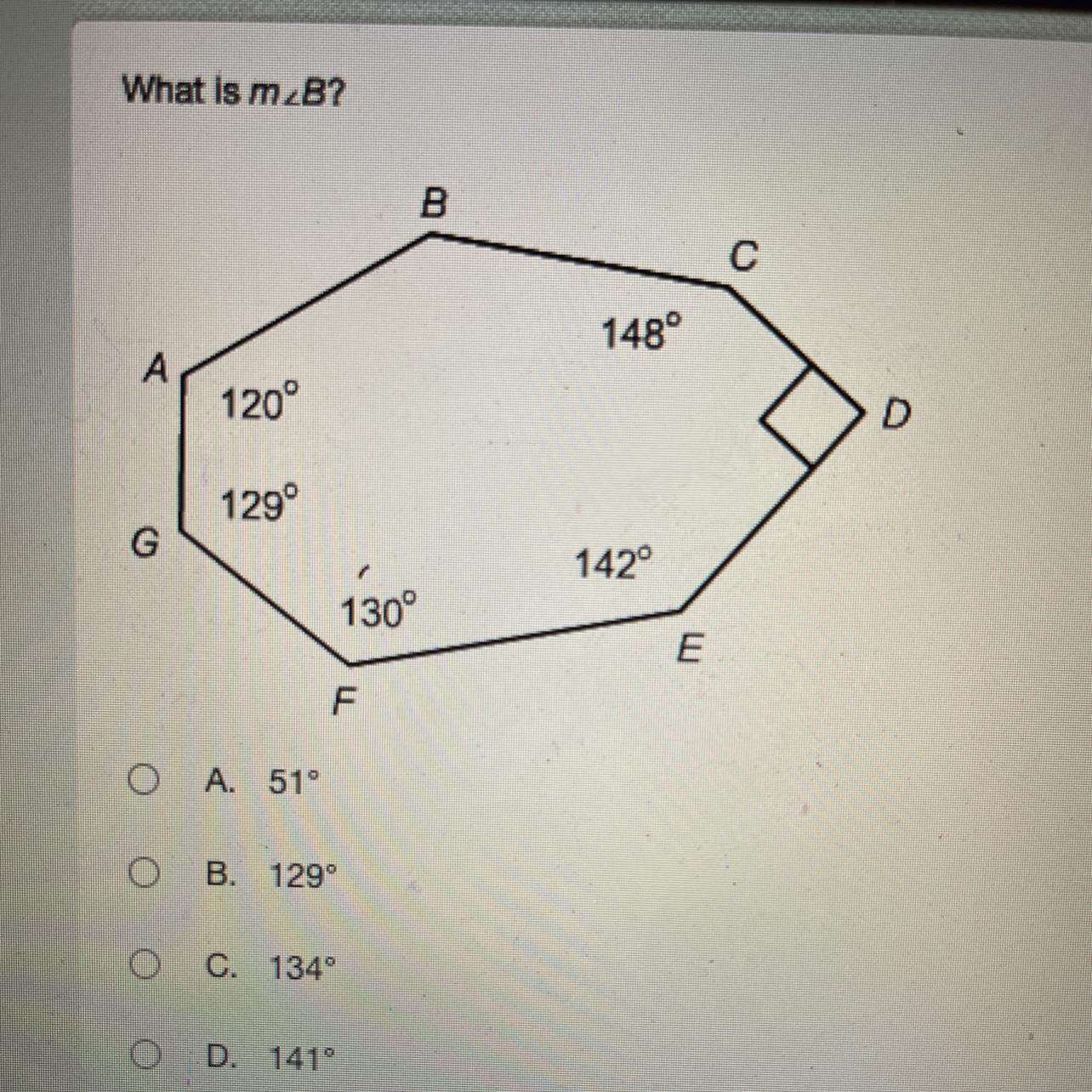
Answers
Answer:
D. <B = 141°
Step-by-step explanation:
The sum of interior angles of a polygon is
(n-2)*180, where n is the number of sides!
Sum of angles for our polygon that has 7 sides must be:
(7-2) *180 = 5*180 = 900
Sum of angles that are in the picture are:
120+129+130+142+90+148+ <B = 759 + <B
<B = 900 -759 = 141°
Check our work:
120°+129°+130°+142°+90°+148°+ 141° = 900°✅
Factor each expression that can be factored. For an expression that cannot be factored into a product of two binomials, explain why. x²+2 x+1 .
Answers
The factor of the expression will be (x + 1) and (x + 1). Then the product of two binomials will be (x + 1) and (x + 1).
What is factorization?It is a method for dividing a polynomial into pieces that will be multiplied together. At this moment, the polynomial's value will be zero.
The expression is given below.
⇒ x² + 2x + 1
Factorize the expression, then the factor of the expression will be
⇒ x² + x + x + 1
⇒ x(x + 1)x + 1(x + 1)
⇒ (x + 1)(x + 1)
⇒ (x + 1)²
The product of two binomials will be (x + 1) and (x + 1).
More about the factorization link is given below.
https://brainly.com/question/6810544
#SPJ1
For each equation, determine whether it is linear.
Equation
(a) y=x²-5
(b) y=x³
(c)y=-9x
(d) y=-x+3
Is the equation linear?
Yes
No
O

Answers
Answer:
See below.
Step-by-step explanation:
(a) y=x²-5 NO - the x term is squared.
(b) y=x³ NO - the x term is cubed
(c) y=-9x YES
(d) y=-x+3 YES
See attached graph.

Answer:
A = NO
B = NO
C = YES
D = YES
Step-by-step explanation:
A linear equation is an equation of a straight line, which means that the degree of a linear equation must be 0 or 1 for each of its variables.
For A: The degree of variable y is 1 and the degree of variable x is 2.
So it is not linear.
For C: The degree of variable y is 1 and the degree of variable x is 1.
So it is linear.
Round your answer to the nearest hundredth.

Answers
Answer:
26
Step-by-step explanation:
26

Can somebody plz help me with this question!

Answers
180-74=106-48=58/2=29=x. x=29. You would type in "29" (excluding the quotation marks) as your answer.
Let x be a binomial random variable with p = 0.1 and n = 10. calculate the following probabilities
Answers
The probabilities from the binomial probability mass function P(x ≤ 2) will be 0.9298.
What is binomial distribution?Frequency distribution of the percentage of successful outcomes that might occur in a certain number of trials with the same chance of success. If X~B(n, p), then the probability is given as
\(\rm P(X=x) = \ ^nC_x \times p^x \times (1-p)^{n - x}\)
q = 1 - p = 1 - 0.10 = 0.90
Let x be a binomial random variable with p = 0.1 and n = 10.
The probabilities from the binomial probability mass function P(x ≤ 2) will be
P(x ≤ 2) = P(x = 0) + P(x = 1) + P(x = 2)
P(x ≤ 2) = ¹⁰C₀ × (0.1)⁰ × (0.9)¹⁰ + ¹⁰C₁ × (0.1) × (0.9)⁹ + ¹⁰C₂ × (0.1)² × (0.9)⁸
P(x ≤ 2) = 0.3487 + 0.3874 + 0.1937
P(x ≤ 2) = 0.9298
Your question was incomplete but the complete question is given below.
Let x be a binomial random variable with p = 0.1 and n = 10. Calculate the probability from the binomial probability mass function less than or equal to 2.
More about the binomial distribution link is given below.
https://brainly.com/question/14565246
#SPJ1
Help Urgent!!!
I don’t understand what I should do next

Answers
9514 1404 393
Answer:
∠E = ∠A = 34°; ∠AFB = ∠DFE = 107°-34° = 73°
Step-by-step explanation:
Here's where I'd go next.
73° (∠EDA) is exterior to ΔDAC, so is the sum of remote interior angles A and C. That is, ...
∠C + ∠A = 73°
∠C = 73° -∠A = 73° -39° = 34°
Triangle CBE is given as isosceles, so its base angles C and E are congruent, both 34°.
Exterior angle CDF is the sum of remote interior angles DEF and DFE, so we have ...
107° = 34° +∠DFE
∠DFE = 107° -34° = 73°
The angle we just found (∠DFE) is a vertical angle with ∠AFB, so has the same measure.
∠AFB = 73° . . . . vertical angle with ∠DFE
_____
In summary, it looks like we have added some steps to the derivation:
4. ∠A = 34° . . . . exterior angle theorem (∠A +39° = 73°)
5. ∠E = ∠A = 34° . . . . definition of isosceles triangle
6. ∠DFE = 73° . . . . exterior angle theorem (∠E +34° = 107°)
7. ∠AFB = 73° . . . . vertical angles
_____
Additional comment
Any sort of math problem solving has you work from what you know to what you need to know by making use of previously learned relationships between the bits of information you have. Here, you really only need to know (a) angles of a linear pair total 180°; (b) angles in a triangle total 180°; (c) angles opposite congruent sides in a triangle are congruent. Everything we claim above can be concluded using these facts. The various theorems we used are simply shortcuts that allow you to bypass intermediate steps in the problem-solving process.
Circle D is shown with the measures of the minor arcs. Which angles are congruent?
A.) EDH and FDG
B.) FDE and GDH
C.) GDH and EDH
D.) GDF and HDG
Answers
The correct option is B) FDE and GDH, as their corresponding angles have the same intercepted arc and, therefore, are congruent.
To determine which angles are congruent in circle D, we need to analyze the given information about the measures of minor arcs. Since minor arcs are measured in degrees, we can use the following properties:
1. When two arcs are congruent, their corresponding central angles are also congruent.
2. The measure of a central angle is equal to the measure of its intercepted arc.
Given these properties, let's examine the answer choices:
A) EDH and FDG: We cannot determine their congruency based solely on the measures of the minor arcs.
B) FDE and GDH: These angles have the same intercepted arc, so they are congruent.
C) GDH and EDH: The intercepted arcs for these angles are different, so they are not congruent.
D) GDF and HDG: These angles have the same intercepted arc, so they are congruent.
Therefore, the correct option is B) FDE and GDH, as their corresponding angles have the same intercepted arc and, therefore, are congruent.
Learn more about congruent
https://brainly.com/question/2938476
#SPJ11
The main cable of a suspension bridge forms a parabola modeled by the equation y = a(x – H)2 + k where y is the
height in feet of the cable above the road, x is the horizontal distance in feet from the right bridge support, a is a
constant, and (h, k) is the parabola's vertex. What is the maximum and minimum height of the bridge modeled by the
equation y = 0.005(x - 60)2 + 8?
O maximum height = 100 feet and minimum height = 26 feet
O maximum height = 100 feet and minimum height = 8 feet
O maximum height = 60 feet and minimum height = 26 feet
O maximum height = 26 feet and minimum height = 8 feet
Answers
Answer:
D) maximum height = 26 feet and minimum height = 8 feet
Step-by-step explanation:
100% on edge
Answer:D on edge
Step-by-step explanation:
the guy above is right .
I need answer now please its missing now asap :D you will be marked brainliest Is she correct or wrong.

Answers
Answer:
She is wrong
Step-by-step explanation:
1/5 is equivalent to 3/15 but not 4/25
Answer:
The first fraction is correct, the second fraction is wrong. She got the first fraction equivalent by multiplying the numerator by 3.
Explain in words why there is a factor of 2 (or 1/2) in the Virial theorem. Or in other words, what if there is no factor of 2 (i.e. KE=PE) ? Comment, therefore, on a key requirement to apply the Virial Theorem to an astrophysical problem.
Answers
The factor of 2 in the Virial theorem accounts for the balance between average kinetic energy and average potential energy in a system. Without this factor, the theorem would inaccurately represent the energy equilibrium.
The Virial theorem is a fundamental principle in physics that relates the average kinetic energy (KE) of a system to its average potential energy (PE). The theorem states that in a stable system, the average kinetic energy is equal to minus one-half times the average potential energy, or KE = -1/2 PE. The factor of 2 in the theorem is crucial for this relationship to hold.
To understand the significance of this factor, let's consider a hypothetical scenario where there is no factor of 2 in the Virial theorem, meaning KE = PE. In this case, the theorem would suggest an equal balance between kinetic and potential energies. However, this assumption contradicts our understanding of typical astrophysical systems.
Astrophysical systems, such as galaxies, stars, or even gas clouds, often possess a significant amount of gravitational potential energy. If there were no factor of 2, the theorem would imply that the kinetic energy of these systems is equal to the gravitational potential energy. This would imply that the system is on the verge of collapse or expansion, which is not usually the case for stable systems.
The factor of 2 in the Virial theorem accounts for the fact that the average kinetic energy of particles in a system is typically twice as large (in magnitude) as the average potential energy. This factor arises due to the virialization process, where particles oscillate between kinetic and potential energy as they interact with each other. The factor of 2 allows for a more accurate representation of the balance between these energies in a stable system.
In conclusion, the factor of 2 in the Virial theorem is essential to accurately describe the relationship between the average kinetic and potential energies in astrophysical systems. Without this factor, the theorem would fail to capture the typical energy balance observed in stable systems.
The Virial theorem is a powerful tool used in various fields of astrophysics, including the study of galaxies, star clusters, and even the dynamics of gas in the interstellar medium. Understanding its derivation and applications can provide deeper insights into the behavior and evolution of these astronomical systems.
Additionally, exploring the concept of virialization and how it relates to the factor of 2 in the Virial theorem can shed light on the dynamical processes occurring within these astrophysical environments.
Learn more about Virial theorem
brainly.com/question/33291046
#SPJ11
A -10 nC charge is located at (x, y) = (1.2 cm , 0 cm).
What is the x-component of the electric field at the position (x, y) = (−4.1cm, 0 cm)?Express your answer to two significant figures and include the appropriate units.
Answers
We can use Coulomb's law to calculate the magnitude of the electric field at a distance r away from a point charge Q:
E = k * Q / r^2
where k is Coulomb's constant, Q is the charge of the point charge, and r is the distance from the point charge.
In this problem, we have a point charge Q of -10 nC located at (1.2 cm, 0 cm), and we want to find the x-component of the electric field at a distance r = 5.3 cm away at position (-4.1 cm, 0 cm).
To find the x-component of the electric field, we need to use the cosine of the angle between the electric field vector and the x-axis, which is cos(180°) = -1.
So, the x-component of the electric field at position (-4.1 cm, 0 cm) is:
E_x = - E * cos(180°) = - (k * Q / r^2) * (-1)
where k = 9 x 10^9 N*m^2/C^2 is Coulomb's constant.
Substituting the given values, we get:
E_x = - (9 x 10^9 N*m^2/C^2) * (-10 x 10^-9 C) / (0.053 m)^2
E_x ≈ -30,566.04 N/C
Rounding this to two significant figures and including the appropriate units, we get:
The x-component of the electric field is about -3.1 x 10^4 N/C (to the left).
The drama club is selling tickets to their play to raise money for the show's expenses.
Each student ticket sells for $7.50 and each adult ticket sells for $10. The drama club
must make no less than $1200 from ticket sales to cover the show's costs. Write an
inequality that could represent the possible values for the number of student tickets
sold, s, and the number of adult tickets sold, a, that would satisfy the constraint.
Answers
The total revenue from adult ticket sales (10a) must be greater than or equal to $1200.
Let's represent the number of student tickets sold as 's' and the number of adult tickets sold as 'a'.
The revenue from student ticket sales can be calculated as 7.50s, and the revenue from adult ticket sales can be calculated as 10a.
To satisfy the constraint that the drama club must make no less than $1200, we can write the following inequality:
7.50s + 10a ≥ 1200
This inequality states that the total revenue from student ticket sales (7.50s) plus the total revenue from adult ticket sales (10a) must be greater than or equal to $1200.
This inequality ensures that the ticket sales generate enough revenue to cover the show's costs. The drama club needs to sell enough tickets, both student and adult, to meet or exceed the minimum revenue requirement of $1200.
For more such answers on revenue
https://brainly.com/question/30760779
#SPJ8
In a chart, each data point, bar, slice, and so on has a unique ________.
a) Color
b) Label
c) Size
d) Position
Answers
The correct option is b) Label.
In a chart, each data point, bar, slice, and so on has a unique label. This label is used to identify and differentiate the different elements within the chart. It provides a clear and concise description of what each element represents.
For example, in a bar chart that shows the sales of different products, each bar would have a label indicating the specific product it represents. This allows the viewer to easily understand which product corresponds to each bar.
The label is crucial in making the chart understandable and meaningful. Without it, the chart would be confusing and difficult to interpret. It helps to provide context and clarity to the data being presented.
While color, size, and position can also be used to distinguish between elements in a chart, they do not provide the same level of specificity as a label. Color, for instance, may be used to represent different categories, but it does not provide specific information about each individual element.
In summary, the unique label assigned to each data point, bar, slice, or other element in a chart is what helps identify and differentiate them. It provides a clear description of what each element represents, making the chart more understandable and meaningful.
For more such question on charts.
brainly.com/question/29608290
#SPJ11
What inequality is graphed below?

Answers
The ratio of seventh grade students to eighth grade students in the school band is 3 to 4. There are 36 seventh grade students in the school band. What is the total number of students in the school band
Answers
Answer:
84
Step-by-step explanation:
Let A be the number of students in grade 7
Let B be the number of students in grade 8
we have \(\frac{A}{B} =\frac{3}{4}=\frac{36}{B}\)
===> B (number of students in grade 8) = 4x36/3=48
so there are 48+36= 84 students in school band
Which of the following transformations will not produce a congruent image?
A.
rotation
B.
translation
C.
dilation
D.
reflection
Answers
Answer:
Translation, rotation and reflection
Step-by-step explanation:
please brainliest <3
please help i need qn1!!

Answers
Answer:
1a) \(m = \frac{60}{ \sqrt{31 - 2n} } \)
b) 8.47 (3 s.f.)
Step-by-step explanation:
If y is inversely proportional to x, then \(y = \frac{k}{x} \), where k is a constant.
Since m is inversely proportional to \( \sqrt{31 - 2n} \),
\(m = \frac{k}{ \sqrt{31 - 2n} } \), where k is a constant.
When n=3,
\(m = \frac{k}{ \sqrt{31 - 2(3)} } \\ m = \frac{k}{ \sqrt{31 - 6} } \\ m = \frac{k}{ \sqrt{25} } \\ m = \frac{k}{5} \)
when n=11,
\(m = \frac{k}{ \sqrt{31 - 2(11)} } \\ m = \frac{k}{ \sqrt{31 - 22} } \\ m = \frac{k}{ \sqrt{9} } \\ m = \frac{k}{3} \)
Since the sum of the values of m when n=3 and n=11 is 32,
\( \frac{k}{3} + \frac{k}{5} = 32 \\ \frac{5k}{15} + \frac{3k}{15} = 32 \\ \frac{8k}{15} = 32 \\ 8k = 32(15) \\ 8k = 480 \\ k = 60\)
a) \(m = \frac{60}{ \sqrt{31 - 2n} } \)
b) When m=16,
\(16 = \frac{60}{ \sqrt{31 - 2n} } \\ 16( \sqrt{31 - 2n} ) = 60 \\ \sqrt{31 - 2n } = 60 \div 16 \\ \sqrt{31 - 2n} = 3.75 \\ 31 - 2n = 3.75^{2} \\ 31 - 14.0625 = 2n \\ 2n = 16.9375 \\ n = 8.47 \: (3 \: s.f.)\)
a circle has a diameter with endpoints at (-2, 87) and (18, 91). what is the length of the diameter?
Answers
The length of the diameter of a circle with endpoints at points (-2, 87) and (18, 91) is 20.4 units
How to find length of lineThe length of line in an ordered pair is calculated using the formula
d = √{(x₂ - x₁)² + (y₂ - y₁)²}
where
d = distance between the points
x₂ and x₁ = points in x coordinates
y₂ and y₁ = points in y coordinates
distance between points (-2, 87) and (18, 91) is calculated as follows
d = √{(x₂ - x₁)² + (y₂ - y₁)²}
d =√{(-2 - 18)² + (87 - 91)²}
d =√{400 + 16}
d = √416
d = 20.4 units
The diameter has length 20.4 units
Learn more about length of line segment here:
https://brainly.com/question/29706244
#SPJ1
Are polynomials closed under addition and subtraction?
Answers
Polynomials form a system like to that of integers hence they are closed under the operations of addition and subtraction.
Exponents of polynomials are whole numbers.
Hence the resultant exponents will be whole numbers , addition is closed for whole numbers. As a result, polynomials are closed under addition.
If an operation results in the production of another polynomial, the resulting polynomials will be closed.
The outcome of subtracting two polynomials is a polynomial. They are also closed under subtraction as a result.
The word polynomial is a Greek word. We can refer to a polynomial as having many terms because poly means many and nominal means terms. This article will teach us about polynomial expressions, polynomial types, polynomial degrees, and polynomial properties.
To learn more about polynomials
brainly.com/question/27287760
#SPJ4
the allowable is one metric used to determine what conversion rate you need to break even. True or False
Answers
The allowable is a term used in advertising and marketing to refer to the maximum cost per acquisition (CPA) that a business can afford to pay. So, the given statement is False.
"The allowable" is a term used in advertising and marketing to refer to the maximum cost per acquisition (CPA) that a business can afford to pay to acquire a customer while still remaining profitable. It is not directly related to the conversion rate needed to break even.
To determine the conversion rate needed to break even, you would need to consider the cost of acquiring a customer, the profit margin on the product or service being sold, and the total revenue generated by each customer. This calculation can help determine the minimum conversion rate needed to achieve profitability.
Know more about allowable here:
https://brainly.com/question/29646168
#SPJ11