Answers
Answer:
Well what's half of 55?
Step-by-step explanation:
just find what 2 number make 55. Well half of 55 if 27.5 so then you put that into your chart and that should help you move along the rest! Hope this helps!
Related Questions
Is y=-3x^2+10 a function? Justification?
Answers
Ira walked 20 miles last week and 22 miles this week. raj walked 1/3 the distance that ira walked. how much did raj walk?
Answers
Raj walked 14 miles. Since one-third of the combined distance walked by Ira is 14 miles,
Ira walked 20 miles last week and 22 miles this week. To find out how much Raj walked, we need to calculate one-third of the distance Ira walked.
One-third of the distance Ira walked is found by multiplying the total distance by 1/3:
Distance walked by Raj = (1/3) * (20 + 22)
= (1/3) * 42
= 14
Therefore, Raj walked 14 miles. Since one-third of the combined distance walked by Ira is 14 miles, it indicates that Raj walked a shorter distance compared to Ira. This calculation provides the answer for how much Raj walked .
To learn more about distance click here:
brainly.com/question/30290364
#SPJ11
What is the equation of the line that is perpendicular to the line y=3/4x - 6 and passes through the point (-3,5)?
Answers
Answer:
\(y=-\frac{4}{3}x+1\)
Step-by-step explanation:
Hi there!
What we need to know:
Linear equations are typically organized in slope-intercept form: \(y=mx+b\) where m is the slope and b is the y-intercept (the value of y when the line crosses the y-axis)Perpendicular lines always have slopes that are negative reciprocals of each other (ex. -1/3 and 3, 1/2 and -2, etc.)1) Determine the slope (m)
\(y=\frac{3}{4} x - 6\)
Looking at the given equation, we can identify clearly that \(\frac{3}{4}\) is in the place of m, making it the slope of the line. Because perpendicular lines always have slopes that are negative reciprocals, we know that the slope of the line we're solving for will be \(-\frac{4}{3}\). Plug this into \(y=mx+b\):
\(y=-\frac{4}{3}x+b\)
2) Determine the y-intercept (b)
\(y=-\frac{4}{3}x+b\)
Plug in the given point (-3,5)
\(5=-\frac{4}{3}(-3)+b\\5=4+b\)
Subtract 4 from both sides
\(5-4=4+b-4\\1=b\)
Therefore, the y-intercept is 1. Plug this back into \(y=-\frac{4}{3}x+b\):
\(y=-\frac{4}{3}x+1\)
I hope this helps!
Kedwin has $150.00 and wants to buy a new pair of headphones that cost $175.00 plus 10% shipping. He decides to
wait until the headphones go on sale. What is the smallest sale rate that he needs to be able to afford them?
A.10%
B.20%
C.25%
D.30%
PLZZZ ANSWER I have 30 minutes til the end of term and have 40 questions left :[
Answers
Answer:
I believe its 20% thats counting the 10% shipping cost
Step-by-step explanation:
Hope it helps
Hii can someone who is really good at math please help me with these 2 math questions. I'm struggling with them!!


Answers
For an infinitesimal material volume δV=δx1δx2δx3, show that the rate of volume change per unit volume is equal to the first invariant of the strain rate tensor, i.e., 1/δVD(δV)/Dt=Sii
Answers
The rate of volume change per unit volume is equal to the first invariant of the strain rate tensor, i.e., 1/δVD(δV)/Dt=Sii.
Given δV = δx1 δx2 δx3 which represents an infinitesimal material volume.
To prove that the rate of volume change per unit volume is equal to the first invariant of the strain rate tensor, we need to use the definition of the rate of volume change.
δV is the infinitesimal material volume, the rate of change in the volume per unit volume is defined as:
1/δV D(δV)/Dt
Now, we use the strain rate tensor to derive the same quantity.
The rate of deformation tensor Dij is defined as the time derivative of the strain tensor εij, thus:
Dij=∂vi/∂xj
The trace of the rate of deformation tensor is called the divergence of the velocity field and is given as Dii=∂vi/∂xi
The divergence of the velocity field is a measure of the expansion or contraction of the fluid.
The incompressible fluids have zero divergence i.e.
δVD/Dt=0
The rate of volume change per unit volume is equal to the first invariant of the strain rate tensor, i.e., 1/δVD(δV)/Dt=Sii.
To know more about strain rate tensor visit:
https://brainly.com/question/33394539
#SPJ11
5/9 is 11% of what number?
write the proportion
Answers
Answer:
ratios, fractions, statistics, and percentage increase or decrease.
Step-by-step explanation:
Answer:
The answer is 5.05
Step-by-step explanation:
Let the number be x,
5/9 = 0.56
Now,
x × 11/100 = 5/9
x = 5 × 100 ÷ 9 × 11
x = 500/99
x = 5.05
Thus, The number is 5.05
-TheUnknownScientist 72
A
B
Value: 10
AB=
12√2
BC=
45°
Find the missing side lengths. (Enter your answer
without units.)
C
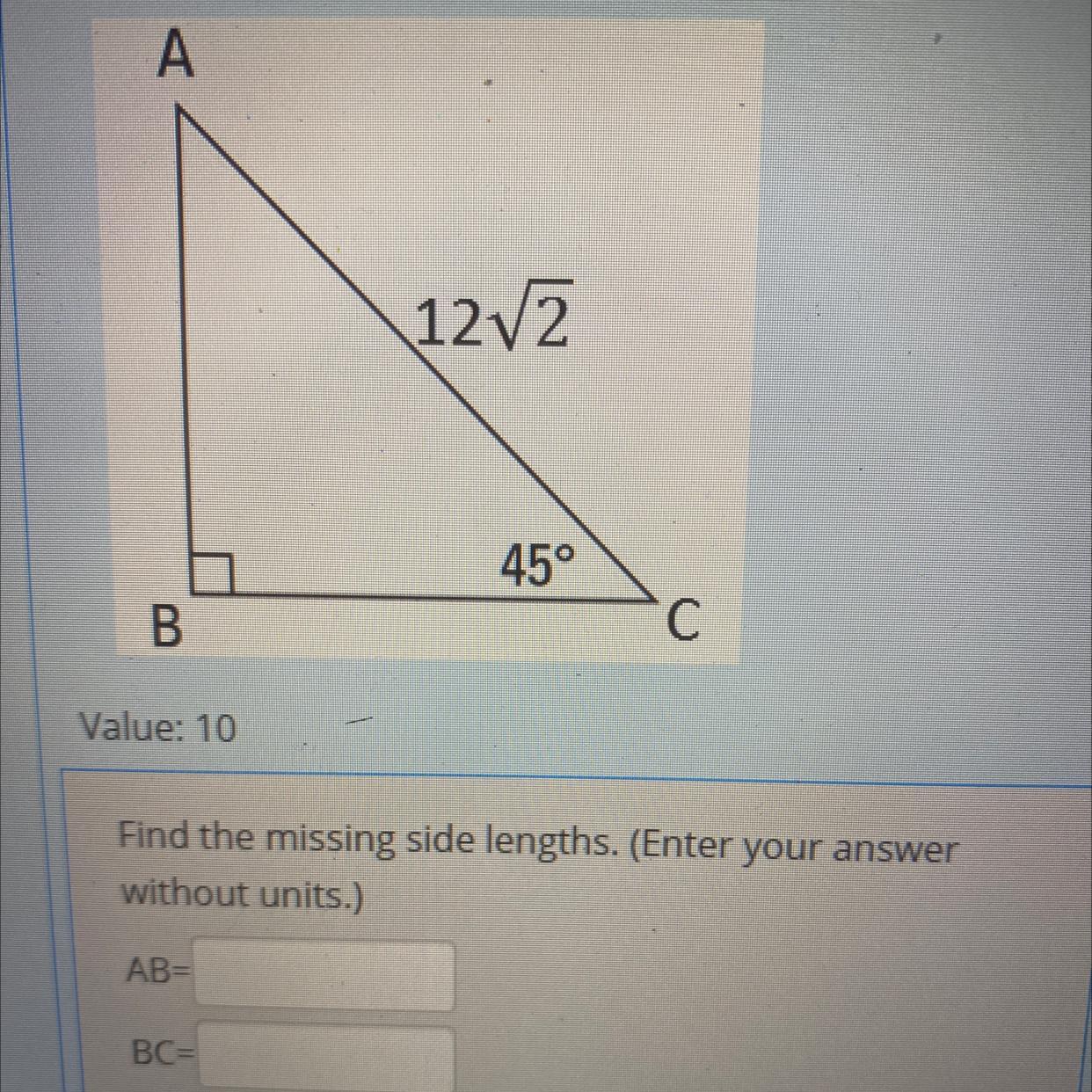
Answers
Answer:
12
Step-by-step explanation:
This is a special triangle with angle measure 45-45-90
The side lengths for this special right triangle is as follows:
The side length that sees angle measure 90 is represented by a\(\sqrt{2}\)
The side lengths that see angle measures 45 is represented by a
We can see from the image hypotenuse (the side that sees angle 90)
is given as 12\(\sqrt{2}\) that means a = 12
So the missing side lengths (both see angle measure 45) = 12
what is the measurement of SRT
A: 101 degrees
B: 79 degrees
C: 99 degrees
D: 69 degrees

Answers
Answer:
79°
Step-by-step explanation:
Remember, the sum of all the angles in a triangle is 180
So 180-RST-RTS=180-53-48=79
what is 5+10/5 i cant find the answer
Answers
we'll divide 10 by 5..the answer is 2 then we'll add it to the other 5 making it to be 7
Answer: 7
How to: To add fractions, find the LCD and then combine.
If the mean of the four numbers 2,4,x,and 6 is 5,then x is
Answers
The value of the unknown number x is 8.
What is the mean?Mean is the average of the given numbers and is calculated by dividing the sum of given numbers by the total number of numbers.
The given numbers include:
2, 4, x, 6mean = 5The sum of the given numbers is calculated as follows:
\(2 + 4 + \text{x} + 6 = 12 + \text{x}\)
The mean of the given 4 numbers is calculated as follows:
\(\dfrac{12+\text{x}}{4} =5\)
\(12+\text{x}=20\)
\(\text{x}=20-12\)
\(\text{x}=8\)
Thus, the value of the unknown number x is 8.
Learn more about the mean of numbers at: https://brainly.com/question/32305540
a root of the equation 3x-8=13
Answers
Answer:
x= 7
Step-by-step explanation:
3x= 13+8
3x= 21
x= 21/3
x= 7
Use C++ to multiply two square matrices of random integers between 1 and 10. The size of the matrices is 250, 500, 1000 etc elements, the since may later change. In other words, multiply two 250x250 matrices or two 500x500 matrices of random integers. DO NOT use any external libraries, instead use a nested loops.
Answers
To multiply two square matrices of random integers between 1 and 10 of different sizes, i.e., 250 x 250, 500 x 500, and 1000 x 1000, we can use nested loops and the C++ language.
Here is the solution:
#include
using namespace std;
int main(){
int m1[1001][1001], m2[1001][1001], m3[1001][1001];
int n; // size of matrix
cout << "Enter the size of matrix: ";
cin >> n;//filling matrix m1 and m2 with random integer values between 1 and 10
for(int i=1;i<=n;i++)
{for(int j=1;j<=n;j++){m1[i][j] = rand() % 10 + 1; //random value between 1 and 10
m2[i][j] = rand() % 10 + 1;}
} //multiplying two matrices
for(int i=1;i<=n;i++){
for(int j=1;j<=n;j++){
m3[i][j] = 0;
for(int k=1;k<=n;k++){
m3[i][j] += m1[i][k] * m2[k][j];
}
}
}
return 0;
}
Explanation: The first thing you have to do is to declare three matrices using the integer data type. These are m1, m2, and m3. m1 and m2 matrices are used to store the random integers between 1 and 10, and m3 is used to store the result of the multiplication of the two matrices. Next, you have to declare the size of the matrix using the integer data type and input it using the cin function. Then, using a nested loop, you can fill in the matrices m1 and m2 with random integers between 1 and 10 using the rand() function. To multiply two matrices using nested loops, the first outer loop iterates through the rows of the first matrix m1. The second outer loop iterates through the columns of the second matrix m2. The inner loop is used to compute the dot product of the row and column from the two matrices. The resulting value is stored in the matrix m3 using the same row and column as the corresponding values in the two matrices m1 and m2.
Learn more about matrices:
https://brainly.com/question/14375349
#SPJ11
A manufacturer of a traditional medicine claims that the medicine is 90% effective in relieving backache for a period of eight hours. In a sample of 200 people who have backache, the medicine provided relief for 160 people. Test the manufacturer's claim at 1% significance level
Answers
The critical value of 2.576. If |z| > 2.576, reject the null hypothesis; otherwise, fail to reject the null hypothesis.
To test the manufacturer's claim at a 1% significance level, we need to perform a hypothesis test. Let's define the null and alternative hypotheses:
Null hypothesis (H₀): The medicine is 90% effective in relieving backache.
H₀: p = 0.9
Alternative hypothesis (H₁): The medicine is not 90% effective in relieving backache.
H₁: p ≠ 0.9
Where p represents the true proportion of people who experience relief from backache after taking the medicine.
To conduct the hypothesis test, we will use the sample proportion and perform a z-test.
Calculate the sample proportion:
p = x/n
where x is the number of people who experienced relief (160) and n is the sample size (200).
p= 160/200 = 0.8
Calculate the standard error:
SE = √(p(1 - p)/n)
SE = √((0.8 * (1 - 0.8))/200)
Calculate the test statistic (z-score):
z = (p - p₀) / SE
where p₀ is the hypothesized proportion (0.9 in this case).
z = (0.8 - 0.9) / SE
Determine the critical value for a two-tailed test at a 1% significance level.
Since we have a two-tailed test at a 1% significance level, the critical value will be z* = ±2.576 (obtained from a standard normal distribution table or calculator).
Compare the absolute value of the test statistic to the critical value to make a decision:
If the absolute value of the test statistic is greater than the critical value (|z| > z*), we reject the null hypothesis.
If the absolute value of the test statistic is less than or equal to the critical value (|z| ≤ z*), we fail to reject the null hypothesis.
Substituting the values into the equation, we can determine the test statistic and compare it to the critical value.
For more about critical value:
https://brainly.com/question/32389590
#SPJ4
1.
Madison's monthly salary is $2,810. If Madison's budget for rent is 26%
of salary, thep how much is budgeted for rent?
Answers
Answer:
Step-by-step explanation:
Madison's rent = 26% of 2810
= 0.26 * 2810
= $ 730.60
26/100=0.26
0.26*2810=730.6 dollars
Please help me with this and explain

Answers
Answer:
4.104
Step-by-step explanation:
Volume of any prism = Area of cross section x height.
so, volume = 0.6 x 1.9 x 3.6 = 4.104
why is it possible for condition means to differ at the end of an experiment even if the independent variable had no effect? type ii error has ocurrederror variancerandomizationa null finding has been obtained
Answers
Answer:
Answer:- 1) option b) error variance.Have a Nice Best Day : ) Please Give Me Brainliest
HELP WHICH EXPRESSION

Answers
(n^-3)^6
Explanation:
(n^-3)^6
=n^-18
We can use the law that a^-n=1/a^n to help us simplify n^-18.
n^-18
=1/n^18
I hope this helps! Please comment if you have any questions.
Answer:
\((n^{-3}) ^{6}\)
Step-by-step explanation:
Using the rules of exponents
\((a^{m}) ^{n}\) ⇔ \(a^{mn}\)
\(a^{-m}\) ⇔ \(\frac{1}{a^{m} }\)
Consider
\((n^{-3}) ^{6}\)
= \(n^{-3(6)}\)
= \(n^{-18}\)
= \(\frac{1}{n^{18} }\)
The other 3 all simplify to \(n^{18}\)
Recall that in a 30 – 60 – 90 triangle, if the shortest leg measures x units, then the longer leg measures xStartRoot 3 EndRoot units and the hypotenuse measures 2x units. (150StartRoot 3 EndRoot – 75π) ft2 (300 – 75π) ft2 (150StartRoot 3 EndRoot – 25π) ft2 (300 – 25π) ft2.
Answers
The area of the shaded region is \(\rm (150\sqrt{3} \ - 75\pi ) \ feet^2\) option first is correct.
It is given that a circle is inscribed in a regular hexagon with sides of 10 feet.
It is required to find the shaded area (missing data is attached shown in the picture).
What is a circle?It is defined as the combination of points that and every point has an equal distance from a fixed point ( called the center of a circle).
We have a hexagon with a side length of 10 feet.
We know the area of the hexagon is given by:
\(\rm A = \frac{3\sqrt{3} }{2} a^2\) where a is the side length.
\(\rm A = \frac{3\sqrt{3} }{2} 10^2\) ⇒ \(150\sqrt{3}\) \(\rm feet^2\)
We have the shortest length = x feet and from the figure:
2x = 10
x = 5 feet
The radius of the circle r = longer leg
\(\rm r = x\sqrt{3} \Rightarrow 5\sqrt{3}\) feet
The area of the circle a = \(\pi r^2\) ⇒ \(\pi (5\sqrt{3} )^2 \Rightarrow 75\pi \ \rm feet^2\)
The area of the shaded region = A - a
\(\rm =(150\sqrt{3} \ - 75\pi ) \ feet^2\)
Thus, the area of the shaded region is \(\rm (150\sqrt{3} \ - 75\pi ) \ feet^2\)
Learn more about circle here:
brainly.com/question/11833983

Answer:
option A
Step-by-step explanation:
distribute expression -(5-b)
Answers
Answer:
-5+b or b-5
Step-by-step explanation:
Tell whether the angles are complementary or supplementary. Then find the value of x.

Answers
Answer:
The angles are supplementary.
x = 31
Step-by-step explanation:
The way the two angles lay together and make a straight line shows that they are supplementary. Supplementary means that they add up to 180°
Use this idea to write an equation.
2x + 3x + 25 = 180
combine like terms
5x + 25 = 180
subtract 25
5x = 155
divide by 5
x = 31
Answer:
x = 31
Step-by-step explanation:
(3x + 25) and 2x are a linear pair and are supplementary, that is
3x + 25 + 2x = 180
5x + 25 = 180 ( subtract 25 from both sides )
5x = 155 ( divide both sides by 5 )
x = 31
Leo wanted to surprise his brother on New Years Eve, by filling his Lightsaber full of confetti for their big dual. He needs to know how many bags to buy.


Answers
Given:
The lightsaber is filled with full of confetti.
The lightsaber:
\(\begin{gathered} h=30in \\ d=2.5in \end{gathered}\)The confetti bag is 10oz.
To find:
The number of bags of confetti.
Explanation:
The radius of the lightsaber is,
\(r=\frac{d}{2}=\frac{2.5}{2}=1.25in\)The volume of the lightsaber is,
\(\begin{gathered} V=\pi r^2h \\ =\frac{22}{7}\times1.25^2\times30 \\ V=147.32in^3 \end{gathered}\)In oz,
\(\begin{gathered} V=\frac{147.32}{1.804} \\ V=81.66oz \end{gathered}\)So, the number of bags will be,
\(\begin{gathered} n=\frac{Volume\text{ }of\text{ }lightsaber}{Volume\text{ of a bag}} \\ =\frac{81.66}{10} \\ =8.166 \\ \approx8bags \end{gathered}\)The number of bags required is 8.
Final answer:
The volume of the lightsaber is 81.66 ounces.
The number of bags required is 8.
12 identically sized pieces of timber are
x
cm long when put together. How long is each individual piece?
Answers
Answer:
\(\frac{x}{12}\) (x/12)
Step-by-step explanation:
if 12 pieces combine to make x, [we know they are identical], each piece must be 1/12 of x [1/12·x = x/12]
(think of it like this: "x" length must be divided amongst 12 pieces, so, we divide x/ 12)
Answer:
Timber=x/12
Step-by-step explanation:
12 pieces long is x, if the length of each one is same, so the length of each one is x/12.
approximately enter your response here% of women in this group have platelet counts between and . (type an integer or a decimal. do not round.)
Answers
The approximate percentage of women in this group with platelet counts between 71.3 and 443.9 is approximately 95% .
To find the approximate percentages using the empirical rule, we can refer to the standard deviations from the mean.
The range within 1 standard deviation of the mean includes approximately 68% of the data in a bell-shaped distribution. In this case, the mean is 257.62, and the standard deviation is 62.1. Therefore, the approximate percentage of women with platelet counts within 1 standard deviation of the mean, or between 195.5 and 319.7, is also approximately 68%.
To find the approximate percentage of women with platelet counts between 71.3 and 443.9, we need to determine the number of standard deviations away from the mean these values are.
For the lower value of 71.3:
Standard deviations below the mean = (71.3 - 257.62) / 62.1 ≈ -2.99
According to the empirical rule, the percentage below 2 standard deviations is approximately 2.5%.
For the upper value of 443.9:
Standard deviations above the mean = (443.9 - 257.62) / 62.1 ≈ 2.99
According to the empirical rule, the percentage above 2 standard deviations is also approximately 2.5%.
Since the values of 71.3 and 443.9 fall outside of the range within 2 standard deviations from the mean, the approximate percentage of women with platelet counts between 71.3 and 443.9 is approximately 100% - (2.5% + 2.5%) = 95%.
Therefore, the approximate percentage of women in this group with platelet counts between 71.3 and 443.9 is approximately 95%.
To know more about percentage click here :
https://brainly.com/question/29079019
#SPJ4
The question is incomplete the complete question is :
The blood platelet counts of a group of women have a bell-shaped distribution with a mean of 257.62 and a standard deviation of 62.1 (All units are 1000 cells/muμ L.)
Using the empirical rule, find each approximate percentage below.
What is the approximate percentage of women with platelet counts within 1 standard deviation of the mean, or between 195.5 and 319.7 ?
What is the approximate percentage of women with platelet counts between 71.3 and 443.9 ?
a. Approximately 68 % of women in this group have platelet counts within 1 standard deviation of the mean, or between 195.5 and 319.7.
(Type an integer or a decimal. Do not round.)
Approximately ____ % of women in this group have platelet counts between 71.3 and 443.9.
(Type an integer or a decimal. Do not round.)
at 95% confidence, what is the margin of error? if required, round your answer to four decimal places. g
Answers
At 95% confidence interval for a sample of size 100 where p = 0.15 , the margin of error is 0.0700 .
In the question ,
it is given that ,
the sample size is = 100 and
the value of p is ⇒ 0.15 .
we have to find the "margin of error"(E) for the 95% confidence interval ,
we know that the z value for the 95% confidence interval is = 1.96
the formula for margin of error is :
E = Z×√p(1-p)/n .
Substituting the values of z , p and n ,
we get ,
E = 1.96×√0.15(1-0.15)/100
= 1.96×√0.15(0.85)/100
= 1.96×√0.1275/100
= 1.96×√0.001275
= 1.96×0.03570
Simplifying further ,
we get ,
= 0.06995899
rounding to the four decimal places ,
≈ 0.0700
Therefore , the required margin of error is 0.0700 .
The given question is incomplete , the complete question is
At 95% confidence, for a sample of size 100 where p = 0.15 . What is the Margin Of Error ? if required, round your answer to four decimal places.
Learn more about Margin Of Error here
https://brainly.com/question/29110508
#SPJ4
Ashok and Brian are both walking east along the same path; Ashok walks at a faster constant speed than does Brian. If Brian starts 30 miles east of Ashok and both begin walking at the same time, how many miles will Brian walk before Ashok catches up with him
Answers
Answer:
60 miles
Step-by-step explanation:
Ashok and Brian are both walking east along the same path; Ashok walks at a faster constant speed than does Brian. If Brian starts 30 miles east of Ashok and both begin walking at the same time, how many miles will Brian walk before Ashok catches up with him?
Statement 1. Brian’s walking speed is twice the difference between Ashok’s walking speed and his own
Statement 2. If Ashok’s walking speed were five times as great, it would be three times the sum of his and Brian’s actual walking speeds
Solution
A. Brian’s walking speed is twice the difference between Ashok’s walking speed and his own.
Let Brian speed=b
Ashok speed=a
Brian's walking speed=2(a-b)
b=2(a-b)
Divide both sides by 2
b/2=a-b
Ashok catches up in (time)= distance /( relative rate
=30/(a-b)
=30/(b/2)
=30÷b/2
=30*2/b
=60/b.
By that time Brian will cover a distance of
distance=rate*time
=b*60/b
=2(a-b)*60/2(a-b)
=60 miles
(2) If Ashok’s walking speed were five times as great, it would be three times the sum of his and Brian’s actual walking speeds.
5a=3(a+b)
5a=3a+3b
5a-3a=3b
2a=3b
Solve for r.
4r+10>6
71 points
Help Plslsspsl
Answers
Answer:
r > -1
Step-by-step explanation:
4r + 10 > 6
4r + 4 > 0
4 > -4r
-4 < 4r
-1 < r
r > -1
Answer:
r > - 1 or r ∈ (- 1, + ∞)Step-by-step explanation:
4r + 10 > 64r > 6 - 104r > - 4r > - 4/4r > - 1 or r ∈ (- 1, + ∞)why can't you just use the sample mean to estimate the population mean without including a margin of error?
Answers
It is not advisable to use the sample mean as an estimate of the population mean without including a margin of error.
When estimating a population parameter, such as the population mean, using a sample, it is essential to consider the uncertainty or variability in the sample estimate. This uncertainty is captured by the margin of error.
The sample mean provides an estimate of the population mean based on the available sample data. However, it is subject to sampling variability, meaning that different samples from the same population may yield different sample means. This variability arises due to the inherent randomness in the sampling process.
By including a margin of error, we acknowledge and quantify this sampling variability. The margin of error provides a range within which the true population mean is likely to lie. It accounts for the uncertainty associated with estimating the population parameter based on a finite sample.
Ignoring the margin of error means disregarding the inherent variability in the sample mean and assuming that it perfectly represents the true population mean. This assumption is generally not valid and can lead to inaccurate or misleading conclusions about the population.
By including a margin of error, we convey the level of confidence or precision associated with our estimate and provide a more realistic assessment of the population mean. This helps in making informed decisions or drawing valid statistical inferences based on the sample data.
Visit here to learn more about the margin of error:
brainly.com/question/29419047
#SPJ11
The volume of a rectangular prism is given by the expression10x3 + 46x2 – 21x – 27. The area of the base of the prism is given by the expression 2x2 + 8x – 9. Which of the following expressions represents the height of the prism? (V = Bh)
8x - 3
3x - 5
5x + 3
42x + 3
Answers
The height of the prism is 5x + 3 units.
What is volume?
A measurement of three-dimensional space is volume. It is frequently expressed numerically using SI-derived units, as well as different imperial or US-standard units. Volume and the definition of length are related.
Given:
The volume of a rectangular prism is given by the expression
10x^3 + 46x^2 – 21x – 27. The area of the base of the prism is given by the expression 2x^2 + 8x – 9.
We have to find the height of prism.
Volume of the rectangular prism = Base × Height
The expression is in the Question be
10x ³ + 46 x² - 21x -27
And the area of the base of the prism is given by the expression
2x² + 8x - 9 .
Put in the formula
10x ³ + 46 x² - 21x -27 = 2x² + 8x - 9 × Height
The factor of 10x ³ + 46 x² - 21x -27 are (5x +3 )(2x² + 8x - 9) .
put in the formula
(5x +3 )(2x² + 8x - 9) = (2x² + 8x - 9) × Height
Cancelled 2x² + 8x - 9 on both side.
(5x+3)unit = Height
Hence, the height of the prism is 5x + 3 units.
To know more about volume, click on the link
https://brainly.com/question/2887044
#SPJ1
In order for the data in the table to represent a linear function with a rate of change of –8, what must be the value of a?
Answers
Given:
Consider the below figure attached with this question.
The rate of change of the linear function is -8.
To find:
The value of a.
Solution:
If a linear function passes through the points \((x_1,y_1)\) and \((x_2,y_2)\), then the rate of change of the linear function is:
\(m=\dfrac{y_2-y_1}{x_2-x_1}\)
From the given table, it is clear that the linear function passes through the points (10,27) and (11,a). So, the slope of the linear function is:
\(m=\dfrac{a-27}{11-10}\)
\(m=\dfrac{a-27}{1}\)
\(m=a-27\)
The rate of change of the linear function is -8.
\(-8=a-27\)
\(-8+27=a\)
\(19=a\)
Therefore, the value of a is 19.

Answer:
it is C
Step-by-step explanation:
