Given f of x is equal to the quantity 8x plus 1 end quantity divided by the quantity 2x minus 9 end quantity, what is the end behavior of the function?
Answers
Answer:
Step-by-step explanation:
Equation
f(x) = (8x + 1) / (2x + 9)
Notice the brackets. They are necessary to show what comes before the division sign, and what comes after.
Behavior
The best way to state the behavior is to get something like a graphing program to show you what the curve looks like -- where it's critical points are for example. I use Desmos for this kind of question, Just do a search for Desmos and bookmark it. You will use it quite often.
So you want to know the behavior.
Right Curve
Crosses the x axis at (-0.125, 0)
Crosses the y axis at (0,0,111)
The right curve has a domain of -∞ < x < ∞
The right curve has a range of -∞ < x < ∞
The range is going to have to go out quite a ways before you see any dramatic decrease in the way it is drawn.
Left Curve.
The right hand curve never crosses either the x or y axis. Nor does it just touch the x or y axis.
The domain is -∞ <x < 0
The range is 0<x<∞

Related Questions
If you have a jumbo pack of gum and 4 friends ask for 8 How much gum do you have left?
(random questions)
Answers
Answer:
5
Step-by-step explanation:
37 - 4(8)
37 - 32
5
Hope this helps! May I please have brainliest? :)
Answer:
solution given:
total pack of gum=x gums
If 4 friends ask for 8=8×4gum= 32gums
Remaining gums=x-32gums
What is the difference between Glasgow and Copenhagen. (Glasgow= -7.8 Copenhagen=-3.8)
Answers
Answer:
Step-by-step explanation:
The difference of the two values is 4 as calculated by subtraction.
An arithmetic operation called subtraction is the process of deleting or removing items from a collection. The negative symbol (-) denotes subtraction.
Subtraction can mean eliminating or lowering physical and abstract quantities utilizing a variety of things, such as fractions, matrices, vectors, irrational numbers, decimals, functions, and however negative integers. it is typically linked with natural numbers in mathematics.There are several significant patterns in subtraction. It is anticommutative, which means that altering the sequence alters the answer's sign. Additionally, it is not associative, which means that when subtracting more than two numbers, the method used matters. 0 is the additive identity, hence subtracting it has no effect on a number. In relation to related operations like addition and multiplication, subtraction also abides by predictable principles.The given values for Glasgow and Copenhagen are given as -7.8 and -3.8 .
Therefore difference = -3.8 - (-7.8) = -3.8 +7.8 = 4
To learn more about subtraction visit:
https://brainly.com/question/2346316
#SPJ1
Can someone explain this more and help me

Answers
Answer:
-4
x^2
3
Step-by-step explanation:
just find the equation by forming the equation for each section for the given interval
first part is f(x)=4 since it is always 4
second part is f(x)=x^2 since it is a standard quadratic equation
third part is f(x)=3 since it is always 3
What is The distance between The points(-6,7) and(-1,1) round to the nearest whole unit
Answers
Answer:
8
Step-by-step explanation:
using the distance formula
I need help with my homework please help

Answers
The formulas for the rigid transformations of the figures are listed below:
Case 1: (x, y) → (x - 10, y + 9)
Case 2: (x, y) → (- y, x)
Case 3: (x, y) → (- x, y)
Case 4: (x, y) → (- y, x)
Case 5: (x, y) → (x, - y)
Case 6: (x, y) → (x, y + 10)
How to determine the transformation rule for each case
In this question we find six cases of rigid transformations applied on figures. The most common rigid transformations are listed below:
Translation: (x, y) → (x + a, y + b), where a, b are real numbers.Reflection about x-axis: (x, y) → (x, - y)Reflection about y-axis: (x, y) → (- x, y) Rotation: (x, y) → (x · cos θ - y · sin θ, x · sin θ + y · cos θ), where θ is the angle of rotation.Then, by direct inspection, we derive the following rigid transformations:
Case 1 (Translation)
(x, y) → (x - 10, y + 9)
Case 2 (Rotation)
(x, y) → (x · cos (- 270°) - y · sin (- 270°), x · sin (- 270°) + y · cos (- 270°))
(x, y) → (- y, x)
Case 3 (Reflection about y-axis)
(x, y) → (- x, y)
Case 4 (Rotation)
(x, y) → (x · cos 90° - y · sin 90°, x · sin 90° + y · cos 90°)
(x, y) → (- y, x)
Case 5 (Reflection about x-axis)
(x, y) → (x, - y)
Case 6 (Translation)
(x, y) → (x, y + 10)
To learn more on rigid transformations: https://brainly.com/question/28004150
#SPJ1
which is the correct? help me please

Answers
Answer:
The answer is B. AAA
Step-by-step explanation:
This (AAA) is one of the three ways to test that two triangles are similar . ... If all three angles in one triangle are the same as the corresponding angles in the other, then the triangles are similar.
Answer:
B.
Step-by-step explanation:
Look at this square. Find the value of
S.
S
Perimeter = 16 miles
S =
miles
Answers
The value of S, which represents the length of each side of the square, is 4 miles.
To find the value of S, we need to determine the length of each side of the square. Given that the perimeter of the square is 16 miles, we can use the formula for the perimeter of a square, which is 4 times the length of a side.
Perimeter = 4 × S
Given that the perimeter is 16 miles, we can substitute this value into the equation:
16 = 4 × S
To solve for S, we divide both sides of the equation by 4:
16 ÷ 4 = S
4 = S
Therefore, S, which stands for the square's side lengths, has a value of 4 miles.
So, S = 4 miles.
This means that each side of the square measures 4 miles, and the square has equal sides and right angles at each corner.
for such more question on value
https://brainly.com/question/4344214
#SPJ8
Write the standard form of the equation of theline passing through the point (-5, 3) andperpendicular to the line - 2x – 3y = -6.
Answers
The first step we need to follow is to find the slope of the perpendicular line to -2x-3y = -6.
Then, we have:
\(-2x-3y=-6\Rightarrow-3y=-6+2x\Rightarrow y=\frac{-6}{-3}+\frac{2}{-3}x\Rightarrow y=-\frac{2}{3}x+2\)Then, the equivalent equation for the line has a slope of m1 = - 2/3. The perpendicular line must have an inverse and reciprocal to this slope, that is m2 = 3/2. The product of these slopes must be -1, that is: m1 * m2 = -2/3 * 3/2 = -1.
The second step is to find the line equation for the perpendicular. We know that it has a slope of m = 3/2, and that passes through the point (-5, 3). Then, we can use the point-slope form of the line:
\(y-y_1=m(x-x_1)\)\(y-3=\frac{3}{2}(x-(-5))\Rightarrow y-3=\frac{3}{2}(x+5)\Rightarrow y-3=\frac{3}{2}x+\frac{15}{2}\)Then
\(y=\frac{3}{2}x+\frac{15}{2}+3\Rightarrow y=\frac{3}{2}x+\frac{21}{2}\)The Standard Form of the line is of the form:
\(Ax+By=C\)Then, taking the previous equation:
\(2y=3x+21\Rightarrow-3x+2y=21\)Therefore, the Standard Form of the equation is -3x + 2y = 21.
Answer:-3x + 2y = 21.
Step-by-step explanation:
The first step we need to follow is to find the slope of the perpendicular line to -2x-3y = -6.
Then, we have:
Then, the equivalent equation for the line has a slope of m1 = - 2/3. The perpendicular line must have an inverse and reciprocal to this slope, that is m2 = 3/2. The product of these slopes must be -1, that is: m1 * m2 = -2/3 * 3/2 = -1.
The second step is to find the line equation for the perpendicular. We know that it has a slope of m = 3/2, and that passes through the point (-5, 3). Then, we can use the point-slope form of the line:
Then
The Standard Form of the line is of the form:
Then, taking the previous equation:
Therefore, the Standard Form of the equation is -3x + 2y = 21.
Please help!!!!!!
y=[?]°
![Please help!!!!!!y=[?]](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/JKte3myOEvNxysZvu1N1Q9EH69hKAdxh.png)
Answers
Answer:
Since there are already 2 angles shown, which are 48 and 90 (indicated by the square in the bigger triangle, we can set up an equation.
48 + 90 + y = 180
138 + y = 180
-138 + 138 + y = 180 - 138
y = 42°
how many moles of calcuim oxide will be produced when 1.60 moles of iron(iii) oxide reacts with calcuim phosphate that yeilds iron(iii) phosphate and calcuim oxide
Answers
The reaction will produce 4.8 mol of CaO.
How does a mole work?
The unit of measurement used by chemists known as a mole is very significant. In the same way that having a dozen eggs implies you have twelve eggs, having a mole of anything indicates you have 602,214,076,000,000,000,000,000 of that thing.
When measuring very small objects like atoms, molecules, or other particles, chemists must use moles.
The balanced equation is
Fe₂O₃ + Ca₃(PO₄)₂ ⟶2FePO₄ + 3CaO
You want to convert moles of Fe₂O₃ to moles of CaO.
The molar ratio is 3 mol CaO:1 mol Fe₂O₃.
Moles of CaO = 1.6 mol Fe₂O₃ × (3 mol CaO/1 mol Fe₂O₃)
Moles of CaO = 4.8 mol CaO
The reaction will produce 4.8 mol of CaO.
Learn more about mole
brainly.com/question/26416088
#SPJ1
The complete question is -
how many moles of calcium oxide will be produced when 1.6 moles of Iron(III)oxide reacts with calcium phosphate according to the following (unbalanced) reaction: ____ Fe2O3 + ____ Ca3(PO4)2 —> Fe(PO4) + CaO
The density of a spherical solid of radius 2, centered at the origin, is given by D(p)=3p grams per cm^3. Calculate the mass of the portion of the sphere lying above the plane z= √2.
Answers
M = DV = 3p [2π(2(4-√2) - 2√2) - 2(2-√2)^(3/2) π/3] = 3(4-√2)pπ - 2(2-√2)^(3/2)pπ. Therefore, the mass of the portion of the sphere lying above the plane z= √2 is 3(4-√2) pπ - 2(2-√2)^(3/2)pπ.
The problem requires to determine the mass of the portion of the sphere that lies above the plane z=√2. The density of a spherical solid of radius 2, centered at the origin, is given by D(p)=3p grams per cm^3. Therefore, we can find the mass of the portion of the sphere that lies above the plane z=√2 by integration.To compute the mass, we need to find the volume of the portion of the sphere lying above the plane z= √2, and multiply this by the density. As the sphere is centered at the origin and has a radius of 2, its equation is given by: x² + y² + z² = 4The intersection of the sphere with the plane z=√2 gives a circle with radius 4 - √2. This is the base of the portion of the sphere that lies above the plane z=√2. To find the volume of this portion of the sphere, we can use cylindrical coordinates.
The circle that is the base lies in the plane z=√2, so we take z as the vertical coordinate, and use polar coordinates (r, θ) for the horizontal plane: 0 ≤ r ≤ 4 - √2 , 0 ≤ θ ≤ 2πThe height of the portion is given by the difference between the upper and lower bounds of the z-coordinate. For points on the sphere, the height is given by z = √(4 - x² - y²), so the upper bound of the height is given by z = √(4 - r²), and the lower bound by z = √2. Therefore, the integral for the volume is given by:V = ∫∫∫V dV = ρ∫∫∫V dV = 3p ∫02π∫0^(4-√2) ∫√2^(√(4-r²)) rdzdrdθ.
We can integrate with respect to z first to get:V = 3p ∫02π∫0^(4-√2) (r(√(4-r²)) - r√2) drdθNow, we can integrate with respect to r: V = 3p ∫02π[-(2-r²)^(3/2)/3 + 2(4-√2) - 2√2] dθ= 3p [2π(2(4-√2) - 2√2) - 2(2-√2)^(3/2) π/3]Finally, multiplying by the density, we get the mass: M = DV = 3p [2π(2(4-√2) - 2√2) - 2(2-√2)^(3/2) π/3] = 3(4-√2)pπ - 2(2-√2)^(3/2)pπTherefore, the mass of the portion of the sphere lying above the plane z= √2 is 3(4-√2)pπ - 2(2-√2)^(3/2)pπ.
To know more about Sphere, visit:
https://brainly.com/question/30612663
#SPJ11
A main meal in a Japanese restaurant cost 4374 yen. The exchange rate is £1 = 189 yen. What is the cost in pounds?
Answers
Answer:
23,21 pounds
Step-by-step explanation:
4387/189 = 23,21
Find the area of the circle. Round your answer to the nearest hundredth. It is a whole circle with a diameter of twelve, use the formula a=pi r ^2. I'll give 45 points and brainliest quick pls I have 15 minutes
Answers
Answer: 113
Step by Step explanation:
12/2=6
inches.π⋅6^2≈113
The area of the circle with diameter 12 units is 113.04 square units.
What is Circle?A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre.
The given circle has a diameter of 12.
We have to find the area of the circle.
The formula for Area of circle=πr²
Radius of circle = Diameter/2
=12/2
Radius=6
Now let us put r value in Area of circle formula
A=3.14×6²
=3.14×36
=113.04 square units.
Hence, the area of the circle with diameter 12 units is 113.04 square units.
To learn more on Circles click:
https://brainly.com/question/11833983
#SPJ5
Use a table to show the sample space of two-digit numbers using the digits 1,4,5,9. Use the column label as the tens digit and the row label as the ones digit to complete the table that represents the sample space of two-digit numbers.
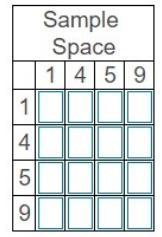
Answers
Answer:
19
Step-by-step explanation:
The volume of a cylinder is 24 cubic feet. The height is 6 feet.
Determine the radius of the cylinder. Use 3.14 for pi.
Answers
Answer:
1.13 ft
Step-by-step explanation:
use the equation then fill in the missing values. when you have your answer round to the nearest thousandths.

PLEASE HELP QUICK 10 POINTS AND I GIVE BRAINLEIST!!! what is the
GCF of 110 and 132
Answers
Answer: 22 because 2x 11 = 22
Hope I helped
Pls give me brainlist.
Step-by-step explanation:
Need help with this question please

Answers
Answer: 22
Step-by-step explanation:
perimeter = a+b+c+d
84 = 4x+4x+(4x+2)+(4x+2)
84 = 16x + 4
80 = 16x
5 = x
length:
=4x+2
=4(5)+2
=22
This is so confusing I just need to find out which linear function it is

Answers
which type of rigid transformation is the equivalent of two reflections across intersecting lines?
Answers
Rotation is the type of rigid transformation which is equivalent of two reflections across intersecting lines.
Define rigid transformation
A rigid transformation is a transformation that persists the original size or shape of a given figure. Examples of rigid transformations are rotation, reflection etc;
In mathematics a rigid transformation is also known as Euclidean Isometry or Euclidean transformation. Euclidean Isometry deals mainly with transformation of any figure. But, doesn't changes the length, shape or size of a given figure.
The concept of of two reflections across intersecting lines is equivalent to single rotation transformation of the original figure.
Therefore the rigid transformation or Euclidean isometry which is similar to two reflections across intersecting lines is rotation.
To know more about rigid transformation visit:
https://brainly.com/question/29001060
#SPJ4
What is the value of x in the equation: 2^(3^x) = 10^(6^x) ?
____________________
False answer will be REPORTED immediately. Do not waste your time if you do not have sufficient knowledge.
Answers
Answer:
\(log\ (log\ 2)/\ log\ 2\) or ≈ - 1.73------------------------------
Solve in below steps, using log and exponent rules:
\(2^{3^x}=10^{6^x}\) Given\(log\ 2^{3^x}=log\ 10^{6^x}\) Log both sides\(3^x\ log\ 2=6^x\ log\ 10\) Log of power rule\(3^x\ log\ 2=6^x\) log 10 = 1\(3^x\ log\ 2=(3*2)^x\) Power of the product rule\(3^x\ log\ 2=3^x*2^x\) Cancel 3ˣ on both sides\(log\ 2=2^x\) Log both sides\(log\ (log\ 2)=x\ log\ 2\) Divide both sides by log 2\(x=log\ (log\ 2)/\ log\ 2\) Answerdr. b. orders a liter of d5lr to infuse over 8 hours. the drop factor is 15 gtts/cc. what is the drip rate per minute?
Answers
A drip rate is the amount of fluid that is being infused into the body of a patient per minute.
The drip rate per minute is 31 drops per minute.
This is usually indicated in drops per minute. In this problem, we have been given the following details:
Volume = 1 liter (1000 cc)
Time = 8 hours (480 minutes)
Drop factor = 15 gtt/cc
We need to determine the drip rate per minute.
Here's how we can calculate it:
Step 1: Find the total number of drops needed for 1 liter of D5LR. We know that 1 cc of the solution has 15 drops. Therefore, 1 liter (1000 cc) will have: 15 drops/cc x 1000 cc = 15,000 drops
Step 2: Determine the drip rate per minute. To infuse 15,000 drops in 8 hours (480 minutes),
we need: 15,000 drops / 480 minutes = 31.25 drops/minute (rounded off to the nearest whole number)
Therefore, the drip rate per minute is 31 drops per minute.
To learn more about “drip rate” refer to the https://brainly.com/question/664324
#SPJ11
1 If one leg of a right triangle is 3 meters, and the hypotenuse is 5 meters, what is the length of the
missing leg?
Answers
i'm gonna assume that "leg" refers to length of the triangle, so comment if you think it is wrong.
Now when it comes to a right-angled triangle, the pythagoras theorem formula can be used.
Using algebra,
let the missing length be X.
By pythagoras theorem,
5² = 3² + X²
5² - 3² = X²
X² = 5² - 3²
X = sq root ( 5² - 3² )
= 4 m
Therefore, the length of the missing leg is 4m .
Help meeeeeeeeeeeeeeeeeee

Answers
Answer:
1 1/2 cups
Step-by-step explanation:
original amount = 2 1/4 cups = (2 × 4 + 1)/4 cups = 9/4 cups
2/3 of original amount = 2/3 × 9/4 cups = 18/12 cups = 3/2 cups = 1 1/2 cups
Challenge You have 2 different savings accounts. For Account A, the simple interest earned after
6 months is $4.13. For Account B, the simple interest earned after 21 months is $26.25. If the
interest rate is 3.3% for Account A and 2.5% for Account B, how much is the principal in each
account? Which account earned you the most interest the first month? Explain your answer.
Account A has a principal of $
(Round to the nearest dollar as needed.)
Answers
Answer:
Save without the temptation of touching it. Earn attractive interest. Better Articulation. Showcase your Strengths.
A car is bought for $22,000. 5 years later it is worth $14,000
a)Write the linear model for this situation
b) How many years will it take for the car to be worth $7,000?
c)In 12 years how much will the car be worth?
Answers
Answer:
b) around 6 years
c) around 2,500
Step-by-step explanation:
Find the value of x. Round your answer to the nearest tenth.

Answers
Answer: 35.1
Step-by-step explanation:
Note that the altitude to the hypotenuse splits the hypotenuse into segments of lengths 16 and 28.
Using the geometric mean theorem, the length of the altitude to the hypotenuse is \(\sqrt{16(28)}=8\sqrt{7}\).
Using the Pythagorean theorem, \(x=\sqrt{(8\sqrt{7})^2 +28^2} \approx 35.1\).
AC=A, C, equals
Round your answer to the nearest hundredth.
Answers
Answer:
We have the equation A*C = A
Now, as both sides of the equality are the same thing, we can do the same operation to both sides and the equality will remain true.
We can divide both sides by A and get:
(A*C)/A = A/A
C = 1
So here we finded the value of A.
If A and C are matrices, then C is the identity matrix.
How many possible 10-digit phone numbers are possible (7 digits plus the
area code)? Type your answer into the box.
Answers
Using the Fundamental Counting Theorem, it is found that 3,250,000,000 phone numbers are possible.
What is the Fundamental Counting Theorem?It is a theorem that states that if there are n things, each with \(n_1, n_2, \cdots, n_n\) ways to be done, each thing independent of the other, the number of ways they can be done is:
\(N = n_1 \times n_2 \times \cdots \times n_n\)
In this problem:
For the first 3 digits, which correspond to the area code, there are 325 options in total, that is, \(n_1n_2n_3 = 325\).For the final 7 digits, each of them has 10 options.Hence:
\(T = 325 \times 10^7 = 3,250,000,000\)
3,250,000,000 phone numbers are possible.
More can be learned about the Fundamental Counting Theorem at https://brainly.com/question/24314866
SOLVE FOR M
Super easy math
Will mark as brainlist

Answers
Answer:
-(m-9.6)/5=-0.9
-m+9.6=-0.9x5
-m+9.6=4.5
-m=4.5-9.6
-m=-5.1
m=5.1
Which of the lines below is a line of symmetry?

Answers
Answer:
D. Both
Step-by-step explanation:
If you look at one line at a time you'll see that they actually show the exact same thing but the lines are in different spots! Hope this helps!^^