Find the number if:
5/9 of it is 90.
And another similar question;
Find the number if:
7/3 of it is 5 5/6
I need help with both of these questions.
Answers
Answer:
162 and \(\frac{5}{2}\)
Step-by-step explanation:
To find the original number multiply by the reciprocal of the multiplier, that is
90 × \(\frac{9}{5}\) = \(\frac{810}{5}\) = 162
------------------------------------------------------------------------
Similarly
Change the mixed number into an improper fraction
5 \(\frac{5}{6}\) = \(\frac{35}{6}\) , then
\(\frac{35}{6}\) × \(\frac{3}{7}\) ( cancel 35 and 7 by 7 / cancel 6 and 3 by 3 )
= \(\frac{5}{2}\) × \(\frac{1}{1}\)
= \(\frac{5}{2}\)
Answer:
162
Step-by-step explanation:
If it is 5/9 of the original number is 90 we can just divide 90 by 5 to find 1/9 and then we can multiply that with 9 to get 9/9 which is the whole number.
90 ÷ 5 = 18
18 × 9 = 162
Related Questions
How do I do this? I need answers to 2 decimal places, please. Thanks :)

Answers
Step-by-step explanation:
perimeter = 30+(2×25)+(½×3.14×30)
= 30+50+47.1
= 127.1 cm
Area = (25×30)+ (½×3.14×15²)
= 750 + 353.25
= 1.103.25 cm²
============================================================
Explanation:
Divide the figure so we have a semicircle on the left and a rectangle on the right. The rectangle is 30 by 25. The semicircle has diameter 30.
If we consider a full circle of diameter 30, then its circumference is exactly C = pi*d = pi*30 = 30pi centimeters.
But we only have half a circle, so we cut that in half to get 30pi/2 = 15pi. That's the distance around the curved portion of this diagram. The straight line portions are handled as exactly as you expect.
Add up the distances around the outer edges to get:
15pi+25+30+25 = 15pi+80
The exact perimeter, in terms of pi, is 15pi+80 centimeters.
To get an approximation of this, we can use pi = 3.14 to get
15*pi + 80 = 15*3.14+80 = 127.1 cm
To get a better approximation, use more decimal digits in pi. Or you can let your calculator handle the decimal digits of pi (in other words, use the stored version of it).
Elizabeth has $252. 00 in her account on Sunday. Over the next week, she makes the following changes to her balance via deposits and purchases: Day Debit ($) Credit ($) Monday 114. 60 150. 00 Tuesday 79. 68 --- Wednesday 161. 39 --- Thursday 57. 40 --- Friday 22. 85 75. 00 Saturday 140. 55 --- On what day does Elizabeth first get charged an overdraft fee? a. Wednesday b. Thursday c. Friday d. Saturday.
Answers
The correct option is d. The day on which Elizabeth first gets charged an overdraft fee is Saturday. Her account balance first becomes negative on this day.
From the given data, we can calculate the balance on each day as shown:
Balance on Monday = $252 - $114.60 + $150.00 = $287.40
Balance on Tuesday = $287.40 - $79.68 = $207.72
Balance on Wednesday = $207.72 - $161.39 = $46.33
Balance on Thursday = $46.33 - $57.40 = -$11.07
Balance on Friday = -$11.07 - $22.85 + $75.00 = $41.08
Balance on Saturday = $41.08 - $140.55 = -$99.47
We see that Elizabeth's balance first becomes negative on Saturday, so she will be charged an overdraft fee on that day.Answer: d. Saturday
The day on which Elizabeth first gets charged an overdraft fee is Saturday. Her account balance first becomes negative on this day.
To know more about account balance visit:
brainly.com/question/23271078
#SPJ11
What is the answer plsssss help

Answers
Answer:
f=9 x 20
Step-by-step explanation:
PLEASE HELP ME WITH THIS
Elijah started a college fund of $25,000 for a term of five years.The interest rate is 2.9 percent annually.How much interest will he have earned at the end of the term?
Answers
Answer:
Interest = Principal x rate x time
I = 25000(.029)(5) = $3,625
Step-by-step explanation:
1. A rectangular room has an area of 400 sq. ft. The length is 7 ft less than twice the width. Find the
dimensions of the room.
Answers
Answer:
25 feet long and 16 feet wide
Step-by-step explanation:
16 x 2 = 32
32 - 7 = 25
25 x 16 = 400
A deluxe bakery sells a cookie for $4.01, a brownie for $4.1 and a cake for $30.54. On Wednesday they sold 13 cookies, 5 brownies and 3 cakes. The cost in ingredients for each cookie is $1.79, for,each brownie is $0.63 and for each cake is $6. 71. How much profit did they make on Wednesday?
Answers
Answer:
\(Profit = \$117.7\)
Step-by-step explanation:
Given
Selling Price of:
\(Cookies = \$4.01\)
\(Brownie = \$4.1\)
\(Cake = \$30.54\)
Quantities Sold:
\(Cookies = 13\)
\(Brownies = 5\)
\(Cake = 3\)
Cost Price:
\(Cookies = \$1.79\)
\(Brownie = \$0.63\)
\(Cake = \$6.71\)
Required
Determine the profit
First, we need to calculate the total cost price:
Total Cost Price = Item Cost Price * Quantity ---- For each
i.e.
\(Total\ Cost\ Price = \$1.79 * 13 + \$0.63 * 5 +\$6.71 * 3\)
\(Total\ Cost\ Price = \$23.27 + \$3.15 +\$20.13\)
\(Total\ Cost\ Price = \$46.55\)
Then, the total selling price
Total Selling Price = Item Selling Price * Quantity ---- For each
\(Total\ Selling\ Price = \$4.01 * 13 + \$4.1 * 5 + \$30.54 * 3\)
\(Total\ Selling\ Price = \$52.13 + \$20.5+ \$91.62\)
\(Total\ Selling\ Price = \$164.25\)
Profit is calculated as thus:
\(Profit = Total\ Selling\ Price - Total\ Cost\ Price\)
\(Profit = \$164.25 - \$46.55\)
\(Profit = \$117.7\)
4.4.24
Question Help
Let AABC ADEF. Find mZE.
The value of mZE is 19
(Simplify your answer. Type an integer or a decimal.)
(83+y)
2x+63
С
6x +3
(49-23)
E
Help please

Answers
Answer:
The angle marked E is 120 degrees
Step-by-step explanation:
Here, we want to get the value of the angle marked E
when two triangles are similar/congruent, we have equal angle measures
Using the markings, we can deduce the following relationships;
4y -28 = 83 + y
4y-y = 83 + 28
3y = 111
y = 111/3
y = 37
So, we have;
4y-28 as
4(37) - 28
= 120
120 bats are independently sampled from a population. if 25% of the bats in the population are infected by coronaviruses, which possible numbers of infected bats in the sample are within one standard deviation of the mean?
Answers
The possible numbers of infected bats in the sample that are within one standard deviation of the mean are 20 to 30.
To determine this, we can use the binomial distribution. Let's define the following variables:
- \(n\) represents the number of bats sampled from the population, which is 120 in this case.
- \(p\) represents the probability of a single bat being infected, which is 25% or 0.25.
- \(q\) represents the probability of a single bat not being infected, which is 1 - p, or 0.75.
The mean (\(\mu\)) of the binomial distribution is given by \(\mu = np\), and the standard deviation \((\(\sigma\)) is given by \(\sigma = \sqrt{npq}\).\)
In this case, the mean is \(\mu = 120 \times 0.25 = 30\) and the standard deviation is \(\(\sigma = \sqrt{120 \times 0.25 \times 0.75} \approx 5.49\).\)
To find the possible numbers of infected bats within one standard deviation of the mean, we need to consider values that are within the range of \(\mu \pm \sigma\). Therefore, the range is from \(\mu - \sigma\) to \(\mu + \sigma\).
Calculating the lower and upper bounds:
Lower bound: \(\(\mu - \sigma = 30 - 5.49 \approx 24.51\)\)
Upper bound: \(\(\mu + \sigma = 30 + 5.49 \approx 35.49\)\)
Since the number of bats must be an integer, we round the bounds to the nearest whole numbers:
Lower bound: 25
Upper bound: 36
Thus, the possible numbers of infected bats in the sample that are within one standard deviation of the mean are 25 to 36.
Learn more about standard deviation here
https://brainly.com/question/475676
#SPJ11
Ms. Soffer paid $42. 75 for 9 boxes of gummies for the state test last year. This year she will need to order 13 boxes of gummies for the state test. If the price per box does not change, what would be the total cost of this year’s order?
Answers
Answer:
$61.75
Step-by-step explanation:
I divided the $42.75 by 9 to get 4.75. Then i multiplied the 4.75 by 13 to get $61.75.
Riya is applying mulch to her garden. She applies it at a rate of 250{,}000\text{ cm}^3250,000 cm
Answers
The rate which the mulch is being applied is 250,000 cm^3/cm^2
How to determine the rate which the mulch is being applied?The statement is given as
Rita is applying 250,000 cm^3 of mulch per m^2 of the garden space
This means that the rate is
Rate = Volume of mulch/Area of garden space
Substitute the known values in the above equation
So, we have
Rate = 250,000 cm^3/cm^2
Hence, the rate which the mulch is being applied is 250,000 cm^3/cm^2
Read more about rates at
brainly.com/question/2021001
#SPJ1
How do you find 1/3 of a line segment?
Answers
To find 1/3 of a line segment, divide the line segment into three equal parts. Measure the length of one of these parts and this will be 1/3 of the line segment.
This can be done by using a ruler or a measuring tape. Alternatively, if the length of the line segment is known, divide it by 3 to find 1/3 of the line segment. For example, if the line segment has a length of 12 inches, then the length of 1/3 of the line segment is 4 inches. If the line segment is curved, you can divide it into three equal arc lengths using a compass.
You can then measure the length of one of these arcs to find 1/3 of the line segment. To make sure that the three parts are equal, you can use the Pythagorean theorem or the triangle inequality theorem. This will help you to accurately divide the line segment into three equal parts.
To know more about line segment refer to the link brainly.com/question/30072605
#SPJ4
in a shipment of 21 smartphones, 2 are defective. how many ways can a quality control inspector randomly test 5 smartphones, of which 2 are defective?
Answers
There are 969 methods for the quality control inspector to randomly check 5 smartphones, of which 2 are defective, from the shipment of 21 smartphones.
We can solve this problem using combinations formula, that is a way of counting the number of ways to select k objects from a fixed of n items without regard to order. The range of combinations of k items from a fixed of n objects is given via:
\(C(n, k) = n! / (k! * (n-k)!)\)
in which:
n: the total number of objects within the setk: the number of objects to pick from the setIn this situation, we want to pick out 2 defective smartphones out of 2 defective smartphones and 19 non-defective smartphones, and we need to pick out out three non-defective smartphones out of the ultimate 19 non-defective smartphones.
Consequently, the number of approaches to choose 2 defective smartphones and 3 non-Defective smartphones from the set of 21 smartphones is:
\(C(2, 2) * C(19, 3) = 1 * (19! / (3! * 16!)) = 191817 / (321) = 969\)
Therefore, there are 969 methods for quality control.
Learn more about Combination formula:-
https://brainly.com/question/28065038
#SPJ4
Use the Distance Formula to find VW PLEASE HELP I WILL MARK BRAINLIEST!!

Answers
Answer:
\(d=5\)
Step-by-step explanation:
Distance Formula: \(d=\sqrt{(x_2-x_1)^2+(y_2-y_1)^2}\)
Simply plug in the 2 coordinates into the formula to find distance d:
\(d=\sqrt{(4-1)^2+(-1-3)^2}\)
\(d=\sqrt{(3)^2+(-4)^2}\)
\(d=\sqrt{9+16}\)
\(d=\sqrt{25}\)
\(d=5\)
Which mapping(s) below represent(s) a function?

Answers
Answer: D
Step-by-step explanation:
a poker hand consists of 5 cards dealth from a deck of 52 cards. let x and y be the number of aces and kings in a poker hand. find the joint distribution of x and uy
Answers
Joint Distribution of x and y is 25.
Joint Distribution is based on joint probability, which can be simply defined as the probability of two events happening together.
We know the formula for joint distribution
F(x,y) = P(X ≤ x and Y ≤ y) = ∑∑p(xi,yi)
Here x is the number of aces and y is the number of kings in a poker hand.
That means x can take values from 0 to 4 and y can take values from 0 to 4.
∴ F(x,y) = P(X ≤ 4 and Y ≤ 4)
⇒ F(x,y) = p(0,0) + p(0,1) + p(0,2) + p(0,3) + p(0,4) + p(1,0) + p(1,1) + p(1,2) + p(1,3) + p(1,4) + p(2,0) + p(2,1) + p(2,2) + p(2,3) + p(2,4) + p(3,0) + p(3,1) + p(3,2) + p(3,3) + p(3,4) + p(4,0) + p(4,1) + p(4,2) + p(4,3) + p(4,4).
To find all these values we will construct a table as follows;
P(x,y) Y=0 Y=1 Y=2 Y=3 Y=4
X=0 0 1/4 1/2 3/4 1
X=1 1/4 1/2 3/4 1 5/4
X=2 1/2 3/4 1 5/4 3/2
X=3 3/4 1 5/4 3/2 7/4
X=4 1 5/4 3/2 7/4 2
Now the joint distribution will become,
F(x,y) = 0 + 1/4 + 1/2 + 3/4 + 1 + 1/4 + 1/2 + 3/4 +1 + 5/4 + 1/2 + 3/4 + 1 + 5/4 + 3/2 + 3/4 + 1 + 5/4 + 3/2 + 7/4 + 1 + 5/4 + 3/2 + 7/4 + 2
F(x,y) = 25
Therefore the joint distribution of x and y is 25.
To know more about Joint distribution here
https://brainly.com/question/14310262
#SPJ4
if the median of 127 numbers is 35, which of the following must be true? i) at least 64 of the numbers are greater than or equal to 35 ii) at least 64 of the numbers are smaller than 35 iii) at most 64 numbers are greater than or equal to 35
Answers
Answer:
Option i) At least 64 of the numbers are greater than or equal to 35 must be true
Since the median is 35, we know that there are 63 numbers greater than 35 and 63 numbers smaller than 35. However, since there is an odd number of total numbers (127), the median itself must be included in one of these groups. Therefore, there are at least 64 numbers that are greater than or equal to 35.
17. Sally put $960 in a savings account that earns 7% interest compounded quarterly. How much interest will she earn at the end of the thrid quarter? What will be her account balance at that time?
Answers
well, one quarter of a year is 3 months, so at the end of third quarter, 9 months have passed, and since a year has 12 months, 9 months are simply 9/12 of a year.
\(~~~~~~ \textit{Compound Interest Earned Amount} \\\\ A=P\left(1+\frac{r}{n}\right)^{nt} \quad \begin{cases} A=\textit{accumulated amount}\\ P=\textit{original amount deposited}\dotfill &\$960\\ r=rate\to 7\%\to \frac{7}{100}\dotfill &0.07\\ n= \begin{array}{llll} \textit{times it compounds per year}\\ \textit{quarterly, thus four} \end{array}\dotfill &4\\ t=years\to \frac{9}{12}\dotfill &\frac{3}{4} \end{cases}\)
\(A=960\left(1+\frac{0.07}{4}\right)^{4\cdot \frac{3}{4}}\implies A=960(1.0175)^3\implies A\approx 1011.29\)
The angles of a triangle are 989, 51°, and x®,
What is the value of x?
Answers
9514 1404 393
Answer:
x = 31
Step-by-step explanation:
The sum of angles in a triangle is 180°.
98° +51° +x° = 180°
x° = 31° . . . . . . . . . . . . subtract 149°
x = 31
Comparing two algorithms.
Say we have two different algorithms with respective runtimes of f(n) and g(n). Given the following cases, prove whether or not f(n) = ϴ(g(n)) is true in each case. Show your work but with the crucial steps only. P.S. sqrt(n) means the square-root of n, aka n^(½).
Case
f(n)
g(n)
A
log(n^200)
log(n^2)
B
sqrt(n)
log(n)
C
3^n
5^n
D
sin(n)+3
cos(n)+1
Answers
f(n) = ϴ(g(n)) is not true in cases B(sqrt(n)log(n), C(\(3^n 5^n\)), and D(sin(n)+3 cos(n)+1).
A) \(log(n^200) log(n^2)\)
Here, f(n) = \(log(n^200)\) and g(n) = \(log(n^2)\). Now, if we take the limit of f(n) / g(n) as n approaches infinity, then:
f(n) / g(n) = \([log(n^200) / log(n^2)]\) = 100
This means that as n approaches infinity, the ratio f(n) / g(n) is constant, and so we can say that f(n) = ϴ(g(n)). Therefore, f(n) = ϴ(g(n)) is true in this case.
B) sqrt(n) log(n) Here, f(n) = sqrt(n) and g(n) = log(n). Now, if we take the limit of f(n) / g(n) as n approaches infinity, then:
f(n) / g(n) = [sqrt(n) / log(n)]
As log(n) grows much slower than sqrt(n) as n approaches infinity, this limit approaches infinity. Therefore, we cannot say that f(n) = ϴ(g(n)) is true in this case.
C) 3^n 5^n
Here, f(n) = \(3^n\) and g(n) = \(5^n\) . Now, if we take the limit of f(n) / g(n) as n approaches infinity, then:
f(n) / g(n) = \([3^n / 5^n]\)
As \(3^n\) grows much slower than \(5^n\) as n approaches infinity, this limit approaches zero. Therefore, we cannot say that f(n) = ϴ(g(n)) is true in this case.
D) sin(n) + 3 cos(n) + 1
Here, f(n) = sin(n) + 3 and g(n) = cos(n) + 1. Now, if we take the limit of f(n) / g(n) as n approaches infinity, then:
f(n) / g(n) = [sin(n) + 3] / [cos(n) + 1]
As this limit oscillates between positive and negative infinity as n approaches infinity, we cannot say that f(n) = ϴ(g(n)) is true in this case.
Therefore, f(n) = ϴ(g(n)) is not true in cases B, C, and D.
To know more about log refer here:
https://brainly.com/question/32621120
#SPJ11
What is the frequency of the sinusoidal graph?
Answers
Answer:
equal to 1
Step-by-step explanation:
I believe this is the answer. Also I see that ur new. Well welcome to Brainly.
solve for x, 2^2=128
Answers
Answer:
x=32
Step-by-step explanation:
I'm quite confused on this question. (will thanks and rate!)

Answers
This scenario, the median for the morning meeting is 34 and the median for the afternoon meeting is 38.
Then the actual answer is 4 because it's asking how many more years is the median age of the Afternoon Meetings to the Morning Meetings, which you can find by subtracting 38 from 34.
Hope this helps!
What is the area of a circle with a diameter of 29 centimeters?
cm2
(Use 3.14 for Pi.)
Answers
Answer:
Step-by-step explanation:
AREA OF CIRCLE = 660.185CM^2
HOPE IT HELPS
suppose x is a normal random variable with exam image and exam image find p(3.6 < x < 53.5). a) exam image b) exam image c) exam image d) exam image e) exam image f) none of the above.
Answers
After using the test statistic z, P(3.6<X<53.5) = 0.967.
In the given question, suppose X is a normal random variable with mean 35 and sdev 10.
We have to find P(3.6<X<53.5).
Fro the given question,
Mean x = 35
sdev s = 10
Z = X - mu / sd
P(3.6<X<53.5) = P ( 3.6-35/10 < Z < 53.5-35/10 )
P(3.6<X<53.5) = P ( -31.4/10 < Z < 18.5/10 )
P(3.6<X<53.5) = P ( -3.14 < Z < 1.85 )
P(3.6<X<53.5) = P ( Z < 1.85) - P ( Z < -3.14)
left tail of both
P(3.6<X<53.5) = 0.9678 - 0.0008
P(3.6<X<53.5) = 0.967
To learn more about test statistic z link is here
brainly.com/question/3006526
#SPJ4
The right answer is:
Suppose X is a normal random variable with mean 35 and sdev 10. Find P(3.6<X<53.5)
3.
X
5
10
15
20
y
13
28
43
58
Is it a Linear or non linear?
Answers
i think the answer is linear I am not sure though
hmm need help please
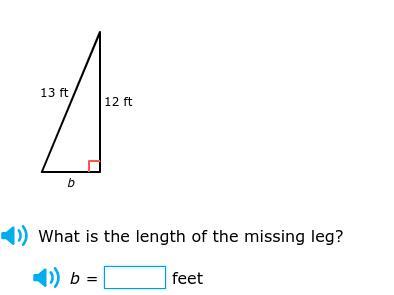
Answers
Answer:
Hello! answer: b = 5
Step-by-step explanation:
13 × 13 = 169 12 × 12 = 144 169 - 144 = 25 5 × 5 = 25 therefore b = 5 hope that helps!
13x13=169
169-144=25
5^2= 25
B=5 feet
Help!!
I need help with this question, and can you also explain how you did it so I can understand it.

Answers
can someone please help me with this ?

Answers
Answer:
Tess
Step-by-step explanation:
Indi: 2-3= -1
Mark: -7 - (-4)= -7+4 which is -3
Tess: -1-(-7)= -1+7 which is 6
Tess wins the prize since, 6 is the biggest
Indi comes in second since, it's bigger than -3
Mark come in last place
FAKE ANSWERS/LINKS WILL BE REPORTED. PLEASE HELP
The population of Metropolis was approximately 2,600,000 people in 2000. The population began decreasing at a rate of 1.1% every year after 2000.
Which function models the population of Metropolis x years after 2000?
f(x)=0.989(2,600,000)x
f(x)=2,600,000(1.011)x
f(x)=2,600,000(0.989)x
f(x)=1.011(2,600,000)x
Answers
Answer:
f(x) = 2,600,000(0.989)x
Step-by-step explanation:
2. What is the slope of a line perpendicular to the line y=-4x + 16?
1/4
-4
4
-1/4
Answers
The slope of a line perpendicular to y=-4x + 16 is (1/4), so the correct option is the first one (counting from the top).
How to get the slope of the perpendicular line?
The general linear equation in the slope-intercept form is:
y = a*x + b
Where a is the slope and b is the y-intercept.
Let's say that we have another line:
y = c*x + d
These two lines are perpendicular if and only if the slope of one of the lines is equal to the inverse of the opposite of the other line.
So, the two lines:
y = a*x + b
y = c*x + d
Are perpendicular only if c = -(1/a).
Now, we want to find a line perpendicular to:
y=-4x + 16
Then the slope of that perpendicular line must be:
-(1/-4) = (1/4)
So the slope of a line perpendicular to y=-4x + 16 is (1/4).
Learn more about linear equations:
https://brainly.com/question/1884491
#SPJ1