Find the mean and compare it with the median. Find the standard deviation and compare it with the interquartile range
Answers
Question:
Find the mean and compare it with the median. Find the standard deviation and compare it with the interquartile range. Calculate s for the data 4, 1, 3, 10, 2.
Answer:
(a)
\(Mean = 4\)
\(Median=3\)
\(Mean > Median\)
(b)
\(IQR = 2\)
\(SD = 3.2\)
\(SD> IQR\)
Step-by-step explanation:
Given:
Data: 4, 1, 3, 10, 2.
Solving (a): The mean and the Median
The mean is calculated as follows:
\(Mean = \frac{1}{n} \sum x\)
Where
\(n = 5\) i.e 5 data
So, the expression becomes:
\(Mean = \frac{1}{5}(4 + 1+3+10+2)\)
\(Mean = \frac{1}{5}(20)\)
\(Mean = 4\)
Calculating the Median:
First, arrange the order (ascending order):
\(Data: 1, 2,3,4,10\)
Because n is odd
The median is represented as:
\(Median = (\frac{n+1}{2})th\ item\)
\(Median = (\frac{5+1}{2})th\ item\\\)
\(Median = (\frac{6}{2})th\ item\)
\(Median = 3rd\ item\)
From the arranged data, the 3rd item is 3.
Hence:
\(Median=3\)
By comparison, the mean is greater than the median because \(4 > 3\)
Solving (b): Standard Deviation and IQR
The standard deviation is calculated as follows:
\(SD= \sqrt{\frac{\sum (x_i - Mean)^2}{n}\)
So, we have:
\(SD= \sqrt{\frac{(4 - 4)^2+(1 - 4)^2+(3 - 4)^2+(10 - 4)^2+(2 - 4)^2}{5}\)
\(SD= \sqrt{\frac{(0)^2+(- 3)^2+(- 1)^2+(6)^2+(-2)^2}{5}\)
\(SD= \sqrt{\frac{0+9+1+36+4}{5}\)
\(SD= \sqrt{\frac{50}{5}\)
\(SD= \sqrt{10}\)
\(SD = 3.2\)
Calculating IQR
\(IQR = Q_3 - Q_1\)
\(Data: 1, 2,3,4,10\)
In (a), we calculate the median as:
\(Median=3\)
First, we calculate \(Q_1\)
\(Q_1 = Median\ of\ the\ first\ half.\)
The first half is:
\(First\ Half = 1,2,3\)
So:
\(Q_1 = 2\)
First, we calculate \(Q_3\)
\(Q_3 = Median\ of\ the\ second\ half.\)
\(Second\ Half = 3,4,10\)
So:
\(Q_3 = 4\)
Recall that:
\(IQR = Q_3 - Q_1\)
\(IQR = 4 - 2\)
\(IQR = 2\)
So, we have:
\(IQR = 2\)
\(SD = 3.2\)
By comparison, the standard deviation is greater than the IQR because:
\(3.2 > 2\)
So,
\(SD> IQR\)
Related Questions
00210.0 points Assuming 69.2 heartbeats/minutes, estimate the total number of times the heart of a human beats in an average lifetime of 68.4y. Assume 365.25 days per year. Answer in units of heartbeats. Your answer must be within ±5.0% 003 10.0 points How many (whole number of) 91 kg people can safely occupy an elevator that can hold a maximum mass of exactly 1 metric ton? A metric ton is 1.000×10
3
kg. Answer in units of people. 00410.0 points A human hair is approximately 56μm in diameter. Express this diameter in meters. Answer in units of m. 00510.0 points Convert 74mi/h to m/s. 1mi=1609 m. Answer in units of m/s.
Answers
The estimated total number of heartbeats in an average lifetime of 68.4 years is 2.9 billion heartbeats. The maximum number of 91 kg people that can safely occupy an elevator is 10 people. The diameter of human hair is 0.000056 meters.
(a) To estimate the total number of heartbeats in a lifetime, we multiply the heart rate (69.2 beats/minute) by the number of minutes in a year (60 minutes/hour * 24 hours/day * 365.25 days/year) and then multiply by the number of years in a lifetime (68.4 years). The calculation is: 69.2 beats/minute * 60 minutes/hour * 24 hours/day * 365.25 days/year * 68.4 years ≈ 2,886,699,648 beats. Therefore, the estimated total number of heartbeats in an average lifetime is approximately 2.9 billion heartbeats.
(b) To determine the maximum number of 91 kg people that can occupy the elevator, we divide the maximum mass the elevator can hold (1 metric ton or 1000 kg) by the mass of each person (91 kg). The calculation is: 1000 kg / 91 kg ≈ 10.98. Since we can only have whole numbers of people, the maximum number of people that can safely occupy the elevator is 10.
(c) To express the diameter of a human hair in meters, we convert the given diameter of 56 μm to meters by dividing by 1 million (since 1 μm = 1/1,000,000 meters). Therefore, the diameter of human hair is approximately 0.000056 meters.
(d) To convert 74 miles per hour to meters per second, we multiply the given value by the conversion factor 1609 meters/mile and divide by 3600 seconds/hour. The calculation is 74 miles/hour * 1609 meters/mile / 3600 seconds/hour ≈ 33.12 meters/second. Therefore, 74 miles per hour is approximately equal to 33.12 meters per second.
Learn more about diameter here:
https://brainly.com/question/32968193
#SPJ11
1. Find the geometric mean of the
two numbers.
4 and 42.25
Answers
Answer:
13
Step-by-step explanation:
Graph the linear equation x=−1.
Answers
we define a uniform random variable between 5 and 15. what is the value of the probability density function (pdf) at 0?
Answers
The value of the probability density function (pdf) at 0 for a uniform random variable between 5 and 15 is 0, because the pdf for a uniform distribution is constant between its minimum and maximum values, and is 0 elsewhere.
To explain further, a uniform distribution is a continuous probability distribution where every value within a certain range has an equal chance of being selected. In this case, the range is between 5 and 15. The pdf for a uniform distribution is constant within the range of the distribution and is 0 outside of it.
Since 0 is not within the range of the uniform distribution, the pdf at 0 is 0. This means that the probability of selecting a value of 0 from this uniform distribution is 0. The area under the pdf curve between 5 and 15 is equal to 1, which means that the probability of selecting a value within this range is 1.
For more questions like Probability visit the link below:
https://brainly.com/question/30831431
#SPJ11
Mary is buying tickets for a movie.
*Each adult ticket costs $99.
*Each child ticket costs $55.
*Mary spends $110110 on tickets.
*Mary buys 1414 total tickets.
What is the total number of adult tickets and the total number of child tickets she buys?
Answers
Answer:
she bought 21 child tickets
we want to determine the probability of obtaining at most 4 successful operations in 10 independent surgical operations where the probability of success is the same for each operation. the appropriate formula to be used is
Answers
Using (D) the binomial formula, which is used to determine probability when independent events are known, we will get the probability in the scenario.
What is the binomial formula?In an experiment with two possible outcomes, the likelihood of exactly x successes on n repeated trials is known as the binomial probability (commonly called a binomial experiment).
The binomial probability is nCx⋅px⋅(1−p)n−x if the likelihood of success on a single trial is p.
When a process is repeated a certain number of times (for example, in a set of patients), the result for each patient can either be a success or a failure, the binomial distribution model enables us to calculate the probability of witnessing a defined number of "successes."
So, in the given situation we will find the probability with help of the binomial formula which is used to calculate the probability when independent events are given.
Therefore, using (D) the binomial formula, which is used to determine probability when independent events are known, we will get the probability in the scenario.
Know more about the binomial formula here:
https://brainly.com/question/9325204
#SPJ4
Complete question:
We want to determine the probability of obtaining at most 4 successful operations in 10 independent surgical operations where the probability of success is the same for each operation. The appropriate formula to be used is
(A) the mean of the binomial distribution.
(B) the hypergeometric formula.
(C) the mean of the hypergeometric distribution.
(D) the binomial formula.
plz help me on this its timed

Answers
Answer:
-2.72
Step-by-step explanation:
You need to dived -8.16 by 3t to get the answer
abc is a right triangle with ab=ac. bisector of <a meets bc at d. prove that bc = 2ad.
Answers
Answer:
Let ac=ab=5
With this, bc= 5√2
Step-by-step explanation:
So to find ad, Let ad be x
5√2=(2)(x)
(5√2/2)= x
This proves that bc=2ad

The dimension of the row space of a 3 x 3 matrix A is 2. (a) What is the dimension of the column space of A? (b) What is the rank of A? (c) What is the nullity of A? (d) What is the dimension of the solution space of the homogeneous system Ax = 0?
Answers
a) the dimension of its column space is also 2. b) the rank of A is 2. c) the nullity of matrix A is 1. d) the dimension of the solution space of the homogeneous system \(A_x = 0\) is also 1.
(a) The dimension of the row space of a matrix is equal to the dimension of its column space. So, if the dimension of the row space of matrix A is 2, then the dimension of its column space is also 2.
(b) The rank of a matrix is defined as the maximum number of linearly independent rows or columns in the matrix. Since the dimension of the row space of matrix A is 2, the rank of A is also 2.
(c) The nullity of a matrix is defined as the dimension of the null space, which is the set of all solutions to the homogeneous equation Ax = 0. In this case, the matrix A is a 3 x 3 matrix, so the nullity can be calculated using the formula:
nullity = number of columns - rank
nullity = 3 - 2 = 1
Therefore, the nullity of matrix A is 1.
(d) The dimension of the solution space of the homogeneous system Ax = 0 is equal to the nullity of the matrix A. In this case, we have already determined that the nullity of matrix A is 1. Therefore, the dimension of the solution space of the homogeneous system \(A_x = 0\) is also 1.
Know more about matrix here:
brainly.com/question/24079385
#SPJ4
EX24) 29 du Use the chain rule to find the indicated derivative. og, where du g(u, v) = f(x(u, v),y(u, v)), f(x,y) = 7x³y³.x(u, v) = ucosv, y(u, v) = usiny = 56u² cos v sin³ v
Answers
∂g/∂u is equal to 21u⁵cos⁴(v)sin⁴(v)(cos(v) + u³cos⁴(v)sin²(v)sin(v)).
To find the indicated derivative, we need to use the chain rule. Let's differentiate step by step:
Given:
g(u, v) = f(x(u, v), y(u, v))
f(x, y) = 7x³y³
x(u, v) = ucos(v)
y(u, v) = usin(v)
To find ∂g/∂u, we differentiate g(u, v) with respect to u while treating v as a constant:
∂g/∂u = (∂f/∂x) * (∂x/∂u) + (∂f/∂y) * (∂y/∂u)
To find ∂f/∂x, we differentiate f(x, y) with respect to x:
∂f/∂x = 21x²y³
To find ∂x/∂u, we differentiate x(u, v) with respect to u:
∂x/∂u = cos(v)
To find ∂f/∂y, we differentiate f(x, y) with respect to y:
∂f/∂y = 21x³y²
To find ∂y/∂u, we differentiate y(u, v) with respect to u:
∂y/∂u = sin(v)
Now, we can substitute these partial derivatives into the equation for ∂g/∂u:
∂g/∂u = (21x²y³) * (cos(v)) + (21x³y²) * (sin(v))
To find the simplified form, we substitute the given values of x(u, v) and y(u, v) into the equation:
x(u, v) = ucos(v) = u * cos(v)
y(u, v) = usin(v) = u * sin(v)
∂g/∂u = (21(u * cos(v))²(u * sin(v))³) * (cos(v)) + (21(u * cos(v))³(u * sin(v))²) * (sin(v))
Simplifying further, we get:
∂g/∂u = 21u⁵cos⁴(v)sin⁴(v)(cos(v) + u³cos⁴(v)sin²(v)sin(v))
Know more about derivative here:
https://brainly.com/question/25324584
#SPJ11
how many 25s are in 2
Answers
Answer: is the same as asking "How much is 25 divided by 2?" Thus, the answer can be calculated as follows: 25/2 = 12.5. "How many times does 2 go into 25?" is also the same as asking "What (x) do you multiply by 2 to get 25?" We solve the problem with an easy algebra equation:
2(x) = 25
x = 12.5
Step-by-step explanation:
use the level curves to predict the location of the critical points of f and determine whether f has a saddle point, local maximum, or local minimum at each point. then use the second derivatives test to confirm your predictions. (if an answer does not exist, enter dne.)
Answers
(0,0) is a saddle point and (1,1) is a local minimum.
The point as in domain of something like the functions with the lowest value is known as the local minimum. You can calculate the local minimum by determining the function's derivative.A saddle point of minimax point is a location on the graph's surface where the slopes of all orthogonal derivatives are zero (a crucial point), but which isn't the function's local extremum.From the contour map, the level curves intersect at (0,0) . Hence (0,0) is a saddle point.
and the center of level curve 3.2 (1,1) is a local minimum.
Hence (0,0) is a saddle point and (1,1) is a local minimum.
Learn more about saddle point here:
https://brainly.com/question/28853367
#SPJ4
Chen is offered isolation pay of $1250.00 for a job in northern Manitoba.
Alternatively, he can have a bonus payment of 28% of his salary. If his salary is
$532.00/wk, which is the better option if it takes 10 weeks to complete the job?
Answers
Answer:
He should choose a bonus of 28% of his salary.

The number of calories burned y after x minutes of kayaking is represented by the linear function y=4.5x. The graph shows the calories burned by hiking.
Answers
I can answer is ez probably
Answer:
22.5 more calories are burned
Hiking:
y=255
kayak
y=202.5

-7/4+0+-9/5+19/10+11/4
Answers
Answer:
bbbbbbbbbbbbbbbbbbbbbbbbbbbbbb
Step-by-step explanation:
bbbbbbbbb
jermaine is testing the effectiveness of a new acne medication. there are 100 people with acne in the study. forty patients received the acne medication, and 60 other patients did not receive treatment. fifteen of the patients who received the medication reported clearer skin at the end of the study. twenty of the patients who did not receive medication reported clearer skin at the end of the study. what is the probability that a patient chosen at random from this study took the medication, given that they reported clearer skin? 0.15 0.33 0.38 0.43
Answers
The probability that a patient chosen at random from this study took the medication, given that they reported clearer skin, is approximately 0.43.
To find the probability that a patient chosen at random from the study took the medication, given that they reported clearer skin, we can use conditional probability.
Let's denote the events:
A: Patient took the medication.
B: Patient reported clearer skin.
We want to find P(A|B), which is the probability that a patient took the medication given that they reported clearer skin.
From the information given:
Number of patients who received the medication and reported clearer skin = 15
Number of patients who did not receive the medication and reported clearer skin = 20
Total number of patients who reported clearer skin = 15 + 20 = 35
Number of patients who received the medication = 40
Total number of patients in the study = 100
Using these values, we can calculate P(A|B) using the formula for conditional probability:
P(A|B) = P(A ∩ B) / P(B)
P(A ∩ B) is the probability that a patient both took the medication and reported clearer skin, which is given as 15.
P(B) is the probability that a patient reported clearer skin, which is calculated as the number of patients who reported clearer skin divided by the total number of patients in the study:
P(B) = 35 / 100 = 0.35
Therefore, we can now calculate P(A|B):
P(A|B) = P(A ∩ B) / P(B) = 15 / 0.35 ≈ 0.43
Hence, the probability that a patient chosen at random from this study took the medication, given that they reported clearer skin, is approximately 0.43.
Learn more about conditional probability here:
https://brainly.com/question/30144287
#SPJ11
Solve this problem.
348-3435+847
Answers
Answer:
3934Step-by-step explanation:
PLS MARK BRAILIESTAnswer:
-2240
Step-by-step explanation:
1. 348-3435 = -3087
2. -3087 + 847 = -2240
What is the solution to the inequality 5.25 - b 2 6.52
+
+→
5
-5 -4 -3 -2 -1 0
1
2
3
4
OH ++
-5 -4 -3 -2 -1 0 1 2
+
3
+
4
5
OA
-5 -4 -3 -2 -1 0 1
2
3 4 5
-5 -4 -3 -2 -1 0 1 2 3 3 4 4 5

Answers
Answer:
1st option
Step-by-step explanation:
Solving the inequality
5.25 - b ≥ 6.5 ( subtract 5.25 from both sides )
- b ≥ 1.25
Divide both sides by - 1, reversing the symbol as a result of dividing by a negative quantity.
b ≤ - 1.25
Since less than or equal to the number line will have a solid circle at - 1.25 and the arrow pointing left.
The solution is represented on the first diagram
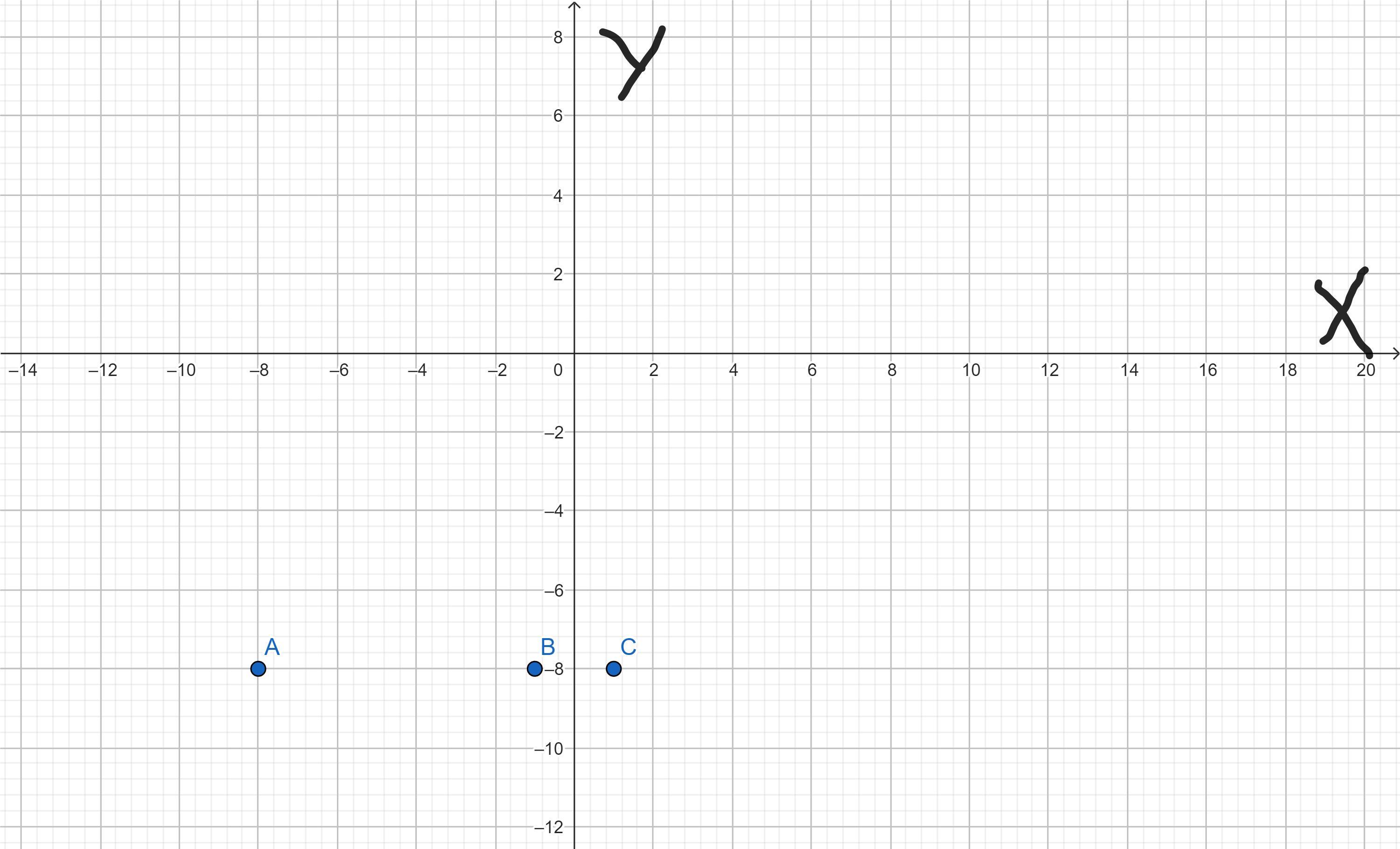
Please lmk asap, I’m so lost.

Answers
The complete table is:
x f(x)
-8 -8
-1 -8
1 -8
And the graph can be seen in the image at the end.
How to complete the table?Here we have the function:
f(x) = -8
This is a constant function, for every input that we use, the output will be the same one, then:
f(-8) = -8
f(-1) = -8
f(1) = -8
Then the complete table is:
x f(x)
-8 -8
-1 -8
1 -8
And the graph of these 3 points can be seen in the image at the end.
Learn more about constant functions at.
https://brainly.com/question/18102431
#SPJ1
A bag of shapes contains 4 red circles, 3 blue circles, and 9 yellow triangles. What is the probability of drawing a shape that is red or a circle?
Answers
The probability of drawing a shape that is red or a circle is 7/16 from the bag that contains 4 red circles, 3 blue circles, and 9 yellow triangles.
Number of red circles = 4
Number of blue circles = 3
Number of yellow triangles = 9
Total number of all items = 4+3+9 = 16
Thus, the total number of possible outcomes is 16.
The probability of getting a red shape = 4/16
The probability of getting a circle = 4/16 + 3/16 = 7/16
To calculate the probability of drawing an item circle or red color,
P(red or circle) = P(red) + P(circle) - P(red and circle)
P(red or circle) = 4/16 + 7/16 - 4/16
P(red or circle) = 7/16
Therefore, we can conclude that the probability of drawing a shape that is red or a circle is 7/16.
To learn more about Probability
https://brainly.com/question/30034780
#SPJ4
HELPPP- this maths question is confusing me

Answers
Jude has enough money to buy 30 calculator and 30 pens.
The cost of 2 calculators = $10.40
Here we have to use the unitary method
The cost of on calculator = 10.40/2
= $5.2
The cost of 3 pens = $3.54
The cost of one pen = 3.54/3
= $1.18
The total amount that she has = $200
The cost of 30 calculator = 30 × 5.2
= $156
The cost of 30 pens = 30 × 1.18
= $35.4
Total cost of 30 calculators and 30 pens = 156+35.4
= $191.4
Total cost of 30 calculators and 30 pen is $191.4 and she has $200
Hence, Jude has enough money to buy 30 calculator and 30 pens.
Learn more about unitary method here
brainly.com/question/22056199
#SPJ1
uke has blue and red balls. Every day, he wins 2 blue balls and loses 3 red ones. After 5 days, he has the same amount of blue as red. After 9 days, he has twice as many blues as reds. How many red balls did he have at the beginning? Question not Showing?
Answers
A. The number of red balls he had was 8 at the beginning.
Duke's starting red ball total can be found by setting up a system of equations. First, let x represent the number of red balls and y represent the number of blue balls.
After 5 days, the equation is x-15=y+10. This equation states that after 5 days, the number of red balls (x) minus 15 will equal the number of blue balls (y) plus 10. After 9 days, the equation is x-27=2y+20.
This equation states that after 9 days, the number of red balls (x) minus 27 will equal twice the number of blue balls (y) plus 20. To solve for x, both equations can be set equal to each other and solved. This results in x=8. Therefore, Duke had 8 red balls at the beginning.
For more questions like Equation click the link below:
https://brainly.com/question/29657992
#SPJ4
What is y=2/3x-5 in standard form?
Answers
9514 1404 393
Answer:
2x -3y = 15
Step-by-step explanation:
Add 5-y to put the variables on the same side of the equal sign. Then multiply by 3 to clear the fraction.
5 = 2/3x -y
15 = 2x -3y
2x -3y = 15 . . . . standard form
what are the extraneous variables eliminated by randomly selecting schools into the experiment and control groups?
Answers
Regression to the mean and selection bias are the superfluous variables that are removed by randomly choosing schools for the experiment and control groups.
A statistical phenomenon known as regression to the mean (RTM) states that if a random outcome of any measurement or event is severe in the first example, the second or following outcomes will be less extreme. In other words, it will be somewhat near to the distribution's mean or center.
According to regression to the mean (RTM), if an experiment's first result is extreme, the second result will be more in line with the population mean.
Decisions are made incorrectly as a result of this prejudice.
To mitigate the detrimental impacts of regression to the mean, organizations can exercise critical thinking and undertake a randomized controlled trial (RCT) with an experimental group and a control group.
Learn more about Regression :
brainly.com/question/14548066
#SPJ4
what is the solution for 15= -x +10
Answers
Answer:
-5
Step-by-step explanation:
15=-x+10
x-10=-15
x=-15+10
x=-5
Sam threw a baseball into the air and found that the height of the baseball followed
the equation f(x) = -16x² +24x + 4
What is the range of the function representing the baseball's height?
Answers
The range of the function representing the baseball's height is 4 ≤ f(x) ≤ 24.
What is equation?An equation is a mathematical statement that expresses the relationship between two or more quantities and usually contains one or more unknowns, represented by a letter or symbol. An equation is written in symbolic form and is used to solve a problem or to describe a physical law. Equations can be used to describe anything from simple arithmetic operations to complex scientific equations.
This is because the highest value of the function is f(x) = 24, which is the starting height of the ball before it is thrown, and the lowest value of the function is f(x) = 4, which is the height of the ball when it returns to the ground. Therefore, the range of the function is 4 ≤ f(x) ≤ 24.
To know more about Equation click-
https://brainly.com/question/2972832
#SPJ4
What is 427 thousand in scientific notation
Answers
Answer:
4.27x10^2
Step-by-step explanation:
3. A cone has a radius of 1.2 inches and a height of 2.9 inches. What is the volume, to the nearest tenth of a cubic inch of the cone?
A. 3.6 cubic inches.
B. 4.4 cubic inches.
C. 10.6 cubic inches.
D. 13.1 cubic inches
Answers
The Volume of the Cone is 4.4 Cubic Inches
What is Cone?
A cone is a three-dimensional geometric object with a smooth taper from a flat base to the tip or vertex. A cone is made up of a series of line segments, half-lines, or lines that link a common point, the apex, to all of the points on a base on a plane that does not contain the apex.
Solution:
Given:
Radius = 1.2 inches
Height = 2.9 inches
To Find: Volume fo the Cone
Volume of Cone = 1/3 * 3.14 * \(radius^{2} * height\)
= 1/3 * 3.14 * 1.2 * 1.2 * 2.9
= 4.37088
= 4.4 cubic inches
To learn more about Cone from the given link
https://brainly.com/question/26998273
#SPJ1
ASAP
200% of rabbits population (last year)
1,300 rabbits this year
how many rabbits were there last year??
13000 = ?
w ? Please answer in fraction form.
Answers
The number of rabbits last year is 1083.
What is a percentage?A percentage is a value or ratio that may be stated as a fraction of 100. If we need to calculate a percentage of a number, we should divide it's entirety and then multiply it by 100.
Let last year's population be x.
The information will be illustrated as:
x + (20% × x) = 1300
x + 0.2x = 1300
1.2x = 1300
Divide
x = 1300/1.2
x = 1083
The rabbits are 1083 last year.
Learn more about percentages on;
brainly.com/question/24304697
#SPJ1
There is a 20% increase in rabbits population from last year and 1,300 rabbits this year. How many rabbits were there last year??
Round 0.698 to the nearest hundredth.
PLZ PLZ HELP
Answers
Answer:
0.70
Step-by-step explanation:
The nine is in the hundreths place, and it is over five, so you would round the number to the left of it up (the six). Basically, ignore the 8.
The number 0.698, when rounded off to the nearest hundredth, will become 0.70.
For the Rounding, some number to a specific value is making its value, mostly done for better readability or accessibility.
We need to round 0.698 to the nearest hundredth.
The hundredth place in the number is 9, that is greater than or equal to 5.
Since, we round up the tenths place to 7 + 1 = 8 and drop all other digits after the hundredth place and replace them with zeros.
0.698 rounded to the nearest hundredth is 0.70.
Learn more about rounding numbers here:
brainly.com/question/1285054
#SPJ6