Find the common ratio of the geometric sequence 19, 171, 1539, ...

Answer:

The formula for ABC Triangle

(AB×AC)/2

= (4.7×2.4)/

= 11,28/2

= 5,64 in²

Answer:

A rational number is any number that can be written as a fraction, where both the numerator (the top number) and the denominator (the bottom number) are integers, and the denominator is not equal to zero.

Step-by-step explanation:

In other words, a rational number can be expressed as p/q, where p and q are both integers and q ≠ 0.

An integer is a number that is not a fraction. So basically, a whole number.

Hope this helped!

Any integer that can be expressed in the form p/q, with q≠0, is referred to as a rational number in mathematics.

What are integers?

The Latin word "Integer," which means entire or intact, is where the word "integer" initially appeared. Zero, positive numbers, and negative numbers make up the specific class of numbers known as integers.

Any number that can be expressed as a fraction and has an integer denominator that is not zero and both the numerator and the numerator are integers is considered to be rational.

To put it another way, a rational number can be written as p/q, where p and q are both integers and q≠0.

Hence, rational number is any integer that can be expressed in the form p/q, with q≠0.

Learn more about integers from the given link

https://brainly.com/question/929808

#SPJ4

Rigoberto invests $8,000, at 6% interest, compounded semiannually for 1 year the compound interest for his investment is 8487.2

A = P(1 + r/n)²

In the formula

A = Accrued amount (principal + interest)

P = Principal amount = 8000

r = Annual nominal interest rate as a decimal = 0.06

R = Annual nominal interest rate as a percent

r = R/100

n = number of compounding periods per unit of time

t = time in decimal years;

A = 8000(1 + 0.06/2)²

= 8000(1 + 0.03)²

= 8000(1.0609)

= 8487.2

Therefore, the compound interest for his investment is 8487.2

To learn more about compound interest refer here

https://brainly.com/question/24274034

#SPJ4

The triangles that are congruent to (a) ΔCDE, and (b) ΔCDF include ΔBFG, ΔADG, ΔBDE, and  ΔABH, ΔBHCΔ, ΔGHE respectively

We should know that when two or more objects in geometry have the same shape and size, or if one has the same shape and size as the mirror image of the other, they are congruent.

From the object drawn the congruent triangles to ΔCDE include the following

ΔBDF

ΔABG

ΔBDE

ΔABI

ΔACH

ΔBCJ

ΔIHJ

All the triangle has side, side, right angle

The triangles that are congruent to ΔCDF include the following

ΔABG

ΔBHC

HGE

These triangles have SIDE, SIDE, SIDE (SSS)

Read more about congruent triangles on https://brainly.com/question/12135679

#SPJ1

Answer:

6

Step-by-step explanation:

6 times 1/4 is equal to 6/4, which is 1 1/2 :)

Answer:

25 1/2 square meters remaining

Step-by-step explanation:

Total area of the pool deck=76 1/2 square meters

He painted 2/3 of the deck before stopping for lunch

Total painted=2/3 of 76 1/2

=2/3*153/2

=306/6

=51 square meters

Total remaining=Total area - total painted area

=76 1/2 - 51

=153/2 - 51

=153-102/2

=51/2

=25 1/2 square meters remaining

Answer:

x= 2.18265833

Step-by-step explanation:

is this for solve for x, because i got decimal if it was solve for x

3^x=4+7

3^x=11

In (3^x)=In (11)

xIn(3)=In (11)

x= In (11)/ In (3) Divide

X= 2.18265833

The probability of landing heads exactly 11 times when a coin is tossed 20 times is option a) 0.1602

The repeated tossing of a coin follows a binomial distribution

P(X = x) = ⁿCₓ pˣ (1 - p)⁽ⁿ ⁻ ˣ⁾

where,

n = No. of times the experiment was repeated

x = random variable defining the number of "successes"

p = probability of "success"

Here

"succeess" is the event of landing a head.

n = 20

x = no. of times heads should show, i.e 11

p = probability of landing a head in a single toss

= 1/2

Hence, putting all this in the formula above we get

P(X = 11) = ²⁰C₁₁ 0.5¹¹ (1 - 0.5)⁽²⁰ ⁻ ¹¹⁾

= ²⁰C₁₁ 0.5¹¹ 0.5⁹

= ²⁰C₁₁ 0.5²⁰

= 20!/ 11! (20 - 11)! X 0.5²⁰

= a) 0.1602

Complete Question

An unbiased coin is tossed 20 times.

Find the probability that the coin lands heads exactly 11 times

a. 0.1602

b. 0.5731

c. 0.2941

d. 0.1527

e. 0.6374

To learn more about probability visit

https://brainly.com/question/12844710

#SPJ4

Answer:

Step-by-step explanation:

according to the question

2x+2+2x+2+3x+1=180

solve:

given:

M∠R=3x+1

substitute the value of x

3×25+1

75+1

76

Answer:

M∠R=76°

Step-by-step explanation:

First, I realized that ∠S was equal to ∠T, meaning ∠S=2x+2. I then added all 3 angles together and set them equal to 180, seeing the angles of a triangle must add up to 180 (2x+2+2x+2+3x+1=7x+5=180)

After that, I subtracted 5 from 180 and then divided by 7 to get x=25. I then solved the equation 3x+1 by plugging 25 in for x and ended up with 76 as my final answer.

True. The f(x²) is also irreducible over R.

The statement is true. If a polynomial function f(x) is irreducible over the real numbers (R), it means that it cannot be factored into polynomials of lower degree with coefficients in R.

When we substitute x² for x in the polynomial f(x), we get f(x²). If f(x²) is reducible over R, it would mean that it can be factored into polynomials of lower degree with coefficients in R.

However, since f(x) is irreducible, it implies that f(x²) cannot be factored into polynomials of lower degree with coefficients in R.

Learn more about irreducible

brainly.com/question/31955518

#SPJ11

Answer:

1)  0.498

2.49/5 =0.498

2)0.4395

8.79/20=0.4395

Step-by-step explanation:

Step-by-step explanation:

To calculate the value of Ella's investment at the end of 2 years, we can use the formula for compound interest:

A = P(1 + r/n)^(nt)

where A is the final amount, P is the principal (initial amount), r is the annual interest rate as a decimal, n is the number of times the interest is compounded per year, and t is the time in years.

For the first year, we have:

P = £7000

r = 0.03 (3% as a decimal)

n = 1 (compounded annually)

t = 1 (one year)

Substituting these values into the formula, we get:

A₁ = £7000(1 + 0.03/1)^(1x1)

A₁ = £7210

Therefore, the investment is worth £7210 after one year.

For the second year, we have:

P = £7210 (the new principal after one year)

r = 0.015 (1.5% as a decimal)

n = 1 (compounded annually)

t = 1 (one year)

Substituting these values into the formula, we get:

A₂ = £7210(1 + 0.015/1)^(1x1)

A₂ = £7323.15

Therefore, the investment is worth £7323.15 at the end of two years.

Therefore, the value of Ella's investment at the end of 2 years is £7323.15.

Answer:

2 hours

Step-by-step explanation:

\(\frac{5}{10}\) = \(\frac{60}{x}\)  Cross multiply and solve for x

5x = 600  Divide both sides by 5

x = 120

This is 120 minutes.  120 minutes is equal to 2 hours.

Helping in the name of Jesus.

Answer:

2 hours

Step-by-step explanation:

If 5 pages in 10 minutes and 60 pages than 60/5, which is 12.  12*10=120 minutes.  120 minutes is equivalent to 2 hours.

a) The complex solution to the equation 1+2x = -5i+2 is x = -0.5-1.5i.

b) The complex solutions to the equation x² + 2x + 2 = 0 are x = -1 + i and x = -1 - i.

c) The complex solution to the equation x³ = -2 + 2i is x = 1 + i.

d) The complex solution to the equation x¹ = -i 22 1 is x = -i.

a) To solve the equation 1+2x = -5i+2, we rearrange it to isolate the variable x. Subtracting 2 from both sides gives 2x = -5i, and dividing by 2 yields x = -2.5i. Therefore, the complex solution is x = -0.5-1.5i.

b) For the equation x² + 2x + 2 = 0, we can apply the quadratic formula. Substituting the coefficients into the formula gives x = (-2 ± √(-4(1)(2))) / (2(1)). Simplifying further, we have x = (-2 ± √(-8)) / 2. Since the square root of a negative number is an imaginary number, we can express it as x = (-2 ± 2i√2) / 2. Dividing both the numerator and denominator by 2 gives x = -1 ± i√2. Hence, the complex solutions are x = -1 + i and x = -1 - i.

c) To solve x³ = -2 + 2i, we can start by finding the cube root of both sides. The cube root of -2 + 2i is equal to the cube root of its magnitude times the cube root of the complex number itself. The magnitude of -2 + 2i is √((-2)² + 2²) = √8 = 2√2. The cube root of -2 + 2i can be expressed as 2√2 (cos(θ) + i sin(θ)), where θ is the angle whose tangent is 2/(-2) = -1. Therefore, θ = -π/4. The cube root of -2 + 2i is 2√2 (cos(-π/4) + i sin(-π/4)), which simplifies to 2√2 (-√2/2 - i√2/2). The final solution is x = 2√2 (-√2/2 - i√2/2) = -2 - 2i.

d) The equation x¹ = -i 22 1 is equivalent to x = -i. Therefore, the complex solution is x = -i.

Learn more about complex solutions here:

https://brainly.com/question/29070036

#SPJ11

Answer:

use symbolab

Step-by-step explanation:

it works

Answer:

m=4

Step-by-step explanation:

6.33 × 10^9 / 1.79 × 10^5=3.54 × 10^m

Find m

6.33 × 10^9 / 1.79 × 10^5 =3.54 × 10^m

Divide the multipliers

6.33/1.79

=3.54

Divide the other multipliers

10^9/10^5

Division in scientific notation with superscript with the SAME base requires subtraction of the superscript using one of the bases

10^9/10^5

= 10^9-5

=10^4

Therefore,

6.33 × 10^9 / 1.79 × 10^5 = 3.54 × 10^4

m = 4

Answer:

4 :)

Step-by-step explanation:

Therefore , the solution of the given problem of function comes out to be there is a 0.061071 absolute difference between the integral's approximate value and its real value.

There will be questions on design, arithmetic, each subject, and both real and imagined locations on the midterm exam. An account of the relationships between different elements that combine to create the same result. A service is composed of numerous distinctive components that work in tandem to produce distinctive results for each input.

Here,

We must divide the interval [1, 0] into two subintervals of equal length in order to use a midway Riemann sum with two intervals of equal length:

=> [1, 1/2] and [1/2, 0]

Each subinterval's breadth is given by x = (1-0)/2 = 1/2, and its midpoint is given by

=>x1 = (1 + 1/2)/2 = 3/4 and x2 = (1/2 + 0)/2 = 1/4

Therefore, the midway Riemann sum is:

=> F(x1,x) + F(x2,x)

=>  (3/4)^2 * e^(-3/4) * 1/2 + (1/4)^2 * e^(-1/4) * 1/2

=> 0.099532

The exact discrepancy between this estimate and the true integral value is:

=> |0.160603 - 0.099532| = 0.061071

As a result, there is a 0.061071 absolute difference between the integral's approximate value and its real value.

To know more about function visit:

https://brainly.com/question/28193995

#SPJ1

The measure of ∠O in ΔMNO is approximately 41.5°.

To find the measure of ∠O, we can use the Law of Cosines in ΔMNO, with sides M = 540 inches, N = 330 inches, and O = 600 inches. The Law of Cosines states:

O² = M² + N² - 2MN * cos(∠O)

Rearrange the equation to solve for cos(∠O):

cos(∠O) = (M² + N² - O²) / (2MN)

Substitute the values:

cos(∠O) = (540² + 330² - 600²) / (2 * 540 * 330)

cos(∠O) ≈ -0.7944

Now, find the angle using the inverse cosine function:

∠O ≈ arccos(-0.7944) ≈ 141.5°

Since ∠O is an obtuse angle, we need to find its supplement to the nearest 10th:

180° - 141.5° ≈ 38.5°

Thus, the measure of ∠O is approximately 38.5°.

To know more about Law of Cosines click on below link:

https://brainly.com/question/30766161#

#SPJ11

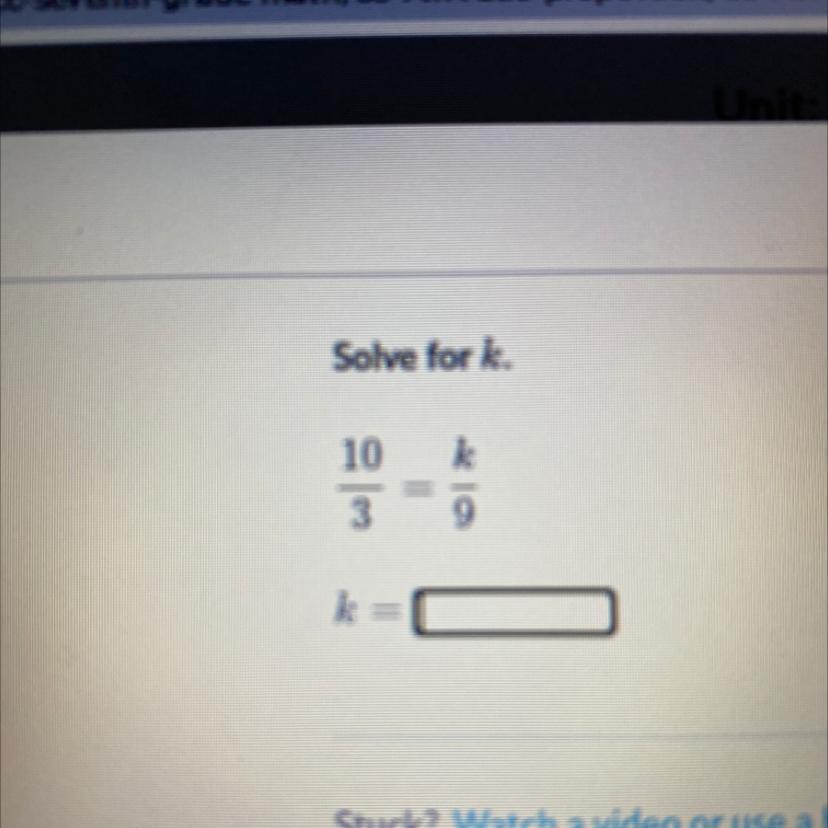
Answer:

Step-by-step explanation:

k/9=10/3 multiply both sides by 9

k=90/3

k=30

Answer:

Hello

\( \frac{10}{3} = \frac{k}{9} \\ 10 \times 9 = 3k \\3 k = 90 \\ k = 90 \div 3 \\ k = 30\)

hope it helps

Have a nice day

Answer:760

Step-by-step explanation:

Answer:

760

Step-by-step explanation:

Answer:

non function

Step-by-step explanation:

A function is a process or a relation that associates each element x of a set X, the domain of the function, to a single element y of another set Y (possibly the same set), the codomain of the function. so that is not a function

(a) Without more information, we cannot determine the initial number of insects. (b) The differential equation satisfied by P(t) is: dP/dt = kP, where k is the growth rate of the insect population.

(c) To find the time it takes for the population to double, we can use the formula:

2P(0) = P(0)e^(kt)

where P(0) is the initial population size. Solving for t, we get:

t = ln(2)/k

(d) Without more information, we cannot determine the time at which the population will equal a certain value.
Hi! To answer your question, I need the specific function P(t). However, I can provide you with a general framework to answer each part of your question once you have the function.

(a) To find the initial number of insects, evaluate P(t) at t=0:
P(0) = [Insert the function with t=0]

(b) To find the differential equation satisfied by P(t), differentiate P(t) with respect to t:
dP(t)/dt = [Insert the derivative of the function]

(c) To find the time at which the population doubles, first determine the initial population, P(0), then solve for t when P(t) is twice that value:
2*P(0) = P(t)
Solve for t: [Insert the solution for t]

(d) To find the time at which the population equals a specific value (let's call it N), set P(t) equal to N and solve for t:
N = P(t)
Solve for t: [Insert the solution for t]

Once you have the specific function P(t), you can follow these steps to find the answers to each part of your question.

Learn more about population here : brainly.com/question/27991860

#SPJ11

Answer:

ok so when it shows something like this, also what is 45 divided by 15? it's pretty obvious to see because add this 15+15+15=45 so, three would be the answer that you need or think of it this way when it says when y=45 you about dividing the the 2 numbers to your answer

Step-by-step explanation:

Hope that I could you

have a great day :)

Answer:

angle y = 60

Step-by-step explanation:

They are equal due to the rule that vertical angles are always equal

"A pair of vertically opposite angles are always equal to each other."

hope this helps

Answer:A

Step-by-step explanation:

20/25 = 4/5

16/20 = 8/10 = 4/5

Answer:

It is 63 or 63/1. Billy used change in x over change in y, and the correct formula for slope is the change in y over the change in x.

He might have done it backwards.

1/63

and could be 63 miles in 1 hour

63/1.

Because the graph looks good. (i think)

Rewriting the integral f (x,y,z) dz dy dx as an iterated integral, the integral is - ∫(from -1 to 1) ∫(from 0 to 1-y) ∫(from x^2 to 1-z) f(x, y, z) dy dz dx.

To rewrite the integral f(x, y, z) dz dy dx as an iterated integral in the order dx dy dz and dy dz dx, with given limits, follow these steps:

For dx dy dz:

1. Identify the limits for x: -1 to 1

2. Determine the limits for y: x^2 to 1 (from the given limits)

3. Determine the limits for z: 0 to 1-y (from the given limits)

Therefore, ∫(from -1 to 1) ∫(from x^2 to 1) ∫(from 0 to 1-y) f(x, y, z) dx dy dz

For dy dz dx:

1. Identify the limits for x: -1 to 1

2. Determine the limits for z: 0 to 1-y (from the given limits)

3. Determine the limits for y, keeping in mind that y goes from x^2 to 1:
  - For z, solve 1-y = z, which gives y = 1-z
  - So, y goes from x^2 to 1-z

Therefore, ∫(from -1 to 1) ∫(from 0 to 1-y) ∫(from x^2 to 1-z) f(x, y, z) dy dz dx

To know more about integrals refer here :

https://brainly.com/question/31477896#

#SPJ11

Answer:

I think it's 8 because 1 is added to 2

Answer:

3:10.5

Step-by-step explanation:

3/2 = 1.5. Therefore, you have to multiply 2 and 7 by 1.5 to find the equivalent ratio.

Using the Secant method with initial guesses p0 = -0.5 and p1 = -0.8, the solution of the equation m(x) = 2sin(x) accurate within 10^-1 is approximately 1.5211.

This method was chosen because it is an iterative numerical method that provides a good approximation of the root without requiring the derivative of the function.

The Secant method is an iterative numerical method used to find roots of equations. It is similar to the Newton-Raphson method but does not require the computation of the derivative.

To apply the Secant method, we start with two initial guesses, p0 and p1, which are -0.5 and -0.8 in this case. We then iterate using the formula:

p_{n+1} = p_n - f(p_n) * (p_n - p_{n-1}) / (f(p_n) - f(p_{n-1})),

where p_n and p_{n-1} are the current and previous approximations, and f(p_n) is the function value at p_n.

In this case, the function m(x) = 2sin(x) is given, and we need to find a solution accurate within 10^-1. We start with initial guesses p0 = -0.5 and p1 = -0.8.

We then evaluate the function at p0 and p1 to get f(p0) = 2sin(-0.5) and f(p1) = 2sin(-0.8).

Next, we substitute the values into the Secant formula to compute p2:

p2 = p1 - f(p1) * (p1 - p0) / (f(p1) - f(p0)).

We repeat this process until we reach a desired level of accuracy or convergence.

Using this method, we find that the solution accurate within 10^-1 is approximately 1.5211.

The Secant method is chosen in this case because it does not require the derivative of the function, making it suitable when the derivative is difficult to compute. Additionally, it provides a good approximation of the root even with initial guesses that are not as close to the actual root. Therefore, it is a suitable choice for finding the solution of the given equation accurate within 10^-1.

Learn more about Secant method here:

brainly.com/question/32599965

#SPJ11

Answer:

18

Step-by-step explanation: