Find the absolute minimum and maximum value of the function: f(x) = 2 2 sin(z) over the interval of [5, 21 27 2 Absolute maximum value: Absolute minimum value:
Answers
The absolute maximum value of f(x) is f(5) ≈ 1.284, and the absolute minimum value is f(21) ≈ -0.026.
To find the absolute minimum and maximum value of the function f(x) = 2sin(x) over the interval [5, 21], we need to follow these steps:
1. Identify critical points by finding where the derivative is zero or undefined.
2. Evaluate the function at the critical points and endpoints of the interval.
3. Compare the function values to determine the absolute maximum and minimum.
Step 1:
The derivative of f(x) = 2sin(x) is f'(x) = 2cos(x). To find critical points, set f'(x) to 0:
Taking the derivative of f(x) with respect to x, we get:
2cos(x) = 0
cos(x) = 0
Setting f'(x) equal to zero to find the critical points, we get:
4x*cos(x^2) = 0
The solutions within the interval [5, 21] are x = (3π)/2 and x = (7π)/2.
Step 2:
Evaluate the function at the critical points and endpoints of the interval:
Next, we need to check the endpoints of the interval, which are x = 5 and x = 21. Evaluating f(x) at these endpoints, we get:
f(5) ≈ -1.9178
f(21) ≈ 1.9764
f((3π)/2) = 2sin((3π)/2) = -2
f((7π)/2) = 2sin((7π)/2) = 2
Step 3:
Compare the function values:
\Absolute maximum value: 2 at x = (7π)/2
Absolute minimum value: -2 at x = (3π)/2
Learn more about Minimum:
brainly.com/question/21426575
#SPJ11
Related Questions
At a local manufacturing plant, employees must complete new machine set ups within 30 minutes. New machine set-up times can be described by a normal model with a mean of 22 minutes and a standard deviation of four minutes.
The typical worker needs five minutes to adjust to their surroundings before beginning their duties. What percent of new machine set ups are completed in less than 25 minutes?
A. Approximately 25%
B. Approximately 68%
C. Approximately 22.7%
D. Approximately 77,3%
Answers
The correct option is (D). Approximately 77.3% of new machine set ups are completed in less than 25 minutes.
Given a local manufacturing plant, employees must complete new machine set ups within 30 minutes.
The new machine set-up times can be described by a normal model with a mean of 22 minutes and a standard deviation of four minutes. The typical worker needs five minutes to adjust to their surroundings before beginning their duties.
To find the percentage of new machine set ups completed in less than 25 minutes, we need to calculate the z-score. For this, we will use the formula:
z = (X - μ) / σ
where X = 25 minutes, μ = 22 minutes, and σ = 4 minutes
z = (25 - 22) / 4z = 0.75
We can now look up the percentage of the area under the normal distribution curve that corresponds to z = 0.75. Using a standard normal distribution table, we find that the area to the left of z = 0.75 is approximately 0.7734.
So, the percentage of new machine set ups completed in less than 25 minutes is approximately 77.34%.
Therefore, the correct option is (D).Approximately 77.3% of new machine set ups are completed in less than 25 minutes.
Learn more about normal distribution curve here:
https://brainly.com/question/30783928
#SPJ11
IS IT 3/4 PLSS HELPP

Answers
Answer:i think its 3/4
Step-by-step explanation:
Answer:
im pretty sure it is 3/4
Step-by-step explanation:
Find the probability that a male college student from the group chose "housework" as their most likely activity on Saturday mornings? (Round to the nearest thousandth)
Answers
The probability that a male college student from the group chose "housework" as their most likely activity on Saturday mornings is 0.1, or 10% (rounded to the nearest thousandth).
The probability that a male college student from the group chose "housework" as their most likely activity on Saturday mornings depends on the available data or information provided. Without specific data or information on the preferences or behaviors of male college students in the group, it is not possible to determine the exact probability.
To calculate the probability, we would need to know the total number of male college students in the group and the number of male college students who chose "housework" as their most likely activity on Saturday mornings.
Let's assume there are 100 male college students in the group, and out of those, 10 male college students chose "housework" as their most likely activity on Saturday mornings.
The probability can be calculated as the ratio of the number of male college students who chose "housework" to the total number of male college students in the group:
Probability = Number of male college students who chose "housework" / Total number of male college students in the group
Plugging in the values, we get:
Probability = 10 / 100 = 0.1
Therefore, the probability that a male college student from the group chose "housework" as their most likely activity on Saturday mornings is 0.1, or 10% (rounded to the nearest thousandth).
To learn more about probability here:
brainly.com/question/30034780#
#SPJ11
2(x-7)-5x=13
how do i solve this
Answers
Answer:
x=-9
Step-by-step explanation:
2(x-7)-5x=13
Remove the parenthesis
2x-14-5x=13
Collect like terms
-3x - 14 = 13
Move the constant to the right
-3x=13+14
Calculate
-3x=27
Divide on both sides to get the answer
y is directly proportional to the square root of ( x-3 ). When x = 28, y = 20. Find y when x = 39.
Answers
Answer:
24Step-by-step explanation:
y is directly proportional to the square root of ( x-3):
y = k\(\sqrt{x-3}\), where k is the coefficient of proportionalityGiven:
y is 20 when x is 28Find k:
20 = k\(\sqrt{28-3}\)20 = k* 5k = 20/5k = 4Find y when x = 39:
y = 4\(\sqrt{39-3}\)y =4\(\sqrt{36}\)y = 4*6y = 24Answer:
y = 24
Step-by-step explanation:
Given y is directly proportional to \(\sqrt{x-3}\) then the equation relating them is
y = k\(\sqrt{x-3}\) ← k is the constant of proportion
To find k use the condition when x = 28, y = 20 , then
20 = k × \(\sqrt{28-3}\) = k × \(\sqrt{25}\) = 5k ( divide both sides by 5 )
4 = k
y = 4 \(\sqrt{x-3}\) ← equation of proportion
When x = 39 , then
y = 4 × \(\sqrt{39-3}\) = 4 × \(\sqrt{36}\) = 4 × 6 = 24
In preparing to construct a one‐sample t interval for a population mean, suppose we are not sure if the population distribution is Normal. In which of the following circumstances would we not be safe constructing the interval based on an SRS of size 24 from the population?
i. A stemplot of the data is roughly bell‐shaped.
ii. A histogram of the data shows slight skewness.
iii. A stemplot of the data has a large outlier.
iv. The sample standard deviation is large.
v. The t procedures are robust, so it is always safe.
Answers
We would not be safe constructing the interval based on an SRS of size 24 from the population if the sample data exhibits a strong departure from normality.
Under what circumstances would it be unsafe to construct a one-sample t interval based on an SRS of size 24?Constructing a one-sample t interval assumes that the population distribution is approximately normal. However, if the sample data shows a significant departure from normality, it would be unsafe to rely on the t interval. In such cases, alternative approaches or non-parametric methods may be more appropriate for estimating the population mean.
When the sample size is large, the Central Limit Theorem allows for a certain degree of departure from normality. However, with a small sample size of 24, if the data is heavily skewed, exhibits strong outliers, or deviates significantly from a normal distribution, the assumptions underlying the t interval may not hold. In these situations, using the t interval would not provide reliable or valid inferences about the population mean.
Learn more about assumptions of the one-sample t interval
brainly.com/question/31524117
#SPJ11
What is the volume of a sphere with a diameter of 8.6 m, rounded to the nearest
tenth of a cubic meter?
Answers
How can the tiles be sorted? (I need it quick please)

Answers
Answer:
by fraction and decimals
Step-by-step explication:
I need help fast plss

Answers
The measures of the angles are ∠1 = 127°, ∠2 = 53°, ∠3 = 127°, ∠4 = 37°, ∠5 = 53°, ∠6 = 90°, ∠7 = 37°, ∠8 = 143°, ∠9 = 37° and ∠10 = 143°
Finding the measures of the anglesFrom the question, we have the following parameters that can be used in our computation:
The transversal lines and the other lines
So, we have
∠1 = 180 - 53
Evaluate
∠1 = 127°
Also, we have
∠5 = 53°
By vertical angles, we have
∠2 = 53°
∠3 = 127°
Next, we have
∠4 = 127 - 90°
∠4 = 37°
Solving further, we have
∠6 = 90°
By corresponding angles, we have
∠7 = 37°
∠9 = 180 - 90 - 53°
∠9 = 37°
∠10 = 90 + 53°
∠10 = 143°
∠8 = 143°
Read more about angles at
https://brainly.com/question/28293784
#SPJ1
Please help me I need to finish this :)

Answers
Anyone know how to solve this? I have kept getting the same irrational number for the past 10 minutes.

Answers
Answer: 151/14
Step-by-step explanation:
6x+13+4x+16+4x=180
14x + 29 = 180
14x = 151
x = 151/14
What is the equation of the line that passes through the point (-1,4) and has a slope of -5?
Answers
Answer:
y = -5x + 4
Step-by-step explanation:
In the equation y = mx + b , m stands for slope and b stands for the y intercept. In the coordinates (-1,4) the y value is 4 meaning that it's your y-intercept. The slope is already given to you so just add the X.
Answer:
y = -5x - 1
Step-by-step explanation:
Since you have the slope and a given point you can use the point-slope formula to solve for the y-intercept formula.
Point slope formula = y - y = m(x - x)
m = -5 (-1, 4)
y - 4 = -5(x +1)
y - 4 = -5x - 5
y = -5x - 1
There are 30 giraffe and 6 penguin at the zoo. Which tatement correctly compare the two quantitie?
Answers
To compare the two quantities, divide the number of giraffes by the number of penguins There are 5 times as many giraffes as penguins at the zoo.
To compare the two quantities, you need to divide the number of giraffes by the number of penguins.
30 giraffes / 6 penguins = 5
This means that there are 5 times as many giraffes as penguins at the zoo.
There are 5 times as many giraffes as penguins at the zoo. To compare the two quantities, divide the number of giraffes by the number of penguins (30/6 = 5). This means that there are 5 times more giraffes than penguins at the zoo.
Learn more about number here
https://brainly.com/question/10547079
#SPJ4
Gracie has six dollars to buy a gallon of milk and some apples. The gallon of milk cost $3.59 and the apples cost $.60 each. Which any inequality could she use to find out how many apples she can buy?
Answers
Answer: 4
Step-by-step explanation:
Let the number of gallon milk cartons be M and the number of apples be N. The total cost would be 3.59M + 0.60N <= 6.00. If M = 1, then
3.59 + 0.60N <= 6.00
0.60N <= 2.41
N <= 4 [2.40, with 0.01 left over]
A plumber charges a flat fee of $77 to visit a home and examine a clogged drain. The plumber charges an additional $21 per hour spent fixing the drain. The
total cost, C (in dollars), for fixing a drain that takes h hours is given by the following.
C=77+21h
Answer the following questions.
(a) What is the total cost for fixing a drain that takes 6 hours?
(b) If the plumber charged a total of $329, how many hours did she spend
fixing the drain?
Answers
(a) 77+21(6) = $203
(b) 329 = 77 + 21h means 252 = 21h, and thus h = 12 hours
im so lost im in algebra 1 and i feel so slow
Answers
Answer:
Hey but you get better at in no time lol
Step-by-step explanation:
no need to worry
James takes out a loan of 9000 euros which keeps on charging simple interest at a rate of 3% of the original amount per annum until it is cleared. James pays of 770 euros each year to reduce the loan. After how many years will James have fully cleared the loan?
Answers
James will fully clear the loan after approximately 12 years when the remaining balance reaches zero.
To determine the number of years it will take for James to fully clear the loan, we need to calculate the remaining balance after each payment and divide the initial loan amount by the annual payment until the remaining balance reaches zero.
The loan amount is 9000 euros, and James pays off 770 euros each year. Since the interest is charged at a rate of 3% of the original amount per annum, the interest for each year will be \(0.03 \times 9000 = 270\) euros.
In the first year, James pays off 770 euros, and the interest on the remaining balance of 9000 - 770 = 8230 euros is \(8230 \times 0.03 = 246.9\)euros. Therefore, the remaining balance after the first year is 8230 + 246.9 = 8476.9 euros.
In the second year, James again pays off 770 euros, and the interest on the remaining balance of 8476.9 - 770 = 7706.9 euros is \(7706.9 \times 0.03 = 231.21\) euros. The remaining balance after the second year is 7706.9 + 231.21 = 7938.11 euros.
This process continues until the remaining balance reaches zero. We can set up the equation \((9000 - x) + 0.03 \times (9000 - x) = x\), where x represents the remaining balance.
Simplifying the equation, we get 9000 - x + 270 - 0.03x = x.
Combining like terms, we have 9000 + 270 = 1.04x.
Solving for x, we find x = 9270 / 1.04 = 8913.46 euros.
For more such questions on loan
https://brainly.com/question/25696681
#SPJ8
What is the surface area of a cylinder with base radius 3 and height 6?
Either enter an exact answer in terms of 7 or use 3.14 for at and enter your answer as a decimal.
3
6
square units

Answers
Answer:
Your correct answer is 169.65 square units.
Step-by-step explanation:
Since the radius of the cylinder is 3, and the height of it is 6, you get a total of 169.65 square units.
Here is proof that I am correct:

Answer:
54 pi
Step-by-step explanation:
khan
find the median of these numbers 5,9,13,7,5,7
Answers
Answer:
The mean is 7
Step-by-step explanation:
5,5,7,7,9,13
9-7 is going to get 7
customers arrive at a post office with a single server. fifty percent of the customers buy stamps, and take 2 minutes to do so. twenty percent come to pick up mail and need 3 minutes to do so. the rest take 5 minutes. assuming all these times are deterministic and that the arrivals form a poisson process with a rate of 18 per hour, compute the expected number of customers in the post office in steady state
Answers
the expected number of customers in the post office in steady state is 13.16.
We can use the M/M/1 queue model to solve this problem. Since customers arrive at a Poisson rate of 18 per hour, the arrival rate is λ = 18/60 = 0.3 customers per minute. The service times are deterministic, so we can use the mean service time to calculate the service rate. The mean service time is:
(0.5 x 2) + (0.2 x 3) + (0.3 x 5) = 3.1 minutes
So the service rate is μ = 1/3.1 = 0.3226 customers per minute.
The utilization of the system is ρ = λ/μ = 0.3/0.3226 = 0.929. Since the system is stable, the expected number of customers in the system in steady state is:
L = ρ/(1 - ρ) = 0.929/(1 - 0.929) = 13.16 customers
To learn more about service click here://brainly.com/question/30418810
#SPJ11
A population has a current size of 150. If λ is 1. 2, what will the expected population size be after two generations?.
Answers
The expected population size be after two generations which has a current size of 150 and λ of 1.2 is 216.
Nt = N₀ × λ^t
Nt = Population size at generation t
N₀ = Current population size
t = Number of generations
λ = Finite rate of increase
λ = 1.2
N₀ = 150
Nt = 150 × 1.2²
Nt = 150 × 1.44
Nt = 216
Population size is defined as the number of individuals present in a designated region. Finite rate of increase is the ratio of population size from increase from one year to the next.
Therefore, the expected population size be after two generations is 216
To know more about expected population size here:
brainly.com/question/20598565
#SPJ1
April shoots an arrow upward at a speed of 80 ft/sec from a platform of 25 ft. High. The pathway of the arrow can be represented by the equation h = -16t^2 + 80t + 25, where h is the height and t is the time in seconds. What is the maximum height that the arrow reaches
Answers
Answer:
125feet
Step-by-step explanation:
Given the equation that modeled the height expressed as h = -16t^2 + 80t + 25, where h is the height and t is the time in seconds.
The arrow reaches the maximum height at dh/dt = 0
dh/dt = -32t + 80
0= -32t+80
32t = 80
t = 80/32
t = 2.5secs
substitute t = 2.5 into the formula;
h = -16t^2 + 80t + 25
h = -16(2.5)^2 + 80(2.5) + 25
h = -16(6.25)+225
h = -100+225
h = 125
Hence the maximum height the arrow reaches is 125feet
Devise an algorithm that finds all terms of a finite sequence of integers that are greater than the sum of all
previous terms of the sequence.
Answers
This algorithm efficiently finds all terms in a finite sequence of integers that are greater than the sum of all previous terms in the sequence.
To devise an algorithm that finds all terms of a finite sequence of integers that are greater than the sum of all previous terms of the sequence, follow these steps:
Start by defining the input sequence as a list or an array of integers. Let's call it `sequence`.
Initialize an empty list or array called `results` to store the terms that meet the condition.
Initialize a variable called `sum_of_previous_terms` and set its value to 0. This variable will be used to store the sum of all previous terms in the sequence as we iterate through it.
Loop through the sequence using a for loop, comparing each term with the sum of all previous terms.
a. For each term in the sequence, compare it with the value of `sum_of_previous_terms`.
b. If the term is greater than `sum_of_previous_terms`, add it to the `results` list or array.
c. Update the value of `sum_of_previous_terms` by adding the current term to it.
Continue the loop until all terms in the sequence have been checked.
After the loop is completed, return the `results` list or array, which contains all the terms that meet the condition.
Here is the algorithm in a more concise form:
Input: `sequence` (a list or array of integers)
Initialize: `results` (an empty list or array), `sum_of_previous_terms` (0)
For each term in `sequence`:
a. Compare the term with `sum_of_previous_terms`
b. If term > sum_of_previous_terms, add term to `results`
c. Update `sum_of_previous_terms` with the current term
Return `results`
This algorithm efficiently finds all terms in a finite sequence of integers that are greater than the sum of all previous terms in the sequence.
for more questions on algorithm
https://brainly.com/question/24953880
#SPJ11
At the Middleton School festival, a tent covers a rectangular space 30 1/2 yards long and 9 1/3 yards wide. What is the area, in square yards, covered by the tent?
Answers
Answer:
284 2/3 square yardsStep-by-step explanation:
Area of rectangle:
A = lwA = 30 1/2 * 9 1/3 = 61/2 * 28/3 = 854/ 3 = 284 2/3 square yardsAnswer:
284 ²/₃ square yards
Step-by-step explanation:
Area of a rectangle
Area = length × width
Given:
length = 30 ¹/₂ yardswidth = 9 ¹/₃ yardsSubstitute the given values into the formula and solve for area:
\(\begin{aligned}\sf Area & = \left(30+\dfrac{1}{2}\right) \times \left(9+\dfrac{1}{3}\right)\\\\ & = \dfrac{30 \times2+1}{2} \times \dfrac{9 \times 3+1}{3}\\\\ & = \dfrac{61}{2} \times \dfrac{28}{3}\\\\& = \dfrac{61 \times 28}{2 \times 3}\\\\ & = \dfrac{1708}{6}\\\\ & = \dfrac{1704+4}{6}\\\\ & = \dfrac{1704}{6}+\dfrac{4}{6}\\\\ & =284\: \frac{2}{3}\:\:\sf square\:yards\end{aligned}\)
Therefore, the area covered by the tent is 284 ²/₃ square yards.
same price, d.
) Which model represents the situation?
d
d
10
d
19
.) What is the price of one drink?
d=
7
8
9
ex
4
5
6
Answers
Answer: ddddd
Step-by-step explanation:
Daniel took her dog for a walk. She decided to count how many dog ears. She passed on her walk. She passed 14 dogs. How many ears did she count?
Answers
Answer:
Each dog has 2 ears (unless a dog had genetic disorder)
So-
14 x 2
= 28
So she counted of 28 Ears of total
(honestly that's a weird thing to do but ok)
Answer:
14 x 2 = 28
Step-by-step explanation:
Each dog has 2 ears
14 dogs has 28 ears
If a quadrilateral has exactly one pair of congruent, opposite angles, then the quadrilateral is a ______
Answers
a model scale is 1 in. = 1.5 ft. if the actual object is 18 feet, how long is the model? a) 12 inches b) 16 inches c) 24 inches d) 27 inches
Answers
To find the length of the model, we need to use the given scale, which states that 1 inch on the model represents 1.5 feet in reality.
The length of the actual object is given as 18 feet. Let's calculate the length of the model:
Length of model = Length of actual object / Scale factor
Length of model = 18 feet / 1.5 feet/inch
Length of model = 12 inches
Therefore, the length of the model is 12 inches. Therefore, the correct option is (a) 12 inches.
Learn more about Length here :
https://brainly.com/question/29133107
#SPJ11
PLEASE I NEED TO FINISH THIS!!
name:
a chord
a diameter
a radius
a minor arc
and a semi circle of the circle
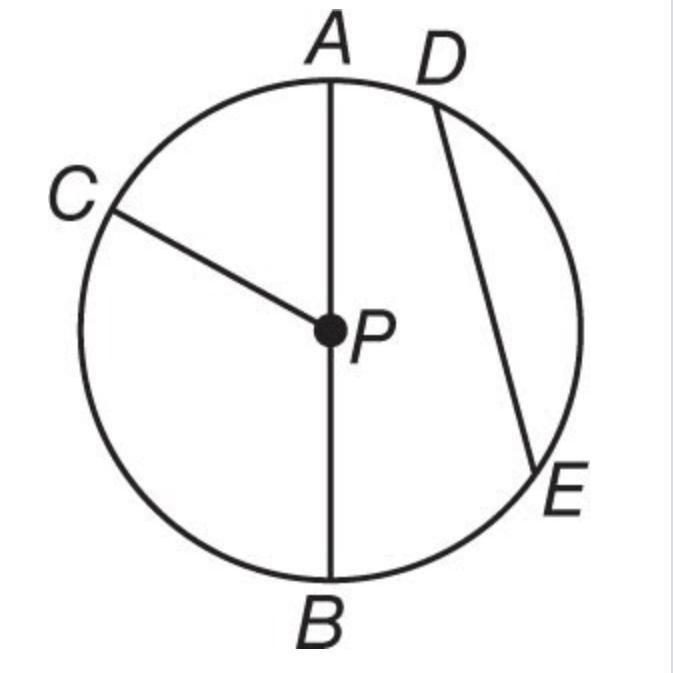
Answers
Ab diameter
Cp radius
Which expression is equivalent to 4 - (2m - 3)?
4 - 2m - 3
4- 2m + 3
-8m + 3
0-8m + 12
Answers
Answer:
4 - 2m + 3
Step-by-step explanation:
when you distribute the negative sign you will get this
Answer:
B on Edge
Step-by-step explanation: