Find dy/dx by implicit differentiation. 4 sin(x) + cos(y) = sin(x) cos(y) Step 1 We begin with the left side. Remembering that y is a function of x, we have [4 sin(x) + cos(y)] = - Dy'. dx
Answers
The derivative dy/dx is undefined for the given equation. To find dy/dx using implicit differentiation for the equation 4sin(x) + cos(y) = sin(x)cos(y).
We start by differentiating both sides of the equation. The left side becomes [4sin(x) + cos(y)], and the right side becomes -dy/dx.
To find the derivative dy/dx, we need to differentiate both sides of the equation with respect to x.
Starting with the left side, we have 4sin(x) + cos(y). The derivative of 4sin(x) with respect to x is 4cos(x) by the chain rule, and the derivative of cos(y) with respect to x is -sin(y) * dy/dx using the chain rule and implicit differentiation.
So, the left side becomes 4cos(x) - sin(y) * dy/dx.
Moving to the right side, we have sin(x)cos(y). Differentiating sin(x) with respect to x gives us cos(x), and differentiating cos(y) with respect to x gives us -sin(y) * dy/dx.
Thus, the right side becomes cos(x) - sin(y) * dy/dx.
Now, equating the left and right sides, we have 4cos(x) - sin(y) * dy/dx = cos(x) - sin(y) * dy/dx.
To isolate dy/dx, we can move the sin(y) * dy/dx terms to one side and the remaining terms to the other side:
4cos(x) - cos(x) = sin(y) * dy/dx - sin(y) * dy/dx.
Simplifying, we get 3cos(x) = 0.
Since cos(x) can never be equal to zero for any value of x, the equation 3cos(x) = 0 has no solutions. Therefore, the derivative dy/dx is undefined for the given equation.
Learn more about implicit differentiation:
https://brainly.com/question/11887805
#SPJ11
Related Questions
F of x equals the integral from 0 to x of the cosine of t squared . Use your calculator to find f '(1).
1. 0.709
2. 0.292
3. 0.842
4. 0.540
Answers
Answer:
First, if we have a function defined as:
\(G(x) = \int\limits^x_0 {f(t)} \, dt\)
then:
G'(x) = f(x)
\(F(x) = \int\limits^x_0 {cos(t)^2} \, dt\)
then:
F' = dF/dt = cos(t)^2
F'(1) = cos(1)^2 = 0.2919
If we round it up to the third digit after the decimal point we get:
F'(1) = 0.292
The correct option is the second one.
WILL MARK BRAINLIEST!!!
What is the boat’s velocity vector? PLEASE SHOW YOUR WORK AND INCLUDE ALL CORRECT UNITS!

Answers
Answer:
Magnitude: 23.4 kph
Direction: 50.2° below horizontal.
Step-by-step explanation:
15² + 18² = s²
s² = 549
s = 23.4
Θ = tan^-1 18/15
Θ = 50.2°
The velocity vector is the sum of the two vectors which is a vector that starts at the boat and goes until the lower tip of the vertical arrow.
Its magnitude is 23.4 kph, and its direction is 50.2° below horizontal.
Answer: boats vector is 23.45 kph, 50.2° south of east
Step-by-step explanation:
A vector has both direction and magnitude.
The vector for the boat is the shortest route from the boat to that end point which is a diagonal.
In order to find magnitude you use pythagorean
c²=a²+b²
m=15²+18²
m=√549
magnitude=23.45 kph
direction you would use tan∅
tan∅=opp/adj=18/15
∅= \(tan^{-1} \frac{18}{15}\)
∅=50.2
So the boats vector is 23.45 kph, 50.2° south of east

express the following limit as a definite integral: lim n→[infinity] n∑i=1 i6/n7=∫b1 f(x)dx
Answers
The given limit can be expressed as the definite integral: lim (n→∞) n ∑(i=1 to n) i⁶/n⁷ = ∫[1/n, 1] x⁶ dx
To express the given limit as a definite integral, we need to determine the appropriate function f(x) and the integration limits b and 1.
Let's start by rewriting the given limit:
lim (n→∞) (1/n) ∑(i=1 to n) \(i^6/n^7\)
Notice that the term i⁶/n⁷ can be written as (i/n)⁶/n.
Therefore, we can rewrite the above limit as:
lim (n→∞) (1/n) ∑(i=1 to n) (i/n)⁶/n
This can be further rearranged as:
lim (n→∞) (1/n^7) ∑(i=1 to n) (i/n)⁶
Now, let's define the function f(x) = x⁶, and rewrite the limit using the integral notation:
lim (n→∞) (1/n^7) ∑(i=1 to n) (i/n)⁶ = ∫[a,b] f(x) dx
To determine the integration limits a and b, we need to consider the range of values that x can take. In this case, x = i/n, and as i varies from 1 to n, x varies from 1/n to 1. Therefore, we have a = 1/n and b = 1.
Hence, the given limit can be expressed as the definite integral:
lim (n→∞) n ∑(i=1 to n) i⁶/n⁷ = ∫[1/n, 1] x⁶ dx
To learn more about definite integral visit:
brainly.com/question/32525875
#SPJ11
An initial population of 295 quail increases at an annual rate of 7%. Write an exponential function to model the quail population. What will the approximate population be after 2 year
Answers
Answer:
the answer is d just to the exam
The required population after 2 years is 337.75 quail.
What is an exponential function?The function which is in format f(x) =aˣ where a is constant and x is variable, the domain of this exponential function lies (-∞, ∞).
here,
An initial population of 295 quail increases at an annual rate of 7%. ,
the exponential growth of the population can be shown as,
y = 295[1 + 0.07]ˣ
Where x is the number of years and y is the population of that year.
Now put x = 2
y = 295[1.07]²
y = 337.75
Thus, the required population after 2 years is 337.75 quail.
Learn more about exponential function here:
brainly.com/question/15352175
#SPJ2
d. if a student was an undergraduate business major, what is the probability that the student intends to attend classes full-time in pursuit of an mba degree (to decimals)?
Answers
0.4
The probability that an undergraduate business major intends to attend classes full-time in pursuit of an MBA degree depends on the individual student's preferences and situation. Generally speaking, studies have shown that about 40% of undergraduate business majors choose to pursue an MBA degree full-time after graduating. Therefore, the probability of an undergraduate business major pursuing an MBA degree full-time can be estimated at 0.4.
Learn more about probability.
brainly.com/question/30034780
#SPJ11
Please Evaluate These Expressions:
1. 18 • (7+2²) ÷ 3 - 40 =
2. c² - y ÷ 2 =
Hint:
For Question two c = 6 and y = 16
Answers
1. -33.963333
2. 28
but i'm not sure
All of the following are measures of central tendency except the ________.
Select one:
A. None of the answers are correct
B. mode
C. range
D. mean
E. median
Answers
All of the following are measures of central tendency except the range.
The range is a measure of dispersion, representing the difference between the largest and smallest values in a dataset. It provides information about the spread or variability of the data but does not provide information about the central tendency.
On the other hand, measures of central tendency are statistical measures that aim to summarize the center or typical value of a dataset. They include the mode, median, and mean.
The mode represents the most frequently occurring value(s) in the dataset.
The median is the middle value when the data is arranged in ascending or descending order. It divides the dataset into two equal halves.
The mean is the arithmetic average of all the values in the dataset. It is calculated by summing all the values and dividing by the total number of values.
Therefore, option C, range, is not a measure of central tendency as it focuses on the spread or dispersion of the data rather than summarizing the central or typical value.
Learn more about central tendency from
https://brainly.com/question/1288901
#SPJ11
SOS I WILL VOTE BRAINLIEST IN YOUR ANSWER IS GOOD
y=0.182x+1.326
Use that equation to calculate how high the line will be when x=100
Answers
Answer:
×= 51
7
Step-by-step explanation:
Divide both sides of the equation by –0.182
As part of a back-to-school special, all calculators are 10% off. What is the sale price of a $28
calculator?
The sale price of the calculator is $
Answers
How long can someone shower to use only 10 gallons of water?
Answers
Answer:
4 minutes
Step-by-step explanation:
A standard showerhead uses 2.5 gallons a minute, or 25 gallons for 10 minutes, therefore 10/2.5 = 4.
a large cube is made up of 125 small cubes. in the figure shown below, three sides of the large cube are visible. how many small cubes have at least one of their sides visible from this view?
Answers
Answer:5566
Step-by-step explanation:
Define Torsion, pure torsion and it's assumptions, torsion
equation and limitation of its formula?
Answers
Torsion refers to the twisting of a structural member due to the application of torque. Pure torsion occurs when a structural member is subjected to torsional loading only. It is analyzed using assumptions such as linear elasticity, circular cross-sections, and small deformations. The torsion equation relates the applied torque, the polar moment of inertia, and the twist angle of the member. However, this formula has limitations in cases of non-circular cross-sections, material non-linearity, and large deformations.
Torsion is the deformation that occurs in a structural member when torque is applied, causing it to twist. In pure torsion, the member experiences torsional loading without any other external forces or moments acting on it. This idealized scenario allows for simplified analysis and calculations. The assumptions made in pure torsion analysis include linear elasticity, which assumes the material behaves elastically, circular cross-sections, which simplifies the geometry, and small deformations, where the twist angle remains small enough for linear relationships to hold.
To analyze pure torsion, engineers use the torsion equation, also known as the Saint-Venant's torsion equation. This equation relates the applied torque (T), the polar moment of inertia (J), and the twist angle (θ) of the member. The torsion equation is given as T = G * J * (dθ/dr), where G is the shear modulus of elasticity, J is the polar moment of inertia of the cross-section, and (dθ/dr) represents the rate of twist along the length of the member.
However, the torsion equation has its limitations. It assumes circular cross-sections, which may not accurately represent the geometry of some structural members. Non-circular cross-sections require more complex calculations using numerical methods or specialized formulas. Additionally, the torsion equation assumes linear elasticity, disregarding material non-linearity, such as plastic deformation. It also assumes small deformations, neglecting cases where the twist angle becomes significant, requiring the consideration of non-linear relationships. Therefore, in practical applications involving non-circular cross-sections, material non-linearity, or large deformations, more advanced analysis techniques and formulas must be employed.
To learn more about torsion click here: brainly.com/question/30612977
#SPJ11
How could i find the base?What is the volume of the cylinder?Approximate volume of cylinder in 3 decimals.
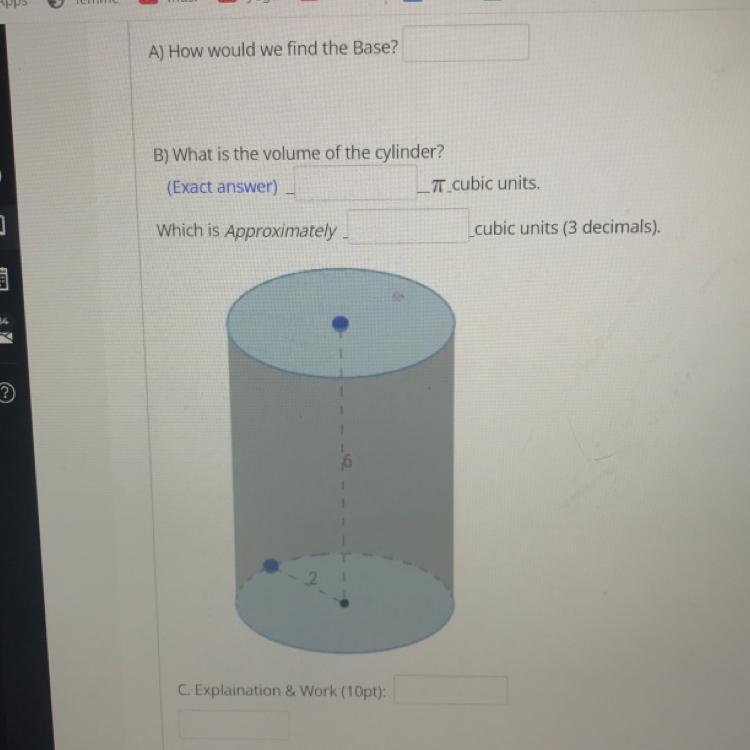
Answers
Given:
Here a cylinder is given with radius is 2 unit and height is 6 unit.
Required:
Find the base and volume.
Explanation:
The base of cylinder is circle and the area of circle is
\(A=\pi r^2=3.14*2*2=12.56\text{ unit}^2\)Now we have to find the volume of cylinder
\(V=\pi r^2h=2*2*6*\pi=24\pi\text{ unit}^3\)now put the value of
\(\pi=3.14\)so now volume of cylinder is
\(V=24*3.14=75.398\text{ unit}^3\)Final answer:
The base of cylinder is circle and the value of base is 12.56 square unit
Exact volume of cylinder is 24pi cubic unit and approximate volume of cylinder is 75.398 cubic unit
a boy travelled a distance of 10 km in 2 minutes calculate the speed of that boy
Answers
Step-by-step explanation:
(i hope this helps)
speed=distance/time taken

For the function g(x)=32/x+3, find (g^-1o g) (5)
Answers
The value of the composite function is found as 68/5.
What is meant as the inverse of the function?An inverse function is one that reverses the action of another function. A function g seems to be the inverse of a function f if and only if y=f(x) and x=g (y).The function is given as;
g(x) = 32/(x+3)
Write the function as 'y'
y = 32/(x+3)
Replace the value of x with y.
x = 32/(y+3)
Solve for y.
x(y + 3) = 32
xy = 32 - 3x
y = (32 - 3x)/x
Thus, g⁻¹ (x) = (32 - 3x)/x is the inverse of the function.
Now, find the value composite function; (g⁻¹ o g) (5).
(g⁻¹ o g) (5) = (32 - 3x)/x . 32/(x+3)
Put x = 5
(g⁻¹ o g) (5) = (32 - 15)/5 . 32/(5+3)
(g⁻¹ o g) (5) = 17×4/5
(g⁻¹ o g) (5) = 68/5
Thus, the value of the composite function is found as 68/5.
To know more about the inverse of the function, here
https://brainly.com/question/3831584
#SPJ1
Convert 77/9 into a mixed number.
Answers
Answer:
8 5/9
Step-by-step explanation:
9 goes into 77 8 whole times and then 5 is a leftover from 8. and 9 will stay the same so it will be 8 5/9
Answer:
\(8\frac{5}{9}\)
Step-by-step explanation:
77 ÷ 9 = 8.55555....8.55555... = \(8\frac{5}{9}\)I hope this helps!
11
?
Find the arc length of the semicircle.
Either enter an exact answer in terms of a or
use 3.14 fora and enter your answer as a
decimal.
units

Answers
Answer:
\(34.5575191895\)
Step-by-step explanation:
\(2\pi r(180/360)\\2\pi *11*1/2\\=11\pi\)
The arc length of the semicircle is half the circumference i.e., 11π.
What is circumference?The circumference is the perimeter of a circle.
Formula of Circumference = 2πr.
= Arc length of a semicircle
= half of the circle's circumference
= 2πr/2
= πr
=11r
Learn more about circumference here
https://brainly.com/question/4268218
#SPJ2
A plant that floats on the surface of rural bodies of water is known as duckweed. The initial mass of a population of duckweed is 500g The mass of the population triples every Ͷͷ hours and can be represented by the function m(t)= 500x3^t/45 where (t) is time in hours. Determine how many hours it would take for the duckweed to grow to a mass triple the original size.
Answers
Therefore , the solution of the given problem of function comes out to be the duckweed to grow a mass triple the original size will be 45 hours.
Explain function.The mathematics curriculum involves examining numbers, the equation the environment we live in, structures, and both actual and hypothetical locations. A function is a variable representation of the relationship between the quantities of intake and the corresponding outputs for each. Simply explained, a function is a collection of inputs that, when combined, provide unique outputs for each input.
Here,
Given :
mass of a population of duckweed is 500g
=> function m(t)= 500x³^t/45
So,
=> t = 45 hours
Since it is given that the mass of the population of the duckweed triples every 45 hours
it is clear that the time taken for the duckweed to grow a mass triple the original size will be 45 hours .
Therefore , the solution of the given problem of function comes out to be the duckweed to grow a mass triple the original size will be 45 hours.
To know more about function visit:
https://brainly.com/question/28193995
#SPJ4
h(x)=1-3x, domain= {1, 0, 1, 2}
Write the range of h using set notation. Then graph h.
Answers
The domain of the given function h(x) = 1 - 3x is {-2, 1, -2, -5}.
What are the domain and the range of a set of ordered pairs?The domain of a set of ordered pairs includes all possible x values of a function, and the range includes all possible y values of a set of ordered pairs.
The function is given in the question as:
h(x) = 1 - 3x ....(i)
Here domain= {1, 0, 1, 2}
The range includes all possible y values of a set of ordered pairs.
Substitute the values of the domain i.e. x = 1, 0, 1, 2 in the equation (i),
h(x) = 1 - 3(1)
h(x) = 1 - 3
h(x) = -2
h(x) = 1 - 3(0)
h(x) = 1
h(x) = 1 - 3(1)
h(x) = 1 - 3
h(x) = -2
h(x) = 1 - 3(2)
h(x) = 1 - 6
h(x) = -5
Therefore, the domain of the given function is {-2, 1, -2, -5}.
Learn more about the domain and the range here:
brainly.com/question/21027387
#SPJ1

Allen's score in a video game was changed by -75 points because he missed some targets. He got -15 points fro each missed target. How many targets did he miss?
Answers
BRIDGES The lower arch of the Sydney Harbor Bridge can be modeled by g(x) = - 0.0018 * (x - 251.5) ^ 2 + 118 where in the distance from one base of the arch and g(x) is the height of the arch. Select all of the transformations that occur in g(x) as it relates to the graph of f(x) = x ^ 2

Answers
The transformations to the graph of the quadratic function are given as follows:
The graph stretches vertically because of the multiplication by 3.The graph is translated left because x -> x + 7.The graph is translated down because y -> y - 6.What is a translation?A translation happens when either a figure or a function is moved horizontally or vertically on the coordinate plane.
The four translation rules for functions are defined as follows:
Translation left a units: f(x + a).Translation right a units: f(x - a).Translation up a units: f(x) + a.Translation down a units: f(x) - a.Additionally, when the function is multiplied by |a| > 1, it is said that the function is vertically stretched by a factor of a.
More can be learned about translations at brainly.com/question/28174785
#SPJ1
Problem Solving A London airport is 200 miles from Manchester airport. A plane leaves Manchester airport at 10 am to fly to the London airport. The plane flies at an average speed of 120 mph. What time does the plane arrive at the London airport?
Answers
The time the plane arrives at the London airport from the airport in Manchester, found using the kinematic equation for speed, and the conversion of the units from hours to minutes is 11:40 a.m.
What are the kinematic equations of motion?The kinematic equations describe the motion of an object that moves with constant acceleration.
Distance from the London airport to the Manchester airport, D = 200 miles
The average speed of the plane, v = 120 mph
Time the plane leaves Manchester airport = 10 a.m.
The kinematic equation that can be used to find the time of flight is presented as follows;
\(Speed, \, v = \dfrac{Distance, \, D }{Time, \, t}\)
Therefore;
\(t = \dfrac{D}{v}\)
The time of flight is therefore;
\(t = \dfrac{200}{120} = \dfrac{5}{3} = 1\dfrac{2}{3}\)
The time of flight is \(1\frac{2}{3}\) hours or 5/3 hours which in minutes is therefore:
\(t = \dfrac{5}{3} \, hr \times 60 \, min/hr= 100 \, min\)
100 minutes = 1 hour 40 minutesThe time of arrival is 10 a.m. + 1 hour 40 minutes = 11:40 a.m.
Learn more about the kinematic equations of motion here:
https://brainly.com/question/29580588
#SPJ1
Inflation is at a rate of 7% per year. Today jennas favorite bread costs $3.79 what would it have cost 10 years ago?
Answers
DO u need the percetage or the price?Step-by-step explanation:
12. The distribution of the length of fish in a hatchery is approximately Normal with mean 14 inches and standard
deviation 2 inches.
(a) What is the probability that 16 randomly selected fish have a mean length more than 14.5 inches?
(b) What is the probability that 64 randomly selected fish have a mean length more than 14.5 inches?
(c) Explain why the probabilities in (a) and (b) are not equal.
Answers
a) P(mean length of 16 fish > 14.5 inches) ≈ 0.0668; b) P(mean length of 64 fish > 14.5 inches) ≈ 0.499; c) probabilities not equal due to Central Limit Theorem.
(a) The probability that the mean length of 16 randomly selected fish is more than 14.5 inches can be found using the standard normal distribution and the formula for a z-score. The z-score represents how many standard deviations a value is from the mean, and can be calculated as (14.5 - 14) / (2 / √16). Using a standard normal table or a calculator with a normal distribution function, the z-score can be used to find the probability of a value being above 14.5 inches.
(b) The probability that the mean length of 64 randomly selected fish is more than 14.5 inches can be found using the same method as in (a), with the only difference being the sample size in the calculation of the z-score. The z-score would be (14.5 - 14) / (2 / √64).
(c) The probabilities in (a) and (b) are not equal because the sample size is different. As the sample size increases, the standard deviation of the sample mean (known as the standard error) decreases, making it more likely that the sample mean will be close to the population mean. Therefore, as the sample size increases, the probability of the sample mean being above a certain value decreases.
Learn more about standard error here:
https://brainly.com/question/14524236
#SPJ4
Let LaTeX: A\:=\:\log_72A = log 7 2 and LaTeX: B\:=\:\log_73B = log 7 3 and LaTeX: C\:=\:\log_75C = log 7 5. Use the properties of logarithms to write each expression in terms of A and B and C (with possibly whole numbers as well).
Please type answers with no spaces. For example, an answer of A+B would be correct, whereas A + B would be incorrect. Use + - / for addition, subtraction, division. For multiplication, just leave the letters and/or coefficients next to each other.
LaTeX: \log_735
Answers
We are given that LaTeX: A\:=\:\log_72
A = log 7 2 and LaTeX:
B\:=\:\log_73
B = log 7 3 and LaTeX:
C\:=\:\log_75
C = log 7 5 and need to use the properties of logarithms to write LaTeX:
\log_735 in terms of A and B and C. Let us use the following properties of logarithms which are frequently used for simplification of logarithmic expressions: Product rule:
LaTeX: \log_{b}(mn)=\log_{b}m+\log_{b}n
Quotient rule: LaTeX: \log_{b}\left(\frac{m}{n}\right)=\log_{b}m-\log_{b}n.
Power rule: LaTeX: \log_{b}(m^n)=n\log_{b}(m) Applying these properties, we have,
LaTeX: \begin{aligned}
\log_7 35 &= \log_7 (5\cdot 7) \\ &
= \log_7 5 + \log_7 7 \\ &
= C + 1 \end{aligned}Therefore,
LaTeX: \log_735=C+1.
To know more about logarithms visit :
https://brainly.com/question/30226560
#SPJ11
The following equation can be used to determine c, the cost of a taxicab ride of m miles.
c = 3.5m + 3
What will happen to the cost of the taxicab ride if the distance increases by 2 miles?
A. The cost will go up by $2.00
B. The cost will go up by $3.50
C. The cost will go up by $6.50
D. The cost will go up by $7.00
Answers
The sum of two numbers is 23. Their difference is 1. whats the smaller number
Answers
On a scale drawing of a basketball court, 1 inch equals 8 feet. What is the actual area of the basketball court if the scale drawing is 11. 75 inches by 6. 25 inches? Enter your answer in the box.
Answers
On a scale drawing of a basketball court, 1 inch equals 8 feet the actual area of the basketball court if the scale drawing is 11. 75 inches by 6. 25 inches will be 4,700 square feet.
Given:
1 inch =8 feet 11.75 * 8 = 94 feet6.25 * 8 = 50 feetTo solve the given question we will assume the court is rectangular in shape so we will solve by using formula of Area of rectangle
\(\rm Area \;of\;rectangle\;= l\times b\)
Where, l=length
b=breadth
According to the given question,
\(\rm Area = 94\; feet \times 50\; feet\\\\ = 4,700 \;square\; feet.\\\)
Therefore, Area of the basketball court will be 4,700 square feet.
Learn more about Area here : https://brainly.com/question/931874
can someone pealse help me

Answers
Answer:
-1/2
Step-by-step explanation:
The side lengths of a triangle are 12,^149,^5. Is the triangle a right triangle?
PLEASE THE TEST IS DUE IN 10 MINE
Answers
Answer:
no
Step-by-step explanation:
12 squared plus 5 squared=169