Drag each set of coordinates to the correct location on the table.
Calculate the distance between the pairs of coordinates, and classify them according to the distance between them.
(3 4) and (2,
(3, 7) and (5, 2)
(5, -2) and (3,
3) and (1, 2)
-2) and (-3,
(4, -1) and (-1, '
10 units
29 units
Answers
The first pair of coordinates is located at (2, -4).
The second pair of coordinates is classified as a long distance of 29 units.
The third pair of coordinates is located at (3, 5).
The fourth pair of coordinates is classified as a short distance of 2.24 units.
The fifth pair of coordinates is located at (-3, -1).
The sixth pair of coordinates is classified as a long distance of 6.4 units.
To solve this problem, we need to use the distance formula, which is:
distance = sqrt((x2 - x1)^2 + (y2 - y1)^2)
For the first pair of coordinates, we are given one coordinate and the distance between the two points, so we need to use the distance formula to solve for the missing coordinate. Plugging in the values we know, we get:
10 = sqrt((x2 - 3)^2 + (y2 - 4)^2)
Squaring both sides, we get:
100 = (x2 - 3)^2 + (y2 - 4)^2
Since we know that the missing coordinate has an x-value of 2, we can plug that in and solve for the y-value:
100 = (-1)^2 + (y2 - 4)^2
96 = (y2 - 4)^2
y2 = 8 or -0.8
Since the coordinates must be integers, we choose the y-value of 8, and the coordinates of the first pair are (2, 8).
For the second pair of coordinates, we simply plug in the values and solve:
distance = sqrt((5 - 3)^2 + (2 - 7)^2)
distance = sqrt(2^2 + (-5)^2)
distance = sqrt(29)
The distance between the second pair of coordinates is 29 units, which is classified as a long distance.
For the third pair of coordinates, we are given both coordinates, so there is nothing to solve. The third pair of coordinates is (3, 5).
For the fourth pair of coordinates, we simply plug in the values and solve:
distance = sqrt((1 - 3)^2 + (2 - (-4))^2)
distance = sqrt((-2)^2 + 6^2)
distance = sqrt(40)
distance = 2.24 (rounded to two decimal places)
The distance between the fourth pair of coordinates is 2.24 units, which is classified as a short distance.
For the fifth pair of coordinates, we are given one coordinate and the distance between the two points, so we need to use the distance formula to solve for the missing coordinate. Plugging in the values we know, we get:
6.4 = sqrt((x2 - (-3))^2 + (y2 - (-1))^2)
Squaring both sides, we get:
40.96 = (x2 + 3)^2 + (y2 + 1)^2
Since we know that the missing coordinate has an x-value of -1, we can plug that in and solve for the y-value:
40.96 = (2)^2 + (y2 + 1)^2
37.96 = (y2 + 1)^2
y2 = 5 or -7
Since the coordinates must be integers, we choose the y-value of -7, and the coordinates of the fifth pair are (-1, -7).
Overall, we were given six pairs of coordinates and classified each of them according to their distance. We used the distance formula to find the missing coordinates for the first and fifth pairs and found the distances.
Learn more about integers here: - brainly.com/question/1768255
#SPJ11
Related Questions
The midpoint AB is (1,2). The coordinates of A are (-3,6) Find the coordinates of B
Can someone help me with this exercise and show me the procedure of how to do it??
Answers
========================================================
Explanation:
We have these points
A = (-3,6)M = midpoint = (1,2)B = (x,y)Focus on the x coordinates for now.
The x coordinates of A and B are -3 and x in that order. Add them up and divide in half to get the x coordinate of the midpoint M
(-3+x)/2 = 1
-3+x = 2*1
-3+x = 2
x = 2+3
x = 5
Repeat for the y coordinates
(6+y)/2 = 2
6+y = 2*2
6+y = 4
y = 4-6
y = -2
Therefore, the location of point B is (5, -2)
You can use a tool like GeoGebra to check your work.

Solve the equation: (12)/(x^(2))+5=9
Answers
Answer:
1.7321
Step-by-step explanation:
I got this answer when I went through the steps.
Ann, Deandre, and Bob have a total of $ 94 in their wallets. Bob has 2 times what Ann has. Ann has $10 less than Deandre. How much do they have in their wallets
Answers
Ann has 21, Deandre has 31, and Bob has 42 in their wallets.
Let's start by using variables to represent the amount of money each person has:
Let A be the amount of money Ann has.
Let B be the amount of money Bob has.
Let D be the amount of money Deandre has.
We can then translate the problem into a system of equations:
A + B + D = 94 (the total amount of money they have is 94)
B = 2A (Bob has twice what Ann has)
A = D - 10 (Ann has 10 less than Deandre)
We can use the third equation to substitute A in terms of D in the first two equations:
A = D - 10
B = 2A = 2(D - 10) = 2D - 20
A + B + D = 94 => (D - 10) + (2D - 20) + D = 94 => 4D - 30 = 94 => 4D = 124 => D = 31
So Deandre has 31. We can use the third equation again to find that Ann has 21, and then we can use the second equation to find that Bob has 42.
for such more question on variables
https://brainly.com/question/27761372
#SPJ11
The perimeter of a rectangular field is 240 m. If its length is 90 m, find (i) breadth (ii) area of the rectangle
Answers
Answer :
Breadth = 30 m
area of the rectangle = 2700 m²
Explanation :
⋆Given :
↦Perimeter = 240 m
↦Length = 90 m
i) Breadth
Solution :-
↬ Perimeter of a rectangle = 2 ( l + b )
↬ 240 = 2(90 + b)
↬ 240 = 180 + 2b
↬ 240 - 180 = 2b
↬ \( \frac{60}{2} \) = b
↬ 30m = b
(ii) Area of a rectangle
Solution :-
↬ Area of a rectangle = l × b
↬ Area of a rectangle = 90 m × 30 m
↬ Area of a rectangle = 2700 m²
Help me with this question

Answers
Answer:
The correct option is: x > z
Hence, option D is correct.
Step-by-step explanation:
Given
\(\frac{3y+1}{2}=5\)\(\:\frac{4x}{3}=8\)\(\frac{z}{3}+\frac{z}{4}=2\)solving
\(\frac{3y+1}{2}=5\)
Multiply both sides by 2
\(\frac{2\left(3y+1\right)}{2}=5\cdot \:2\)
Simplify
\(3y+1=10\)
Subtract 1 from both sides
\(3y+1-1=10-1\)
Simplify
\(3y=9\)
Divide both sides by 3
\(\frac{3y}{3}=\frac{9}{3}\)
Simplify
\(y=3\)
also solving
\(\:\frac{4x}{3}=8\)
Multiply both sides by 3
\(\frac{3\cdot \:4x}{3}=8\cdot \:3\)
\(4x=24\)
Divide both sides by 4
\(\frac{4x}{4}=\frac{24}{4}\)
Simplify
\(x=6\)
also solving
\(\frac{z}{3}+\frac{z}{4}=2\)
Multiply by L.C.M
\(\frac{z}{3}\cdot \:12+\frac{z}{4}\cdot \:12=2\cdot \:12\)
Simplify
\(4z+3z=24\)
\(7z=24\)
Divide both sides by 7
\(\frac{7z}{7}=\frac{24}{7}\)
Simplify
\(z=\frac{24}{7}\)
\(z=3.4\)
Thus,
\(y=3\)
\(x=6\)
\(z=3.4\)
so
x > z
Therefore, the correct option is: x > z
Hence, option D is correct.
PLEASE GIVE ME RIGHT ANSWERS HELP ME FAST I BEG YOU




Answers
Reason 1: Given
Reason 4: Reflexive Property
Reason 5: ASA
Proof 2:
Reason 2: Alternate Interior Angles Theorem
Reason 3: Given
Reason 4: Reflexive Property
Reason 5: AAS
Proof 3:
Reason 2: Given
Reason 3: Symmetric Property
Reason 4: Hypotenuse-Leg Theorem
Reason 5: CPCTC
Proof 4:
Reason 2: Definition of bisector
Reason 3: Given
Reason 4: Reflexive Property
Reason 5: SAS
Conversion and Perimeter assignment
Can anyone help me please and thank you please use a step by step
explaination ty

Answers
As a result of answering the given question, we may state that Therefore, the perimeter of the pentagon is \(5\frac{5}{16}\) in.
what is a pentagon?A pentagon is a five-sided polygon that is used in geometry. The inner angles of a simple pentagon sum up to 540°. Pentagons can be simple or self-intersecting. A pentagram is a regular pentagon that intersects itself. A regular pentagon has five equal angles and all sides are equal. If the side lengths and angle measurements do not match, it is called an irregular pentagon. As far as we know, all outside angles balance out inside angles. The exterior angle total of the polygon is thus n(360°/n). Because a pentagon has five sides, n must be equal to five. As a result, the total of the pentagon's outer angles is 5(360°/5) = 360°.
to calculate the perimeter on pentagon,
perimeter = \(1\frac{1}{8} + 1\frac{1}{8} + 1\frac{1}{16} + 1\frac{1}{16} + 1\frac{1}{8} + 1\frac{1}{16}\)
Perimeter = \(5\frac{5}{16}\)
Therefore, the perimeter of the pentagon is \(5\frac{5}{16}\) in.
To know more about pentagon visit:
https://brainly.com/question/17054992
#SPJ1
The perimeter of the pentagon is 5 and 9/16 inches or 89/16 in.
Describe Pentagon?A Pentagon is a geometric shape that consists of five sides and five angles. It is a regular polygon, which means that all five of its sides are of equal length and all five of its angles are of equal measure. The interior angles of a regular pentagon measure 108 degrees each, and the sum of all its interior angles is 540 degrees.The pentagon shape can also be found in nature, such as in the shape of some types of starfish, sea urchins, and other marine creatures. It is also used in art and design, such as in the design of jewelry, architecture, and other decorative objects.
To find the perimeter of the pentagon, we need to add up the length of all five sides.
The sides are given in mixed fractions, so we first need to convert them to improper fractions. We can do this by multiplying the whole number by the denominator and adding the numerator, then putting the result over the denominator. For example:
1 and 1/8 in = (1 x 8 + 1) / 8 = 9/8 in
Using this method for all five sides, we get:
9/8 in, 9/8 in, 17/16 in, 17/16 in, 17/16 in
Now we can add them up to get the perimeter:
9/8 in + 9/8 in + 17/16 in + 17/16 in + 17/16 in
To add fractions with unlike denominators, we need to find a common denominator. In this case, the lowest common multiple of 8 and 16 is 16, so we can convert all the fractions to have a denominator of 16:
18/16 in + 18/16 in + 17/16 in + 17/16 in + 17/16 in
Now we can add them up:
89/16 in
This is an improper fraction, so we can simplify it by dividing the numerator by the denominator:
5 9/16 in
Therefore, the perimeter of the pentagon is 5 9/16 inches.
To know more about angles visit:
https://brainly.com/question/2176794
#SPJ1
When you listen to the sound of a bouncing ping-pong ball that has been dropped onto a cement floor, what mathematical pattern do you hear? Explain,
Answers
A drop of water is denser than a ping-pong ball.
Usually, water is made of particles that are firmly pressed together. In differentiation, plastic (the material ping pong balls are made of) may be a lightweight fabric and the particles are not as firmly stuffed together.
The thickness of a ping pong ball is 0.0840 g/cm³, though water’s thickness is 997 kg/m³. Subsequently, ping pong balls aren’t about as thick as water and will continuously coast and surface greatly quickly.
The ping pong ball appears to oppose gravity and coast within the air.
Ping-pong balls drift within the water since they are amazingly lightweight, empty, and filled with air. Too, the water’s surface pressure makes it simple for the ping pong ball to drift.
In expansion, water is denser than ping pong balls, making them look for the most noteworthy point of water.
Learn more about density at
brainly.com/question/15164682
#SPJ4
The sound which we hear when the pig pong ball is bounced on the floor
is 19.48 DB
The repeating of sounds, especially in rhyme, is the form of repetition that most people connect with poetry. Alliteration, assonance, and onomatopoeia are other sound patterns in poetry that give additional meaning in addition to rhyme. Every one of these audio elements has a certain purpose in a poem.
a) \(\sum \ log(n)\)
by expanding the series for each value of n is
log (1) + log (2) + log(3) + log (4) + ......... + log ( 96)
simplify the expanded form we get
0 + 0.3010 + 0.4771+0.6020 ......................... + 1.982
=> 149.9963
b) \(\sum_{n=0}\) to infinity \(\sqrt{0.9^n}\)
formula for the sum of number in geometric progression
is a/1-r
to find the ratio of the successive terms
plugging into the formula
r = \(\frac{a_{n+1}}{a_n}\)
r = \(\frac{\sqrt{0.9^{n+1}} }{\sqrt{0.9^n} }\)
=> r = \(\frac{\sqrt{0.9^n \times 0.9} }{\sqrt{0.9^n} .1}\)
=> r = \(\frac{\sqrt{0.9} }{1}\)
=> r = \(\sqrt{0.9}\)
=> a = \(\sqrt{0.9^0}\)
=> a = \(\sqrt{1}\)
=> a= 1
by applying the formula having the value a =1 is
\(\frac{1}{1-\sqrt{0.9} }\)
rationalize the denominator by multiplying with \(1+\sqrt{0.9}\)
=> \(\frac{1+\sqrt{0.9} }{(1-\sqrt{0.9} ) (1+\sqrt{0.9}) }\)
=> 19.4868
To learn more about Sound :
https://brainly.com/question/30168219
#SPJ4
what is the equation?

Answers
Hope this helps
Prove that if A is invertible and AB = 0, then B = 0
Answers
we can conclude that if A is invertible and AB = 0, then B must be 0.
To prove that if A is invertible and AB = 0, then B = 0, we can use a proof by contradiction.
Assume that A is an invertible matrix and AB = 0, but B ≠ 0.
Since A is invertible, we can multiply both sides of AB = 0 by A⁻¹ (the inverse of A) to obtain:
A⁻¹ * (AB) = A⁻¹ * 0
By the associative property of matrix multiplication, we can rearrange the expression as:
(A⁻¹ * A) * B = 0
Since A⁻¹ * A is the identity matrix (I), the expression simplifies to:
I * B = 0
Multiplying any matrix by the identity matrix yields the same matrix. Therefore, we have:
B = 0
This contradicts our initial assumption that B ≠ 0.
Since our assumption led to a contradiction, we can conclude that if A is invertible and AB = 0, then B must be 0.
Learn more about Matrix here
https://brainly.com/question/30828476
#SPJ4
The length of a rectangle is three times it’s width if the perimeter of the rectangle is 80m find it’s area
Answers
Answer:
=3y^2
Step-by-step explanation:
let,
length: x
width: y
According to condition,
x = 3y -----------(1)
Second condition
Perimeter = 2 (l + b)
80 = 2 (x + y)
x + y = 40 -------------(2)
To find area,
Area = L x B
=3y x y
= 3y^2
How much work is done when a hoist lifts a 290-kg rock to a height of 6 m? (Use 9.8 m/s² for the acceleration due to gravity.)'
Answers
The work done when lifting the 290-kg rock to a height of 6 m is 17,052 Joules.
To calculate the work done when lifting the rock, we need to use the formula:
Work = Force × Distance × cos(theta)
In this case, the force required to lift the rock is equal to its weight, which can be calculated using the formula:
Force = mass × acceleration due to gravity
Given:
mass of the rock (m) = 290 kg
acceleration due to gravity (g) = 9.8 m/s²
height (distance) lifted (h) = 6 m
theta (angle between the direction of force and the direction of motion) = 0° (since the force and displacement are in the same direction)
First, let's calculate the force:
Force = mass × acceleration due to gravity
Force = 290 kg × 9.8 m/s²
Force = 2842 N
Now, we can calculate the work done:
Work = Force × Distance × cos(theta)
Work = 2842 N × 6 m × cos(0°)
Work = 2842 N × 6 m × 1
Work = 17,052 Joules
Therefore, the work done when lifting the 290-kg rock to a height of 6 m is 17,052 Joules.
Learn more about height here:
https://brainly.com/question/29131380
#SPJ11
The measures of the two angles in a triangle are 82 degrees and 55 degrees. Find
the missing angle measure. Please show your math work step by step and don’t
forget the unit.
Answers
Answer: 43°
Step-by-step explanation:
First, find the sum of the 2 angles: 82+55=137
Then, subtract 137 from 180 because the sum of all the angles in the triangle is 180°: 180-137=43
Therefore, the missing angle is 43°
What does the point (4, 122) represent?
Answers
The x-coordinate is 4: This tells us that the point lies on the vertical line that passes through x = 4 on the coordinate plane.
The y-coordinate is 122: This tells us that the point also lies on the horizontal line that passes through y = 122 on the coordinate plane.
Combining these two pieces of information, we can conclude that the point (4, 122) represents the intersection of the vertical line x = 4 and the horizontal line y = 122 on the coordinate plane.
In other words, if we were to plot this point on the coordinate plane, it would be 4 units to the right of the origin and 122 units above the origin.
Which of these triangles appears not to be congruent to any others show here? Check all that apply.
A. Triangle A
B. Triangle E
C. Triangle C
D. Triangle F
E. Triangle B
F. Triangle D

Answers
Triangles E and F are not to be congruent to any other triangles.
What is Triangle?A triangle is a three-sided polygon that consists of three edges and three vertices.
We have to check triangles which appears not to be congruent to any others.
Two triangles are said to be similar if their corresponding angles are congruent and the corresponding sides are in proportion .
The triangles A and B are congruent as they have same shape and same angles.
The triangles C and D have same shape and same angles so they are congruent.
The triangles E and F are not congruent to any of the other triangles because they have unique shape and angles.
Hence, Triangles E and F are not to be congruent to any other triangles.
To learn more on Triangles click:
https://brainly.com/question/2773823
#SPJ1
Find the volume, the total surface area and the lateral surface area of the cuboid having: 1) Length = 10 m, breadth = 35cm and height = 1.2m
Answers
Answer:
Volume = 4.2m³
Total surface area = 31.84m²
Lateral surface area = 24.84m²
Step-by-step explanation:
1m = 100cm
Cuboid's;
Length (l) = 10m
Width (w) = 35cm = 0.35m
height(h) = 1.2m
Volume:
Volume (v) of a cuboid is given by: Length × Width × Height = l × w × h
v = 10m × 0.35m × 1.2m = 4.2m³
Total Surface Area:
Total surface area (\(A_{T}\)) is given by adding the areas of all rectangles that make the cuboid.
\(A_{T}\) = 2(10m × 1.2m) + 2(0.35m × 1.2m) + 2(10m × 0.35m) = 24m² + 0.84m² + 7m² = 31.84m²
\(A_{T}\) = 31.84m²
Lateral Surface Area:
Lateral surface area (\(A_{L}\)) is found by subtracting base and top area from the total surface area.
Base and top area = 2(10m × 0.35m) = 7m²
∴ \(A_{L}\) = 31.84m² = 7m² = 24.84m²
Simplify 2 + 17x - 5x + 9

Answers
Answer:
12x + 11
Step-by-step explanation:
Combine like terms
Answer:
B. 12x + 11 :)
Step-by-step explanation:
Does -3(x-4)= -3x=12 have one solution, many solutions, or no solutions at all?
Answers
Answer:
If the expression is:
-3x + 12 = -3x + 12, infinite solutions
If the expression is:
-3x + 12 = -3x - 12, no solutions
Step-by-step explanation:
Step 1: Distribute
-3x + 12 = 3x = 12
If both lines have the same slope and same y-int, they are the same line and therefore have infinite solutions.
If both lines have the same slope but different y-int, then they are parallel and therefore have no solutions.
Evaluate: 3(2x + z) when x = 8 and z = 2
Answers
\(\huge\boxed{\mathcal{HELLO!:)}}\)
First of all, let's use the Distributive Property and distribute 3:
\(\rm{3(2x+z)\)
\(\rm{6x+3z\)
Now, plug in the values of x and z:
\(\rm{6(8)+3(2)\)
Multiply:
48+6
Add:
\(\huge\boxed{\boxed{\maltese\pmb{Answer:54}}}\)
Hope it helps! Enjoy your day!
~Just a determined gal
\(\bf{erutaNtneliS\)
Graph the line with the points (2,3) with the slope =1/2
Answers
To solve this problem, first, we compute the equation of the line that passes through the point (2,3) and has slope 1/2 using the slope-point formula for the equation of a line:
\(y-3=\frac{1}{2}(x-2)\text{.}\)Answer:

What is the answer ?????

Answers
Step-by-step explanation:
very difficult............
What value must b have in the function f(x)=x^4+bx^2+1 so that the function has at least one inflection point over the interval (1,2)?
Answers
The function f(x) = x^4 + bx^2 + 1 has an inflection point at x = 0,
What is the inflection point?
An inflection point of a function is a point on the graph of the function where the concavity (curvature) of the graph changes. In other words, an inflection point is a point where the curve switches from being concave up to concave down, or vice versa.
The function f(x) = x^4 + bx^2 + 1 has an inflection point at x = 0, because the concavity of the graph changes at that point. To find the value of b such that the function has at least one inflection point over the interval (1,2), we can set x = 1 and x = 2 in the function and solve for b.
If we set x = 1, we get the equation f(1) = 1 + b + 1 = 0. Solving for b, we find that b = -2.
If we set x = 2, we get the equation f(2) = 16 + 2b + 1 = 0. Solving for b, we find that b = -9.
Since the function has an inflection point at x = 0 for any value of b, it will have at least one inflection point over the interval (1,2) for any value of b.
Therefore, the value of b can be any real number.
To learn more about Inflection point, Visit
brainly.com/question/10352137
#SPJ4
1. Time-series analysis
a. White noise definition
b. How can you tell if the specified model describes a stationary or non-stationary process? We discussed this in the contest of MA and AR models
c. What is the purpose of Box Pierce, Dickey-Fuller, Ljung-Box, Durbin-Watson tests.
Answers
Time-series analysis is a statistical method that's used to analyze time series data or data that's correlated through time. In this method, the data is studied to identify patterns in the data over time. The data is used to make forecasts and predictions. In this method, there are different models that are used to analyze data, such as the AR model, MA model, and ARMA model.
a. White noise definition In time series analysis, white noise refers to a random sequence of observations with a constant mean and variance. The term white noise is used to describe a series of random numbers that are uncorrelated and have equal variance. The autocorrelation function of white noise is 0 at all lags. White noise is an important concept in time series analysis since it is often used as a reference against which the performance of other models can be compared .b. How can you tell if the specified model describes a stationary or non-stationary process? We discussed this in the contest of MA and AR models To determine if a specified model describes a stationary or non-stationary process, we look at the values of the coefficients of the model.
For an AR model, if the roots of the characteristic equation are outside the unit circle, then the model is non-stationary. On the other hand, if the roots of the characteristic equation are inside the unit circle, then the model is stationary.For an MA model, if the series is non-stationary, then the model is non-stationary. If the series is stationary, then the model is stationary.c. What is the purpose of Box Pierce, Dickey-Fuller, Ljung-Box, Durbin-Watson testsThe Box-Pierce test is used to test whether the residuals of a model are uncorrelated. The Dickey-Fuller test is used to test for the presence of a unit root in a time series. The Ljung-Box test is used to test whether the residuals of a model are white noise. Finally, the Durbin-Watson test is used to test for the presence of autocorrelation in the residuals of a model. These tests are all used to assess the adequacy of a fitted model.
To know more about Time-series analysis visit:-
https://brainly.com/question/33083862
#SPJ11
Use the given function value and the trigonometric Identities to find the exact value of each indicated trigonometric function
0˚ ≤ ᵝ ≤ 90˚ , 0≤ ᵝ≤π/2 cost(ᵝ)=√11/6
a. sec(ᵝ) 6/11
b. sin (ᵝ) 25/36
c. Cot(ᵝ) 11/5
d. Sih(ᵝ- 90˚) 11/6
Answers
:a. sec(β) = `6/11`b. sin (β) = `5/6`c. Cot(β) = `2.01` (approximately)d. Sin(β - 90˚) = `√11/6` = `0.95` (approximately) are the exact values of each indicated trigonometric function.
Given function value is `cos(β) = √11/6` where `0˚ ≤ β ≤ 90˚` and `0 ≤ β ≤ π/2`.
To find the exact value of each indicated trigonometric function, we need to first find sin(β), tan(β), cos(β), csc(β), sec(β), and cot(β) where `0˚ ≤ β ≤ 90˚` and `0 ≤ β ≤ π/2`.
We know that `sin²(β) + cos²(β) = 1`.So, `sin(β) = ± √(1 - cos²(β))`Since `0˚ ≤ β ≤ 90˚`, `sin(β) = √(1 - cos²(β))`Now, `sin(β) = √(1 - (√11/6)²)` = √(1 - 11/6) = √(6/6 - 11/6) = √(-5/6)
Since the value of β lies in the first quadrant, sin(β) is positive. Therefore, `sin(β) = √(5/6)`We also know that `tan(β) = sin(β)/cos(β)`.So, `tan(β) = (√(5/6))/((√11)/6)`
Now, `tan(β) = (6√5)/11`Similarly, we can find the values of all other trigonometric functions.a. sec(β) = `1/cos(β)` = `1/(√11/6)` = `6/√11` = `6/11`b. sin (β) = `√(5/6)` = `5/6`c. Cot(β) = `1/tan(β)` = `1/((6√5)/11)` = `11/(6√5)` = `(11/6) * (1/√5)` = `11/(6√5)` * `(√5/√5)` = `(11√5)/30` = `(11/5.48)` = `2.01` (approximately)d. Sin(β - 90˚) = `cos(β)` = `√11/6`
Therefore, the exact value of each indicated trigonometric function is:a. sec(β) = `6/11`b. sin (β) = `5/6`c. Cot(β) = `2.01` (approximately)d. Sin(β - 90˚) = `√11/6` = `0.95` (approximately).
Therefore, the options (a), (b), (c), and (d) are (6/11), (25/36), (11/5), and (11/6) respectively.
To learn more about function, refer below:
https://brainly.com/question/30721594
#SPJ11
4
Find the area of the shape.
Either enter an exact answer in terms of π or use 3.14 for and enter your answer as a decimal.
units2
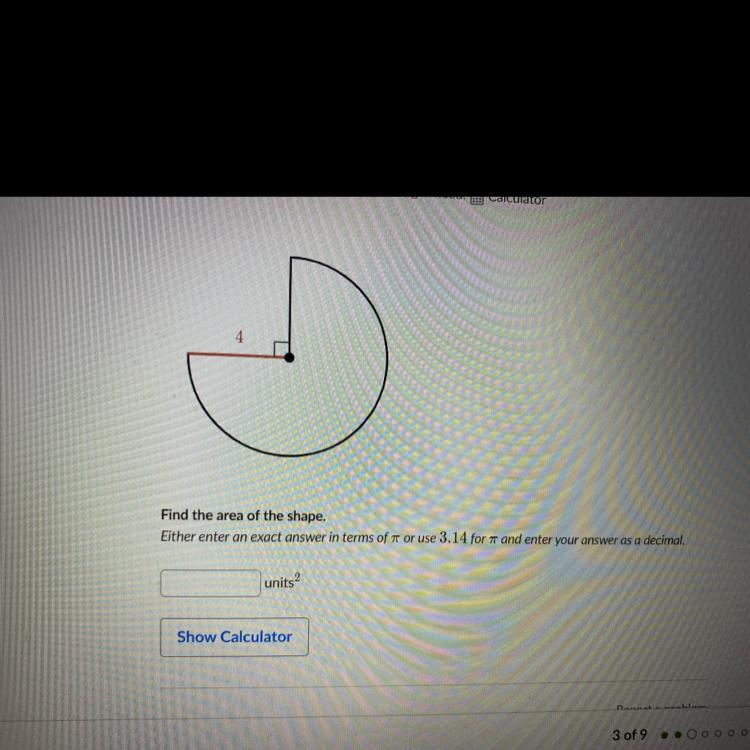
Answers
Answer:
12π un²
Step-by-step explanation:
Hello!
Area of a Circle: \(A = \pi r^2\)
You are technically being asked for 3/4 of the area of a circle. The radius is 4.
Find the Area\(A = \frac34 \pi r^2\)\(A = \frac34 \pi (4)^2\)\(A = \frac34 (16)\pi\)\(A = 12\pi\)The answer is 12π un².
You invest $20,000 in the stock market. The stock market then plummets
over the next few weeks. Each day, your investment loses half of its value. How
much will you have invested after 14 days? Write the geometric sequence
formula and show all of your work.
Answers
After 14 days, you will have approximately $2.4414 invested in the stock market.
The amount you will have invested after 14 days can be calculated using the geometric sequence formula. The formula for the nth term of a geometric sequence is given by:
an = a1 x \(r^{(n-1)\)
Where:
an is the nth term,
a1 is the first term,
r is the common ratio, and
n is the number of terms.
In this case, the initial investment is $20,000, and each day the investment loses half of its value, which means the common ratio (r) is 1/2. We want to find the value after 14 days, so n = 14.
Substituting the given values into the formula, we have:
a14 = 20000 x\((1/2)^{(14-1)\)
a14 = 20000 x \((1/2)^{13\)
a14 = 20000 x (1/8192)
a14 ≈ 2.4414
Therefore, after 14 days, you will have approximately $2.4414 invested in the stock market.
For more such answers on ratio
https://brainly.com/question/12024093
#SPJ8
The amount you will have invested after 14 days is given as follows:
$2.44.
What is a geometric sequence?A geometric sequence is a sequence of numbers where each term is obtained by multiplying the previous term by a fixed number called the common ratio q.
The explicit formula of the sequence is given as follows:
\(a_n = a_1q^{n-1}\)
In which \(a_1\) is the first term of the sequence.
The parameters for this problem are given as follows:
\(a_1 = 20000, q = 0.5\)
Hence the amount after 14 days is given as follows:
\(a_{14} = 20000(0.5)^{13}\)
\(a_{14} = 2.44\)
More can be learned about geometric sequences at https://brainly.com/question/24643676
#SPJ1
Question below, please help me

Answers
The school puts up fencing around an area in the shape of a hexagon for an outdoor concert. how much fencing does the school use? round your answer to the nearest whole yard.
Answers
The total length of school installs fencing around a hexagon-shaped area for an outside concert, is 36 yards.
What is the distance formula?Distance is a numerical measurement of the distance between two objects or places. Distance can refer to a physical length or an estimate based on other criteria in physics or common usage. The distance between two points A and B is commonly expressed as |AB|.To find the fencing used by the school:
The distance formula can be given as: \(d=\sqrt{(x_{2}-x_{1} )^{2} +(y_{2} -y_{1})^{2} }\)
First, we must calculate the length of the hexagon using the distance formula.
The hexagon's coordinates, according to the graph, are:
(-5, 4), (0, 6), (5, 4), (6, -2), (0, -4), and (-6, -2)
The distance between (-5, 4), (0, 6): \(d=\sqrt{(5)^{2} +(6-4)^{2} }\)
d = √29 yardsSimilarly, the distance between (-5, 4) and (6, -2):
= √37 yardsAnd the distance between (6, -2) and (0, -4):
= √40 yardsAmount of fencing required = 2(√29+√37+√40):
= 35.58 units ≈ 36 yardsTherefore, the total length of school installs fencing around a hexagon-shaped area for an outside concert, is 36 yards.
Know more about the distance formula here:
https://brainly.com/question/20761638
#SPJ4
The complete question is given below:
Type the correct answer in the box. Use numerals instead of words. If necessary, use / for the fraction bar.
The school puts up fencing around an area in the shape of a hexagon for an outdoor concert. How much fencing does the school use? Round your answer to the nearest whole yard.

Answer:
36 yards of fencing
Step-by-step explanation:
the reason I know this is because I got it right.
How do you measure the size of an angle using a protractor or a compass? Explain the steps and show your work.
Answers
The size of the angles is measured in the form of degrees by using a protractor and compass.
The symbol for degrees is a little circle °.
The FULL CIRCLE is 360° (360 degrees). A half circle or a straight angle is 180°.A quarter circle or a right angle is 90°A protractor is a tool that measures the number of degrees in an angle.
To measure an angle with a protractor:
Place the midpoint of the protractor on the VERTEX of the angle.Line up one side of the angle with the zero line of the protractor.Read the protractor to see where the other side of the angle crosses the number scale.Most protractors have two number scales. It's important to use the same number scale for both sides of the angle.
To know more about the measure of angle:
https://brainly.com/question/15842847
#SPJ4





In a recent election, 63% of all registered voters participated in voting. In a survey of 275 retired voters, 162 participated in voting. Which is higher, the population proportion who participated or the sample proportion from this survey?
Answers
The population proportion who participated in voting (63%) is higher than the sample proportion from this survey (58.91%).
To determine whether the population proportion who participated in voting or the sample proportion from the survey is higher, we need to compare the percentages.
The population proportion who participated in voting is given as 63% of all registered voters.
This means that out of every 100 registered voters, 63 participated in voting.
In the survey of retired voters, 162 out of 275 participants voted. To calculate the sample proportion, we divide the number of retired voters who participated (162) by the total number of retired voters in the sample (275) and multiply by 100 to get a percentage.
Sample proportion = (162 / 275) \(\times\) 100 ≈ 58.91%, .
Comparing the population proportion (63%) with the sample proportion (58.91%), we can see that the population proportion who participated in voting (63%) is higher than the sample proportion from this survey (58.91%).
Therefore, based on the given data, the population proportion who participated in voting is higher than the sample proportion from this survey.
It's important to note that the sample proportion is an estimate based on the surveyed retired voters and may not perfectly represent the entire population of registered voters.
For similar question on population proportion.
https://brainly.com/question/29516589
#SPJ8