Answers
Answer:
65
Step-by-step explanation:
triangle on right:
180-62-50= 68
left triangle:
180-80-53=47
middle triangle: using both answers above
180-68-47= 65
since X is the vertical angle of 65, X would also equal 65 degrees
Related Questions
For their twentieth wedding anniversary, Richard and Sylvia had a party for several of their best friends. Things were going along just fine until one of their friends asked the happy couple who was older! Here's what they had to say. Richard: "I am older than my wife." Sylvia: "I am younger than my husband." That might have been the end of it, but one of the guests knew that at least one member of the couple was lying. Well, who is older, Richard or Sylvia?
Answers
Answer:
Richard is older
Step-by-step explanation:
We can set up an inequality for both statements.
Let r equal Richard's age and s equal Sylvia's age.
"I am older than my wife."
Since Richard is speaking, the inequality would look like this:
r > s
This means Richard is older than Sylvia.
"I am younger than my husband."
Since Sylvia is speaking, the inequality would look like this:
s < r
This means that Sylvia is younger than Richard.
We can flip one inequality to "see" them from the same perspective.
Let's use s < r
To make it so that we can see the relationship from Richard's perspective, flip the entire inequality.
s < r
to
r > s
The inequality from the first quote is identical to this one!
Therefore, Richard is older than Sylvia.
Solve the following variation problem.
The interest on an investment varies directly as the rate of interest. If the interest is $45 when the interest rate is 4%, find the interest when the rate is 9%.
The interest is $____. (Round to the nearest cent.)
Please help asap! Tysm!
Answers
The interest on the investment when the interest rate is 9% is; $101.25.
What is the interest when the interest rate is 9%?It follows from the task content that the interest on an investment varies directly as the rate of interest.
Hence, it follows from proportion that the interest when the interest rate is; 9% can be calculated as follows;
= 9 ×45/4
= $101.25
Ultimately, the interest on the investment evaluated by means of proportional reasoning is therefore; $101.25.
Read more on proportional relationship;
https://brainly.com/question/21302696
#SPJ1
Chris is buying supplies for a school fundraiser and has $56 to spend. He buys popcorn for $3 per bag and cotton candy for
$7 per bag. He needs at least 7 bags of popcorn.
Graph the boundary lines of the linear inequalities on the graph below.
Also, plot the points that are part of the solution set from the list below.
(3, 7), (2, 2), (8, 1), (10, 2), (5, 5)
Answers
The points that are part of the solution set are (3, 7), (8, 1), and (10, 2).
What do you mean by Coordinate plane ?The coordinate plane is a two-dimensional space formed by two perpendicular lines called the x-axis and y-axis. The point where the two axes intersect is called the origin. Each point in the plane is identified by a pair of numbers called its coordinates, where the first number represents its position along the x-axis and the second number represents its position along the y-axis. Coordinates are typically written as (x, y), with the x-coordinate listed first and the y-coordinate listed second. The coordinate plane is commonly used in mathematics to graph and analyze equations, functions, and geometric shapes.
Let x be the number of bags of popcorn and y be the number of bags of cotton candy. Then the cost constraint is:
3x + 7y ≤ 56
We also have the constraint that Chris needs at least 7 bags of popcorn:
x ≥ 7
To graph these constraints, we can rewrite them in slope-intercept form:
y ≤ (-3/7)x + 8
x ≥ 7
The first inequality has a slope of -3/7 and a y-intercept of 8, and the second inequality is a vertical line at x = 7.
To plot the points that are part of the solution set, we can simply plug them into the two inequalities and see if they are true. The points that satisfy both inequalities are part of the solution set.
(3, 7) satisfies both inequalities:
7 ≤ (-3/7)(3) + 8
3 ≥ 7
(2, 2) does not satisfy the first inequality:
2(7) > 3(2) + 7
(8, 1) satisfies both inequalities:
1 ≤ (-3/7)(8) + 8
8 ≥ 7
(10, 2) satisfies both inequalities:
2 ≤ (-3/7)(10) + 8
10 ≥ 7
(5, 5) does not satisfy the first inequality:
5(7) > 3(5) + 7
Therefore, the points that are part of the solution set are (3, 7), (8, 1), and (10, 2).
To graph these on the coordinate plane, we can plot the vertical line x=7 and the line y= (-3/7)x + 8, and then shade in the feasible region below the line and to the right of the vertical line.
Learn more about Coordinate plane here
https://brainly.com/question/24134413
#SPJ1
arrange the given steps in the correct order to prove that a⊆c if a⊆b and b⊆c.
Answers
To prove that a⊆c if a⊆b and b⊆c, we need to arrange the steps in the correct order to construct a logical proof.
The correct order is as follows:
Step 1: Start by assuming that a⊆b and b⊆c are true.
Step 2: Take an arbitrary element x and assume that x∈a.
Step 3: Using the assumption a⊆b, conclude that x∈b.
Step 4: Using the assumption b⊆c, conclude that x∈c.
Step 5: Since x was an arbitrary element, we have shown that for any element x, if x∈a, then x∈c.
Step 6: Therefore, by definition, a⊆c.
Let's briefly explain the reasoning behind each step:
Step 1: This is the initial assumption given in the problem statement. We assume that a⊆b and b⊆c are true.
Step 2: To prove that a⊆c, we need to show that every element of a is also an element of c. We start by taking an arbitrary element x and assuming that it belongs to a (x∈a).
Step 3: Using the assumption a⊆b, we know that if x∈a, then x∈b. This follows directly from the definition of subset: every element of a is also an element of b.
Step 4: Similarly, using the assumption b⊆c, we know that if x∈b, then x∈c. Again, this follows directly from the definition of subset: every element of b is also an element of c.
Step 5: Combining the conclusions from Steps 3 and 4, we can infer that if x∈a, then x∈c. Since x was an arbitrary element, this holds true for any element in a. Therefore, we have shown that every element of a is also an element of c.
Step 6: Finally, by definition, if every element of a is also an element of c, we can conclude that a is a subset of c (a⊆c).
By following these steps in the given order, we have logically proven that a⊆c based on the assumptions a⊆b and b⊆c.vTo prove that a⊆c if a⊆b and b⊆c, we need to arrange the steps in the correct order to construct a logical proof. The correct order is as follows:
Step 1: Start by assuming that a⊆b and b⊆c are true.
Step 2: Take an arbitrary element x and assume that x∈a.
Step 3: Using the assumption a⊆b, conclude that x∈b.
Step 4: Using the assumption b⊆c, conclude that x∈c.
Step 5: Since x was an arbitrary element, we have shown that for any element x, if x∈a, then x∈c.
Step 6: Therefore, by definition, a⊆c.
Let's briefly explain the reasoning behind each step:
Step 1: This is the initial assumption given in the problem statement. We assume that a⊆b and b⊆c are true.
Step 2: To prove that a⊆c, we need to show that every element of a is also an element of c. We start by taking an arbitrary element x and assuming that it belongs to a (x∈a).
Step 3: Using the assumption a⊆b, we know that if x∈a, then x∈b. This follows directly from the definition of subset: every element of a is also an element of b.
Step 4: Similarly, using the assumption b⊆c, we know that if x∈b, then x∈c. Again, this follows directly from the definition of subset: every element of b is also an element of c.
Step 5: Combining the conclusions from Steps 3 and 4, we can infer that if x∈a, then x∈c. Since x was an arbitrary element, this holds true for any element in a. Therefore, we have shown that every element of a is also an element of c.
Step 6: Finally, by definition, if every element of a is also an element of c, we can conclude that a is a subset of c (a⊆c).
By following these steps in the given order, we have logically proven that a⊆c based on the assumptions a⊆b and b⊆c.
Learn more about arbitrary element at: brainly.com/question/31230709
#SPJ11
Instructions: Find the measure of the indicated angle to the
nearest degree

Answers
Answer:
See below.
Step-by-step explanation:
We want to find the unknown angle and we know the sides opposite to it and the hypotenuse. Therefore, we can use sine.
Recall that sine is the ratio of the opposite to the hypotenuse:
\(\sin(\theta)=opp/hyp\)
Plug in the numbers. The opposite is 20 and the hypotenuse is 55.
\(\sin(\theta)=20/55\)
Solve for theta (use a calculator):
\(\sin(\theta)=20/55\\\theta=\arcsin(4/11)\\\theta\approx21.23\approx21\textdegree\)
In the figure shown, CF intersects AD and EH at points B and F, respectively

Answers
As we have proved that the triangles ABF and BFE are congruent using the Congruence of Triangles criteria.
To prove that the triangles ABF and BFE are congruent, we will use the Congruence of Triangles criteria. According to this criteria, if two triangles have three congruent parts, they are congruent. These parts can be angles or sides. In our case, we are given that the triangles CBD and BFE are congruent. This means that the corresponding sides and angles of these triangles are congruent.
To prove that the angle ABF is congruent to the angle BFE, we can use the fact that CF intersects AD and EH at points B and F, respectively. This means that the line CF is a transversal that intersects the parallel lines AB and FE. Therefore, we can use the Alternate Interior Angles Theorem, which states that if a transversal intersects two parallel lines, then the alternate interior angles are congruent. Thus, we can say that the angle ABF is congruent to the angle FBE.
To prove that the side AB is congruent to the side FE, we can again use the fact that CF intersects AD and EH at points B and F, respectively. This means that the line CF is a transversal that intersects the parallel lines AB and FE. Therefore, we can use the Corresponding Angles Theorem, which states that if two parallel lines are intersected by a transversal, then corresponding angles are congruent. Thus, we can say that the angles ABC and FBE are congruent, and the angles ABD and FCE are congruent.
Now, we can use the fact that CBD ≅ BFE to conclude that the angle CBD is congruent to the angle BFE, and the side CB is congruent to the side BE. Using the Transitive Property of Congruence, we can say that the angle ABF is congruent to the angle BFE, and the side AB is congruent to the side FE.
To know more about triangle here
https://brainly.com/question/8587906
#SPJ1
I NEED HELP ASAP
A student wrote and simplified an expression for the perimeter of the rectangle. (Using the picture provided.)
perimeter
Explain the mistake that was made in the simplified expression. Include the correct simplified expression in your explanation. Be sure to use math vocabulary words.


Answers
Answer:
Step-by-step explanation:
Last one
What is the value of -3*(7(+(-3)^2) dived by 3
13
-2
-16
-48
Answers
Answer:
c) -16
Step-by-step explanation:
Q) [-3 × (7 + (-3²))] ÷ 3 = ?
→ [-3 × (7 + (-3²))] ÷ 3
→ [-3 × (7 + 9)] ÷ 3
→ [-3 × 16] ÷ 3
→ -48 ÷ 3 = -16
Hence, the value is -16.
the ratio of dividends to the average number of common shares outstanding is:
Answers
The ratio of dividends to the average number of common shares outstanding is known as the dividend yield. It is a measure of the return on an investment in the form of dividends received relative to the number of shares held.
To calculate the dividend yield, you need to divide the annual dividends per share by the average number of common shares outstanding during a specific period. The annual dividends per share can be obtained by dividing the total dividends paid by the number of outstanding shares. The average number of common shares outstanding can be calculated by adding the beginning and ending shares outstanding and dividing by 2.
For example, let's say a company paid total dividends of $10,000 and had 1,000 common shares outstanding at the beginning of the year and 1,500 shares at the end. The average number of common shares outstanding would be (1,000 + 1,500) / 2 = 1,250. If the annual dividends per share is $2, the dividend yield would be $2 / 1,250 = 0.0016 or 0.16%.
In summary, the ratio of dividends to the average number of common shares outstanding is the dividend yield, which measures the return on an investment in terms of dividends received per share held.
To know more about dividend, here
brainly.com/question/3161471
#SPJ11
Find m<1 and m<2. Justify your answer.

Answers
Answer:
M<1 80 by the corresponding angles postulate
Step-by-step explanation:
I need to be able to show work
i.
ii.
iii.
are the steps i’m supposed to used but I don’t know the answer

Answers
The equation 1 + 4 + 9 + ... + n² = n(n + 1)(2n + 1) / 6 is proven by mathematical induction.
We have,
To prove the equation 1 + 4 + 9 + ... + n² = n(n + 1)(2n + 1) / 6 using mathematical induction,
We will follow the three steps of mathematical induction:
The base case, the induction hypothesis, and the inductive step.
Step 1: Base case
Let's start by checking if the equation holds true for the base case, which is n = 1.
When n = 1, the left-hand side (LHS) is 1² = 1, and the right-hand side (RHS) is 1(1 + 1)(2(1) + 1) / 6 = 1.
Since LHS = RHS for the base case, the equation holds true.
Step 2: Induction hypothesis
Assume the equation holds true for some positive integer k, where k ≥ 1. This is our induction hypothesis:
1 + 4 + 9 + ... + k² = k(k + 1)(2k + 1) / 6
Step 3: Inductive step
We need to prove that if the equation holds true for k, it also holds true for k + 1.
Starting with the left-hand side of the equation, we add (k + 1)² to both sides:
1 + 4 + 9 + ... + k² + (k + 1)² = k(k + 1)(2k + 1) / 6 + (k + 1)²
Simplifying the right-hand side:
= [k(k + 1)(2k + 1) + 6(k + 1)²] / 6
= [(2k³ + 3k² + k) + (6k² + 12k + 6)] / 6
= (2k³ + 9k² + 13k + 6) / 6
= [(k + 1)(k + 2)(2k + 3)] / 6
We can see that the right-hand side is now in the form
(k + 1)((k + 1) + 1)(2(k + 1) + 1) / 6, which matches the equation for k + 1.
Since the equation holds true for k implies it holds true for k + 1, and the base case is true, we have proven the equation using mathematical induction.
Therefore,
The equation 1 + 4 + 9 + ... + n² = n(n + 1)(2n + 1) / 6 is proven by mathematical induction.
Learn more about mathematical induction here:
https://brainly.com/question/29503103
#SPJ1
HELP!! Find the value of x in the isosceles triangle shown below.

Answers
2-3 times how much is 2-1?
Answers
Answer:
the answer is -1
Step-by-step explanation:
2-3=-1
2-1=1
-1x=1
x=-1
-1*-1=1
pleaseeeeeeeeeeeeeeee helppppppppppppp pleaaeseeee fasttttt pleaseeee hellpppp

Answers
PLEASE HELP ASAP:(.....

Answers
Answer:
5
Step-by-step explanation:
23 - 10 = 13
13 - 8 = 5
Answer:
5
Step-by-step explanation:
p = a + b + c
23 = 8 + 10 + c
23 = 18 + c
5 = c
I could use help please

Answers
just designed a cloister (a rectangular garden surrounded by a covered walkway on all four sides). the outside dimensions of the garden are 12 feets by 8 feet, and the area of the garden and the walkway together are 252 square feet. what is the width of the walkway?
Answers
The width of the rectangular walkway is 3 feet around.
The walkway has a uniform width around the rectangle.
Let the width of walkway be x
The total length of the garden and walkway will be (12+2x) feet
The total width of the walkway and garden would be (8+2x) feet
Area of the garden and the walkway together are 252 square
Let’s express this mathematically:
(8+2x)(12+2x) = 252
Let’s open the brackets;
96+ 16x+ 24x + 4x^2 = 252
4x^2 + 40x + 96 = 252
4x^2 + 40x + 96-252 = 0
4x^2 + 40x - 156 = 0
Let’s divide all through by 10. We have;
x^2 + 10x -39 = 0
X^2 +13x-3x-39 = 0
x(x + 13) -3(x+ 13) = 0
(x-3)(x + 13) = 0
x -3 = 0 or x + 13 = 0
x = 3 or -13
We ignore x = -13 since width cannot be negative in size
Therefore, the width of the rectangular walkway is 3 feet around.
To learn more about area refer here
https://brainly.com/question/25292087
#SPJ4
I'm very confused on this....please answer thank you!!

Answers
Explanation:
Y=|3/4x| is an absolute value
Y=x^3+8 is quadratic
Y=8+5/6x^2 is also quadratic (you can tell it’s quadratic because it has an exponent)
A company ships a cube with side lengths of 2 feet in a crate that is a larger cube with side lengths of 4 feet. Packing material is placed around the smaller cube.
Which expression can be used to find the volume of the part of the larger cube that is filled with packing material?
A. 4²-2²
B. 4³-2³
C. (4-2)²
D. (4-2)³
Answers
Answer:
yeah tbh i wanna know to
Step-by-step explanation:
sorry
Points that are on the same line are collinear. Use the definition of slope to determine whether the given points are collinear.
(-2,6),(0,2),(1,0)
Answers
Using the definition of slope, the points (-2 , 6), (0 , 2), and (1 , 0) are collinear.
Three or more points are said to be collinear if they lie on a single straight line.
To determine whether the given points are collinear, use the definition of slope. The slope, m, determines the steepness of a line. It can be measured by the vertical distance from one point to another divided by the horizontal distance of the same points.
m = (y2 - y1)/(x2 - x1)
Getting the slope of each pair of points:
(-2 , 6) and (0 , 2)
m = (y2 - y1)/(x2 - x1)
m = (2 - 6)/(0 - -2)
m = -4/2
m = -2
(0 , 2), and (1 , 0)
m = (y2 - y1)/(x2 - x1)
m = (0 - 2)/(1 - 0)
m = -2/1
m = -2
(-2 , 6) and (1 , 0)
m = (y2 - y1)/(x2 - x1)
m = (0 - 6)/(1 - -2)
m = -6/3
m = -2
Since collinear points lies on the same line, the slope of any pair of points should be the same. Since the slopes of each pair of points is equal with each other, then they are collinear.
Learn more about collinear points here: https://brainly.com/question/17314715
#SPJ4

15 is what percent of 40
Answers
The correct representation of the sentence 15 is what percent of 40 is 15 is 37.5% of 40.
What are percentages?A value or ratio that may be stated as a fraction of 100 is referred to as a percentage in mathematics. If we need to calculate a percentage of a number, we should divide it by its entirety and then multiply it by 100. The proportion therefore refers to a component per hundred. Per 100 is what the word percent means. The letter "%" stands for it.
The algebraic representation of the statement 15 is what percent of 40 is:
40 (x/100) = 15
40x / 100 = 15
40x = 1500
x = 1500/40
x = 37.5
Hence, the correct representation of the sentence 15 is what percent of 40 is 15 is 37.5% of 40.
Learn more about percentage here:
https://brainly.com/question/29306119
#SPJ1
how much increase from 40$ to 50$ in percentages
Answers
Answer:
25%
Step-by-step explanation:
\(\frac{New Value - Original}{Original} = Percent gain\)
\(\frac{50 - 40}{40}\) = Percent gain
\(\frac{10}{40}\) = Percent gain
\(\frac{10}{40}=\frac{1}{4} = 0.25\)
0.25 multiply by 100 = 25
25%
Carson owes Nigel $110. If Carson repays this debt at a rate of $15 per week, which graph best represents when, in weeks, Carson’s debt will be less than $50?
Responses
Answers
After doing the equitation the at rate of $15 per week.
How to do graph representation?
Graph representation is a way of visualizing data as a graph or chart. It is used to easily identify relationships between data points and to illustrate trends in the data. Graphs can be used to present data in a variety of ways, such as bar graphs, line graphs, pie charts, scatter plots and more. Graphs are especially useful when trying to compare two or more sets of data and to highlight any patterns or trends that may exist. Additionally, graphs are often used to represent numerical data in a more visually appealing way. By using graphs, it is easier to identify relationships, trends and outliers in the data. Graphs also help to make data easier to interpret and understand.
Carson owes = $110
At rate of $15 per week.
The graph representation will follows:
To know more about Graph Representation visit,
https://brainly.com/question/28732353
#SPJ1
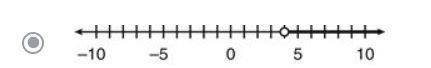
i. The uniform probability distribution's shape is a rectangle. ii. The uniform probability distribution is symmetric about the mode. iii. In a uniform probability distribution, P(x) is constant between the distribution's minimum and maximum values. Multiple Choice (ii) and, (iii) are correct statements but not (i). (i). (ii), and (iii) are all false statements. (i) is a correct statement but not (ii) or (iii). (i) and, (iii) are correct statements but not (ii).
Answers
The correct option is: (i) and (iii) are correct statements but not (ii).
The correct answer is:
(i) The uniform probability distribution's shape is a rectangle.
(ii) The uniform probability distribution is symmetric about the mode.
(iii) In a uniform probability distribution, P(x) is constant between the distribution's minimum and maximum values.
Therefore, the correct option is: (i) and (iii) are correct statements but not (ii).
To know more about "Probability" refer here:
brainly.com/question/30034780#
#SPJ4
In the given figure QR ∥AB, RP∥ BD,CQ=x+2,QA=x,CP=5x+4,PD=3x.The value of x is
Answers
Answer:
where is the figure and without figure what to do
helppppppppppppp plssssssssssssssssssssssss

Answers
Solve the system by any method.
(3x – 4y = -5
(5x + 8y = -1

Answers
Answer:
( x , y ) = ( -1,1/2)
Step-by-step explanation:
Solve the equation for X
\(\left \{ {{x=-5/3+4/3y} \atop {5x+8y=-1}} \right.\)
Substitute the given value of x into the equation 5x + 8y = -1
5(-5/3+4/3y)+8y=-1
Solve the equation for Y
y=-1/2
Substitute the given value of y into the equation x = -5 / 3 + 4 / 3y
x=-5/3+4/3×1/2
Solve the equation for X
x=-1
The system solution is the computer pair
(x,y)=(-1,1/2)
Check if the ordered pair given to the solution of the system of equations
\(\left \{ {{3*(-1)-4*1/2=-5} \atop {5*(-1)+8*1/2=-1}} \right.\)
Simplify the equations
\(\left \{ {{-5=-5} \atop {-1=-1}} \right.\)
The ordered pair is the solution of the system of equations, since both equations are true
(x,y)=(-1,1/2)
Simplify the expression.
cube root of 9y^4 minus cube root of y
Thank you.
Answers
The simplified expression is\((3y^2 - 1)\)· cube root of y.
The given expression is cube root of 9y^4 minus cube root of y.
We can express \(\(9y^4\)\) as \(\((3y^2)(3y^2)(y)\)\).
Hence, we can rewrite the cube root of \(\(9y^4\)\) as \(\(3y^2\)\) times the cube root of \(\(y\)\), i.e.,
cube root of \(\(9y^4\) = \(3y^2\)\) times cube root of \(\(y\)\).
We can now substitute this expression into the original expression and obtain:
Cube root of \(\(9y^4\)\) minus cube root of \(\(y\) = \(3y^2\)\) times cube root of \(\(y\)\) minus cube root of \(\(y\) = \((3y^2 - 1)\)\) times cube root of \(\(y\)\).
Therefore, the simplified expression is \(\((3y^2 - 1)\)\) times cube root of \(\(y\)\).
cube root of y
We can now substitute this expression into the original expression and obtain:
cube root of \(9y^4\)- cube root of \(y = 3y^2\) · cube root of y - cube root of \(y = (3y^2 - 1)\) · cube root of y
Therefore, the simplified expression is\((3y^2 - 1)\)· cube root of y.
To know more about cube root visit:
https://brainly.com/question/12726345
#SPJ11
Given the function defined in the table below, find the average rate of change, in
simplest form, of the function over the interval 5 ≤ x ≤9.
7
8
9
6
x
4
5
f(x)
4
8
16
32
64
medically explained PAINL X
128
Deltal/ath
Answers
The average rate of change, in simplest form, is -2/5.
What is average rate ?
Divide the change in y-values by the change in x-values to determine the average rate of change. Identifying changes in quantifiable parameters like average speed or average velocity calls for the knowledge of the average rate of change.
The rate of change of the function is its gradient or slope.
The formula for calculating the gradient of a function is expressed as:
\(m=\frac{d y}{d x}=\frac{y_2-y_1}{x_2-x_1}$$\)
Using the coordinate points from the table (0,41) and (15,35)
Substitute the coordinate into the expression:
\($$\begin{aligned}& m=\frac{35-41}{15-0} \\& m=\frac{-6}{15} \\& m=\frac{-2}{5}\end{aligned}$$\)
To learn more about average rate visit:https://brainly.com/question/3605446
#SPJ1
how many teenagers (people from ages 13-19) must you select to ensure that 4 of them were born on the exact same date (mm/dd/yyyy)? simplify your answer to an integer.
Answers
Assuming that there are 365 days in a year (ignoring leap years) and that all dates are equally likely, we can use the Pigeonhole Principle to determine the minimum number of teenagers needed to ensure that 4 of them were born on the same date.
There are 365 possible days in a year on which a person could be born. Therefore, if we select k teenagers, the total number of possible birthdates is 365k.
To guarantee that 4 of them were born on the exact same date, we need to find the smallest value of k for which 365k is greater than or equal to 4 times the number of possible birthdates. In other words:365k ≥ 4(365)
Simplifying this inequality, we get: k ≥ 4
Therefore, we need to select at least 4 + 1 = 5 teenagers to ensure that 4 of them were born on the exact same date.
To know more about "Pogeonhole Principle" refer here:
https://brainly.com/question/31687163#
#SPJ11
