(Approximate e) Approximate e using the following series: -- 1+ () + 6 + )+6) + 6) +-+) In order to get better precision, use BigDecimal with 25 digits of precision in the computation. Write a program that displays the e value for i= 100, 200, ..., 1000.
Answers
This code uses BigDecimal and a loop to approximate the value of e for i = 100, 200, ..., 1000 with 25 digits of precision. The loop iterates over each value of i, and the inner loop calculates the sum of the series by dividing 1 by the factorial of each term.
To approximate e using the given series, we can use the formula:
e = 1 + 1/1! + 1/2! + 1/3! + ...
In the given series, we can see that each term is of the form:
1/n! * (n^2 + n + 1)
So, we can rewrite the formula for e as:
e = 1 + 1/1! * (1^2 + 1 + 1) + 1/2! * (2^2 + 2 + 1) + 1/3! * (3^2 + 3 + 1) + ...
Now, to compute the value of e for different values of i, we can write a Java program that uses the BigDecimal class with 25 digits of precision. Here's a sample program:
import java.math.BigDecimal;
public class ApproximateE {
public static void main(String[] args) {
for (int i = 100; i <= 1000; i += 100) {
BigDecimal e = BigDecimal.ONE;
BigDecimal term = BigDecimal.ONE;
for (int j = 1; j <= i; j++) {
term = term.multiply(new BigDecimal(j*j + j + 1)).divide(new BigDecimal(j), 25, BigDecimal.ROUND_HALF_UP);
e = e.add(term);
}
System.out.println("e for i = " + i + " is " + e);
}
}
}
In this program, we use a nested loop to compute the value of e for different values of i. In the inner loop, we compute the value of each term in the series using the BigDecimal class with 25 digits of precision. We then add each term to the previous terms to get the final value of e. Finally, we print out the value of e for each value of i.
To learn more about sum of the series click here
brainly.com/question/8919590
#SPJ11
Related Questions
How would you find the two triangles are similar?

Answers
Answer:
The answer should be A.
Step-by-step explanation:
Well, to determine how two triangles are similar you first look at the image of what they give you.
As you can see the images shows only degree angles.
Looking at your option to see degree angles.
In some options, there is a side length of a triangle, but we aren't given a side length on both triangles so that answer does that apply.
Our last option is between A and D.
D is false as we determine the triangles are similar to each other.
RCW and VCB are vertically opposite meaning their 25° are the same to each other.
We know that every triangle adds up to 180.
y=7x-4
- 14x+2y=-8
Is it a solution?

Answers
Answer: No it is not a solution
Step-by-step explanation:
-14x-2=28
2x5=10
28+10=38
Given the system of equations below. Use the Inverse of the matrix method to solve. x+2y+3z=11
2x+4y+5z=21
3x+5y+6z=27
Answers
The solution of the given system of equations is x = -4, y = 5 and z = 2 is the answer.
The system of equations given below:x + 2y + 3z = 11;2x + 4y + 5z = 21;3x + 5y + 6z = 27.
Here, we will solve this system of equations using inverse of the matrix method as follows:
We can write the given system of equations in matrix form as AX = B where, A = [1 2 3; 2 4 5; 3 5 6], X = [x; y; z] and B = [11; 21; 27].
The inverse of matrix A is given by the formula: A-1 = (1/ det(A)) [d11 d12 d13; d21 d22 d23; d31 d32 d33] where,
d11 = A22A33 – A23A32 = (4 × 6) – (5 × 5) = -1,
d12 = -(A21A33 – A23A31) = -[ (2 × 6) – (5 × 3)] = 3,
d13 = A21A32 – A22A31 = (2 × 5) – (4 × 3) = -2,
d21 = -(A12A33 – A13A32) = -[(2 × 6) – (5 × 3)] = 3,
d22 = A11A33 – A13A31 = (1 × 6) – (3 × 3) = 0,
d23 = -(A11A32 – A12A31) = -[(1 × 5) – (2 × 3)] = 1,
d31 = A12A23 – A13A22 = (2 × 5) – (3 × 4) = -2,
d32 = -(A11A23 – A13A21) = -[(1 × 5) – (3 × 3)] = 4,
d33 = A11A22 – A12A21 = (1 × 4) – (2 × 2) = 0.
We have A-1 = (-1/1) [0 3 -2; 3 0 1; -2 1 0] = [0 -3 2; -3 0 -1; 2 -1 0]
Now, X = A-1 B = [0 -3 2; -3 0 -1; 2 -1 0] [11; 21; 27] = [-4; 5; 2]
Therefore, the solution of the given system of equations is x = -4, y = 5 and z = 2.
know more about matrix method
https://brainly.com/question/32106604
#SPJ11
evaluate the integral by reversing the order of integration. 4 0 12 11ex2 dx dy 3y
Answers
To evaluate the integral by reversing the order of integration, we first need to draw the region of integration. From the given limits of integration, we can see that the region is a rectangle with vertices at (0,4), (0,12), (11,4), and (11,12).
Now, we can reverse the order of integration by integrating with respect to y first, and then x. The new limits of integration will be y = 4 to y = 12 and x = 0 to x = 11e^(2y/3).
So, the new integral will be:
∫(0 to 11) ∫(4 to 12) 3y e^(2x/3) dy dx
We can evaluate this integral using integration by parts. Integrating with respect to y gives us:
∫(0 to 11) [3y^2/2 e^(2x/3)] from y = 4 to y = 12
Simplifying this expression gives us:
∫(0 to 11) [36e^(2x/3) - 6e^(8x/3)]/2 dx
Now, integrating with respect to x gives us:
[27e^(2x/3) - 9e^(8x/3)] from x = 0 to x = 11
Substituting these values and simplifying gives us the final answer:
(27e^22/3 - 9e^88/3) - (27 - 9) = 27e^22/3 - 9e^88/3 - 18
To know more about integration visit:
https://brainly.com/question/31744185
#SPJ11
Jack took out a 6-year loan for $25,000 to purchase a boat at a 4.5% interest rate. If the interest is compounded monthly, what will he have paid total over the course of the loan?
Answers
Answer: $32,732.58
Step-by-step explanation:
To calculate the total loan payment over the course of the loan period, use the future value formula:
= Loan amount * (1 + rate) ^ number of years
As this loan is compounded monthly, you need to convert certain terms to monthly figures:
Number of periods = 6 * 12 months = 72 months
Interest = 4.5 / 12 = 0.375%
Total payment:
= 25,000 * ( 1 + 0.375%)⁷²
= $32,732.58
He will have to pay $34,236.31 over the course of the loan.
Using the compound interest formula expressed as:
\(A =P(1+r/n)^{nt}\)
P is the principal = $25000
r is the rate = 4.5% = 0.045
t is the time in years = 6 years
n is the compounding amount = (12)monthly
Substitute into the formula:
\(A =25000(1+0.045/12)^{12(7)}\\A=25000(1.36945)\\A = \$34,236.31\)
Hence he will have to pay $34,236.31 over the course of the loan
Learn more on compound interest here: https://brainly.com/question/24274034
n element with mass 570 grams decays by 18% per minute. How much of the element is remaining after 13 minutes, to the nearest 10th of a gram?
Answers
Answer:
Step-by-step explanation:
Exponential Functions:
y=ab^x
a=starting value=570
r=rate=18%=0.18
Exponential Decay:
b = 1 - r = 1 - 0.18 = 0.82
Write Exponential Function: put it all together
y=570(0.82)^13
Plug in time for x:
y=570(0.82)^13
y=43.197134 evaluate
ANSWER: y≈43.2 round
what is 40% of 4x-10?
Answers
A dime weighs about 0.08 ounce. About how many dimes weigh a pound?
(I will mark brainliest)
Answers
Answer:
200
Step-by-step explanation:
Well, 1 pound is 16 ouces, so you would just need to do 16/0.08 and it should give you the awnser!
16/0.08=200
200 dimes would be a pound
Answer:
200 dimes = 16 ounces
step-by-step explanation:
16 ounces in a pound
16 = .08 * d where d = number of dimes
d = 16/.08 = 200 dimes
Solve for x.
X
A
=
2
C
1
B
1
E
X
D
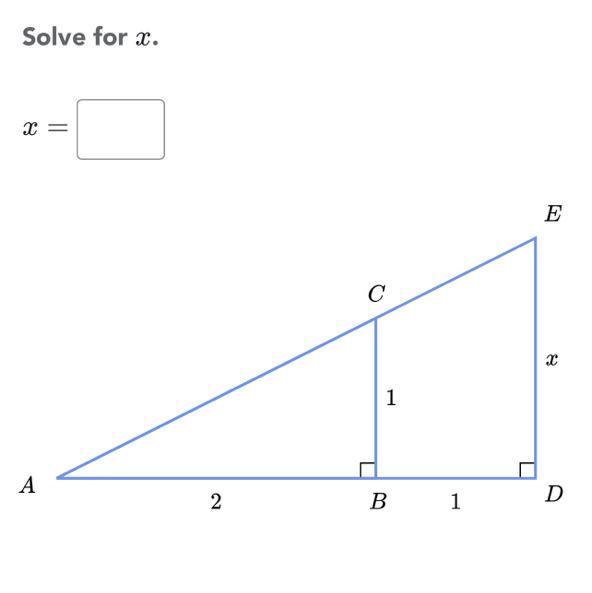
Answers
Step-by-step explanation:
ABC and ADE are similar triangles.
that means they have the same angles, and there is one scaling factor for all sides from one triangle to the other.
so, we see that AB = 2, AD = 2+1 = 3
the scaling factor f from AB to AD is then
AB × f = AD
2 × f = 3
f = 3/2
the same scaling factor now applies between BC and DE (= x).
BC × f = x
1 × 3/2 = x
x = 3/2 = 1.5
When a new machine is functioning properly, only 6% of the items produced are defective. Assume that we will randomly select two parts produced on the machine and that we are interested in the number of defective parts found.b. How many experimental outcomes result in exactly one defect being found?c. Compute the probabilities associated with finding no defects, exactly one defect, and two defects (to 4 decimals).P (no defects)P (1 defect)P (2 defects)
Answers
b)There are 0.10608 experimental outcomes that result in exactly one defect being found.
c) The probabilities of finding no defects, exactly one defect, and two defects are:
P (no defects) = 0.8836
P (1 defect) = 0.10608
P (2 defects) = 0.0036
b. To find the number of experimental outcomes that result in exactly one defect being found:
We can use the binomial distribution formula. The formula is:
\(P(x) = (n choose x) * p^x * (1-p)^(n-x)\)
where:
- P(x) is the probability of finding exactly x defects
- n is the total number of parts we select (in this case, n = 2)
- p is the probability of finding a defect in one part (in this case, p = 0.06)
- (n choose x) is the binomial coefficient, which represents the number of ways to choose x items out of n.
So for exactly one defect, we have:
\(P(1) = (2 choose 1) * 0.06^1 * (1-0.06)^(2-1) = 2 * 0.06 * 0.94 = 0.10608\)
Therefore, there are 0.10608 experimental outcomes that result in exactly one defect being found.
c. To compute the probabilities associated with finding no defects, exactly one defect, and two defects:
We can use the same binomial distribution formula with different values of x:
- P(no defects): x = 0
\(P(0) = (2 choose 0) * 0.06^0 * (1-0.06)^(2-0) = 1 * 1 * 0.8836 = 0.8836\)
Therefore, the probability of finding no defects is 0.8836.
- P(1 defect): we already calculated this in part b.
P(1) = 0.10608
Therefore, the probability of finding exactly one defect is 0.10608.
- P(2 defects): x = 2
\(P(2) = (2 choose 2) * 0.06^2 * (1-0.06)^(2-2) = 1 * 0.0036 * 1 = 0.0036\)
Therefore, the probability of finding two defects is 0.0036.
In summary, the probabilities of finding no defects, exactly one defect, and two defects are:
P (no defects) = 0.8836
P (1 defect) = 0.10608
P (2 defects) = 0.0036
To know more about Probability:
https://brainly.com/question/30034780
#SPJ11
Easy question? Please help I’ll give brainliest! :( Please provide small explanation like instructions it’s okay if not though

Answers
Answer: Ok so what i'v got for this one is that i averaged up all three numbers and i got an average of about 85%. Now you have to put in a fourth number which will actually be another 80% i believe
Step-by-step explanation: add up all 3 divide them all by the numbers then once your done with that plug in the 80% and see what your answer will be.
Find the area of this triangle. Round to the nearest tenth.

Answers
The area of the triangle rounded to the nearest tenth is 33.3 squared inches.
What is the area of the triangle?
Given the data in the diagram;
Angle B = 133°Side a = 7Side c = 13Side b = ?Angle C = ?First we find the dimension of side b.
From the rule of cosines.
b = √[ a² + c² - 2acCosB ]
We substitute into the formula.
b = √[ 7² + 13² - ( 2 × 7 × 13 × cos( 133° ) ]
b = √[ 49 + 169 - ( 182 × cos( 133° ) ) ]
b = √[ 218 - 182×cos( 133° ) ]
b = √[ 342.1237 ]
b = 18.5
Next, we find angle C.
From rule of cosines.
cosC = [ b² + a² - c² ] / 2ba
cosC = [ 18.5² + 7² - 13² ] / [ 2 × 18.5 × 7 ]
cosC = [ 342.25 + 49 - 169 ] / [ 259 ]
cosC = [ 222.25 ] / [ 259 ]
cosC = [ 0.8581 ]
C = cos⁻¹[ 0.8581 ]
C = 30.9°
Now, we can find the area of the triangle.
Area = [ ab × sinC ] / 2
Area = [ 7 × 18.5 × sin( 30.9 ) ] / 2
Area = [ 129.5 × 0.51354 ] / 2
Area = 66.5 / 2
Area = 33.3 in²
The area of the triangle rounded to the nearest tenth is 33.3 squared inches.
Learn about cosine rule here: brainly.com/question/20839703
#SPJ1

*PLEASE ANSWER!!! THANK YOU*
Greg is a pharmaceutical sales rep who works on commission. He needs to sell his product to at least 5 doctors each month. What is the term that represents needing to sell a certain amount before getting paid?
a.) Quota
b.) Salary
c.) Gross pay
Answers
Answer:
A
Step-by-step explanation:
Graph the following:
(x - 1)2 + (y - 3)2 = 4
Center
Radius
Answers
Answer:
Center = (1,2) radius = 2
Step-by-step explanation:
I used Demos to graph the circle.
Then I added two line to find the center of the circle
The radius is 2
outside a home, there is -key keypad with letters that can be used to open the garage if the correct -letter code is entered. each key may be used only once. how many codes are possible
Answers
The number of possible codes depends on the number of keys on the keypad and can be calculated using factorial notation: n!, where n is the number of keys.
the keypad has a certain number of keys, and each key can be used only once in the code. This implies that the code length is equal to the number of keys on the keypad.
To calculate the number of possible codes, we can use the concept of permutations. In a permutation, the order of the elements matters, and repetition is not allowed.
If there are n keys on the keypad, the first key can be chosen in n ways. After selecting the first key, the second key can be chosen from the remaining (n-1) keys in (n-1) ways. Similarly, the third key can be chosen in (n-2) ways, and so on.
Therefore, the total number of possible codes is given by the product of these choices: n * (n-1) * (n-2) * ... * 2 * 1, which is equal to n factorial (n!).
For example, if the keypad has 4 keys, the number of possible codes would be 4 factorial (4!) = 4 × 3 × 2 × 1 = 24. So, there would be 24 different codes that can be entered using the available keys on the keypad.
Learn more about factorial here:
https://brainly.com/question/30136880
#SPJ11
a normal population has a mean of $60 and standard deviation of $12. you select random samples of nine. required: a. apply the central limit theorem to describe the sampling distribution of the sample mean with n
Answers
The sampling distribution of the sample mean with n would have a mean of $60 (same as the population mean) and a standard error of $4.
The Central Limit Theorem states that as the sample size increases, the sampling distribution of the sample mean approaches a normal distribution, regardless of the shape of the original population. In this case, you have a normal population with a mean of $60 and a standard deviation of $12.
To apply the Central Limit Theorem to describe the sampling distribution of the sample mean with n, we need to calculate the standard deviation of the sampling distribution (also known as the standard error).
The formula to calculate the standard error is: standard deviation / square root of sample size.
In this case, the standard deviation is $12 and the sample size is 9.
So, the standard error would be $12 / √9 = $12 / 3 = $4.
Therefore, the sampling distribution of the sample mean with n would have a mean of $60 (same as the population mean) and a standard error of $4.
Please note that the Central Limit Theorem applies when the sample size is large enough (typically n > 30), but it can still provide a reasonable approximation even for smaller sample sizes.
Learn more about standard error'
https://brainly.com/question/32854773
#SPJ11
A buyer secured a $125,000 loan at 4.5% interest rate. to receive a lower interest rate, the buyer was required to buy down 3 discount points. how much were the discount points?
having trouble solving please help!
Answers
A buyer secured a $125,000 loan at 4.5% interest rate. To receive a lower interest rate, the buyer was required to buy down 3 discount points. The amount of the discount points can be calculated by multiplying the loan amount by the number of points and then dividing by 100. In this case, the discount points would be $3,750.
How much did the buyer have to pay for the discount points in order to secure a lower interest rate on their $125,000 loan?The buyer had to pay $3,750 in discount points to secure a lower interest rate on their $125,000 loan.
When a buyer is required to buy down discount points, it means that they pay a certain percentage of the loan amount upfront to reduce the interest rate on the loan. In this case, the buyer had to purchase 3 discount points. Each discount point is equal to 1% of the loan amount. So, to calculate the amount of the discount points, we multiply the loan amount ($125,000) by the number of points (3) and then divide by 100. This gives us a total of $3,750 for the discount points.
Learn more about Discount points
brainly.com/question/30239477
#SPJ11
since yall are smart uh i need help so
What is the difference between the 15th square number and the 3rd square number?
Answers
Answer:
216
Step-by-step explanation:
15^2=225
3^2=9
225-9=216
What is the measure of ZXYZ?
X
112
y
A. 170
V
O B. 72°
55°
C. 55°
w
D. 36°
Answers
Answer: D. 36
Step-by-step explanation: Angle subtended by an arc at the centre is twice of the angle subtended at any point on the circle . And in the given diagram, angle subtended on the circle by arc XZ=60 degree . So arc XZ =2*60=120. Therefore measurement of arc xyz=360-120=240 degree.
The measurement of arc xyz is 240 degrees.
What is Trigonometry?Trigonometry is a branch of mathematics that studies relationships between side lengths and angles of triangles.
Angle subtended by an arc at the centre is twice of the angle subtended at any point on the circle .
In the given diagram, angle subtended on the circle by arc XZ=60 degree .
So arc XZ =2 times of 60
=2×60
=120.
The measurement of arc xyz=360-120=240 degree.
Hence, the measurement of arc xyz is 240 degrees.
To learn more on trigonometry click:
https://brainly.com/question/25122835
#SPJ7

The proportional relationship between the number of songs Nolan downloads, s, and the total cost in dollars and cents, c, can be represented by the equation c = 0. 35s. How much does it cost to download a single song?
Please I need help!!!
Answers
A single song may be downloaded for $0.35. Adjusting the solution c = 0.35s yields the answer because c/s = 0.35.
Equation: What is it?An equation is an mathematical statement that uses an equal sign to express the relationship across two or more objects, such as variables or integers. Equations can be used to represent relationships between physical, chemical, and other sorts of events as well as to solve mathematical problems. An equation in mathematics is a claim made regarding the equivalence of two expressions. A assertion claiming two expressions equal equal is what an equation is, in other words.
This indicates that each music download costs $0.35. Consequently, it costs $0.35 to buy a single song.
To know more about Equation visit:
https://brainly.com/question/22688504
#SPJ4
Which value of x makes the equation true?
X - ⅔ = ⅔
Answers
Answer:
4/3
Step-by-step explanation:
Please let me know if you want me to add an explanation as to why this is the answer. I can definitely do that, I just wouldn’t want to write it if you don’t want me to :)
Answer:
x = \(\frac{4}{3}\)
Step-by-step explanation:
Given
x - \(\frac{2}{3}\) = \(\frac{2}{3}\) ( isolate x by adding \(\frac{2}{3}\) to both sides )
x = \(\frac{4}{3}\)
Pretend you have been given 1 million dollars and must spend it at the rate of $1 per second. How long will it take you to spend it all
Answers
It will take almost 11.57 days to spend 1 million dollars if I must spend it at the rate of $1 per second.
We know that,
1 minutes = 60 seconds
1 hour = 60 minutes
1 day = 24 hours
Hence, we can write the conversion as,
1 day = 24*60*60 = 86400 seconds
Hence, I will spend $ 86400 in 86400 seconds or in a day.
We know that,
1 million dollars = $ 1,000,000
Hence,
Time taken by me to spend 1 million dollars if I spend at the rate of $1 per second = 1000000/86400 = 11.57 days.
To learn more about conversion, here:-
https://brainly.com/question/3477680
#SPJ1
let us suppose that sixteen adult polar bears are weighed in an attempt to estimate the average weight of all adult polar bears. the standard deviation of the population of weights is not known, so a t-interval will be reported. what will be the degrees of freedom for the t-procedure?
Answers
Answer:
your mom + your mom = big mama there you go your welcome
Step-by-step explanation:
your mom + your mom = big mama there you go your welcome
please help!!!!!!!!!!!!!!!
this

Answers
Answer: B A
Step-by-step explanation:
Let X denote the distance (m) that an animal moves from its birth site to the first territorial vacancy it encounters. Suppose that for banner-tailed kangaroo rats, X has an exponential distribution with parameter λ = .01386(as suggested in the article "Competition and Dispersal from Multiple Nests," Ecology, 1997: 873–883).
a. What is the probability that the distance is at most 100 m? At most 200 m? Between 100 and 200 m?
b. What is the probability that distance exceeds the mean distance by more than 2 standard deviations?
c. What is the value of the median distance?
Answers
The solutions and probabilities are,
(a)
P(X < 100) = 0.748
P(X < 200) = 0.936
P(100 < X < 200) = 0.188
(b) P(X > M+2σ) = 0.0498
(c) Median = 50.01 m
Given data,
Let X denote the distance (m) that an animal moves from its birth site to the first territorial vacancy it encounters. Suppose that for banner-tailed kangaroo rats, X has an exponential distribution with parameter,
λ = 0.01386
\(F(x) = 1 - e^-^λ^x\)
(a)
The probability that the distance is at most 100 m is,
\(F(100) = 1 - e^-^0^.^0^1^3^8^*^1^0^0\) = 0.748
The probability that the distance is at most 200 m is,
\(F(200) = 1 - e^-^0^.^0^1^3^8^*^2^0^0\) = 0.936
The probability that the distance is between 100 and 200 m is,
F(200) - F(100) = 0.936 - 0.748 = 0.188
(b)
The probability that distance exceeds the mean distance by more than 2 standard deviations
Mean, μ = 1/λ = 1/0.01386 = 72.1501
Standard deviation, σ = 1/λ = 72.1501
Value more than 2 SD from mean = µ + 2*σ
= 72.1501 + 2*72.1501 = 216.4503
Probability of greater than 216.4503,
P(X > 216.4503) = e^(-0.0139 * 216.4503)
= 0.0498
(c)
The value of the median distance is,
Median = ln(2) / λ
= ln(2) / 0.0138 = 50.01
To learn more about probability click here:
brainly.com/question/11234923
#SPJ4
Hi can someone help me with Qs 11 & 12 or the one you know pls !
-
Solve for “x” in each problem below (#11 and #12) Round to nearest TENTH.

Answers
Answer:
Step-by-step explanation:
sin 32 degree = x/48
0.529*48 = x
25.392 = x
x = 25.4 (after converting to the nearest tenth)
tan 62 degree = 25/x
1.88 = 25/x
x = 25/1.88
x = 13.29
x = 13.3 (after converting to the nearest tenth)
Help me please I don't know how to solve this problem.

Answers
The sum of two numbers is 38. The larger number is 22 more than the smaller number. What are the numbers?
Answers
Answer:
Larger number = 30
Smaller number = 8
Step-by-step explanation:
Let x = larger number
Let y = smaller number
x + y = 38 ————> (1)
x = y + 22 ————> (2)
Substitute for x using first equation
(y + 22) + y = 38
2y + 22 = 38
2y = 16
y = 8
Substitute for y using second equation
x = 8 + 22
x = 30
I hope this helped and please mark me as brainliest!
a consignment of 12 electronic components contains 1 component that is faulty. two components are chosen randomly from this consignment for testing. a. how many different combinations of 2 components could be chosen? b. what is the probability that the faulty component will be chosen for testing?
Answers
Answer:
a. The number of different combinations of 2 components that can be chosen from a group of 12 is given by the formula:
nC2 = (n!)/(2!(n-2)!), where n is the total number of components
Substituting n = 12, we get:
nC2 = (12!)/(2!(12-2)!) = (12 x 11)/2 = 66
Therefore, there are 66 different combinations of 2 components that can be chosen from the group of 12.
b. The probability that the faulty component will be chosen for testing depends on the number of ways in which the faulty component can be chosen, and the total number of ways in which any 2 components can be chosen.
The probability of choosing the faulty component on the first pick is 1/12, as there is one faulty component out of a total of 12 components.
After the first component has been picked, there will be 11 components left, including one faulty component. Therefore, the probability of picking the faulty component on the second pick, given that the first pick did not pick the faulty component, is 1/11.
Therefore, the probability of picking the faulty component on either the first or second pick is:
P(faulty component) = P(faulty on first pick) + P(faulty on second pick, given not picked on first pick)
P(faulty component) = (1/12) + ((11/12) x (1/11))
P(faulty component) = 1/12 + 1/12
P(faulty component) = 1/6
Therefore, the probability of choosing the faulty component for testing is 1/6 or approximately 0.1667.
The density of a certain material is such that it weighs 5 ounces per gallon of volume. Express this density in pounds per cubic meter. Round your answer to the nearest tenth.
Answers
Answer:
82.6 pounds per cubic meter
Step-by-step explanation:
