(9 points) Find the directional derivative of f(x, y, z) = yx + z4 at the point (2,3,1) in the direction of a vector making an angle of some with V f(2,3,1). f =
Answers
The directional derivative of f at the given point in the direction of v can be calculated as D_v(f) = ∇f(2, 3, 1) ⋅ (v / ||v||).
In this case, we have the function f(x, y, z) = yx + z^4 and we want to find the directional derivative at the point (2, 3, 1) in the direction of a vector making an angle of θ with the vector ⟨2, 3, 1⟩.
First, we need to calculate the gradient of f. Taking the partial derivatives with respect to x, y, and z, we have ∇f = ⟨∂f/∂x, ∂f/∂y, ∂f/∂z⟩ = ⟨y, x, 4z^3⟩.
Next, we normalize the direction vector v to have unit length by dividing it by its magnitude. Let's assume the magnitude of v is denoted as ||v||.
Then, the directional derivative of f at the given point in the direction of v can be calculated as D_v(f) = ∇f(2, 3, 1) ⋅ (v / ||v||).
Without the specific values or the angle θ, we cannot provide the exact numerical result. However, using the formula mentioned above, you can compute the directional derivative by substituting the values of ∇f(2, 3, 1) and the normalized direction vector.
To learn more about function click here, brainly.com/question/30721594
#SPJ11
Related Questions
A zucchini plant in Darnell’s garden was 10 centimeters tall when it was first planted. Since then, it has grown approximately 0.5 centimeter per day.
a. Write a rule to describe the function.
b. After how many days will the zucchini plant be 18.5 centimeters tall?
A. h(d) = 0.5d + 10; 17 days
B. h(d) = 10d + 0.5; 1.1 days
C. h(d) = \(\frac{d}{0.5}\)+10; 4 days
D. h(d) = 0.5d; 37 days
Answers
Answer:
Let's start with part B. if it was originally 10 cm tall and it goes up 0.5 cm. each day, then we know that to go up one cm it needs two days. With that information we can say that 8*2 = 16. So it needs 17 days to go up 8.5 cm which would make it 18.5 cm tall.
Step-by-step explanation:
f(x) = 0.5x + 10
0.5x + 10 = 18.5
0.5x = 18.5 - 10
0.5x = 8.5
x = 8.5/0.5
x = 17 days
consider the equation

Answers
Answer: 6
Step-by-step explanation:
1. 2(4 + px) = 12x (write it down)
2. 8 + 2px = 12x (distributive property)
3. 8/2 + 2px/2 = 12x/2 (divide all by 2
4. 4 + px = 6x
5. 4 = 6x - px
Plug all in:
4 = 6x - 2x (possinle)
4 = 6x - 6x (not possible)
4 = 6x - 12x (possible)
4 = 6x - 24x (possible)
Answer: 6
because:
4 = 6x - 6x
4 = 0
PLEASE HELP IT WORTH 50 points!!!!!

Answers
-3(-2)=6
-3+-2=-5
(X-3)(x-2)
Answer:
(x-3) (x-2)
Step-by-step explanation:
\(x^{2}\) - 5x + 6
How to break down the equation and factorise it:
-3 x -2 = 6
-3 + -2 = -5
Final Answer:
(x-3) (x-2)
Let f be a function of two variables that has continuous partial derivatives and consider the points
A(8, 9),
B(10, 9),
C(8, 10),
and
D(11, 13).
The directional derivative of f at A in the direction of the vector AB is 9 and the directional derivative at A in the direction of
AC is 2. Find the directional derivative of f at A in the direction of the vector AD.
(Round your answer to two decimal places.)
Answers
Answer:
The directional derivative of f at A in the direction of \(\vec{u}\) AD is 7.
Step-by-step explanation:
Step 1:
Directional of a function f in direction of the unit vector \(\vec{u}=(a,b)\) is denoted by \(D\vec{u}f(x,y)\),
\(D\vec{u}f(x,y)=f_{x}\left ( x ,y\right ).a+f_{y}(x,y).b\).
Now the given points are
\(A(8,9),B(10,9),C(8,10) and D(11,13)\),
Step 2:
The vectors are given as
AB = (10-8, 9-9),the direction is
\(\vec{u}_{AB} = \frac{AB}{\left \| AB \right \|}=(1,0)\)
AC=(8-8,10-9), the direction is
\(\vec{u}_{AC} = \frac{AC}{\left \| AC \right \|}=(0,1)\)
AC=(11-8,13-9), the direction is
\(\vec{u}_{AD} = \frac{AD}{\left \| AD \right \|}=\left (\frac{3}{5},\frac{4}{5} \right )\)
Step 3:
The given directional derivative of f at A \(\vec{u}_{AB}\) is 9,
\(D\vec{u}_{AB}f=f_{x} \cdot 1 + f_{y}\cdot 0\\f_{x} =9\)
The given directional derivative of f at A \(\vec{u}_{AC}\) is 2,
\(D\vec{u}_{AB}f=f_{x} \cdot 0 + f_{y}\cdot 1\\f_{y} =2\)
The given directional derivative of f at A \(\vec{u}_{AD}\) is
\(D\vec{u}_{AD}f=f_{x} \cdot \frac{3}{5} + f_{y}\cdot \frac{4}{5}\)
\(D\vec{u}_{AD}f=9 \cdot \frac{3}{5} + 2\cdot \frac{4}{5}\)
\(D\vec{u}_{AD}f= \frac{27+8}{5} =7\)
The directional derivative of f at A in the direction of \(\vec{u}_{AD}\) is 7.
3. Solve 6k + 9 > k – 1.
use photo for options

Answers
Answer:
k > - 2
Step-by-step explanation:
Given
6k + 9 > k - 1 ( subtract k from both sides )
5k + 9 > - 1 ( subtract 9 from both sides )
5k > - 10 ( divide both sides by 5 )
k > - 2
Answer:
k> -2
Step-by-step explanation:
6k + 9 > k – 16k - k > - 1 - 95k > -10k > -10/5k> -2what+value+of+zαzα+would+result+in+a+80%+one-sided+confidence+interval?
Answers
We can be 80% confident that the true value of the parameter falls within the range of our estimate plus or minus 1.282 standard errors of the estimate.
The value of zα that would result in a 80% one-sided confidence interval is 1.282. A confidence interval is a range of values that represents the uncertainty of an estimate.
A confidence level is the probability that the interval actually contains the true value of the parameter.
Confidence intervals are typically expressed as a range of values with an associated level of confidence. In a one-sided confidence interval, all of the values are on one side of the estimate.
For example, if we want to construct a one-sided confidence interval for the mean of a population, we would either be interested in the upper or lower end of the range, but not both.
A one-sided confidence interval is also known as a one-tailed confidence interval.
The formula for a one-sided confidence interval is given by:
zα × (σ / √n), where zα is the z-score for the desired level of confidence, σ is the population standard deviation, and n is the sample size.
For an 80% one-sided confidence interval, the z-score is 1.282.
This means that we can be 80% confident that the true value of the parameter falls within the range of our estimate plus or minus 1.282 standard errors of the estimate.
Learn more about standard errors from the given link!
https://brainly.com/question/30404883
#SPJ11
Solve for x.
10
8
6

Answers
Answer:
18
Step-by-step explanation:
Intersecting Secants Theorem:
6(x + 6) = 8(8 + 10)
6x + 36 = 8(18)
6x + 36 = 144
6x = 144 - 36
6x = 108
x = 18
The image below shows two dilated figures with lines AB and A'B' drawn. If the larger figure was dilated using a scale factor of 3, what relationship do lines AB and A'B' have? (10 points)

Answers
Answer:
They have the same side lengths/angles except one is smaller than the other
Step-by-step explanation:
the smaller one is a "mini" of the larger one. a exact copy
The dilation is solved and the scale factor of line is A'B' = 3AB
What is Dilation?Resizing an item uses a transition called Dilation. Dilation is used to enlarge or contract the items. The result of this transformation is an image with the same shape as the original. However, there is a variation in the shape's size. Dilation transformations ensure that the shape will stay the same and that corresponding angles will be congruent
The result of dilation is that the shapes and boundaries of objects in the input image are expanded or thickened. It is often used in conjunction with other morphological operations such as erosion, opening, and closing to manipulate and enhance images.
Given data ,
Let the scale factor of the dilation be represented as k
Now , the scale factor k = 3
So , the ratio of the corresponding sides is given by
A'B' / AB = 3
Multiply by AB on both sides , we get
A'B' = 3AB
Hence , the dilation is simplified as A'B' = 3AB
To learn more about dilation click :
https://brainly.com/question/13176891
#SPJ2
help me asp please with this answer

Answers
Option B) If the sum of the squares of the two short sides equals the square of the longest side
It is because if we apply Pythagoras Theorem which is\( {hypotenuous}^{2} = {base}^{2} + {perpendicuar}^{2} \)If the left side (hyp^2) is equal to the right side (base^2 + per^2) then the triangle is right angled triangle.Hypotenuse is the longest side And base and height are the other two sidesHelp with this please

Answers
Answer:
C) 0 <\ x OR x />7
Step-by-step explanation:
The first arrow has a closed circle which indicates greater/less than or equal to. All the values shaded are to the left of 0. So, x is less than or equal to 0. The second arrow also has a closed circle. Since the shaded part of the arrow is to the right of 7, x is greater than or equal to 7.
Maya is going to watch a movie in her collection. She has 3 action movies, 11 comedies, and 9 dramas. She will randomly select one movie. What is the probability that the movie she selects is not a comedy?
Answers
Answer:
The probability is 12/23
Step-by-step explanation:
We calculate the number of movies in her selection
Mathematically, that will be;
3 + 11 + 9 = 23
The number of movies that are not comedy will be;
3 + 9 = 12
So the probability of selecting a movie that is not comedy will be 12/23
Use integers that are that are closest to the number in the middle. < - v119

Answers
Answer:
-11 and -10
Step-by-step explanation:
-11² = -121
-(√119)² = -119
-10² = -100
_____
-√119 is between -11 and -10
the sum of the digits in a three digit mumber is 9. the tens digit is half the sum of the other two amd the hundreds digit is half the unit digit. fond the number.
Answers
The number is 234.
Step-by-step explanation:
Let the 3 digits be H , T and U so the number is HTU.
H + T + U = 9
T = 0.5H + 0.5U
U = 2H
So from the last 2 equations
T = 0.5H + 0.5(2H)
T = 0.5H + H
T = 1.5H
So substituting for T and H is the first equation:
H + 1.5H + 2H = 9
4.5H = 9
H = 2.
So T = 1.5*H = 1.5 *2 = 3.
T/F regardless of whether a distribution of scores that is symmetrically shaped has one mode or two modes, the mean value tends to be similar to the median value.
Answers
True
In a symmetrically shaped distribution of scores, regardless of whether it has one mode or two modes, the mean value tends to be similar to the median value.
When a distribution is symmetric, it means that the data is evenly distributed around the central point, resulting in a bell-shaped curve. In such cases, the mean, median, and mode are typically close to each other.
The mean is the arithmetic average of all the scores in a distribution, while the median represents the middle value when the scores are arranged in ascending or descending order. In a symmetric distribution, the mean and median are located at the exact center of the distribution.
If the distribution has only one mode, it means that there is one prominent peak or high point in the data. In this case, the mean and median will coincide with the mode and will be similar.
If the distribution has two modes, it means that there are two prominent peaks in the data, but they are symmetrical around the center. Even though there are multiple modes, the mean and median will still be similar and tend to be located between the two modes.
In summary, in symmetrically shaped distributions, regardless of the presence of one or two modes, the mean and median values are expected to be close to each other.
Learn more about Distribution
brainly.com/question/29664127
#SPJ11
Helppp, 60 points!!!
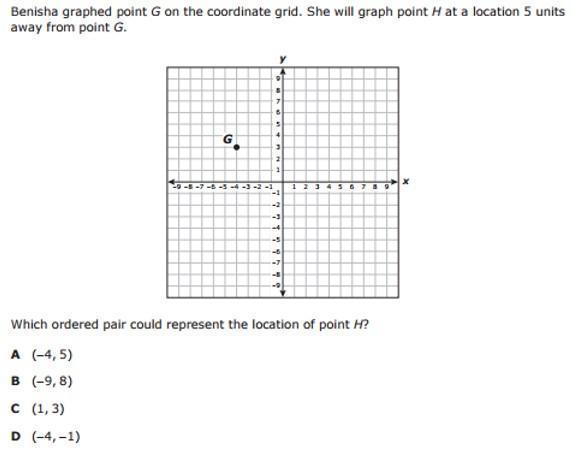
Answers
Answer:
the answer is c
Step-by-step explanation:
please mark this answer as brainliest
Answer:
The answer is c
Step-by-step explanation:
By moving 5 units to the right you lend at x-1 y-3
I need help with the first question pls

Answers
Answer:
67.5 feet
Step-by-step explanation:
If 1 inch = 9 feet, and the model is 7.5 inches,
you just need to do 7.5 • 9 = 67.5 feet.
OR
7 • 9 = 63 and 1/2 (or .5) • 9 = 4.5
63 + 4.5 = 67.5
*I'm pretty sure it says 1 inch = 9 feet and I'm also basing this off the fact that your work for question 2 is showing the same ratio of 1 inch = 9 feet
Given f(x) = 1/3(4 - x)2,what is the value of
f(16)?
Answers
Answer:
f(16)= -8
Step-by-step explanation:
f(16)= 1/3(4-16)2
Answer:
-8
Step-by-step explanation:
To answer this question, you must plug in the given 16 to the equation
f(16)= 1/3(4-16)2
f(16)= 1/3(-12)2
f(16)= 1/3(-24)
f(16)= -8
A trapezoid has bases of lengths 14 and 21. Find the trapezoid's height if it's area is 245
Answers
Answer: 8575/2
Step-by-step explanation:
Increase 100 by 85%
Answers
Answer:
100, percentage increased by 85% (percent) of its value = 185 Jan 16 02:56 UTC (GMT
Answer:
=100+85%
=100.85.......
Simplify this expression.
2(10) + 2(x – 4)
O 2x + 16
O x + 12
0 2x + 12
O
*226
Answers
Given:
The expression is
\(2(10)+2(x-4)\)
To find:
The simplified form of the given expression.
Solution:
We have,
\(2(10)+2(x-4)\)
Using distributive property, we get
\(=20+2(x)+2(-4)\)
\(=20+2x+(-8)\)
\(=20+2x-8\)
On combining like terms, we get
\(=2x+(20-8)\)
\(=2x+16\)
Therefore, the correct option is A.
If I decide to conduct a research to look for associations among variable, which of the following am I likely to find?
No associations
Some association
Either no association or some association
None of the above.
Answers
If you decide to conduct research to look for associations among variables, you are likely to find either no association or some association.
When conducting research to explore associations among variables, the outcome can vary. You may encounter situations where there is no significant association between the variables being studied. This means that the variables are independent of each other, and their values do not vary systematically or predictably in relation to one another.
On the other hand, you may also discover that there is some association between the variables. This indicates that there is a relationship or connection between the variables, and changes in one variable are related to changes in another variable.
It is important to note that the strength and nature of the associations can vary. Associations can be strong or weak, positive or negative, linear or nonlinear, depending on the specific research question and the variables under investigation.
When conducting research to explore associations among variables, it is likely that you will find either no association or some association. The specific outcome will depend on the nature of the variables and the analysis conducted. It is essential to interpret the results carefully and consider the context and limitations of the study when drawing conclusions about the associations observed.
Learn more about association ,visit:
https://brainly.com/question/30329420
#SPJ11
Q.4 What is the difference between price floors and price ceiling? Give example and illustrate graphically in support of your answer.
Answers
A price floor is a law that limits the minimum price at which a good, service, or factor of production can be sold while a price ceiling is a regulation that limits the maximum price at which a good, service, or factor of production can be sold
Price floors are commonly implemented to support producers, while price ceilings are typically put in place to protect consumers from higher prices that might result from shortages or monopolies.
Example of Price Floor:Agricultural subsidies are a common example of price floors. Government price floors ensure that farmers receive a minimum price for their crops.
If the market price of wheat falls below the government-established price floor, the government may buy the excess supply at the guaranteed price, ensuring that farmers are able to make a profit. If there is a price floor, the minimum price is set above the equilibrium price.
Learn more about price ceiling at:
https://brainly.com/question/2562066
#SPJ11
A truck rental company charges a $25 rental fee when someone rents.a
truck in addition to an hourly rental rate of $15 per hour. What is the
equation of the line that represents this truck rental company's rental cost?
Let x be the number of hours and let y be the rental cost.
Answers
Answer:
25 +15x
Step-by-step explanation:
so the fee to rent a truck is 25 but for every hour that you have it, you have to pay $15, so x represents the number of hours you have the truck, so if you have the truck for 3 hours, you would say 25+15(3)= $70
Answer:375
Step-by-step explanation:
8 1/4 divided by 3/8
Answers
Answer:
11over32
Step-by-step explanation:
A ⊆ B
S (B\A)=28, S(B)=5.S(A). S(A)=?
Answers
Answer:
S(A) = 7
Step-by-step explanation:
A ⊆B means A is a proper subset of B. That means all elements of set A are also elements of set B
S(B\A) means the set of all elements of B that are not in set A. This is given as 28
We are also given S(B) = 5.S(A)
Since A is a subset of B, S(B) = set of all elements in A and set of all elements in B but not in A
In other words
S(B) = S(A) + S(B\A)
Since S(B) = 5S(A) we get
5S(A) = S(A) + S(B\A)
5S(A) = S(A) + 28
5S(A) - S(A) = 28
4S(A) = 28
S(A) = 7
show work / explain it

Answers
Answer:inequality form x ≤ -1
for 3x + 9 ≤ 6
Step-by-step explanation:isolate the variable by dividing eash side by factors that dont contain the variable
Answer:
Answer Varies
Step-by-step explanation:
For the 1st equation, there are a lot of numbers that could fit x, such as any negative number, such as -1,-2,-3, etc. For the 2nd equation, 9 and below would work. Please correct me if I am wrong because everybody makes mistakes.
ten students wrote a test, and the distribution of scores is shown on the frequency table. if the average (arithmetic mean) score is 62, what is the value of x ?
Answers
From the given information, the value of x in the given table is 42.5.
To find the value of x, we can use the formula for the arithmetic mean (average) of a set of numbers:
Arithmetic mean = (sum of all numbers) / (number of numbers)
Given that the average score on the test is 62, we can use this information to find the sum of all the scores.
Given that number of students is 1,2,3 and 4 with scores of 40,55,70 respectively, we can use this information to find the sum of all the scores.
Sum of all scores = (1 * 40) + (2 * 55) + (3 * 70) + (4 * x)
Now we can substitute the given average score and the number of students into the equation and solve for x.
62 = (1 * 40) + (2 * 55) + (3 * 70) + (4 * x) / 10
620 = 40 + 110 + 210 + 4x
4x = 170
x = 42.5
So, the value of x is 42.5
Read more about Arithmetic mean:
brainly.com/question/20118982
#SPJ4
The complete question is -
ten students wrote a test, and the distribution of scores is shown in the frequency table. if the average (arithmetic mean) score is 62, what is the value of x?
Score - 40, 55, 70, x
Number of students - 1, 2, 3, 4
Cheyenne interpreted the following function: f(x) =x+4 as the first step before graphing it. She wrote her answers in the table. Find and fix her error
Answers
110 4t + 90 t=?
Please help!

Answers
Answer:
because of some theorm or postulate i forget the name of, angles on opposite sides are equal
110 = 4t + 90
20 = 4t
5 = t
t is equal to 5 degrees
ANSWER FAST BECUASE I NEED THE ANSWER FASTTTTTTTTTTTTT

Answers
Answer: 112°
Step-by-step explanation:
The angles are supplementary so add to 180°
(2x) + (3x + 10) = 180
Combine Like Terms leaves:
5x + 10 = 180
Subtract 10 from each side:
5x = 170
Divide 5 on each side:
x = 34
Plug x into the large angle
3(34) + 10
Solve:
102 + 10 = 112
The large angle is 112°
Hope this helps!