4) A firm determine demand function and total cost function: p =
550 − 0.03x and C(x) = 4x + 100, 000, where x is number of units
manufactured and sold. Find production level that maximize
profit.

The simplified form of the expression 2x^2 - 3x - 2 remains as 2x^2 - 3x - 2.

To simplify the expression 8x - 2x - x^2, we can combine like terms by adding or subtracting coefficients.

8x - 2x - x^2

First, let's combine the x terms:

(8x - 2x) - x^2

This simplifies to:

6x - x^2

Therefore, the simplified form of the expression 8x - 2x - x^2 is 6x - x^2.

Now, let's simplify the expression 2x^2 - 3x - 2:

The expression is already in simplified form, and no further simplification is possible.

Therefore, the simplified form of the expression 2x^2 - 3x - 2 remains as 2x^2 - 3x - 2.

​for such more question on simplified form

https://brainly.com/question/11680269

#SPJ8

Answer:b

Step-by-step explanation:

Answer:

13\(\frac{11}{13}\)

Step-by-step explanation:

As a square has four identical sides:

Step 1 - Multiply the length of one side by four:

3 x 4 = 12

\(\frac{6}{13}\) x 4 = \(\frac{24}{13}\) or 1 \(\frac{11}{13}\)

1\(\frac{11}{13}\) + 12 = 13\(\frac{11}{13}\)

Hope this helps!

Answer:

y-intercept = (0, 6)

x-intercept = (-4, 0)

Step-by-step explanation:

The y-intercept is the point on the graph where it crosses the y-axis, and has coordinates (0, b). It is also the value of y when x = 0.

To find the y-intercept, set x = 0:

4y - 6x = 24

4y - 6(0) = 24

4y = 24

4y/4 = 24/4

y = 6

Therefore, the y-intercept is (0, 6).

The x-intercept is the point the graph where it crosses the x-axis, and has coordinates (a, 0). It is also the value of x when y = 0.

To find the x-intercept, set y = 0:

4(0) - 6x = 24

0 - 6x = 24

-6x/-6 = 24/-6

x = -4

Therefore, the x-intercept is (-4, 0).

Given:-

The line passes through (-7,9).

To find:-

Equation for a line that parallel to y-axis and passes through the point (-7,9).

The images of line which is parallel to y-axis is,

So if the line is parallel towards the y-axis then, the value of x-axis is equal.

That is,

And the value of k here is the x-axis value of the point the line passes through.

So,

So the equation of the line which is parallel to y-axis and passes through the point (-7,9) is,

So the required equation is x+7=0.

The value of lim f(x) using the table values is lim f(x) = 0

To find the limit of f(x) as x approaches 1, we need to compute the values of f(x) for the given x-values in the table and then use those values to determine the limit.

First, let's complete the table by plugging in the given x-values into the function f(x) = (x-1)^2:

|x       |(x-1)^2  |
|--------|---------|
|1.1     |0.01     |
|1.01    |0.0001   |
|1.001   |0.000001 |
|1.0001  |0.00000001|
|0.9     |0.01     |
|0.99    |0.0001   |
|0.999   |0.000001 |
|0.9999  |0.00000001|

Now, we can use these table values to determine the limit of f(x) as x approaches 1. As we can see from the table, as x gets closer and closer to 1 from both sides, the value of f(x) gets closer and closer to 0. Therefore, we can conclude that the limit of f(x) as x approaches 1 is 0:

lim (x-1)^2 = 0

So, the final answer is:

lim f(x) = 0

To know more about limits refer here:

https://brainly.com/question/30118799#

#SPJ11

Answer:

6 5/6

Step-by-step explanation:

diameter is radius multiplied by 2

* means multiplied by

3 5/12 = 41/12

41/12 * 2 = 82/12 = 6 5/6

Answer: 6 5/12

Step-by-step explanation: i just took the k12 test and this is the corret answer

Answer:

c = 20d + 15

Step-by-step explanation:

answer choices may help if the answer is not one of the choices; but here is the answer that it should be:

20 dollars per day is 20 times the length of rental.

15 is a cost that is added and does not change.

c = the total cost therefore:

c = 20d + 15

=======================================================

Explanation:

The table headers will look like choice A or choice C. Notice that we have simple yes or no questions along either the row or column labels; also, the labels are consistent. Choice B can be ruled out because the labels aren't consistent (or don't match up). Choice D is overcomplicated so it can be ruled out also.

We're told that 35 students play an instrument. That means 35 goes at the end of the "plays instrument" row, in the "total" column.

This is enough to see that choice A is the final answer

Furthermore, 30 students are in a band. So we have "30" at the bottom of the "band" column, and in the "total" row. The same applies to the 30 people not in band.

Interactions involving dummy variables can provide insights into the different effects of independent variables across categories.

1. Dummy variables are binary variables that represent categorical variables in a regression analysis. When interactions are included between dummy variables and other independent variables, it allows for differential effects of the independent variables based on the different levels of the categorical variable.

For example, let's consider a regression model to predict income based on education level and gender. We can include an interaction term between education level (represented by dummy variables for different levels) and gender. This interaction term allows us to examine whether the effect of education level on income differs between males and females. It helps capture any gender-specific differences in the relationship between education and income.

1.2 The linear probability model (LPM) is a common approach to estimate the probability of an event occurring using a linear regression framework. However, it has several problems:

1. The predicted probabilities from the LPM can fall outside the [0, 1] range: Since the LPM does not impose any restrictions on the predicted probabilities, they can sometimes exceed the valid probability range. This violates the assumption of probabilities being bounded between 0 and 1.

2. Heteroscedasticity: The LPM assumes constant error variance across the range of the predictors. However, in practice, the variability of the error term may change with different levels of the predictors, resulting in heteroscedasticity. This violates the assumption of homoscedasticity.

3. Non-linearity: The LPM assumes a linear relationship between the predictors and the probability of the event. However, this may not always be the case, and using a linear model can result in misspecification.

To remedy these problems, an alternative to the LPM is to use logistic regression or probit regression models. These models explicitly model the probability of an event occurring and address the issues mentioned above. They provide predicted probabilities that fall within the valid range of 0 to 1, account for heteroscedasticity, and allow for non-linear relationships between the predictors and the probability of the event.

Learn more about LPM here:

https://brainly.com/question/30890632

#SPJ4

In the given Discrete Mathematics class, at least four students were born in the same month.

a) Pascal's Identity:Let us consider a set with n + 1 elements. We need to choose k elements from these. We can choose a subset of k elements in two ways:

(i) Choose one of the n elements as the first element of the subset, then choose k − 1 elements from the remaining n − 1 elements. This can be done in nC1 * (n − 1)C(k − 1) ways.

(ii) The subset does not include the first element. This can be done in nCk ways. Now, we count the number of ways of choosing a subset with k elements from the set with n + 1 elements. This can be done in (n + 1)Ck ways. By the addition principle of counting, we add these two cases and get the identity (n + 1)Ck = nC(k−1) + nCk.b)

The Generalized Pigeonhole Principle:

Let N be the number of objects and k be the number of boxes. Let q be the quotient when N is divided by k. If N is divisible by k, then each box will contain exactly q objects. Otherwise, there will be (N mod k) boxes containing q + 1 objects and the remaining k − (N mod k) boxes containing q objects. At least one of the former boxes will contain at least ⌈N/k⌉ objects.

b') Among 45 students in our Discrete Math at least how many were born in the same month?Let us consider 12 boxes, one for each month. Since there are 45 students, by the Generalized Pigeonhole Principle, at least one box must have ⌈45/12⌉ = 4 students. Therefore, at least four students in our Discrete Math course were born in the same month.

Learn more about: Discrete Mathematics

https://brainly.com/question/30409326

#SPJ11

Answer:

Exact form = - 17/8

Decimal form = -2.125

Mixed number form = -2 1/8

Step-by-step explanation:

Convert the mixed numbers to improper fractions, then find the LCD and combine.

Therefore, the average of the squared distance between the origin and points in the solid cylinder D is 1/2.

The average of the squared distance between the origin and points in the solid cylinder D, we need to integrate the squared distance over the volume of the cylinder and then divide by the volume. The squared distance between the origin and a point (x, y, z) is given by:

d² = x² + y² + z²

The volume of the cylinder is given by:

V = πr²h = π(5²)(2) = 50π

The integral of the squared distance over the volume of the cylinder is:

∭d² dV = ∫₀²π ∫₀⁵ ∫₀² (x² + y² + z²) dz dx dy

Integral by integrating with respect to z first:

∫₀² (x² + y² + z²) dz = x² + y² + 2z³/3 evaluated from z = 0 to z = 2

= x² + y² + (16/3)

Expression back into the integral and integrating with respect to x and y gives:

∭d² dV = ∫₀²π ∫₀⁵ (x² + y² + (16/3)) dx dy

= ∫₀²π [(x³/3) + xy² + (16/3)x] evaluated from x = 0 to x = 5 dy

= ∫₀²π [(125/3) + 5y² + (80/3)] dy

= [(125/3)y + (5/3)y³ + (80/3)y] evaluated from y = 0 to y = √(25-x²)

= [(125/3)√(25-x²) + (5/3)(25-x²)√(25-x²) + (80/3)√(25-x²)] evaluated from x = 0 to x = 5

∭d² dV = 25π

Dividing by the volume of the cylinder gives the average of the squared distance:

(1/V) ∭d² dV = (1/50π) (25π) = 1/2

Learn more about distance visit: brainly.com/question/26550516

#SPJ4

Correct Question:

Find the average of the squared distance between the origin and points in the solid cylinder D = {(x,y,z): x² + y² ≤ 25, 0 ≤ z ≤ 2}. The average of the squared distance is (Simplify your answer. Type an integer or a fraction. )

Therefore, there are 2,436,686,400 ways of combination to divide the teams into 32 pairs.

To calculate the number of ways to do this, you can use the concept of combinations.
In this case, you can use the formula:
C(n, k) = n! / (k!(n-k)!)
Where n is the total number of teams (64) and k is the number of teams in each pair (2).
First, we need to divide the teams into pairs, so there will be 32 pairs in total. To find the number of ways to do this, we can use the combinations formula:
C(64, 2) = 64! / (2!(64-2)!)
C(64, 2) = 64! / (2! * 62!)
Next, we need to multiply this by the number of ways to create the remaining 31 pairs:
=C(62, 2) * C(60, 2) * ... * C(4, 2)
So, the total number of ways to divide the 64 teams into 32 pairs is:
=C(64, 2) * C(62, 2) * ... * C(4, 2) / (32!)
This will give you the number of ways to divide the teams into 32 pairs for the tournament's first round.

(64 x 63 x 62 x ... x 2 x 1) / (2 x 2 x 2 x ... x 2 x 2) = 64! / (2³²)

To know more about combination,

https://brainly.com/question/16199890

#SPJ11

The required number of pallets utilized to build one step of the mansion exists 2 of a pallet of pavers.

Let the equation be (2/3) ÷ (1/3)

simplifying the equation, we get

= (2 × 3)/(1 × 3) = 2

Therefore, the required number of pallets utilized to build one step of the mansion exists 2 of a pallet of pavers.

To learn more about fractions refer to;

https://brainly.com/question/78672

#SPJ4

The length of the given curve between t=0 and t=1 is approximately 13.573 units.

Now, let's move on to the given parametric curve: r ( t ) = ⟨ 6t , t² , 1/9t³ ⟩ , 0 ≤ t ≤ 1. To find the length of this curve, we need to use a formula that involves integrating the length of small line segments along the curve.

The formula we'll use is the arc length formula:

\(L = \int _a^b \sqrt{(dx/dt)^2 + (dy/dt)^2 + (dz/dt)^2 dt}\)

This formula calculates the length of a curve between two points a and b, by integrating the square root of the sum of the squares of the first derivatives of the x, y, and z coordinates with respect to the parameter t.

Using this formula, we can find the length of the given curve as follows:

\(L = \int _0^1 \sqrt{(6)^2 + (2t)^2 + (2/27t^4)^2 dt}\)

\(L = \int _0^1 \sqrt{(36 + 4t^2 + 4/729t^8) dt}\)

\(L = \int_0^1 \sqrt{(2916t^8 + 11664t^6 + 15552t^4 + 6912t^2 + 1296) / 729 dt}\)

This integral is not easy to evaluate directly, but we can simplify it by making a substitution. Let u = 6t² + 6/27t⁶. Then du/dt = 12t + 4/9t⁵, and we can write the integrand as:

\(= > \sqrt{(2916t^8 + 11664t^6 + 15552t^4 + 6912t^2 + 1296) / 729} \\ \\= \sqrt{(u^4 + 3u^2 + 1) / 18t^3}\)

Now we can express the integral in terms of u:

\(L = (1/9) \int _0^6 \sqrt{(u^4 + 3u^2 + 1) / u du}\)

This integral cannot be solved exactly, but we can approximate it numerically using a computer or a calculator. The result is approximately 13.573 units of length.

To know more about curve here

https://brainly.com/question/31376454

#SPJ4

Complete Question:

Find the length of the curve. r ( t ) = ⟨ 6t , t², 1/9t³ ⟩ , 0 ≤ t ≤ 1

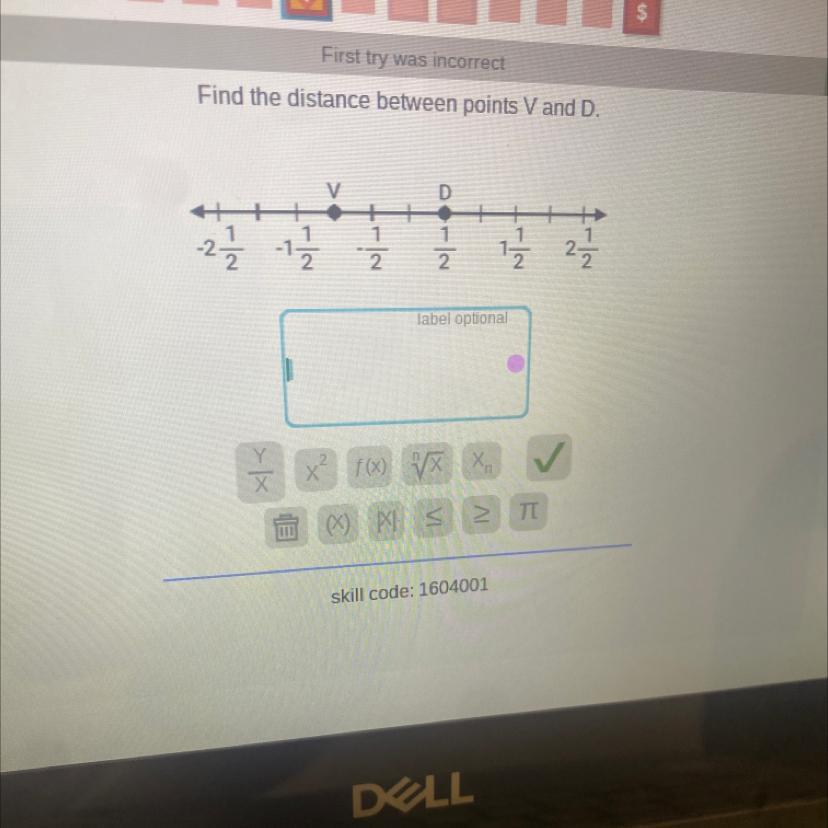
Answer:

Step-by-step explanation:

point v = -1

point d= 1/2 or 0.5

distance is just delta |x1 -x2|.

So, v - d  is

-1 -0.5 => -1.5 => 1.5

Given:

Suppose point D is in the interior of ∠ABC , m∠ABC=12x−110 , m∠ABD=3x+40 , and m∠DBC=2x−10.

To find:

The measure of ∠ABC.

Solution:

Since point D is in the interior of ∠ABC, therefore

\(m\angle ABC=m\angle ABD+m\angle DBC\)

Substitute the given values, we get

\(12x-110=(3x+40)+(2x-10)\)

\(12x-110=5x+30\)

\(12x-5x=110+30\)

\(7x=140\)

Divide both sides by 7.

\(x=20\)

The value of x is 20.

\(m\angle ABC=12x-110\)

\(m\angle ABC=12(20)-110\)

\(m\angle ABC=240-110\)

\(m\angle ABC=130^\circ\)

Therefore, the measure of ∠ABC is 130 degrees.

\(\\ \sf\longmapsto y=\dfrac{2}{7}x+\dfrac{1}{3}\)

Compare to slope intercept form of a line

\(\\ \sf\longmapsto y=mx+b\)

Answer:

2:3

Step-by-step explanation:

Answer:

2-3

Step-by-step explanation:

Answer:

B

Step-by-step explanation:

19/8 ÷ 5/4 = 19/8 ⋅ 4/5

19/8 ⋅ 4/5 = 19/10

19/10 = 1 9/10

Answer:

c

Step-by-step explanation:

Answer:

locate the owner and make a request

Answer:  B

Step-by-step explanation: hope this helps :) <3

O-O

Answer:

3*57=171

Step-by-step explanation:

Answer:

171

Step-by-step explanation:

Well, 3 x 50 is 150 and 3 x 7 is 21 and 150 + 21 = 171

To implement the function ar{x_0}ar{x_1} + x_0x_1 using an 8:1 multiplexer (mux) with inputs x_2 - x_0 and connections to power and ground, you would connect x_0 to select2, x_1 to select1, and x_2 to select0. Connect power to the select input, and ground to the remaining select inputs.

In a multiplexer, the select inputs determine which input is routed to the output. In this case, we want to implement the function ar{x_0}ar{x_1} + x_0x_1. The select inputs of the mux need to be set such that the desired function is achieved.

To connect the inputs of the mux, we start by connecting x_0, the least significant bit (LSB) of the function, to the select input select2. This means that when select2 is low (0), x_0 will be selected as the output. Next, we connect x_1, the middle bit of the function, to the select input select1. When select1 is low (0), x_1 will be selected as the output.

Finally, we connect x_2, the most significant bit (MSB) of the function, to the select input select0. When select0 is low (0), x_2 will be selected as the output. This configuration ensures that the function ar{x_0}ar{x_1} + x_0x_1 is implemented correctly.

Additionally, it's important to connect power to the select input to ensure proper functioning of the multiplexer. The select inputs need a valid voltage level to work correctly, and connecting them to a power source (usually labeled VCC) ensures this. Ground, which is typically labeled GND, should be connected to the remaining select inputs to complete the circuit and provide a reference voltage level.

Learn more about function here: https://brainly.com/question/28278690

#SPJ11

There are 8,416,416 different license plates that can be generated using this format.

To determine the number of license plates that can be generated using this format, we need to calculate the total number of possible combinations.

Since each license plate has four letters and two digits, we can consider them separately.

For the letters, we have 22 options (26 letters minus the excluded letters i, o, q, and u). Since repetition is allowed, there are 22 choices for each of the four positions. Therefore, the total number of letter combinations is 22^4 = 234,256.

For the digits, we have 6 options (10 digits minus the excluded digits 0, 1, 2, and 3). Again, repetition is allowed, so there are 6 choices for each of the two positions. The total number of digit combinations is 6^2 = 36.

To find the total number of license plates, we multiply the number of letter combinations by the number of digit combinations: 234,256 * 36 = 8,416,416.

Therefore, there are 8,416,416 different license plates that can be generated using this format.

To know more about number click here

brainly.com/question/28210925

#SPJ11

One statement that is necessarily true when a quadrilateral is inscribed in a circle is that the opposite angles of the quadrilateral are supplementary. In other words, the sum of the measures of two opposite angles is always 180 degrees.

To understand why this is true, let's consider a specific example. Imagine a quadrilateral PQRS inscribed in circle A. Let's say angle PQR measures 80 degrees. Since angle PQR is an inscribed angle that intercepts arc PS, it is equal in measure to half the measure of arc PS. Therefore, arc PS measures 160 degrees.

Now, let's focus on angle PSR. Angle PSR also intercepts arc PS, so it has the same measure as half the measure of arc PS, which is 160 degrees. Therefore, angle PSR measures 160 degrees as well.

If we add the measures of angles PQR and PSR, we get 80 + 160 = 240 degrees. This violates the fact that the sum of the measures of angles in a quadrilateral is always 360 degrees. Thus, it is clear that angles PQR and PSR cannot both measure 80 degrees.

Based on this example, we can conclude that the opposite angles of a quadrilateral inscribed in a circle are supplementary. This means that their sum is always 180 degrees.

To Know more about  quadrilateral Visit:

https://brainly.com/question/31266965

#SPJ11

In a multi-programming environment where all the jobs are executed at once in a batch, the completion time of the system will be determined by the job that takes the longest time to complete.

In this case, Job 2 and Job 3 both take 10 seconds to complete, which is the longest among all the jobs.

Therefore, the completion time of the system in a multi-programming environment is 10 seconds.

To calculate the throughput, we need to determine the number of jobs completed per unit of time. In this case, we have four jobs completed in 10 seconds.

Throughput = (Number of jobs completed) / (Time taken)

Throughput = 4 jobs / 10 seconds

Throughput = 0.4 jobs/second

To convert the throughput to jobs per minute, we can multiply it by 60:

Throughput = 0.4 jobs/second * 60 seconds/minute

Throughput = 24 jobs/minute

Therefore, the throughput of the system in a multi-programming environment is 24 jobs per minute.

The mean response time is the average time taken for a job to complete from the moment it starts executing.

To calculate the mean response time, we sum up the individual job completion times and divide it by the number of jobs.

Mean response time = (Job 1 completion time + Job 2 completion time + Job 3 completion time + Job 4 completion time) / (Number of jobs)

Mean response time = (3 seconds + 10 seconds + 10 seconds + 7 seconds) / 4

Mean response time = 30 seconds / 4

Mean response time = 7.5 seconds

Therefore, the mean response time of the system in a multi-programming environment is 7.5 seconds.

Learn more about Mean here:

https://brainly.com/question/31101410

#SPJ11

Answer:

111%

Step-by-step explanation:

£3 × 60 = £180

(35 × £8) + ( 25 × £4) = £380

£380 ‐ £180 = £200

200/180 × 100% = 111% (nearest whole number)

The midpoint, that is the middle point of any given line segment can be calculated if the endpoints have been made available.

The endpoints for the line segment is,

(7, 5) and (-3, -5) for points X and Y respectively.

The middle point can be found at this position;

(x1 + x2) / 2 and (y1 + y2) / 2

The points are shown as

(7, 5) = (x1, y1)

(-3, -5) = x2, y2)

Therefore we now have,

Midpoint = (7 + [-3])/2 and (5 + [-5])/2

Midpoint = (7-3)/2 and (0)/2

Midpoint = 4/2 and 0/2

Midpoint = (2, 0)