19. For the following exercises, use the descriptions of each pair of lines given below to find the slopes of Line 1 and Line 2. Is each pair of lines parallel, perpendicular, or neither?
19. Line 1: Passes through (-8, -55) and (10, 89)
Line 2: Passes through (9, -44) and (4, -14)
Answers
Answer:
The given lines are un-related.
Step-by-step explanation:
In the question, two lines are given as line 1 passes through (-8,-55) and (10,89). Whereas, line 2 passes through (9,-44) and (4,-14).
It is required to find the slope of given lines and figure out whether they are perpendicular, parallel or neither.
To solve this question, first find the slope of both lines. Check if their product is equal to -1, then they are perpendicular. If the slopes are equal the lines are parallel.
Step 1 of 2
Find the slope of first line.
\($$\begin{aligned}&m_{1}=\frac{89-(-55)}{10-(-8)} \\&m_{1}=\frac{144}{18} \\&m_{1}=8\end{aligned}$$\)
Step 2 of 2
Find the slope of first line.
\($$\begin{aligned}&m_{2}=\frac{-14-(-44)}{4-9} \\&m_{2}=\frac{30}{-5} \\&m_{2}=-6\end{aligned}$$\)
Since, both slopes are neither equal nor reciprocal of each other.
Therefore, the lines are un-related.
Related Questions
what is the answer to this?

Answers
\(3x^3 + x^2 = 6x\), which is option B, is the right response as Option C,\(3x^3 + x^2 = 0\), does not equal the supplied expression.
what is polynomial ?Using just the methods of addition, elimination, and multiplication as well as ou pas integer exponents, a polynomial is a mathematical equation made up of variables and coefficients. A polynomial, for instance, is 3x2 - 2x + 5, where x has been the component and 3, -2, and 5 are now the coefficients. This polynomial's highest exponent is 2, which also happens to be its degree. Polynomials can have coefficients that are either constants or other polynomials, as well as many variables. Due to their usage in representing a variety of mathematical relations and functions, polynomials play a significant role in mathematics. Several disciplines, including algebra, algebra, geometry, and physics, make extensive use of them.
given
To make \(5x^2-x-8x^2 + 3x^3\)simpler, we can rearrange the terms and join comparable terms:
\(0 + 3x^3 - x - 3x^2 = 5x^2 - 8x^2 + 3x^3 - x\)
The following step is to factor the final three terms to remove a common factor of x:
\(3x^3 - x - 3x^2 = x\\(3x^2 - 1 - 3x)\)
Hence, the abbreviated form is:
\(3x^2 - 1 - 3x^3 = 5x^2-x-8x^2 + 3x^3.\)
Due to the fact that Option C,\(3x^3 + x^2 = 0\), does not equal the supplied expression, it is incorrect.
Due to their failure to simplify to the specified formula, options A, \(-5x^3+ x^2 = 0,\) and D, \(3x^3 -3x^2 =6x\), are likewise incorrect.
\(3x^3 + x^2 = 6x\), which is option B, is the right response.
To know more about polynomials visit:
https://brainly.com/question/11536910
#SPJ1
The complete question is:-
Simplify: \(5x^2-x-8x^2 + 3x^3 = 5x\)
OA.-5x3x2 = 0
B. 3x3 + x2 = 6x
C. 3x3 + x2 = 0
D. 3x3 3x26x
-
Reset Selection
The Cowboys win another game! Let's goooo!!!
Answers
Answer:
cowboys fan:0 im guessing lol
Step-by-step explanation:
cowboys aren't bad so i'm not complaining:)
solve for x round your answer to the nearest tenth
help me PLSSS

Answers
Answer:
42×15=x.....................
Step-by-step explanation:
:)
Denine sees a 2.8-kilo block of cheese.
If there are 2.2 pounds in 1 kilogram (kilo), what is the weight of the block of cheese in pounds?
Answers
Answer:
1.27
Step-by-step explanation:
S part of a statistic project, a math class weighed all the children who were willing to participate as shown below.
How many children weighed less than 50 pounds?

Answers
Answer:
18 children weighed less than 50 pounds.
Step-by-step explanation:
Ok, adding into the explanation what the heck this kind of a table is. The "stem" column is the tens place of each of the children's weights. The "leaf" is the ones digit of each of the children's weights. So in the row where the stem is 2, there were 2 kids weighing 23 and 25 pounds.
For your question, we have to add up how many students have a weight with a "stem" less than 5, because that's where 50 pounds starts.
8 + 2 + 5 + 3 = 18 children weighed less than 50 pounds.
Problem 3. A shoe store sells a new type of basketball shoe. The table shows the pairs sold s over time f
(in weeks).
Week (t)
Pairs sold (s)
1
5
32
3.1 Write a function that models the pairs sold s over time t.
Model:
5
48
3.2 Estimate the number of pairs of shoes sold after 6 weeks.
ANSWER:
7
58
65
Answers
3.1 The function that models the pairs sold (s) over time (t) is:
\(s(t) = -3t^2 + 6t + 2.\)
3.2 The estimated number of pairs of shoes sold after 6 weeks is -70.
3.1 To write a function that models the pairs sold (s) over time (t), we can use the given data points to find the pattern or relationship between the weeks (t) and the pairs sold (s).
From the table:
Week (t) Pairs sold (s)
1 5
3 2
5 48
By observing the data, we can see that the pairs sold (s) increases by a certain amount after each week. Let's calculate the difference between consecutive pairs sold:
Difference between pairs sold at week 3 and week 1: 2 - 5 = -3
Difference between pairs sold at week 5 and week 3: 48 - 2 = 46
We notice that the difference is not constant, which suggests a nonlinear relationship. To model this, we can use a quadratic function.
Let's assume the function is of the form s(t) = at^2 + bt + c, where a, b, and c are constants to be determined.
Substituting the given data point (t, s) = (1, 5) into the function, we get:
\(5 = a(1)^2 + b(1) + c\)
5 = a + b + c (Equation 1)
Substituting the data point (t, s) = (3, 2) into the function, we get:
\(2 = a(3)^2 + b(3) + c\)
2 = 9a + 3b + c (Equation 2)
Substituting the data point (t, s) = (5, 48) into the function, we get:
\(48 = a(5)^2 + b(5) + c\)
48 = 25a + 5b + c (Equation 3)
Now we have a system of three equations (Equations 1, 2, and 3) that we can solve to find the values of a, b, and c.
Solving the system of equations, we find:
a = -3
b = 6
c = 2
Therefore, the function that models the pairs sold (s) over time (t) is:
\(s(t) = -3t^2 + 6t + 2.\)
3.2 To estimate the number of pairs of shoes sold after 6 weeks, we can substitute t = 6 into the function \(s(t) = -3t^2 + 6t + 2.\)
\(s(6) = -3(6)^2 + 6(6) + 2\)
s(6) = -3(36) + 36 + 2
s(6) = -108 + 36 + 2
s(6) = -70
Therefore, the estimated number of pairs of shoes sold after 6 weeks is -70.
for such more question on number
https://brainly.com/question/15858152
#SPJ11
Colleen owns a house. In the house, on a tile floor is a throw rug and a heavy decorative urn. Most likely to meet the definition of a fixture is Group of answer choices the urn. the throw rug. the tile floor. None of them are fixtures
Answers
The item in the Colleen's house that is most likely to meet the definition of a fixture is a tile.
What is a fixture?A fixture is an item in a house that is permanently attached to a house with the use of a glue, nails or bolt. It would not be easy to remove fixtures from a house. Examples of fixtures are tiles, doors and windows.
To learn more about fixtures, please check: https://brainly.com/question/14936262
#SPJ1
Let f(x) = 3x − 1. What ordered pair in f corresponds to the equation f(x) = 5? Recall y = f(x)
Answers
The function f(x) is defined as f(x) = 3x - 1. The ordered pair (x, y) that corresponds to the equation f(x) = 5 is (2, 5), where x = 2 and y = 5.
The function f(x) is defined as f(x) = 3x - 1, and we want to find the ordered pair (x, y) that corresponds to the equation f(x) = 5.
To do this, we can substitute 5 for f(x) in the equation and solve for x.
f(x) = 3x - 1
5 = 3x - 1
Adding 1 to both sides, we get:
6 = 3x
Dividing both sides by 3, we get:
2 = x
So we have found that when x = 2, f(x) = 5.
To find the corresponding y-value, we can substitute x = 2 into the original function:
f(x) = 3x - 1
f(2) = 3(2) - 1
f(2) = 6 - 1
f(2) = 5
Therefore, the ordered pair that corresponds to the equation f(x) = 5 is (2, 5).
To know more about functions, visit:
brainly.com/question/30721594
#SPJ11
2. Finance Planners recommend no more than 30% of your income should be your rent.
1. How much should Hagatha spend on rent if she brings home $2,000 per month?
2. If the average rent for a one-bedroom in Portland is $1,743, how much does Hagatha need to earn per month to have her rent total 30% of his income?
Answers
She needs to make $5810 a month to have her 1743$ rent be 30% of her income because 30/100 = 1743/x
x= 5810
In a third of an hour Josh can run 2 miles. How far can Josh run in 5 hours?
Answers
Answer:
30 miles.Step-by-step explanation:
One-third of an hour is:
60 × ⅓ = 20 minutes.
Miles per minute:
2 ÷ 20 = 0.1 mile.
How far can Josh run in 5 hours?:
5 hours = 5 × 60 = 300 minutes.
1 minute = 0.1 mile.
300 minutes = ?
300 × 0.1 = 30 miles.
Solve for X and Y. Explain your reason (short).

Answers
The value of x = 130°
Reason: same-side-interior angles theorem
The value of y = 130°
Reason: vertical angles theorem
What is the Same-Side-Interior Angles Theorem?The two angles that are formed on the same side of the transversal that cuts across two parallel lines are supplementary, based on the same-side-interior angles theorem.
What is the Vertical Angles Theorem?If two angles are formed when two lines intersect each other and both angles are directly facing each other and share a common vertex, then, according to the vertical angles theorem, both angles are congruent to each other.
x + 50 = 180 [same-side-interior angles theorem]
x + 50 - 50 = 180 - 50
x = 130°
y° = x° [vertical angles theorem]
Substitute
y = 130°
In summary:
The value of x = 130°
Reason: same-side-interior angles theorem
The value of y = 130°
Reason: vertical angles theorem
Learn more about the same-side-interior angles theorem on:
https://brainly.com/question/16976757
#SPJ1
The scale of a room in a blueprint is 5 in.: 9 ft. A wall in the same blueprint is 30 in. Complete the table.
Blueprint length (in.) 5
10
15
20
25
30
Actual length (ft)
9
How long is the actual wall?
The actual wall is
feet long
A window in the room has an actual width of 4.5 feet. Find the width of the window in the blueprint.
The width of the window in the blueprint is
in.
Answers
Answer:
54 feets
2.5 inches
Step-by-step explanation:
Given that:
Scale model:
5 in : 9ft
If 5 in = 9 ft
Hence, 1 in on the blue print equals
5 in = 9 ft
1 in = x
Cross multiply
5x = 9
x = 9/5 feets
Hence, 1 inches on the blueprint equals 9/5 feets actual
Blueprint length (in.) ___ Actual length (ft)
5 _________ 9
10 ________ 18
15 ________ 27
20 _______ 36
25 _______ 45
30 _______ 54
Hence ;
5 * 9/5 = 9 feets
10 * 9/5 = 18 feets
15 * 9/5 = 27 feets
20 * 9/5 = 36 feets
25 * 9/5 = 45 feets
30 * 9/5 = 54 feets
Window with actual width of 4.5 feets
Width of window in blue print :
Actual width ÷ 9/5
Actual width = 4.5 = 4 1/2 = 9/2
9/2 * 5 /9
= 5 /2
= 2 1/2 inches = 2.5 inches
A rectangular area of 28,000 ft2 is to be fenced off. Two types of fencing material are being considered: Metal fencing which costs $2 per ft and Wooden fencing which costs $5 per foot. You will be finding the dimensions (length and width) and the minimum total cost for fencing the area for three different scenarios. In each case you should find the exact value of both dimensions and an approximation to the nearest foot, as well as the total cost for fencing the area (to the nearest dollar).
Answers
The minimum cost for fencing a rectangular area of 28,000 ft²:
Scenario 1: Metal Fencing only - $68,578 for 334 ft by 84 ft
Scenario 2: Wooden Fencing only - $71,162 for 237 ft by 118 ft
Scenario 3: Combination of Metal and Wooden Fencing - $64,141 for 300 ft by 93.3 ft, with 2,000 ft of metal and 13,000 ft of wooden fencing.
How to minimize fencing cost?To find the dimensions and the minimum cost for the fencing rectangular area of 28,000 ft² with metal or wooden fencing, we need to consider three different scenarios.
Scenario 1: Using only Metal Fencing
Let's assume the length of the rectangular area is L and the width is W. The total length of fencing required for this scenario is 2L + 2W. Since we have only metal fencing, the total cost for fencing would be $2 per ft times the total length of fencing required. Therefore, we have the following equation:
Total cost = $2(2L + 2W)
Now, we know that the area of the rectangular area is 28,000 ft². Therefore, we have another equation:
Area = L × W = 28,000
We can use this equation to find one variable in terms of the other, and then substitute it into the total cost equation to get a single variable equation. Let's solve for L:
L × W = 28,000
L = 28,000 ÷ W
Now, we can substitute this into the total cost equation:
Total cost = $2(2L + 2W)
Total cost = $2(2(28,000/W) + 2W)
Total cost = $4(14,000/W + W)
To find the minimum cost, we need to take the derivative of the total cost equation with respect to W and set it equal to zero:
d(Total cost)/dW = - $4(14,000/W²) + $4
$4(14,000/W²) + $4 = 0
W² = 14,000/2
W = √7,000 ≈ 83.7 ft (rounded to the nearest foot)
Now, we can use the area equation to find L:
L = 28,000/W ≈ 334.1 ft (rounded to the nearest foot)
Finally, we can substitute these values into the total cost equation to find the minimum total cost:
Total cost ≈ $68,578
Therefore, for Scenario 1, the dimensions of the rectangular area are approximately 334 ft by 84 ft, and the minimum total cost for fencing the area with metal fencing is approximately $68,578.
Scenario 2: Using only Wooden Fencing
We can follow the same approach as in Scenario 1, but this time we use the cost of wooden fencing, which is $5 per ft. The total cost equation becomes:
Total cost = $5(2L + 2W)
Setting the derivative of this equation with respect to W equal to zero, we get:
d(Total cost)/dW = - $10(14,000/W²) + $10 = 0
W² = 14,000
W = √14,000 ≈ 118.3 ft (rounded to the nearest foot)
Substituting this value into the area equation, we get:
L ≈ 236.7 ft (rounded to the nearest foot)
Finally, we can substitute these values into the total cost equation to find the minimum total cost:
Total cost ≈ $71,162
Therefore, for Scenario 2, the dimensions of the rectangular area are approximately 237 ft by 118 ft, and the minimum total cost for fencing the area with wooden fencing is approximately $71,162.
Scenario 3: Using both Metal and Wooden Fencing
In this scenario, we can use a combination of metal and wooden fencing to minimize the total cost. Let's assume that x ft of the perimeter is fenced with metal fencing, and the remaining 2L.
Learn more about dimensions
brainly.com/question/28688567
#SPJ11
In analyzing hits by certain bombs in a war, an area was partitioned into 574 regions, each with an area of 0.25 km2. A total of 535 bombs hit the combined area of 574 regions. Assume that we want to find the probability that a randomly selected region had exactly two hits. In applying the Poisson probability distribution formula, P(x)=
μx•e−μ
x!, identify the values of μ, x, and e. Also, briefly describe what each of those symbols represents.
Question content area bottom
Part 1
Identify the values of μ, x, and e.
Answers
x is the number of sucesses.
e = 2.71828 is the Euler number.
μ is the mean in the given interval.
The probability that a randomly selected region had exactly two hits is 0.1710.
How to identify the values of μ, x, and e and describe what each of those symbols represents?In a Poisson distribution, the probability that X represents the number of successes of a random variable is given by the following formula:
P(x)= (μ^x * e^-μ)/x!
Where
x is the number of sucesses
e = 2.71828 is the Euler number
μ is the mean in the given interval.
A total of 535 bombs hit the combined area of 574 regions. So the mean hits per region is:
P = 535/574 = 0.9321
Assume that we want to find the probability that a randomly selected region had exactly two hits (i.e P(x = 2)).
P(x) = (μ^x * e^-μ)/x!
P(2) = (0.9321^2 * e^-(0.9321))/2!
P(2) = 0.1710
Therefore, the probability that a randomly selected region had exactly two hits is 0.1710.
Learn more about Poisson distribution on:
https://brainly.com/question/14802212
#SPJ1
Point CC is located at (4,3)(4,3) on the coordinate plane. Point CC is reflected over the xx-axis to create point C'C ′ . Point C'C ′ is then reflected over the yy-axis to create point C''C ′′ . What ordered pair describes the location of C''?C ′′ ?
Answers
Answer:
\(C" = (-4,-3)\)
Step-by-step explanation:
Given
\(C = (4,3)\)
Required
The coordinates of C"
From the question, we understand that:
C is reflected over x-axis to give C'
The rule to this is:
\(C(x,y) \to C'(x,-y)\)
So, we have:
\(C(4,3) \to C'(4,-3)\)
Next, C' is reflected over y-axis to give C''
The rule to this is:
\(C'(x,y) \to C"(-x,y)\)
So, we have:
\(C'(4,-3) \to C"(-4,-3)\)
By multiplying 5/3^4 by _________, we get 5^4
Answers
The missing Value, x, that when multiplied by 5/3^4 gives the result of 5^4 is 13125.
The missing value that, when multiplied by 5/3^4, gives the result of 5^4, we can set up the equation:
(5/3^4) * x = 5^4
To solve for x, we can simplify both sides of the equation. First, let's simplify the right side:
5^4 = 5 * 5 * 5 * 5 = 625
Now, let's simplify the left side:
5/3^4 = 5/(3 * 3 * 3 * 3) = 5/81
Now we have:
(5/81) * x = 625
To solve for x, we can multiply both sides of the equation by the reciprocal of 5/81, which is 81/5:
(81/5) * (5/81) * x = (81/5) * 625
On the left side, the fraction (81/5) * (5/81) simplifies to 1, leaving us with:
1 * x = (81/5) * 625
Simplifying the right side:
(81/5) * 625 = 13125
Therefore, the missing value, x, that when multiplied by 5/3^4 gives the result of 5^4 is 13125.
For more questions on Value.
https://brainly.com/question/843074
#SPJ8
the number of ants in a colony doubles every month. which type of function best models this relationship?
Answers
The relationship described, where the number of ants in a colony doubles every month, can be modeled by an exponential function.
An exponential function is of the form y = ab^x, where 'a' is the initial value or starting point, 'b' is the growth factor, and 'x' represents the time or number of months in this case.
In the given scenario, the initial number of ants is multiplied by a growth factor of 2 every month. This corresponds to the base 'b' of the exponential function being equal to 2. The exponent 'x' represents the number of months elapsed.
Therefore, the exponential function y = 2^x best models the relationship between the number of ants in the colony and the time, as it reflects the doubling pattern observed in the colony's population over time.
Know more about exponential function here;
https://brainly.com/question/29287497
#SPJ11
Find the minimum value of
C=x+3y

Answers
Answer:
DNE
Step-by-step explanation:
If x and y may be any real number, there is no minimum value for C. It can approach negative infinity.
If C is a constant, and the domain of x is all real numbers, there is no minimum for y. It can approach negative infinity.
We're not sure what you want or what restrictions may exist. The given relation does not suggest any minimum. We'd have to say it Does Not Exist
Hello there,
Use the first constraint to isolate y :
\(9x+2y\geq 35\)
\(y \geq \frac{35-9x}{2}\)
Now use the second one to isolate x :
\(x+3y\geq 14\)
\(x\geq 14-3y\)
You know that \(x\geq 0\) and \(y\geq 0\)
So what's the minimum value of x it's 0 ! Same for y !
So substitute x by 0 and y by 0
\(y\geq \frac{35}{2} =17.5\)
\(x\geq 14\)
So the minimum value of C is :
\(C=14+3*17.5\)
\(\boxed{C=66.5}\)
Hope it helps !
Photon
lol!!!!
2×2=?
thank you
show your workings
Answers
Answer:
2×2=4
Step-by-step explanation:
please mark me brainliest
Answer:
here is ur answer
Step-by-step explanation:
2 x 2 = 4
hope it helps you
answer this question for me.

Answers
Answer: 5.7 (rounded)
Step-by-step explanation: The formula to find the diagonal of a square is \(\sqrt{2\) * a, where a is a side length on the square. Since we are solving for half of a diagonal, we use this formula and divide our result by two. We start by multiplying root 2 by 8 and we get 11.31371, next we divide by two and we get 5.656855 which is 5.7 when rounded.
What transformation has changed the parent function f(x) = (0.5)x to its new appearance shown in the graph? exponential graph passing through point negative 3, 2 and negative 2, 1 a f(x) − 2 b f(x + 2) c f(x) + 1 d −1 • f(x)
Answers
Correct option is A, f(x) -2 has changed the parent function f(x) = (0.5)x to its new appearance.
What modification has caused the parent function to change?The parent function f(x) = log5x has been modified by reflecting it over the x-axis, extending it vertically by a factor of three, and moving it down by three units.
In the picture below you can see the blue line is the graph of the function
f(x) = log(5x) and the green line is the graph of the function
g(x) = log[5(x + 4)] - 2
Since the function f passes at point (2, 1), we must reduce it by 2 units to ensure that it also passes at position (-2, -1). To do this, we add 2 to the function f.
We only need to add 4 to the variable x to have the function go left when we obtain log(5x) - 2.
The function shifts to the left when you add a number to x;
The function moves to the right when you remove a number from x;
The function increases when you add a number to it;
The function decreases when you take a number away from it.
Then we get a function g(x) = log[5 (x + 4)] - 2.
To learn more about parent function refers to;
brainly.com/question/12648375
#SPJ1

If Mrs. Manning chooses a student from her three classes at random, find each probability
Answers
The probability of selecting a boy in each of the three classes is: 0.125
What is the probability of selection?The probability of selection is simply the likelihood of selecting something out of a whole.
Now, in the class, there could be both boys and girls.
Now, since there are three classes and if we assume we have an equal number of boys and girls in each class, then we can say that:
Probability of a boy in class 1 = 0.5
Thus:
Probability of a boy in each of the three classes = 0.5 * 0.5 * 0.5
= 0.125
Read more about probability of selection at: https://brainly.com/question/251701
#SPJ1
Mario works at Bluebonnet Ice Cream parior.He earns $87.50 every 7 hours of work.Rachel works at Guadalupe Music Store and earns $39.75 for every 3 hours of work.How does the amount Mario earns per hour compare with the amount Rachel earns per hour?
Answers
Answer:
63
Step-by-step explanation:
g a spinning wheel on a playground with a radius of 3 feet rotates 6 revolutions every 7 seconds. find the linear speed in feet/second of a point on edge of the wheel
Answers
The linear speed of a point on the edge of the wheel is approximately 18.857 feet/second.
What is the linear speed in feet/second of a point on edge of the wheel?To find the linear speed of a point on the edge of the spinning wheel, we need to calculate the distance traveled by that point in one second.
Given:
- Radius of the wheel (r) = 3 feet
- Number of revolutions per second (n) = 6/7 revolutions/second
To convert revolutions per second to radians per second, we need to multiply the number of revolutions by 2π (the conversion factor between revolutions and radians).
n (in radians/second) = (6/7) * 2π
Now, to calculate the linear speed (v) at the edge of the wheel, we can use the formula:
v = r * n
Substituting the values:
v = 3 feet * (6/7) * 2π radians/second
Simplifying:
v ≈ 3 * (6/7) * 2 * 3.14 feet/second
v ≈ 18.857 feet/second
Learn more on linear velocity here;
https://brainly.com/question/15154527
#SPJ4
the population of a country dagne is 20 million in 1997 and increasing at a rate of 0.2 million per year. the average annual income of a person in nalgene during 1997 was 15000 dollars per year and increasing at a rate of 800 dollars per year. how quickly was the total income of the entire population rising in 1997? dollars per year
Answers
The total income of the entire population is rising at a rate of $18.16 billion per year in 1997.
In this problem, we are given the population of a country and its annual growth rate, as well as the average annual income of a person and its growth rate. We need to determine how quickly the total income of the entire population is rising in 1997.
First, let's calculate the total population growth in 1997:
Population in 1997 = 20 million
Population growth rate = 0.2 million per year
So, the population in 1998 will be:
Population in 1998 = 20 million + 0.2 million = 20.2 million
Now, let's calculate the average income growth in 1997:
Average income in 1997 = $15,000 per year
Income growth rate = $800 per year
So, the average income in 1998 will be:
Average income in 1998 = $15,000 + $800 = $15,800 per year
Next, we need to calculate the total income of the entire population in 1997:
Total income in 1997 = Population in 1997 x Average income in 1997
Total income in 1997 = 20 million x $15,000 = $300 billion per year
Now, let's calculate the total income of the entire population in 1998:
Total income in 1998 = Population in 1998 x Average income in 1998
Total income in 1998 = 20.2 million x $15,800 = $318.16 billion per year
Finally, we can determine how quickly the total income of the entire population is rising in 1997:
Total income growth rate = (Total income in 1998 - Total income in 1997) / 1 year
Total income growth rate = ($318.16 billion - $300 billion) / 1 year
Total income growth rate = $18.16 billion per year
To know more about average here.
https://brainly.com/question/16956746
#SPJ4
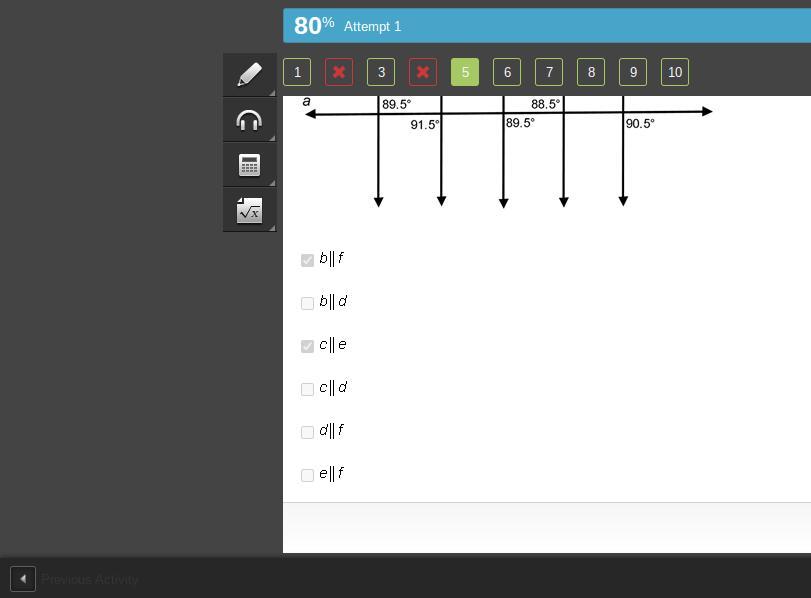
Which lines are parallel? Check all that apply.
Lines b, c, d, e, f are cut by transversal a. Clockwise from top left, the angles formed by lines b and a are blank, 89.5 degrees, blank, blank; by lines c and a are blank, blank, blank, 91.5 degrees; by lines d and a are blank, blank, 89.5 degrees, blank; by lines e and a are 88.5 degrees, blank, blank, blank; by lines f and a are blank, blank, 90.5 degrees, blank.
b is parallel to f
b is parallel to d
c is parallel to e
c is parallel to d
d is parallel to f
e is parallel to f

Answers
Answer:
I think it's A and C
b║f
c║e
(I'm so sorry if it's wrong)
Hope this Helps!
Answer:
A and c are correct because i just took the test
Step-by-step explanation:
PLEASE ANSWER THE FOLLOWING QUESTION GIVEN THE CHOICES!!!

Answers
Answer: 3/52
Step-by-step explanation:
You want to pick a diamond jack, diamond queen or diamond king
There are only 3 of those so
P(DJ or DQ or DK) = 3/52 There are 3 of those out of 52 total
cory ships two paperback books. one weighs 3/8 pound and the other weighs 3/4 pound. which weight is greater than 1/2 pound?
Answers
The book that weighs 3/4 pounds is greater than 1/2 pounds.
To compare the weights of the two books with 1/2 pound, we need to convert 1/2 pound into a fraction with a common denominator as the given weights. The common denominator of 8 and 4 is 8. So, we can convert 1/2 pound to 4/8 pound.
Now we can compare the weights of the two books with 4/8 pounds. The weight of the first book is 3/8 pounds, which is less than 4/8 pounds. The weight of the second book is 3/4 pounds, which is greater than 4/8 pounds.
Therefore, the book that weighs 3/4 pounds is greater than 1/2 pound.
Learn more about weight
https://brainly.com/question/2335828
#SPJ4
a house is 13 ft tall. its shadow is 20 ft long at the same time the shadow of a nearby building is 170 ft long. find the height of the building.
Answers
The height of the building after using proportion is 110.5 ft.
What is proportion ?
The main conceptual underpinnings on which proportion is described are ratio and fractions. When two ratios are written as a fraction using the formula a/b, ratio a:b, and then a proportion, they are equal. A and b in this situation can be any two integers. For grasping the myriad concepts in science and mathematics, the ratio and proportion are crucial constructing elements. With the right amount of proportion, a variety of problems in daily life, such as those encountered in the kitchen or in business when processing transactions, can be resolved. It facilitates comparison by establishing a relationship between two or more quantities.
Here House height = 13ft
Shadow of the house height = 20 ft
Shadow of the building height = 170ft
Noe using proportion ,
=> \(\frac{House height}{shadow of the house} = \frac{building height}{shadow of the building}\)
=> \(\frac{13}{20} = \frac{x}{170}\)
=> x=\(\frac{13*170}{20}\) =110.5 ft.
Hence the height of the building is 110.5 ft tall.
To learn more about proportion refer the below link
https://brainly.com/question/1496357
#SPJ4
an oil tank can take up to 1200 litres of oil
there are already 450 litres of oil in the tank
the price of oil is 81.5p per litre
mr Leonard gets 7.5% discount on the price of oil.
How much discount does he get?
give your answer in £.
Answers
Mr. Leonard receives a discount of £0.061125 on each litre of oil purchased.
Mr. Leonard receives a 7.5% discount on the price of oil. The price of oil is 81.5p per litre. To calculate the discount he receives in pounds, we need to find 7.5% of the total cost.
To begin, we convert the price of oil from pence to pounds by dividing by 100:
81.5p ÷ 100 = £0.815 per litre.
Next, we calculate the discount amount by multiplying the price per litre by the discount percentage:
£0.815 * 7.5% = £0.061125.
Therefore, Mr. Leonard receives a discount of £0.061125 on each litre of oil purchased.
Learn more about discount here
https://brainly.com/question/23865811
#SPJ11