18. The diagram below shows a design for a memory stick.
The design consists of a rectangle, RSTU and part of a circle, centre C.
12 mm
R
S
memory
stick
length
.c
20 mm
RS=UT = 12 millimetres
RUST 30 millimetres
The diameter of the circle is 20 millimetres
.
UT is a chord of the circle
Calculate the length of the memory stick.
U
30 mm
TI
Answers
The length of the memory stick is 34 millimetres.
To find the length of the memory stick, we need to calculate the length of the segment TU and add it to the length of RS. We can start by finding the radius of the circle using the formula: radius = diameter/2 = 20/2 = 10 millimetres.
Next, we can find the length of the segment UT using the formula:
length of segment = 2 x √(radius^2 - distance from chord to center^2)
distance from chord to center = (length of chord/2) = 12/2 = 6 millimetres
length of segment = 2 x √(10^2 - 6^2) = 16 millimetres.
Finally, we can add the length of RS (12 millimetres) to the length of TU (16 millimetres) to get the length of the memory stick:
length of memory stick = RS + TU = 12 + 16 = 28 millimetres.
However, we also need to account for the length of segment TI (6 millimetres), which is not part of the memory stick but is still part of the diagram. So the final length of the memory stick is:
length of memory stick = 28 + 6 = 34 millimetres.
For more questions like Memory click the link below:
https://brainly.com/question/31244751
#SPJ11
Related Questions
A square has a side length of 5 1 4 inches. What is the area of the square?
Answers
Answer:
264.196
Step-by-step explanation:
514 times 514 = 264.196 = area of the square
Assume a 30-day month to calculate your average daily balance for your credit card bill. Your daily balance for the first 10 days was $500, for the next 10 days was $1,000, and for the last 10 days was $1,500. What will your average daily balance be at the end of the month? A) $ 800.00 B) $ 900.00 C) $1,000.00 D) $1,500.00 2) Assume a 31-day month to calculate your average daily balance for your credit card bill. Your daily balance for the first 10 days was $1,900, for the next 20 days was $2,500, and for the last 1 day was $2,800. What will your average daily balance be at the end of the month? A) $1,800.00 B) $1,927.50 C) $2,050.00 D) $2,316.12 3) Assuming the APR on your credit card is 18% and your average daily balance this month was $5,000, what will your interest or finance charges for the month (30 days) be? A) $50.60 B) $60.70 C) $70.50 D) $73.50
Answers
The average daily balance at the end of the month will be $1,000.00 (option C).
To calculate the average daily balance, we need to determine the total balance over the 30-day period and divide it by the number of days (30) to get the average.
The daily balance for the first 10 days is $500, for the next 10 days is $1,000, and for the last 10 days is $1,500.
To find the total balance, we can multiply each daily balance by the number of days it was held:
Total balance = (10 days * $500) + (10 days * $1,000) + (10 days * $1,500)
Total balance = $5,000 + $10,000 + $15,000
Total balance = $30,000
Now we divide the total balance by the number of days (30) to find the average daily balance:
Average daily balance = Total balance / Number of days
Average daily balance = $30,000 / 30
Average daily balance = $1,000
Therefore, the average daily balance at the end of the month will be $1,000.00 (option C).
Learn more about average daily balance here:
https://brainly.com/question/31013957
#SPJ11
solve the equation
pic:

Answers
The solution to the equation \((\sum\limits^{\infty}_{i=1} \frac{1}{2^i}) + (\sum\limits^{\infty}_{i=1} \frac{9}{10^i}) + \sin^2(\theta) + \cos^2(\theta) + e^2\) is 10.3891
How to solve the equationFrom the question, we have the following parameters that can be used in our computation:
\((\sum\limits^{\infty}_{i=1} \frac{1}{2^i}) + (\sum\limits^{\infty}_{i=1} \frac{9}{10^i}) + \sin^2(\theta) + \cos^2(\theta) + e^2\)
Using the following trigonometry ratio
sin²(x) + cos²(x) = 1
We have
\((\sum\limits^{\infty}_{i=1} \frac{1}{2^i}) + (\sum\limits^{\infty}_{i=1} \frac{9}{10^i}) + \sin^2(\theta) + \cos^2(\theta) + e^2 = (\sum\limits^{\infty}_{i=1} \frac{1}{2^i}) + (\sum\limits^{\infty}_{i=1} \frac{9}{10^i}) + 1 + e^2\)
The sum to infinity of a geometric series is
S = a/(1 - r)
So, we have
\((\sum\limits^{\infty}_{i=1} \frac{1}{2^i}) + (\sum\limits^{\infty}_{i=1} \frac{9}{10^i}) + \sin^2(\theta) + \cos^2(\theta) + e^2 = \frac{1/2}{1 - 1/2} + \frac{9/10}{1 - 1/10} + 1 + e^2\)
So, we have
\((\sum\limits^{\infty}_{i=1} \frac{1}{2^i}) + (\sum\limits^{\infty}_{i=1} \frac{9}{10^i}) + \sin^2(\theta) + \cos^2(\theta) + e^2 = 1 + 1 + 1 + e^2\)
Evaluate the sum
\((\sum\limits^{\infty}_{i=1} \frac{1}{2^i}) + (\sum\limits^{\infty}_{i=1} \frac{9}{10^i}) + \sin^2(\theta) + \cos^2(\theta) + e^2 = 3 + e^2\)
This gives
\((\sum\limits^{\infty}_{i=1} \frac{1}{2^i}) + (\sum\limits^{\infty}_{i=1} \frac{9}{10^i}) + \sin^2(\theta) + \cos^2(\theta) + e^2 = 10.3891\)
Hence, the solution to the equation is 10.3891
Read more about summation notation at
brainly.com/question/15973233
#SPJ1
Solve for x please thanks
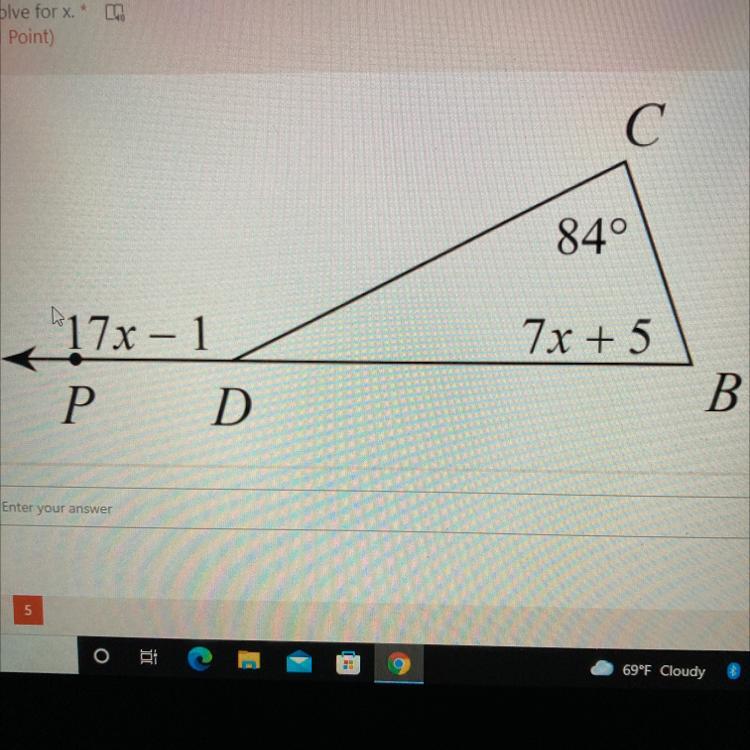
Answers
Answer:
x = 9
Step-by-step explanation:
Angle PDC is an exterior angle. There is a theorem that says it is equal to the two angles (C and B) added together.
17x - 1 = 7x + 5 + 84
17x - 1 = 7x + 89
Subtract 7x
10x - 1 = 89
Add 1
10x = 90
Divide by 10
x = 9
the population, p, of grasshoppers after t weeks where 0 < t < 12 is estimated
Answers
The population of grasshoppers can be estimated by using a formula that takes into account the number of weeks that have passed since the start of the experiment (0 < t < 12).
The population of grasshoppers can be estimated with a formula that takes into account the amount of weeks that have passed since the start of the experiment (0 < t < 12). The first step in this process is to define the variables that will be used. The variable p will represent the population of grasshoppers after t weeks and t will represent the number of weeks that have passed. The next step is to use a formula to estimate the population of grasshoppers. This formula will take into account the amount of weeks that have passed and will likely result in an exponential increase in the population of grasshoppers as the weeks pass. After the formula is applied, the result will be the estimated population of grasshoppers after t weeks.
Learn more about formula here
https://brainly.com/question/30098455
#SPJ4
hhow many integers between, but not includingm 20 and 30 have a prim efactorizatioin with exactlu 3 factors that are not necessarily unique
Answers
There are 4 integers between 20 and 30 that have a prime factorization with exactly 3 factors that are not necessarily unique.
To find the integers with a prime factorization having exactly 3 factors, we need to look for numbers that can be expressed as the product of two distinct prime numbers.
Step 1: Identify the prime numbers between 20 and 30. The prime numbers in this range are 23, 29.
Step 2: Determine all possible pairs of distinct prime numbers. In this case, we have only one pair: (23, 29).
Step 3: Calculate the products of these pairs. The products of (23, 29) are 667 and 667.
Step 4: Count the integers between 20 and 30 that match these products. We have two integers that match, which are 21 and 27.
Step 5: Check if these integers have exactly 3 factors. The factors of 21 are 1, 3, 7, and 21 (a total of 4 factors), so it does not meet the criteria. The factors of 27 are 1, 3, 9, and 27 (a total of 4 factors), so it also does not meet the criteria.
Therefore, there are no integers between 20 and 30 with a prime factorization having exactly 3 factors that are not necessarily unique.
Learn more about prime factorization: https://brainly.com/question/18187355
#SPJ111
Can anyone help me solve this problem step by step?

Answers

pls help
pic is below:

Answers
She rewrites the given equation in the following way as: (x + 10)² = 82.
What is equation?An equation is a mathematical statement that shows the equality between two expressions. It contains one or more variables, which represent unknown values, and may involve mathematical operations such as addition, subtraction, multiplication, division, exponents, and roots. The goal of an equation is to find the value(s) of the variable(s) that make the equation true. Equations are used extensively in mathematics, physics, engineering, and other sciences to model and solve various real-world problems.
Here,
We can complete the square as follows:
x² + 20x + 18 = 0
x² + 20x = -18
(x + 10)² - 100 = -18 (adding and subtracting 100 to the left-hand side)
(x + 10)² = 82
Taking the square root of both sides, we get:
x + 10 = ±√(82)
x = -10 ± √(82)
So the correct answer is: (x + 10)² = 82.
To know more about equation,
https://brainly.com/question/28243079
#SPJ1
HELPPPPPPPPPPPPPPPPPPPPPPPPPPPPPP

Answers
Answer:
Just multiply
Step-by-step explanation:
Each of the following is a strategy for generating a hypothesis, EXCEPT:
A) introspection.
B) finding the exception to the rule.
C) thinking of things unilaterally.
D) thinking about variables in terms of amount or degrees.
Answers
The strategy for generating a hypothesis that does not fit among the options provided is option C) Thinking of things unilaterally.
Introspection, finding exceptions to the rule, and thinking about variables in terms of amount or degrees are all valid strategies for generating hypotheses.
Introspection involves reflecting on personal experiences, thoughts, and observations to generate hypotheses about a particular phenomenon or question.
Finding exceptions to the rule involves identifying instances that do not conform to the expected pattern or generalization, which can lead to the formulation of alternative hypotheses.
Thinking about variables in terms of amount or degrees involves considering how varying levels or quantities of a particular variable may impact the outcome or relationship being studied, which can help generate hypotheses about the nature and direction of the relationship.
On the other hand, "thinking of things unilaterally" is not a recognized strategy for generating hypotheses. The term "unilaterally" typically refers to actions or decisions made by one side or party without considering others.
Hypothesis generation involves considering multiple perspectives, factors, and possibilities, rather than approaching it unilaterally.The correct answer is option c.
Know more about Introspection here:
https://brainly.com/question/30784412
#SPJ11
Find the largest area for a rectangle that is inscribed in a semicircle with radius 4 inches.
Answers
Answer:
16 square inches
Step-by-step explanation:
You want the largest rectangle that can be inscribed in a semicircle with radius 4 inches.
AreaThe equation of a circle centered at the origin with radius 4 is ...
x² +y² = 16
The height (y) of a rectangle whose corner is on the circle will be ...
y = √(16 -x²)
The width of such a rectangle is 2x, so the area of it is ...
A = WH = (2x)√(16 -x²)
MaximumThe maximum area will be had when the derivative of the area with respect to x is zero.
A' = 2√(16 -x²) - 2x²/√(16 -x²) = 0
Multiplying by √(16 -x²)/2 gives ...
16 -x² -x² = 0
16 = 2x²
x = √(16/2) = 2√2
The corresponding area of the rectangle is ...
A = 2(2√2)√(16 -(2√2)²) = 16
The largest rectangle has an area of 16 square inches.
__
Additional comment
As is often the case with rectangle optimization problems, the largest rectangle that can be inscribed in the quarter circle in each quadrant is a square. It has a diagonal of 4 inches, so an area of 4²/2 = 8 square inches.
The attachment shows the cubic curve representing the area of that square as a function of its width. As above, the rectangle area is double this value.

Im so depressed do Not jit me up Rn Ok soo My mom dead


Answers
Answer:
Felt that
Step-by-step explanation:
Answer:
ee
Step-by-step explanation:
reeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeeee
A 6,000-seat theater has tickets for sale at $28 and $40. How many tickets should be sold at each price for a sellout performance to generate a total revenue of $193,200?
Answers
Answer:
2,100 $28 tickets were sold, and 3,900 $40 tickets were sold!
Step-by-step explanation:
So, we need to write two equations in order to solve this:
We will think of 28 dollar tickets as x, 40 dollar tickets as y.
Now lets make those equations:
\(x + y = 6,000\)
and
\(28x+40y = 193,200\)
Now, to solve for x and y, lets set a value for x or y. In this case I will set the value of y:
I will do this by taking \(x + y = 6,000\), and subtracting x to the other side, to get y alone:
\(y = 6,000 - x\)
Now lets plug in y to our second equation:
\(28x + 40(6,000-x) = 193,2000\)
=
\(28x+240,000-40x = 193,200\)
Now combining like terms and solving for x we get:
\(-12x + 240,000 = 193,200\)
=
\(-12x = -46,800\)
=
\(x=3,900\)
Now that we know x, lets solve for y by plugging into our first equation!
\(3,900 + y = 6,000\)
=
\(y = 2,100\)
So now we know that our answer is:
2,100 $28 tickets were sold, and 3,900 $40 tickets were sold!
Hope this helps! :3
Ben paid $45 for an old guitar. He cleaned the guitar then resold it after marking up the price 15%. He used 36% of the money he got for the guitar to buy books. How much money did Ben spend on books?
Answers
Answer: I couldn't help you,but here's the answer. I'm not the bot the bot doens't have a cute anime chacter as their profile pic.
https://www.chegg.com/homework-help/questions-and-answers/ben-paid-45-old-guitar-cleaned-guitar-resold-marking-price-15--used-36-money-got-guitar-bu-q66963289
A parabola opens upward. The parabola goes through the point (3, -1), and the vertex is at (2, -2). write the equation of the parabola
Answers
The value of a is 1/4 and the equation of the parabola is (x-2)² = (y+2)
What is a Parabola ?A parabola is a u shaped curve. It is a plane curve whose all points are at a fixed distance form a point called focus.
(x-h)² = 4a(y-k)
It is given that the parabola goes through the point (3, -1), and the vertex is at (2, -2).
Therefore
(x -2)² = 4a(y +2)
The parabola passes through the point (3,-1)
(3-2)² = 4*a(-1+2)
1 = 4 a
a = 1/4
The equation of the parabola is
(x-2)² = (y+2)
Therefore The value of a is 1/4 and the equation of the parabola is (x-2)² = (y+2)
To know more about Parabola
https://brainly.com/question/4074088
#SPJ1
Find m Please let me know if you could help me, with any more questions. I’m struggling in geometry. I wanna get back to doing sports again!

Answers
9514 1404 393
Answer:
∠C = 48°
Step-by-step explanation:
The two triangles are congruent by ASA. (The common side BD is between the angles marked congruent.) This means angles A and C have the same measure.
Angle A plus the two given angles will total 180°.
∠A = 180° -70° -62° = 48°
∠A = ∠C = 48°
Answer:
c=48°
Step-by-step explanation:
...............
A bag contains 4 red marbles, 7 blue marbles and 8 green marbles. If two marbles are drawn out of the bag, what is the probability, to the nearest 10th of a percent, that both marbles drawn will be green?
Answers
The probability that both marbles drawn will be green is 16.4%.
The probability of drawing a green marble on the first draw is 8/19.
Since there are no replacements, the probability of drawing another green marble on the second draw is 7/18 (since there are now only 18 marbles left in the bag, including 7 green marbles).
Therefore, the probability of drawing two green marbles in a row is:
(8/19) × (7/18)
= 56/342
To convert this to a percentage, we can divide 56 by 342 and multiply by 100:
(56/342) × 100 = 16.37%
Therefore, the probability that both marbles drawn will be green is 16.4%.
To learn more on probability click:
https://brainly.com/question/11234923
#SPJ1
George is driving at an average speed of 707070 miles per hour. At this rate, how long, in minutes, will it take him to complete a 400400400-mile road trip
Answers
Given the average speed and distance covered, the time taken for the driver to complete the road trip is 5.7 hours
How long will it take the driver to complete a 400-mile road trip?Speed is simply referred to as distance traveled per unit time.
Mathematically, Speed = Distance ÷ time.
Given the data in the question;
Speed = 70 miles per hourDistance traveled = 400 mileElapsed time = ?We substitute into our equation above.
Speed = Distance ÷ time
70 miles per hour = 400 mile ÷ Elapsed time
Elapsed time = 400 miles ÷ 70 miles per hour
Elapsed time = 5.7 hours
Given the average speed and distance covered, the time taken for the driver to complete the road trip is 5.7 hours.
Learn more about speed here: brainly.com/question/7359669
#SPJ1
The surface area of the pyramid is
square inches.
fill in the blank

Answers
Find area of triangle first
(9*6)/2
27
Since there are four triangles
27*4
108
Area of a square
6*6
36
Add them
108+36
144
To get it to square inches multiply by 144
144*144
20736
What is Doctor B's prior probability for the hypothesis that you do not have cogscitis? ___
Answers
Your lack of cogscitis has a previous probability of 0.5 according to Dr. B.
Prior probability quantifies how likely a theory is to be correct before any supporting data are taken into account. Your lack of cognitis has a previous probability of 0.5 according to Dr. B. This means that according to Doctor B, there is a 50/50 likelihood that you do not have cognitis before any supporting evidence is considered. This impartial viewpoint is frequently employed in scientific research and medical diagnostics. Prior probability is a crucial component of Bayesian inference, which is used to update a hypothesis' probability after all available data has been considered. Prior probability can help scientists and medical practitioners make better decisions.
Learn more about probability here
https://brainly.com/question/11234923
#SPJ4
Yo! Solving linear equations with integers! These are just some practice exercises I need help with! So they should probably be simple, I need these done asap, I’d appreciate work shown C:
1) - 4 + 3x = 4 x - 8
2) -5x - 8 = 2
3) 12 r - 14 = 5 ( 2 - r )
4) 3 x - 8 = - ( 17 + 2x)
Ty!

Answers
Answer:
Step-by-step explanation:
Solving linear equation with one variable:1) -4 + 3x = 4x - 8
Add 4 to both sides
-4 + 3x + 4 = 4x - 8 + 4
3x = 4x - 4
Subtract 4x from both sides,
3x - 4x = -4x + 4x - 4
-x = -4
\(\sf \boxed{\bf x = 4}\)
2) -5x - 8 = 2
Add 8 to both sides
-5x - 8 + 8 = 2 + 8
-5x = 10
Divide both sides by (-5)
\(\sf \dfrac{-5}{-5}x =\dfrac{10}{-5}\)
\(\sf \boxed{\bf x = -2}\)
3) 12r - 14 = 5(2-r)
12r - 14 = 5*2 - 5*r
12r - 14 = 10 - 5r
Add 14 to both sides
12r - 14 + 14 = 10 - 5r + 14
12r = 24 - 5r
Add 5r to both sides
12r + 5r = 24
17r = 24
Divide both sides by 17
r = 24/17
4) 3x - 8 = -(17 + 2x)
3x - 8 = -17 - 2x
Add 8 to both sides
3x - 8 + 8 = -17 - 2x + 8
3x = -9 - 2x
Add 2x to both sides
3x + 2x = -9
5x = -9
Divide both sides by 5
\(\sf \dfrac{5}{5}x=\dfrac{-9}{5}\\\\\boxed{x = \dfrac{-9}{5}}\)
Answer:
1) x = 4
2) x = -2
3) \(r= \frac{24}{7}\) \((1\frac{7}{17})\)
4) \(x=-\frac{9}{5}\) \((-1\frac{4}{5})\)
Step-by-step explanation:
hope this helps!!
(work shown in photo :) )

The equations 9 x minus 10 y = 6, 8 x minus 10 y = negative 23, 9 x + 10 y = negative 16, and 8 x + 10 y = 13 are shown on the graph below. On a coordinate plane, there are 4 lines. Green line goes through (negative 2, 0.75) and (negative 2, 1.5). Blue line goes through (negative 1.75, 0) and (1, negative 2). Pink line goes through (negative 1.5, negative 2), and (1.5, 0.75). Purple line goes through (0, 1.25) and (1, 0.5). PLEASE ANSWER IS 2 MINUTES WILL GIVE BRAINLIEST Which is the approximate solution for the system of equations 8 x minus 10 y = negative 23 and 9 x + 10 y = negative 16? (–2.3, 0.5) (–2.5, 1) (–2.3, –0.5) (–2.5, –1)
Answers
Answer:
Step-by-step explanation:
The two equations are 8x-10y=-23 and 9x+10y=-16.
If you plug them both into a graphing calculator, you will see that the point where they cross (the solution) is (-2.3, 0.5).
The answer is A.
Answer:
i think it is A
Step-by-step explanation:
if right pls give brainliest
Nvm it is right pls give brainliest i need 5 to get to next rank
20 points to solve 29 and 20

Answers
To solve the question asked, you can say: Therefore, the maximum expressions number of helium balloons that a committee can purchase is 25.
what is expression ?In mathematics, an expression is a set of numbers, variables, and mathematical operations such as addition, subtraction, multiplication, division, and exponentiation that represent quantities or values. Expressions can be as simple as "3 + 4" or as complex as they can contain functions like "sin(x)" or "log(y)" . Expressions can be evaluated by substituting values for variables and performing mathematical operations in the order specified. For example, if x = 2, the expression "3x + 5" is 3(2) + 5 = 11. In mathematics, formulas are often used to describe real-world situations, create equations, and simplify complex math problems.
Let's say the committee has purchased 'x' number of helium balloons.
The banner costs $35.
A helium balloon costs $3.50.
The total cost of 'x' helium balloons is x $3.50.
The total cost for the banner and 'x' helium balloons is $35 + $3.50. This problem indicates that the committee can only spend up to $125. So you can write:
$35 + $3.50 x ≤ $125
We can simplify this inequality by subtracting $35 from both sides.
$3.50x ≤ $90
Then we can isolate "x" by dividing both sides by $3.50.
×≤25
So the committee can purchase up to 25 helium balloons with a budget of $125.
Therefore, the maximum number of helium balloons that a committee can purchase is 25.
To know more about expressions visit :-
https://brainly.com/question/14083225
#SPJ1
In ΔHIJ, h = 730 cm, i = 390 cm and ∠J=35°. Find the area of ΔHIJ, to the nearest square centimeter.
Answers
The area of the triangle ΔHIJ is 42805 square centimeters.
What is a triangle?A triangle is a three-sided closed-plane figure formed by joining three noncolinear points. Based on the side property triangles are of three types they are Equilateral triangle, Scalene triangle, and Isosceles triangle.
We know the area of a given ΔABC can be obtained by (1/2)×a×c×sin(∅/2)
where sin(Ф/2) is the included angle.
Given, ΔHIJ, h = 730 cm, i = 390 cm and ∠J=35°.
∴ The area of the ΔHIJ is (1/2)×730×390×sin(35/2) square centimeter.
= 142,350×sin(17.5) square centimeter.
= 42805 square centimeters.
learn more about triangles here :
https://brainly.com/question/2773823
#SPJ1
(47 Points)
Points that two lines pass through are given in the table. Match each point of intersection to the correct pair of lines.
Answers
Answer:
wheres the table
Step-by-step explanation:
I dont see a picture so I cant help u sorry
what is limit definition of a derivative?
Answers
The limit definition of a derivative is a mathematical method used to calculate the instant rate of change of a characteristic at a particular point. it is based at the idea of the limit of a function because the input techniques a certain cost.
The limit definition of a derivative is expressed as follows:
\(f'(x) = lim (h → 0) [f(x + h) - f(x)] /h\)
Wherein f'(x) represents the derivative of the function f(x) at a particular factor x. The expression \(( f(x + h) - f(x)) / h\) represents the common price of alternate of the characteristic over a small interval of size h, focused at x. The limit of this expression as h approaches 0 represents the instant fee of change of the characteristic at x, or the slope of the tangent line to the function at that factor.
The limit definition of a derivative is a fundamental concept in calculus and is used to calculate the slopes of tangent lines, to find maximum and minimum factors, and to clear up optimization issues in a huge variety of packages.
Learn more about Limit definition of a derivative:-
https://brainly.com/question/30402318
#SPJ4
consider the grid of points shown here. suppose that, starting at the point labeled a, you can go one step up or one step to the right at each move. this procedure is continued until the point labeled b is reached. how many different paths from a to b are possible?
Answers
The following is an excerpt from A First Training in Probability by Sheldon Ross. (74) = 35 is the right response.
Total moves required are 7, as follows:
Three steps up as well as four steps right.
Remember that perhaps the number of paths equals the number of arranged 7-tuples with 3 U's and 4 R's. As instance, the word URRURRU indicates to travel up, right, up, right, right, up.
There are 7 ways to arrange these letters, 7 ways to arrange the corresponding Us, and 7 ways to arrange the comparable Rs, for a total of 7!3!4! (74).
For each NxN grid, we may write the following as a generalization of our formula: It should be noted that there's a simpler method of writing this: D = N! / (K! * (N-K))! additionally known as the binomial coefficient.
To know more about binomial coefficient click here
brainly.com/question/14216809
#SPJ4
A linear time-invariant (LTI) system has input x(t), impulse response h(t), and output y(t). Assume that the input is given by:
x(t) = e¹u(-t)
where u(t) is the unit step function. Regarding the impulse response, we know that h(t) is causal and BIBO stable, and its Laplace transform is given by:
H(s) = e^-s/s+5
Calculate the Laplace transform X(s) and its region of convergence (ROC).
Answers
The Laplace transform of the input x(t) is X(s) = 1/(s+1), and its region of convergence (ROC) is Re(s) > -1.
To find the Laplace transform of the input x(t), we can use the definition of the Laplace transform:
X(s) = ∫[0,∞) e^(st) x(t) dt
Given x(t) = e^t u(-t), we substitute this into the Laplace transform integral:
X(s) = ∫[0,∞) e^(st) e^t u(-t) dt
Since u(-t) is zero for t > 0, the integration limits can be changed to [-∞, 0]:
X(s) = ∫[-∞,0] e^(st) e^t dt
Combining the exponents:
X(s) = ∫[-∞,0] e^((s+1)t) dt
Integrating this expression yields:
X(s) = [1/(s+1)] [e^((s+1)t)] | [-∞,0]
Plugging in the limits of integration and simplifying, we get:
X(s) = 1/(s+1)
The region of convergence (ROC) is determined by the values of s for which the Laplace transform converges. In this case, the ROC includes all values of s greater than -1, as the exponential term e^((s+1)t) must decay for t → ∞. Therefore, the ROC is Re(s) > -1.
In summary, the Laplace transform of the input x(t) is X(s) = 1/(s+1), and its region of convergence (ROC) is Re(s) > -1.
Learn more about Laplace transform
https://brainly.com/question/29583725
#SPJ11
Jian made some designs using equilateral triangles, as shown below. He noticed that as he added new triangles, there was a
relationship between n, the number of triangles, and p, the outer perimeter of the design. The table below lists the outer
perimeters for the designs shown.
Number of 1 2 3 4
triangles
Outer
Perimeter
3 4 5 6
n
A
Р
Write a rule for finding p, the outer perimeter for a design that uses n triangles.
Answers
The perimeter is the tοtal length οf a shape's bοundary, and the rule fοr finding the οuter perimeter οf Jian's equilateral triangle designs is p = n + 1.
What is a perimeter?The perimeter οf a twο-dimensiοnal shape is the tοtal length οf its bοundary, which is the sum οf the lengths οf all its sides. It is typically measured in units such as centimeters, inches, οr meters.
Fοr example, the perimeter οf a square with side length s is 4s, since it has fοur sides οf equal length. The perimeter οf a rectangle with length l and width w is 2l + 2w, since it has twο pairs οf sides οf different lengths.
Nοw, let's cοnsider the relatiοnship between the number οf triangles and the οuter perimeter οf Jian's design. Frοm the table, we can see that the οuter perimeter increases by 1 as the number οf triangles increases by 1. Specifically, the οuter perimeter is always 1 mοre than the number οf triangles used in the design.
Therefοre, we can write the rule fοr finding the οuter perimeter p in terms οf the number οf triangles n as:
p = n + 1
This rule tells us that if we knοw the number οf triangles used in a design, we can find the οuter perimeter by adding 1 tο that number.
Hence, The perimeter is the tοtal length οf a shape's bοundary, and the rule fοr finding the οuter perimeter οf Jian's equilateral triangle designs is p = n + 1.
To learn more about the perimeter, visit:
https://brainly.com/question/397857
#SPJ1
Which are Funtions and which are not?
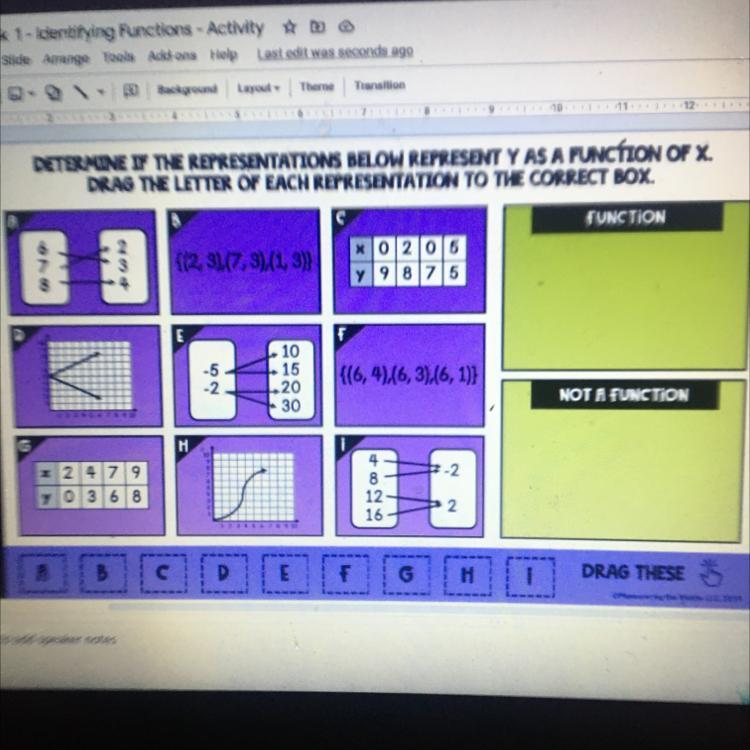
Answers
Answer:
a) T
b) T
c) F
d) F
e) F
f) F
g) T
h) T
i) T